2 box plots. The number line goes from 55 to 105. For pitcher 1, the whiskers range from 55 to 105, and the box ranges from 85 to 95. A line divides the box at 94. For pitcher 2, the whiskers range from 71 to 106, and the box ranges from 78 to 102. A line divides the box at 90.
Which pitcher had speeds that were the most consistent?
Pitcher 1, because the median is greater than that of pitcher 2.
Pitcher 2, because the median is greater than that of pitcher 1.
Pitcher 1, because he has the lesser amount of variability in his pitches.
Pitcher 2, because he has the greater amount of variability in his pitches.
Answers
Pitcher 1 had speeds that were the most consistent because he has the lesser amount of variability in his pitches.
What is speed?
Speed is a measure of how quickly something moves or how quickly a task is completed. In physics, speed is defined as the distance traveled by an object per unit of time. In everyday life, speed can refer to how quickly a person can run, how fast a car can drive, or how quickly a computer can process data.
Speed is an important concept in many fields, including sports, transportation, and technology. In sports, athletes strive to improve their speed to run faster, jump higher, or throw farther. In transportation, engineers design vehicles to be faster and more efficient, while also ensuring safety. In technology, faster processing speeds allow for more efficient and powerful computers, smartphones, and other devices.
Here,
Pitcher 1 had speeds that were more consistent because the range of his speeds is smaller, with a box ranging from 85 to 95, while pitcher 2 had a larger range, with a box ranging from 78 to 102. Additionally, the median of pitcher 1 is higher than that of pitcher 2, which only indicates a difference in the central tendency, not the consistency of the data.
Therefore, the correct answer is: Pitcher 1, because he has the lesser amount of variability in his pitches.
Learn more about speed here,
https://brainly.com/question/26046491
#SPJ1
Related Questions
given 3 nd fdn fdsfasap..................................................enfefejnfjnsjfdnkdsnfkdnfkdsjnfsk
Answers
Answer:
WDym??
xDStep-by-step explanation:
Triangle ABC was transformed to create triangle DEF.
2 triangles are shown. Triangle A B C has point A at the top, B on the bottom right, and point C on the bottom left. Triangle D E F has point D at the top, E on the bottom right, and F on the bottom left. The triangles are identical.
Which statement is true regarding the side in the image that corresponds to Side length B A?
Side length B C corresponds to Side length B A because they are about the same length.
Side length E D corresponds to Side length B A because they are in the same position.
Side length E F corresponds to Side length B A because the transformation is isometric.
Side length F D corresponds to Side length B A because the length is not preserved.
Answers
Answer: Side length E D corresponds to Side length B A because they are in the same position.
Step-by-step explanation:
Answer: b
Step-by-step explanation:
Competition time!
How do you make a Peanut butter and jelly sandwich?
Whoever gives the best answer gets brainiest and a thx and 5 stars.
Second place gets 4 stars and a thx!
Answers
Answer:
For each sandwich, spread 2 tablespoons peanut butter onto 1 slice of bread. Spread 1 tablespoon jelly onto another slice of bread.
Heat 12-inch skillet or griddle over medium heat.
Place 2 sandwiches into skillet. Cook, turning once, 4-6 minutes or until golden brown and peanut butter is melted.
Step-by-step explanation:
Kimberly rolls two six-sided number cubes numbered 1 through 6 and adds up the two numbers construct a tree diagram to determine all the possible outcomes list the sum at the end of each branch of the tree
Answers
When Kimberly rolls two six-sided number cubes numbered 1 through 6, it creates 36 possible outcomes which is represent in the tree diagram below
What is a tree diagram?A tree diagram is a visual representation of outcomes. It consists of branches that represent the possible outcomes of each step.
When it comes to Kimberly rolling two six-sided number cubes, we can start by rolling the first cube, and then rolling the second cube.
For each roll of the first cube, there are six possible outcomes (1 to 6). For each outcome of the first cube, there are six possible outcomes for the second cube.
This results in a total of 6 x 6 = 36 possible outcomes.
Find more exercises on tree diagrams;
https://brainly.com/question/13311154
#SPJ1

Find the length of the longer diagonal of this parallelogram.
AB= 4FT
A= 30°
D= 80°
Round to the nearest tenth.
Answers
The length of the longer diagonal of the parallelogram is approximately 5.1 ft.
We have,
To find the length of the longer diagonal of the parallelogram, we can use the law of cosines.
The law of cosines states that in a triangle with side lengths a, b, and c, and angle C opposite side c, the following equation holds true:
c² = a² + b² - 2ab * cos(C)
In this case, we have side lengths AB = 4 ft and angle A = 30°, and we want to find the length of the longer diagonal.
Let's denote the longer diagonal as d.
Applying the law of cosines, we have:
d² = AB² + AB² - 2(AB)(AB) * cos(D)
d² = 4² + 4² - 2(4)(4) * cos(80°)
d² = 16 + 16 - 32 * cos(80°)
Using a calculator, we can calculate cos(80°) ≈ 0.1736:
d² = 16 + 16 - 32 * 0.1736
d² ≈ 16 + 16 - 5.5552
d² ≈ 26.4448
Taking the square root of both sides, we find:
d ≈ √26.4448
d ≈ 5.1427 ft (rounded to the nearest tenth)
Therefore,
The length of the longer diagonal of the parallelogram is approximately 5.1 ft.
Learn more about parallelograms here:
https://brainly.com/question/1563728
#SPJ12
Which equation describes the graph?

Answers
Dina added five-sixths of a bag of soil to her garden. Her neighbor Natasha added eleven-eighths bags of soil to her
garden. How much more soil did Natasha add than Dina?
Answers
Answer:
Natasha added 13/24ths more than Dina.
Step-by-step explanation:
By getting common denominators you have 66/48-40/48 then you get 26/48 and you just simplify this by 2 to get 13/24ths.
Love you, hope this helps.
2. It takes 6 eggs to make 2 loaves of banana bread. How many eggs are needed to make 1 loaf of
bread?
Answers
Answer:
3
Step-by-step explanation:
Answer:3
Step-by-step explanation: because half of 6 eggs are need to make 2 loaves so split that in half and you have 3
A business school is preparing an informational booklet for entering graduate students. Part of this material is a report on how various factors influence a student's GPA (grade point average). In particular, the school used a multiple regression model to study the relationship between y= graduate GPA and several independent variables x1, x2,...,x8. If data from 166 current graduate students are used to develop the regression model, what are the degrees of freedom (df) for the regression sum of squares, the error sum of squares, and the total sum of squares?
df for the regression sum of squares =
df for the error sum of squares =
df for the total sum of squares =
Answers
The degrees of freedom (df) for the regression sum of squares, the error sum of squares, and the total sum of squares can be calculated using the following formula:
df = n - k - 1
where n is the sample size and k is the number of independent variables in the regression model.
Since the model includes 8 independent variables, k = 8. The sample size is given as n = 166.
Therefore, the degrees of freedom for the regression sum of squares, the error sum of squares, and the total sum of squares are:
df for the regression sum of squares = k = 8
df for the error sum of squares = n - k - 1 = 166 - 8 - 1 = 157
df for the total sum of squares = n - 1 = 166 - 1 = 165
Hence, the degrees of freedom for the regression sum of squares, the error sum of squares, and the total sum of squares are 8, 157, and 165, respectively
Learn more about Regression Model here:
brainly.com/question/14983410
#SPJ11
everyone please help me show ur solutions

Answers
Step-by-step explanation:
FCP fundamental counting principle :
in total there are
3×3×2 = 18
different combinations
listing method means listing all different triplets of such outfit combinations.
white shirt = ws
red blouse = rb
floral shirt = fs
short = sh
jeans = j
jogger pants = jp
shoes = s
sandals = sa
{
(ws, sh, s),
(ws, sh, sa),
(ws, j, s),
(ws, j, sa),
(ws, jp, s),
(ws, jp, sa),
(rb, sh, s),
(rb, sh, sa),
(rb, j, s),
(rb, j, sa),
(rb, jp, s),
(rb, jp, sa),
(fs, sh, s),
(fs, sh, sa),
(fs, j, s),
(fs, j, sa),
(fs, jp, s),
(fs, jp, sa)
}
tree diagram (I cannot draw here) :
OOTD
/ | \
ws rb fs
/ | \ / | \ / | \
sh j jp sh j jp sh j jp
/ | / | / | / | / \ / | / | / | / |
s sa s sa s sa s sa s sa s sa s sa s sa s sa
as you can see, we get a expected 18 different triplets in our listed set, and we get 18 different end leaves in and therefore 3-level paths through our tree.
(1 point) a street light is at the top of a 16 foot tall pole. a 6 foot tall woman walks away from the pole with a speed of 4 ft/sec along a straight path. how fast is the tip of her shadow moving when she is 30 feet from the base of the pole?
Answers
The tip of the shadow is moving at \(4.8\frac{ft}{sec}\)
Consider the image , as depicted below
Based on similar triangle , we have
\(\frac{s(t)}{6} =\frac{s(t)+w(t)}{16}\)
⇒ 10s(t) = 6w(t)
⇒ w(t) = \(\frac{10}{6} s(t)\)
we are told that a certain time t,
\(\frac{d(w(t))}{dt} = 8\frac{ft}{sec}\)
\(\frac{d(w(t))}{dt} = \frac{d(\frac{10}{6}.s(t)) }{dt}\)
\(= \frac{10}{6}\frac{d(s(t))}{dt}\)
Therefore,
\(\frac{10}{6} \frac{d(s(t))}{dt} = 8\frac{ft}{sec}\)
\(\frac{d(s(t))}{dt} = 4.8\frac{ft}{sec}\)
Hence, The tip of the shadow is moving at \(4.8\frac{ft}{sec}\)
Learn more about Implicit Differentiation at
https://brainly.com/question/11887805
#SPJ4

y < x² - 2x
find the vertex
Answers
The vertex of the equation is (1, -1).
The equation of a parabola in vertex form is,
y = a(x-h)² + k
The coordinates of the vertex are (h,k) respectively and a is a constant.
Given:
y = x² - 2x
Rearrange the given equation in the form of the equation of the parabola in vertex form using the method of completing the square.
y = (x² - 2x + 1) - 1
y = (x - 1)² - 1
Therefore, the vertex of the equation is (1, -1).
To know more about equation of a parabola in vertex form visit:
https://brainly.com/question/20333425
#SPJ13
Given: Q = 7m 3n, R = 11 - 2m, S = n 5, and T = -m - 3n 8. Simplify R - S T. M - 2n - 2 -3m - 4n 14 3m - 4n 14 -m 2n - 2.
Answers
To solve the algebraic expression, add or subtract the variables with same power of variable.
The result of the given problem after simplifying is,
\(y=7m+n-6\)
How to write algebraic expression?Algebraic expression are the expression which consist the variables, coefficients of variables and constants.
The algebraic expression are used represent the general problem in the mathematical way to solve them.
To solve the algebraic expression, add or subtract the variables with same power of variable.
Given information-
The given numbers are,
\(Q=7m+3n\\R=11-2m\\S=n+5\\T=-3-3n\)
All the four number given in the form of unknown number \(m\) and \(n\).
The result, need to be find is,
\(R-S+T+M\)
Let the result of above expression is \(y\). Thus,
\(y=R-S+T+M\)
Put the values to solve it further,
\(y=(7m+3n)-(11-2m)+(n+5)+(-m-3n)\)
Solve it further by open the bracket as,
\(y=7m+3n-11+2m+n+5-m-3n\\y=7m+2m-m+3n+n-3n-11+5\\y=7m+n-6\)
Hence the result of the given problem after simplifying is,
\(y=7m+n-6\)
Learn more about the algebraic expression here;
https://brainly.com/question/2164351
i need help please answer correctly

Answers
6. Applying the inscribed angle theorem, x = 33
7. m<ABP = 90°.
m<APB = 48°
What is the Tangent Theorem?The tangent theorem states that the tangent of a circle is perpendicular to the radius of that circle and therefore forms a right angle at the point of tangency.
What is the Inscribed Angle Theorem?An inscribed angle has a measure that is half the measure of the intercepted arc, according to the inscribed angle theorem.
Therefore:
Question 6:
Based on the inscribed angle theorem,
Measure of arc AB = 2(x + 3) = 2x + 6
108 + 2x + 6 = 180 [semicircle]
Solve for x
114 + 2x = 180
2x = 180 - 114
2x = 66
x = 33
Question 7:
Given that line PB is tangent to circle A, therefore, based on the tangent theorem, the measure of angle ABP = 90°.
m<APB = 180 - 90 - 42
m<APB = 48°
Learn more about the tangent theorem on:
https://brainly.com/question/9892082
#SPJ1
you roll two six-sided dice at the same time. what is the probability the second dice landed on an even number given the first one landed on an odd number?
Answers
The probability that the second dice lands on an even number given that the first one landed on an odd number is 1/2 or 0.5.
Step-by-Step Explanation:Let A be the event that the first dice rolls an odd number, and let B be the event that the second dice rolls an even number. We want to find P(B|A), which is the probability of B given A. This can be found using the formula:P(B|A) = P(A and B) / P(A) We know that P(A) = 1/2, as half the numbers on a six-sided dice are odd.
To find P(A and B), we need to find the probability that both dice roll the desired numbers simultaneously. Since we know that the first dice rolled an odd number, only half of the numbers are possible for the second dice to be even. Therefore, P(A and B) = 1/2 x 1/2 = 1/4. So:P(B|A) = P(A and B) / P(A) = (1/4) / (1/2) = 1/2 or 0.5. Therefore, the probability that the second dice lands on an even number given that the first one landed on an odd number is 1/2 or 0.5.
See more about probability at: https://brainly.com/question/13604758
#SPJ11
Find the length of the curve. The spiral r=4θ^2, 0≤θ≤2√3.
Answers
The length of the curve r = 4θ², 0≤θ≤2√3, is 38.786 units.
To find the length of the curve, we can use the formula for arc length:
\(L = \int_{a}^{b}\sqrt{(1 + (dy/dx)^2)} dx\)
In this case, we have the polar equation r = 4θ², which we can convert to Cartesian coordinates using x = r cos(θ) and y = r sin(θ):
x = 4θ² cos(θ)
y = 4θ² sin(θ)
To find dy/dx, we can use the chain rule:
dy/dx = (dy/dθ)/(dx/dθ)
= (4θ² cos(θ) + 8θ sin(θ))/(8θ cos(θ) - 4θ² sin(θ))
Simplifying this expression, we get:
dy/dx =(4θ) (θ cos(θ) + 2 sin(θ))/(2 cos(θ) - θ sin(θ))
Now we can substitute this expression and the expression for x into the arc length formula:
\(L = \int_{0}^{2\sqrt{3}}\sqrt{(1 + ((4\theta)(\theta cos(\theta) + 2sin(\theta))/(2cos(\theta) - \theta sin(\theta)))^2)} d\theta\)
This integral is difficult to solve analytically, so we can use numerical methods to approximate the value. Using a calculator or computer program, we get:
L ≈ 38.786
So the length of the curve is approximately 38.786 units.
Learn more about length:
https://brainly.com/question/24487155
#SPJ11
I need help please I can’t understand these problems.

Answers
Answer:
m∠ABD = 88°, m∠CBD = 23°
Step-by-step explanation:
m∠ABD + m∠CBD = m∠ABC = 111°
(-10x + 58) + (6x + 41) = 111
-4x + 99 = 111
-4x = 12
x = -3
m∠ ABD = -10(-3) + 58 = 88°
m∠CBD = 6(-3) + 41 = 23°
Answer:
Step-by-step explanation:
X= -3
Abd = 88 degrees
Cbd = 23 degrees
Set the equation this way and solve:
-10x + 58 + 6x + 41 = 111
-4x + 99 = 111
-4x = 12
X = -3
Give examples of numbers that you would multiply by 3 to get an answer that is greater than 3, equal to 3, or less than 3
Answers
Answer:
1. 3 x 3 = 9
2. 3 x 1 =3
3. 3 x 0.4 = 1.2
Step-by-step explanation: Hope this helps :)
What do you mean by 3 more than 7?
Answers
The statement 3 more than 7 means, a number is 3 more than a given number. Since here the number is 7 so the required number will be 7+3= 10
In numerical more than simply refers to adding and less than refers to subtracting. If it is given that a number let's say Z is Y more than X then the value will be Z= X+Y
If a given number let's say Z is Y less than X then the value of Z will be
Z=X-Y
More than means add which gives us a bigger value. Less than means subtract which gives us a smaller value
So, 3 more than 7 means 7+3 = 10
Learn more about adding:
https://brainly.com/question/3255453
#SPJ4
a client has orders to receive 3,000 ml of iv fluid at a rate of 150 ml/hr. if the infusion starts at 0800, when would it be finished?
Answers
Step-by-step explanation:
3000 ml / 150 ml/hr = 20 hrs from 0800 would be 0400 the NEXT day.
in triangle $abc$, let angle bisectors $bd$ and $ce$ intersect at $i$. the line through $i$ parallel to $bc$ intersects $ab$ and $ac$ at $m$ and $n$, respectively. if $ab
Answers
In triangle $abc$, let angle bisectors $bd$ and $ce$ intersect at $i$. the line through $i$ parallel prove that $AB < AM < AN < AC$.
To solve this problem, use the angle bisector theorem and some geometric properties of triangles. Let's begin.
Given: Triangle $ABC$ with angle bisectors $BD$ and $CE$ intersecting at $I$. The line through $I$ parallel to $BC$ intersects $AB$ and $AC$ at $M$ and $N$, respectively. Also, $AB < AC$.
to prove: $AB < AM < AN < AC$.
Proof:
Angle Bisector Theorem:
According to the angle bisector theorem,
$frac{BD}{DC} = frac{AB}{AC} quad$ (1)
Parallel Lines:
Since line $MI$ is parallel to $BC$,
$angle MIB = angle IBC quad$ (2)
Angle Bisector Property:
From the angle bisector property,
$frac{AB}{BD} = frac{AC}{DC}quad$ (3)
Combining Equations (2) and (3):
$frac{AB}{BD} = frac{AC}{DC} =frac{AB}{MI} quad$ (4)
Using Equations (1) and (4):
$frac{BD}{DC} = frac{AB}{MI}$
From Equation (5):
$frac{AB}{MI} + 1 = frac{AB}{BD} + 1$
Simplifying Equation (6):
$frac{AB + MI}{MI} = frac{AB + BD}{BD}$
Using Equation (7):
$frac{AM}{MI} = frac{AB}{BD} quad$ (8)
Comparing Equations (1) and (8):
$frac{AB}{AC} = frac{AB}{BD} = frac{AM}{MI}$
Since $AB < AC$, it follows that $frac{AB}{AC} < 1$. Thus, $frac{AM}{MI} < 1$.
From Equation (10), $AM < MI$.
Using the same logic, prove $MI < AN$.
Finally, since $AM < MI < AN$, it follows that $AB < AM < AN < AC$, as required.
To know more about triangle here
https://brainly.com/question/22746827
#SPJ4
What is the common difference in the arithmetic sequence 30, 27, 24, 21, 18,
Answers
1. The quotient of a number and 6 is 12.
Answers
Answer:
2 is your answer
Step-by-step explanation:
6x2=12
Answer:
72
Step-by-step explanation:
\( \frac{x}{6} = 12 \\ x = 12 \times 6 \\ x = 72\)
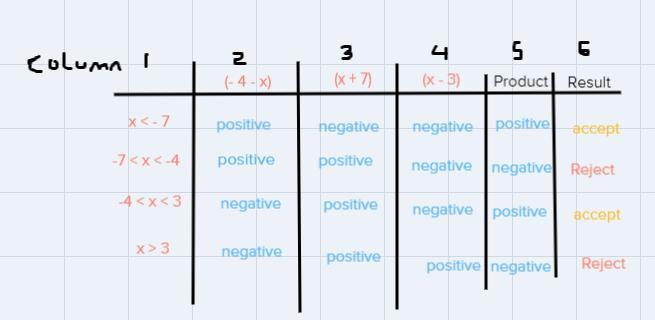
I'm solving polynomial inequalities and I need to know (-4-x)(x+7)(x-3) > 0
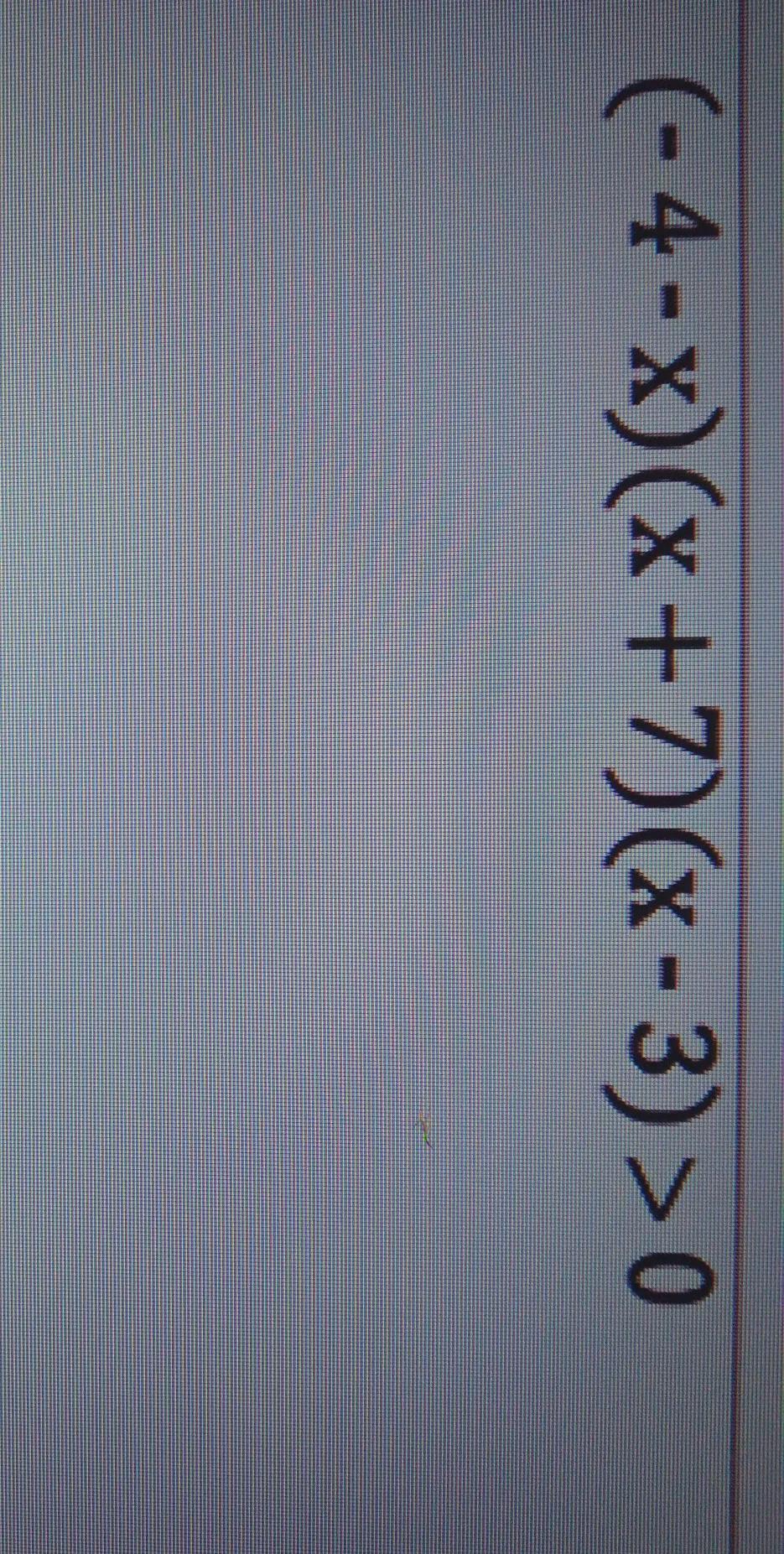
Answers
Given the inequalities
(-4 -x)(x+7)(x-3) >0
Step 1: Find the zeros of the polynomials
For (-4 -x)
\(\begin{gathered} (-4-x)=0 \\ -4\text{ -x =0} \\ -x=4 \\ x\text{ = -4} \end{gathered}\)For (x+7)
\(\begin{gathered} x\text{ +7 = 0} \\ x\text{ = 0 - 7} \\ x\text{ = -7} \end{gathered}\)For (x-3)
\(\begin{gathered} x-3\text{ =}0 \\ x\text{ = 0+ 3} \\ x\text{ = 3} \end{gathered}\)Step 2: Draw the range of values on a number line
Step 3: Construct a table to test the range
The table tests the ranges that were obtained from the number line
column 1 shows the range
The values are then tested for each range.
Column 6 shows the product of all the values gotten
The solution to the polynomial is that which was accepted
Since the inequality sign is that of a greater sign, we will accept those that are positive
Hence the solution is
\(\begin{gathered} x\text{ < -7 } \\ \text{and} \\ -4
Let R be the region Bounded by the following curves. Find the volume of the solid generated when R is revolved about the y-axis.
y= sin ^-1 (x/13), X=0, y= (pi/12)
Answers
The volume if the solid generated is 12.32 cubic units.
The volume of the solid generated by revolving the region bounded by the curves y = sin^-1(x/13), x = 0, and y = pi/12 about the y-axis can be calculated using the method of cylindrical shells.
First, we need to find the limits of integration for y. From the equation y = sin^-1(x/13), we can solve for x to get x = 13 sin(y). Since y ranges from 0 to pi/12, we know that x ranges from 0 to 13 sin(pi/12).
Next, we need to find the height of each cylindrical shell. This is simply the difference between the upper and lower curves, which is pi/12 - sin^-1(x/13).
Finally, we integrate the expression for the volume of a cylindrical shell over the range of y to obtain the total volume:
V = ∫[0, pi/12] 2πx(pi/12 - sin^-1(x/13)) dy
V = π/6 ∫[0, pi/12] (13sin(y))^2 (pi/12 - y) dy
V = π/6 ∫[0, pi/12] 169sin^2(y) (pi/12 - y) dy
V = π/6 [169(1/2)(pi/12)^2 - 169∫[0, pi/12] ysin^2(y) dy]
V ≈ 12.32 cubic units
Therefore, the volume of the solid generated by revolving the region bounded by the curves y = sin^-1(x/13), x = 0, and y = pi/12 about the y-axis is approximately 12.32 cubic units.
For more similar questions on volume:
https://brainly.com/question/6204273
#SPJ11
y-2=3 3x - 2y =5 substitutions
Answers
Answer:
x=5
y=5
Step-by-step explanation:
y-2=3
3x-2y=5
Let's solve for y in the first equation.
y-2=3
Add 2 to both sides,
y=5
Let's substitute y=5 into the other equation.
3x-2y=5
3x-2*5=5
3x-10=5
Add 10 to both sides,
3x=15
Divide by 5 to both sides,
x=5
Therefore, x and y both equal 5.
True or false the product of a complex number and its conjugate is a real numberi
Answers
Answer:
It is true that the product of a complex number and its conjugate is a real number.
Step-by-step explanation:
You're welcome.
Answer:
True
Step-by-step explanation:
....this is how you 'rationalize' denominators that have complex numbers in them
Use the picture below to
# 1) Your realized income is $3,543.22/month.
determine your fixed expenses each month. How much could you save per
month if you take 25% of your discretionary monies and put it in a savings
account?
Answers
The amount you could save per month would be 25% of your discretionary money.
How much could you save per month if you take 25% of your discretionary money?Discretionary income is the money you have left over after paying taxes and necessary cost-of-living expenses.
The formula for discretionary money is: Discretionary money = Realized income - Fixed expenses. Inputting data, we have: Discretionary money = $3,543.22 - Fixed expenses
Amount to be saved = 25% of discretionary money
Amount to be saved = 0.25 * (Realized income - Fixed expenses)
Therefore, the amount savable is calculated as 0.25 times the difference between your realized income and fixed expenses.
Read more about income
brainly.com/question/30157678
#SPJ1
Exercise 10
You randomly choose one of the tiles. Without replacing the first tile, you choose a second tile. What is the probability of the compound event? Write your answer as a fraction or percent rounded to the nearest tenth.

Answers
The probability of choosing a 5 and then a 6 is 1/49
Finding the probability of the compound eventFrom the question, we have the following parameters that can be used in our computation:
The tiles
Where we have
Total = 7
The probability of choosing a 5 and then a 6 is
P = P(5) * P(6)
So, we have
P = 1/7 * 1/7
Evaluate
P = 1/49
Hence, the probability of choosing a 5 and then a 6 is 1/49
Read more about probability at
https://brainly.com/question/31649379
#SPJ1
Question
You randomly choose one of the tiles. Without replacing the first tile, you choose a second tile. Find the probability of the compound event. Write your answer as a fraction or percent rounded to the nearest tenth. The probability of choosing a 5 and then a 6
The expression 2x² + ax is equivalent to x (2x + 7) for some constant a. What is the value of a ?
Answers
2x^2 + ax = 2x^2 + 7x
Subtract 2x^2 from both sides
ax = 7x
a = 7