2. Find the length of the arc shown in red. Leave
your answer in terms of pi.
A) 1.5 pi cm
B) 2.5 pi cm
C) 3.5 pi cm
D) 4.5 pi cm

Answers
Answer:
2.5 B
Step-by-step explanation:
Related Questions
what is not a factor of 16
Answers
Answer:
3
Step-by-step explanation:
3 is not a factor of 16.
Is that a valid answer? lol
Hi there!
First of all, let's determine the factors of 16.
Remember, a factor is a number that a number can be evenly divided by.
Example: 5 is a factor of 10, because 10 can be evenly divided by 5.
So, the factors of 16 are:
1, 2, 4, 8, and 16.
Now, there's an infinite amount of numbers that are not factors of 16. Here are some of them:
3, 5,7,9, 10,11, 12, 13, 14, 15...
Hope it helps.
Feel free to ask if you have any doubts.
\(\bf{-MistySparkles^**^*\)
describe transformation y=(x+5)^2 -19

Answers
Answer:
y=x^2 +10x+6 should be the answer
Step-by-step explanation:
Please help!!!! BRAINLIEST to correct and BEST answer!!!

Answers
Hi! can someone help with these questions? I’ll mark brainliest :) Have a nice day and thank you!

Answers
Answer:
7. 32
8. 68
9. 16
10. 29
Step-by-step explanation:
7. =90°-58
=32
8. =68°
9. = (1 + 5x)°
= (1 + 5(16)°
=81
10. = (2x + 3)°
= (2(29) + 3)°
= 61°
Write in standard form: (4.4x10^3)+(2.1x10^2)/(2x10^2)
Answers
Answer:
4401.05
Step-by-step explanation:
simplified 4400+210/200
ree-ring dating from archaeological excavation sites is used in conjunction with other chronologic evidence to estimate occupation dates of prehistoric Indian ruins in the southwestern United States. Suppose it is thought that a certain pueblo was occupied around 1292 A.D. (based on evidence from potsherds and stone tools). The following data give tree-ring dates (A.D.) from adjacent archaeological sites:1189 1267 1268 1275 1275 1271 1272 1316 1317 1230(ii) Assuming the tree-ring dates in this excavation area follow a distribution that is approximately normal, does this information indicate that the population mean of tree-ring dates in the area is different from (either higher or lower than) 1292 A.D.? Use a 1% level of significance.
Answers
The P-value is greater than the level of significance of 0.05, we fail to reject the null hypothesis and conclude that there is: not enough evidence to suggest that the population mean of tree-ring dates is different from 1284 A.D. at the 5% level of significance.
(a) The sample mean is x = 1271.8 A.D. and the sample standard deviation is s = 35.8 yr.
(b) To test whether the population mean of tree-ring dates is different from 1284 A.D., we can use a one-sample t-test with the null hypothesis H0: μ = 1284 and the alternative hypothesis Ha: μ ≠ 1284, where μ is the population mean of tree-ring dates. Using a calculator or a t-table, the sample test statistic is calculated as:
t = (x - μ) / (s / √n) = (1271.8 - 1284) / (35.8 / √10) = -1.263
(c) The P-value for this test is the probability of obtaining a sample mean as extreme or more extreme than 1271.8 if the null hypothesis is true. Since this is a two-tailed test and the calculated t-value is negative, we need to find the area in the left tail and right tail of the t-distribution with 9 degrees of freedom.
From a t-table or using a calculator, we find the area in the left tail to be 0.1295 and the area in the right tail to be 0.1295. Therefore, the P-value is the sum of the two tail probabilities, which is P = 2 × 0.1295 = 0.259.
Since the P-value is greater than the level of significance of 0.05, we fail to reject the null hypothesis and conclude that there is not enough evidence to suggest that the population mean of tree-ring dates is different from 1284 A.D. at the 5% level of significance.
To know more about hypothesis, refer here:
https://brainly.com/question/30751004#
#SPJ11
Complete question:
Tree-ring dating from archaeological excavation sites is used in conjunction with other chronologic evidence to estimate occupation dates of prehistoric Indian ruins in the southwestern United States. Suppose it is thought that a certain pueblo was occupied around 1284 A.D. (based on evidence from potsherds and stone tools). The following data give tree-ring dates (A.D.) from adjacent archaeological sites:
1189 1267 1268 1275 1275 1271 1272 1316 1317 1230
(i) Use a calculator with sample mean and standard deviation keys to find x and s. (Round your answers to one decimal place.)
x = A.D.
s = yr
(ii) Assuming the tree-ring dates in this excavation area follow a distribution that is approximately normal, does this information indicate that the population mean of tree-ring dates in the area is different from (either higher or lower than) 1284 A.D.? Use a 5% level of significance.
(a) What is the level of significance?
(b) What is the value of the sample test statistic? (Round your answer to three decimal places.)
(c) Find the P-value. (Round your answer to four decimal places.)
A continuous random variable X has probability density function f(x) = c(1+x)(1 - 2 over the domain -1<<1. (a) i. Evaluate the constant e (the integration can be done by MATLAB). ii. Plot the probability density function over the domain (-1,1). Is this density function skewed to the right, skewed to the left, or symmetric? (b) Use MATLAB to evaluate I i. the mean y = E(X)= |- «f(x) dx; ii. E(X)= (- 22 f(x) dx; iii. the variance o2 = Var(X) = E(X) – H?, and the standard deviation o. *(c) i. Use MATLAB to find an expression for the cumulative distribution function F(x). ii. Check the result in (i) by differentiation. Hint: simplify (ans) might help! iii. Evaluate P(-0.2 X <0.2).
Answers
(a)i. Evaluating the constant:
\($$\int_{-1}^{1} c(1+x)(1-2x) dx = 1$$$$\implies c = \frac{3}{4}$$\)
Therefore, the probability density function is:
\($$f(x) = \frac{3}{4} (1+x)(1-2x), -1< x < 1$$\) ii. Plotting the probability density function:
From the graph, it is observed that the density function is skewed to the left.
(b)i. The mean:
\($$E(X) = \int_{-1}^{1} x f(x) dx$$$$E(X) = \int_{-1}^{1} x \frac{3}{4} (1+x)(1-2x) dx$$$$E(X) = 0$$\)
ii. The second moment about the origin:
\($$E(X^2) = \int_{-1}^{1} x^2 f(x) dx$$$$E(X^2) = \int_{-1}^{1} x^2 \frac{3}{4} (1+x)(1-2x) dx$$$$E(X^2) = \frac{1}{5}$$\)
Therefore, the variance is:
\($$\sigma^2 = E(X^2) - E(X)^2$$$$\implies \sigma^2 = \frac{1}{5}$$\)
iii. The standard deviation:
$$\sigma = \sqrt{\sigma^2} = \sqrt{\frac{1}{5}} = \frac{\sqrt{5}}{5}$$(c)
i. The cumulative distribution function:
\($$F(x) = \int_{-1}^{x} f(t) dt$$$$F(x) = \int_{-1}^{x} \frac{3}{4} (1+t)(1-2t) dt$$\)
ii. The probability density function can be obtained by differentiating the cumulative distribution function:
\($$f(x) = F'(x) = \frac{3}{4} (1+x)(1-2x)$$\)
iii. Evaluating\(P(-0.2 < X <0.2):$$P(-0.2 < X <0.2) = F(0.2) - F(-0.2)$$$$P(-0.2 < X <0.2) = \int_{-0.2}^{0.2} f(x) dx$$$$P(-0.2 < X <0.2) = \int_{-0.2}^{0.2} \frac{3}{4} (1+x)(1-2x) dx$$$$P(-0.2 < X <0.2) = 0.0576$$\)
To know more about probability visit:
https://brainly.com/question/31828911
#SPJ11
when a certain number is added to 11 and the result is multiplied by 3, the final result is 45 . find the number
Answers
Answer:
4
Step-by-step explanation:
Let the number = x
Added to 11 = 11+x
Multiply result by 3 = 3(11+x)
Final result = 45
Solving
3(11 + x) =45
33 +3x = 45
3x= 45-33
3x=12
X=4 by dividing both side by 3
1. Jamie purchases gifts for three friends. She chooses a book for $12.80 and then finds two identical necklaces. Her budget is $48 before tax. What is the maximum she can spend on each necklace?
2. The school PTA is sponsoring a dance. They decide to price student tickets at $6. It will cost the PTA $250 to put on the dance. How many tickets will the PTA need to sell in order to make a profit?
3. A wedding venue holds no more than 485 guests. If 319 people have said they are attending, how many more people does the venue have room for?
4. A conference is ordering gift bags for each attendee. The cost will be $14.50 per bag plus an $80 set-up fee. If the total exceeds $500 there is a discount. How many gift bags must the conference order to receive the discount? Write and solve an inequality.
5. A taxi cab charges $3 per person plus $1.25 per mile. What is the greatest distance one passenger could travel with a budget of $28? Write and solve an inequality.
6. A 15-passenger van is rented for a family vacation. The van rental is 460 per day, plus a $145 insurance fee. How many days can the van be rented if they want to spend no more than $625 on the van rental. Write and solve an inequality.
Answers
Step-by-step explanation:
1.
(48 - 12.80)/2 = $17.62.
250/6 ≈ 42 and more3.
485 - 319 = 166 more people4.
14.5x + 80 > 50014.5x > 420x > 420/14.5x > 295.
1.25x + 3 ≤ 281.25x ≤ 25x ≤ 25/1.25x ≤ 20 miles6. This seems a typo... 460 is likely $60
60x + 145 ≤ 62560x ≤ 625 - 14560x ≤ 480x ≤ 480/60x ≤ 8 daysI need to know the slope and the meaning of the answer

Answers
Answer:
Step-by-step explanation:
150/50 using rise over run for the slope
The slope is 3 meaning for every pound the handling fee goes up in 3 dollars
How many ways can a president, vice-president, and secretary be chosen from a club with 12 members?
Answers
In 1320 ways can a president, vice-president, and secretary be chosen from a club with 12 members. Number of ways can be calculated by using permutation.
A permutation of a set in mathematics is, broadly speaking, the rearrangement of its elements if the set already has an ordered structure into a sequence or linear order. The act or procedure of altering the linear order of an ordered set is referred to as a "permutation."
A permutation is a mathematical procedure that counts all possible arrangements of a given set in which the arrangement's order matters.
President can be choose in 12 ways
Vice-president can be choose in 11 ways
Secretary can be choose in 10 ways
Total number of ways = 12 ×11×10=1320
Learn more about permutation here
https://brainly.com/question/1216161
#SPJ4
Math is multi-cultural, analytical, timeless and hands-on. Provide a piece of mathematical concept, hypothesis, law, paradox, rule or theorem to prove this statement.
Answers
It should be noted that mathematics is multicultural. This implies that mathematics applies to different cultures.
Mathematics is multicultural and it's neutral from the issues of races, gender, class, etc. Also, it should be noted that mathematics is analytical.Furthermore, mathematics is timeless and hands-on. This implies that students need to touch and feel what they're learning through a concrete experience.Learn more about mathematics on:
https://brainly.com/question/12798024
Katelyn was designing a cover for her binder and needed to cut some construction paper. The construction paper length can be represented by the expression (a2 + 7a - 3) if katelyn cut a piece that was (2a+5) long, how much construction paper is left
Answers
Answer:
a² + 5a - 8
Step-by-step explanation:
If the construction paper has a length of a² + 7a - 3, and Katelyn cut a piece 2a + 5 long, the amount of construction paper that would be left is,
a² + 7a - 3 - (2a + 5)
= a² + 7a - 3 - 2a - 5
collecting like terms, we have
= a² + 7a - 2a - 3 - 5
simplifying, we have
= a² + 5a - 8
You can compare two marginal distributions to see if the corresponding two variables are related.T or F
Answers
You cannot compare two marginal distributions to see if the corresponding two are related . So, the statement is false .
The answer to the stated question is false . No , you cannot compare two marginal distributions to see if the corresponding two variables are related.
The given question is related to probability and statistics.
Coming to probability distribution , it is the mathematical function that gives the probabilities of occurrence of different possible outcomes for an experiment. It is a mathematical description of a random phenomenon in terms of its sample space and the probabilities of events.
The marginal distribution of a subset of a collection of random variables is the probability distribution of the variables contained in the subset. It gives the probabilities of various values of the variables in the subset without reference to the values of the other variables.
To know more about probability refer:
https://brainly.com/question/13604758
#SPJ4
If the average product of labor is seven units of output per worker per day, the total output of 15 workers will be ______________ units per day.
Answers
The total output of 15 workers will be 105 units per day.Work and time are correlated so using this we could solve the question.
What is work and time?
Time is the duration of any activity or work that occurs or continues.
Work is a task or set of activities designed to achieve a specific result.
Main Body:
This can be done by simply multiplying both number of days and total work in one day.Hence the equation become:
total output = total workers*total work done by one person.
Total output = 7*15
hence,total output = 105 units
to know more about work and time click on the link below
https://brainly.com/question/13086625
#SPJ4
if 30% of r is 33, what is 70% of R
Answers
Answer:
77
Step-by-step explanation:
We Know
30% of r = 33
Find 1% by taking
33 / 30 = 1.1
What is 70% of R?
We take
1.1 x 70 = 77
So, 70% of R is 77
The question requires to first find the value of R by solving the equation (0.30*R = 33). Then, calculate 70% of R by multiplying the found R value with 0.70.
Explanation:The question tells us that 30% of R equals 33. To find the value of R, we can set up an equation where 0.30 (which is 30% in decimal form) times R equals 33. Dividing both sides of the equation by 0.30 will give us the value for R. Once we have found R, we can then find 70% of R by multiplying R by 0.70 (70% in decimal form).
Step-by-step:Set up an equation: 0.30*R = 33Solve for R: R = 33 ÷ 0.30 Calculate 70% of R: 0.70 * R Learn more about Percentage Calculations here:https://brainly.com/question/329987
#SPJ2
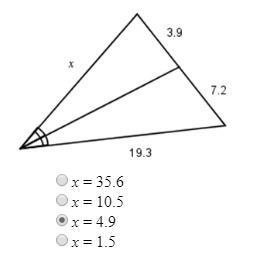
What is the value of x to the nearest tenth? The figure is not drawn to scale.
A. x=35.6
B. x=10.5
C. x=4.9
D. x=1.5
Answers
The value of x corresponds to option B: 10.5 as the figure provided has two congruent figures inside it.
From the given image, we can consider that that the two triangles- one for which side x is given, and another for which measurements of both sides are given- are congruent. This is because they both have one common side and one angle equal to another at a vertex (refer image).
Thus, we have:
(x / 19.3) = (3.9 / 7.2)
Then, attempting to find the value of x we have:
x = (3.9 / 7.2) × (19.3)
= 10.5
Therefore, option B gives the measure of the side x of the given triangle, where x = 10.5.
To know more about triangles, refer:
https://brainly.com/question/1675117
#SPJ4
Isabel bought 4 magazines for a total of $15. At this rate, what is the cost of 16 magazines?
Answers
Answer:
Step-by-step explanation:
we will use ratio
magazines : cost
4 : $15
16 : $60
we will multiply 4 by 4 and 15 also by 4 so 16 magazines will cost $60
The cost of 16 magazines is $22.5.
What is multiplication?
Multiplication is a mathematical arithmetic operation. It is also a process of adding the same types of expression for some number of times.
Example - 2 × 3 means 2 is added three times, or 3 is added 2 times.
Isabel bought 4 magazines for a total of $15.
The unit rate of the magazines,
= 15/4 = $3.75.
The cost of 16 magazines,
= 6 x 3.75
= $22.5
Therefore, the cost is $22.5.
To learn more about the multiplication;
https://brainly.com/question/19634536
#SPJ2
Figure 22-47 shows two parallel nonconducting rings with their central axes along a common line. Ring 1 has uniform charge q1 and radius R; ring 2 has uniform charge q
2
and the same radius R. The rings are separated by distance d=3.00R. The net electric fiel point P on the common line, at distance R from ring 1 , is zero. What is the ratio q
1
/q
2
? Figure 22−47 Problem 23.
Answers
The ratio of charge magnitude \(\frac{q_1}{q_2}\) is : 0.206
Electric Field due to a Charged Ring on axial point:If a ring of radius R carry a charge Q, then electric field at a point P at a distance x is given by
\(E=\frac{kQx}{(R^2+x^{2} )^\frac{3}{2} }\)
Let us recap important information from the question
Radius of each ring is RRatio of electric field at P \(\frac{E_1}{E_2} =1.15\)Electric field at point P due to ring 1
\(E_1=\frac{kq_1R}{(R^2+R^{2} )^\frac{3}{2} }=\frac{kq_1}{R^2}\)
Electric field at point P due to ring 2
\(E_2=\frac{kq_22R}{(R^2+(2R)^{2} )^\frac{3}{2} }=\frac{2kq_1}{11.2R^2}\)
Now,
\(1.15=\frac{11.2q_1}{2q_2}\\ \\\frac{q_1}{q_2} =\frac{2}{11.2}(1.15)=0.206\)
Learn more about Electric field at:
https://brainly.com/question/11482745
#SPJ4

resuelve el monomio 2x²y³z + 3x² y ³z
Answers
Answer:
combine like terms
5x^2 *y^3*zhow to prove converse of. basic proportionality theorem
please answer within one hour
Answers
Step-by-step explanation:
plz mark my answer as brainlist plzzzz.
hope this will be helpful to you.

Answer:
two different ways of theoretical and experimental explanation
both are correct
hope they helped you




Dale invested $900 at 6% simple interest. How much is his investment worth after two years?
Answers
Dale invested $900 at a simple interest rate of 6%. To find the worth of his investment after two years, we can use the formula for simple interest:
I = P * r * t
where I is the interest, P is the principal amount, r is the rate of interest, and t is the time period.
Substituting the given values, we have:
I = $900 * 6% * 2 years = $108
The worth of Dale's investment after 2 years is equal to the sum of the principal amount and the simple interest.
Worth of investment after 2 years = Principal + Simple interest = $900 + $108 = $1008
Therefore, the worth of Dale's investment after two years is $1008.
Know more about simple interest:
brainly.com/question/30964674
#SPJ11
Pls help we just started today

Answers
Answer:
5.4
Step-by-step explanation:
using pythagoras theorem
a²+b²=c²
5²+2²=c²
c²= 25+4
c²=29
\( \sqrt{c ^{2} } = \sqrt{29} \)
c=5.4
When Elizabeth began college, the price of her school books were $345. One year later, the cost for her books increased by 7%. If the price for books continued to increase by 7% each year, what is the price of books after 5 years? Round your answer to the nearest dollar.
need this asap
Answers
Answer:
The initial cost of Elizabeth's books is $345. After one year, the cost of her books increased by 7%, which is an increase of $345 x 7/100 = $24.15
So, the cost of her books after one year is $345 + $24.15 = $369.15
To find the price of her books after five years, we can use the formula for compound interest:
A = P(1 + r/n)^(nt)
Where A is the final amount, P is the initial principal (or starting amount), r is the annual interest rate as a decimal, n is the number of times the interest is compounded per year, and t is the number of years.
In this case, P = $345, r = 7/100, n = 1, and t = 5
So, A = $345 (1 + 7/100)^5 = $345 * 1.07^5 = $475.14
Rounding this to the nearest dollar, we get $475.
Therefore, the price of Elizabeth's books after five years is $475.
Predict the number of times you roll an odd number or a two when you roll a six-sided number cube 300 times.
Answers
Answer:
The probability of rolling an odd number or a two on a six-sided die is 1/2 + 1/6 = 2/3. This means that if you roll a six-sided die 300 times, you can expect to roll an odd number or a two approximately 200 times
Step-by-step explanation:
Answer: 400 TIMES
Step-by-step explanation:
1/6 +1/2
4/6
2/3
find an equation of the tangent line to the graph of y = ln(x2) at the point (5, ln(25)).
Answers
The equation of the tangent line to the graph of y = ln(x^2) at the point (5, ln(25)) is y = (2/5)x - (2/5)ln(25) + ln(25). This line passes through the point (5, ln(25)) and has a slope of 2/5.
To find the equation of the tangent line to the graph of y = ln(x^2) at the point (5, ln(25)), we need to use the formula for the equation of a tangent line:
y - y1 = m(x - x1)
where (x1, y1) is the point of tangency and m is the slope of the tangent line. To find the slope, we need to take the derivative of y = ln(x^2):
y' = 2x/x^2 = 2/x
At x = 5, the slope of the tangent line is:
m = 2/5
So the equation of the tangent line is:
y - ln(25) = (2/5)(x - 5)
Simplifying this equation, we get:
y = (2/5)x - (2/5)ln(25) + ln(25)
Thus, the equation of the tangent line to the graph of y = ln(x^2) at the point (5, ln(25)) is y = (2/5)x - (2/5)ln(25) + ln(25). This line passes through the point (5, ln(25)) and has a slope of 2/5.
To find the equation of the tangent line to the graph of y = ln(x^2) at the point (5, ln(25)), we first need to determine the derivative of the function. The derivative represents the slope of the tangent line at any point on the graph.
The function is y = ln(x^2). Using the chain rule, the derivative is:
dy/dx = (1/x^2) * (2x) = 2/x
Now, we will find the slope of the tangent line at the point (5, ln(25)) by substituting x = 5 into the derivative:
m = 2/5
So, the slope of the tangent line at the point (5, ln(25)) is 2/5. To find the equation of the tangent line, we use the point-slope form:
y - y1 = m(x - x1)
Substitute the point (5, ln(25)) and the slope 2/5 into the equation:
y - ln(25) = (2/5)(x - 5)
This is the equation of the tangent line to the graph of y = ln(x^2) at the point (5, ln(25)).
To know more about tangent line visit:
https://brainly.com/question/31326507
#SPJ11
choices:
x=360-114
x=114-90
360=x +90+114
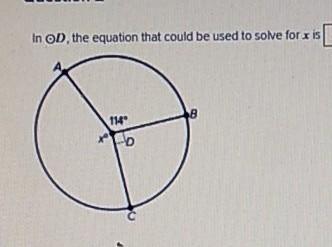
Answers
Answer:
see explanation
Step-by-step explanation:
The sum of the angles around D = 360° , then
x = 360 - (114 + 90) ← equation to find x
= 360 - 156
= 204
1st correcct will get brainliest

Answers
Answer:
it s A
Step-by-step explanation:
if the two to the power of ten is on the outside, you will always need to add that to the other powers of ten
Brianliest plz thanks
An amusement park charges $20 to enter the
park and an additional fee for each time a guest
rides a roller coaster. Emily rode 5 times on a
roller coaster. Her total payment was $40.
Shaheem rode 7 times on a roller coaster. What
was his total payment?
Answers
Answer:
Shaheem paid $76 as his total.
Step-by-step explanation:
He paid $20 to get in, Emily paid $40 for 5 rides, and if you divide that to get the payment for one ride which is $8. So you multiply 8 by 7 because 7 is how many rides he rode which equals to 56, plus 20(his enter fee) would be $76.
Twice Jack's age plus 5 is 21. Write and solve an equation. How old is Jack? Responses A32 B88 C52 D13
Answers
16 divided by 2 = 8