(7-2)² - 5 / 5 I dont get the exponent part can someone help me with whole problem
Answers
(7-2)² - 5/5
Do the math in the parentheses first.
7 - 2 = 5
Now the equation should read
(5)² - 5/5 or just 5² - 5/5
Do the exponent(s).
5² = 25
25 - 5/5
Do the division.
5/5 = 1
25 - 1 = 24
Therefore, according to our equation, the answer is 24.
I hope this answered your question.
Related Questions
Select the statement that best describes SST. Question 3 options: SST measures the variability of the actual data. SST measures the variability between the data and the best guess at a linear model of the data. A large SST guarantees that the independent and dependent variables are related. A low SST minimizes the error between the data's actual y values and the model's y values.
Answers
SST measures the variability of the actual data.
SST, or the Total Sum of Squares, is a statistical measure that quantifies the total variability observed in the data. It represents the total variation of the dependent variable (y) without considering any specific model or independent variables.
SST measures the dispersion or spread of the actual data points around their mean. It provides an overall assessment of the total variability present in the data set, regardless of any relationships or models. By calculating the sum of the squared differences between each data point and the mean of the data, SST captures the total variation or deviation from the mean value.
The other options presented do not accurately describe SST. While SST is related to the variability in the data, it does not measure the variability between the data and a linear model (that would be measured by SSE, or Sum of Squares Error). SST also does not guarantee the presence of a relationship between independent and dependent variables, nor does it aim to minimize the error between actual y values and model y values.
In summary, SST represents the total variation in the data and is a fundamental measure in statistical analysis. It provides insights into the overall spread or dispersion of the observed data points, regardless of any specific models or relationships.
Learn more about Actual data.
brainly.com/question/31430138
#SPJ11
[6.01] Samra went to San Francisco for a vacation. She spent four nights at a hotel and rented a car for two days. Andres stayed at the same hotel and also spent four nights, but he rented a car for five days from the same company. If Samra paid $500 and Andres paid $740, how much did one night at the hotel cost?
Answers
Using substitution method, the cost of hotel per night is $ 85
Let hotel cost per night = x
Let car rental per day = y
For Samra4x + 2y = 500 ____(1)
For Andres4x + 5y = 740 ____(2)
Solving for x in the equation
Equation (1) - (2)
-3y = - 240
y = 80
Substitute the value of y in (1)
4x + 2(80) = 500
4x + 160 = 500
4x = 500-160
4x = 340
x = $85
Therefore, hotel cost per night is $85
Learn more on equations ; https://brainly.com/question/148035
#SPJ1
outliers are extreme values above or below the mean that require special consideration. True/ False
Answers
Answer:
false
Step-by-step explanation:
outliers can be neglected especially when working out the mean
Therefore, The statement that "outliers are extreme values above or below the mean that require special consideration" is True.
Explanation:
Outliers are extreme values that lie significantly above or below the mean. They have special considerations because they can affect the interpretation of the mean and standard deviation. For instance, if an outlier is included in the dataset, the mean will be different from when it is excluded, making the mean unreliable. Therefore, outliers should be examined carefully to determine if they represent a genuine value or an error.
Therefore, The statement that "outliers are extreme values above or below the mean that require special consideration" is True.
To know more about statement visit :
https://brainly.com/question/27839142
#SPJ11
The product (k-9 )^2 can also be written as?
Answers
pls help urgent
is \(\sqrt{20}\) a rational number.
Is \(\sqrt{121}\)a rational number?
is \(\sqrt{3}\) a rational number?
is \(\frac{5}{2}\) a rational number?
is \(\frac{58}{3}\) a rational number?
is - 3.76 a irrational number
is \(\sqrt{14 }\) a irrational number
is .37 a irrational number
Answers
Answer:
N, Y, N, Y, Y, N, Y, N
Step-by-step explanation:
Rational: √121, 5/2, 58/3, -3.76, 0.37
Irrational: √20, √3, √14
__
The square root of a perfect square, such as √121 = √(11^2) = 11, is rational. The square root of any integer that is not a perfect square is irrational.
Any fraction or finite-length decimal number is rational. Any repeated decimal is also rational, regardless of the length of the repeat.
4) f(x) = 2x^5+4x^4+11x^3+22x²+12x+24; -2
please show the steps on how to find the roots
Answers
Answer:
Step-by-step explanation:
f(x) = 2x^4(x + 2) + 11x^2(x + 2) + 12(x + 2)
f(x) = (x+2) [2x^4 + 11x^2 + 12]
f(x) = (x + 2)(2x^2 + 3)(x^2 + 4)
A man invests his savings in two accounts, one paying 6% and the other paying 10% simple interest per year. He puts twice as much in the lower-yielding account because it is less risky. His annual interest is $9878 dollars. How much did he invest at each rate?
Answers
The man invested 37900 dollars at 6% and 28022 dollars at 10%.
What is the interest?
The interest rate is the amount a lender charges a borrower and is a percentage of the principal—the amount loaned.
Let x be the amount invested at 6% and y be the amount invested at 10%. We know that:
x + y = total amount invested
0.06x + 0.1y = 9878 (annual interest)
and that y = 2x (because he put twice as much in the lower-yielding account).
Now we can use the second equation to find x:
0.06x + 0.1(2x) = 9878
0.06x + 0.2x = 9878
0.26x = 9878
x = 37900
Now we can use the first equation to find y:
x + y = total amount invested
37900 + y = total amount invested
y = total amount invested - 37900
We know that the annual interest is $9878, so we can substitute that value into the equation:
y = 9878 - 37900
y = -28,022
So the man invested 37900 dollars at 6% and -28022 dollars at 10%. However, the answer to the second value is not a real value as the investment cannot be negative.
Therefore, the man invested 37900 dollars at 6% and 28022 dollars at 10%.
To learn more about the interest visit,
https://brainly.com/question/2294792
#SPJ1
the order of a moving-average (ma) process can best be determined by the multiple choice partial autocorrelation function. box-pierce chi-square statistic. autocorrelation function. all of the options are correct. durbin-watson statistic.
Answers
The order (p) of an autoregressive (AR) process can be determined by Durbin-Watson Statistic, Box-Pierce Chi-square Statistic, Autocorrelation Function (ACF), and Partial Autocorrelation Function (PACF) coefficients., option E is correct.
The Durbin-Watson statistic is used to test for the presence of autocorrelation in the residuals of a time series model.
It can provide an indication of the order of the AR process if it shows significant autocorrelation at certain lags.
The Box-Pierce test is a statistical test used to assess the goodness-of-fit of a time series model.
It examines the residuals for autocorrelation at different lags and can help determine the appropriate order of the AR process.
Autocorrelation Function (ACF): The ACF is a plot of the correlation between a time series and its lagged values. By analyzing the ACF plot, one can observe the significant autocorrelation at certain lags, which can suggest the order of the AR process.
The PACF measures the direct relationship between a time series and its lagged values after removing the effects of intermediate lags.
Significant coefficients in the PACF plot at certain lags can indicate the appropriate order of the AR process.
By considering all of these methods together and analyzing their results, one can make a more informed decision about the order (p) of an autoregressive (AR) process.
To learn more on Autoregressive process click:
https://brainly.com/question/32519628
#SPJ4
The order (p) of a autogressiove(AR) process best be determined by the :
A. Durbin-Watson Statistic
B. Box Piece Chi-square statistic
C. Autocorrelation function
D. Partial autocorrelation fuction coeficcents to be significant at lagged p
E. all of the above
A tree is growing at a constant rate. It is recorded that at the 7th year since it was planted it was at a height of 25 feet. It was recorded again at the 10th year since it was planted and it was at a height of 31 feet. How much does the tree grow every year?
Answers
9514 1404 393
Answer:
2 feet per year
Step-by-step explanation:
In the 3 years between the 7th and 10th, the tree grew 6 feet from 25 ft to 31 ft. The rate of growth is ...
(6 ft)/(3 yr) = (6/3) ft/yr = 2 ft/yr
What is the probability of rolling 3 Fives in a row on a number cube?
Answers
Answer:
0.5 probability
Step-by-step explanation:
1/6+1/6+1/6= 0.5
Find the indefinite integral. (Remember to use absolute values where appropriate. Use C for the constant of integration.) dx x(ln x2)5
Answers
I'm assuming the integral is
\(\displaystyle \int \frac{dx}{x (\ln(x^2))^5}\)
We have
\(\ln(x^2) = 2 \ln|x| \implies (\ln(x^2))^5 = 32 (\ln|x|)^5\)
Then substituting \(y=\ln|x|\) and \(dy=\frac{dx}x\), the integral transforms and reduces to
\(\displaystyle \int \frac{dx}{x(\ln(x^2))^5} = \frac1{32} \int \frac{dy}{y^5} \\\\ ~~~~~~~~ = \frac1{32} \left(-\frac1{4y^4}\right) + C \\\\ ~~~~~~~~ = -\frac1{128(\ln|x|)^4} + C\)
which we can rewrite as
\(128 (\ln|x|)^4 = 8\cdot2^4(\ln|x|)^4 = 8 (2\ln|x|)^4 = 8 (\ln(x^2))^4\)
and so
\(\displaystyle \int \frac{dx}{x (\ln(x^2))^5} = \boxed{-\frac1{8(\ln(x^2))^4} + C}\)
Pls help plsssss I really need help

Answers
Answer:
B. n ≥ 12
Step-by-step explanation:
As you can see, the number is represented by n. So when n is no less than 12, it means that n is greater than 12 but can also be equal to it.
Therefore, the answer is n ≥ 12
Answer:
AAA
Step-by-step explanation:
A salesman earns 7% commission on all the merchandise that he sells. Last month he sold $8000 worth of merchandise. How much commission (in dollars) did he earn last month?
Answers
He earned 400$
8000 x .07 = 560
Explain how you would use addition to find the product of -2and 5 using the integer tiles and the number line
Answers
To find the product of -2 and 5 using the integer tiles and the number line, you can use the addition method.
Let us understand this with the help of an example.
To find -2 × 5 using integer tiles, follow these steps:
1. Take two red tiles to represent -2. 2.
Take five yellow tiles to represent 5. 3.
Combine the two sets of tiles. 4.
The final count of the tiles will be negative as we had negative tiles in the beginning. Hence the product will be -10.
To find -2 × 5 using a number line, follow these steps:
1. Start at zero on the number line
. 2. Move two places to the left because we have to represent -2.
3. From the point you reach, move five places to the right because we have to represent 5.
4. The final point on the number line is -10, which is the product of -2 and 5.
Therefore, the product of -2 and 5 is -10.
This is how we can use addition to find the product of -2 and 5 using integer tiles and the number line.
To learn more on integer tiles:
https://brainly.com/question/28343162
#SPJ11

Memo No
Date
Find the square root of 13²
Answers
Answer: 13
Step-by-step explanation:
The square root and exponent cancel out and you are left with 13.
I NEED HELP ASAP!! Each month, your phone company charges you a fee of 10 cents per minute as well as a service fee of $2.95 Write a linear function rule that models the number of dollars y you pay each month for any number of minutes x.

Answers
What is the solution to the linear equation?
-12+36-1--5-b
O b=-2
O b=-1.5
O b= 1.5
O b=2
Answers
Answer:
where is the equal to sign????
Solve [ x ′
y ′
]=[ 1
−25
1
−7
][ x
y
]x(0)=1,y(0)=−1 x(t)= y(t)= Question Help: □ Message instructor Question 2 Solve [ x ′
y ′
]=[ 3
−4
2
−1
][ x
y
]x(0)=−8,y(0)=6
Answers
The solution is [ x(t), y(t) ] = [150e^(-t/7) + 6(25e^(t/7)), (5/7)e^(-t/7) - (2/7)e^(t/7)]
Given,[ x′ y′]=[ 1 −25 1 −7 ][ x y ]x(0)=1,y(0)=−1
We can write the system of linear differential equations as follows :x′ = x - 25y .....(1)y′ = x - 7y .....(2)
Taking Laplace transform of both the sides, we get, s X - x(0) = X - 25Y ⇒ s X - 1 = X - 25Y
Similarly, taking Laplace transform of equation (2), we get, sY - y(0) = X - 7Y ⇒ sY + 1 = X - 7Y
Multiplying equation (1) by 7 and equation (2) by 25, we get7x′ - 175y′ = 7x - 175y .....(3)
25x′ + y′ = 25x - 7y .....(4)
Taking Laplace transform of equation (3) and (4), we get,7sX - 175Y - (7X - y(0)) = 7X - 175Y
Similarly, 25sX + sY - (25X + y(0)) = 25X - 7Y
Simplifying the above expressions, we get,(7s + 1)X - 175 Y = 1 .....(5)
(25s + 1)X + sY = -6 .....(6)
Solving the equations (5) and (6), we get, X = 150/(7s + 1) + 6(25s + 1)/(7s + 1)Y = 1/7[(s + 25)X - 1]
Hence, x(t) = Laplace^-1 [X] = Laplace^-1 [150/(7s + 1) + 6(25s + 1)/(7s + 1)] = 150e^(-t/7) + 6(25e^(t/7))y(t)
= Laplace^-1 [Y] = Laplace^-1 [1/7[(s + 25)X - 1]] = (5/7)e^(-t/7) - (2/7)e^(t/7)
Therefore, x(t) = 150e^(-t/7) + 6(25e^(t/7)) and y(t) = (5/7)e^(-t/7) - (2/7)e^(t/7).
Hence, the solution is [ x(t), y(t) ] = [150e^(-t/7) + 6(25e^(t/7)), (5/7)e^(-t/7) - (2/7)e^(t/7)].
Learn more about Laplace transform from this link:
https://brainly.com/question/30402015
#SPJ11
Aiko works in the fish department of a pet store. She must drain, clean, and refill two reef tanks. the first tank hold 175 gallons of water and drains at a rate of 25 gallons per hour. The second tank holds 200 gallons of water and drains at a rate of 30 gallons per hour.
1. Write an equation for each tank representing the total amount of water in gallons in the tank, y, in terms of the number of hours, x, that the tank drains
2. what is the solution to this system of equations.
3. suppose Aiko starts draining the tanks at the same time. When will both tanks have the same amount of water? How much water is in each tank at that time
Answers
The answers to each part is mentioned above.
What are algebraic expressions?In mathematics, an expression or mathematical expression is a finite combination of symbols that is well-formed according to rules that depend on the context.Mathematical symbols can designate numbers (constants), variables, operations, functions, brackets, punctuation, and grouping to help determine order of operations and other aspects of logical syntax.Given is that Aiko works in the fish department of a pet store. She must drain, clean, and refill two reef tanks. The first tank hold 175 gallons of water and drains at a rate of 25 gallons per hour. The second tank holds 200 gallons of water and drains at a rate of 30 gallons per hour.
( 1 ) -Equation for tank {1} -
y = 175 - 25x
Equation for tank {2} -
y = 200 - 30x
( 2 ) -For the solution we can write -
175 - 25x = 200 - 30x
- 25x + 30x = 200 - 175
5x = 25
x = 5
and
y = 200 - 150
y = 50
( 3 ) -For the same amount of water we can write -
175 - 25x = 200 - 30x
- 25x + 30x = 200 - 175
5x = 25
x = 5 hours
In tank (1), there is : 175 - 25 x 5 = 50 gallons
In tank (2), there is : 200 - 30 x 5 = 50 gallons
Therefore, the answers to each part is mentioned above.
To solve more questions on algebraic expressions, visit the link below -
brainly.com/question/1041084
#SPJ1
pls help, im literally almost to 80% in ixl

Answers
Answer:
17.28
Step-by-step explanation:
All u gotta do is 9% of 192 (just search that up)
Which table represents a linear function?

Answers
Answer:
a
Step-by-step explanation:
Pls help me a solve this one math problem:(
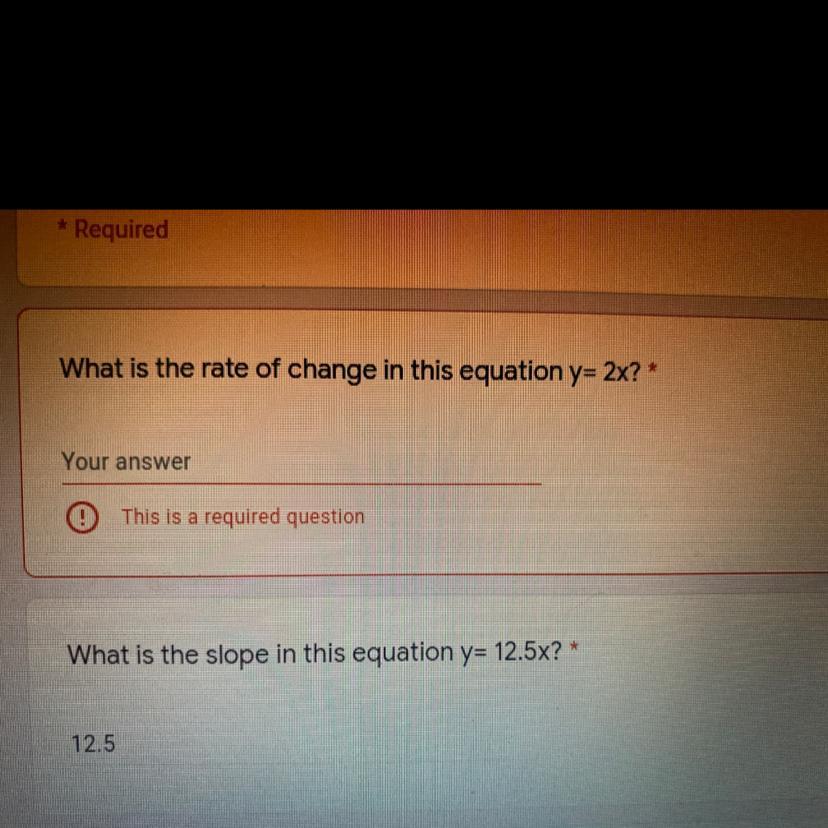
Answers
Answer:
2 units up for every one unit across, or just simply 2
Step-by-step explanation:
use the intermediate value theorem to show that the polynomial has a real zero between the given integers? f(x)= x^3-x-4; between 1 and 7.
Answers
We have demonstrated using the Intermediate Value Theorem that the polynomial function has a real zero between 1 and 7.
To apply the Intermediate Value Theorem to the polynomial function f(x) = x^3 - x - 4 and show that it has a real zero between the integers 1 and 7, we need to verify that f(1) and f(7) have opposite signs.
Let's evaluate f(1) and f(7) to determine their signs:
f(1) = (1)^3 - (1) - 4 = 1 - 1 - 4 = -4
f(7) = (7)^3 - (7) - 4 = 343 - 7 - 4 = 332
From the calculations, we can see that f(1) = -4 and f(7) = 332.
Since f(1) is negative (-4) and f(7) is positive (332), they have opposite signs.
According to the Intermediate Value Theorem, if a continuous function changes sign between two points, then it must have at least one real zero between those points.
Since f(1) = -4 (negative) and f(7) = 332 (positive), the polynomial function f(x) = x^3 - x - 4 must have a real zero between the integers 1 and 7.
Therefore, we have demonstrated using the Intermediate Value Theorem that the polynomial function has a real zero between 1 and 7.
for such more question on Intermediate Value
https://brainly.com/question/24015908
#SPJ8
Choose the scenario below that matches this number sentence:
27 ÷ 5
A) 27 people share 5 bags of chips.
B) 5 people share 27 brownies.
Answers
Step-by-step explanation:
B 27 bags of chips by 5 people
Answer:
B) 5 people share 27 brownies.
Step-by-step explanation:
This is the answer because:
1) If 5 people are sharing 27 brownies, it means they are basically asking, how many brownies did each person get. Then, we would write the numerical sentence: 27 ÷ 5 because we want to know how many brownies each person got.
Hope this helps! :D
Solve the following values for x and y :
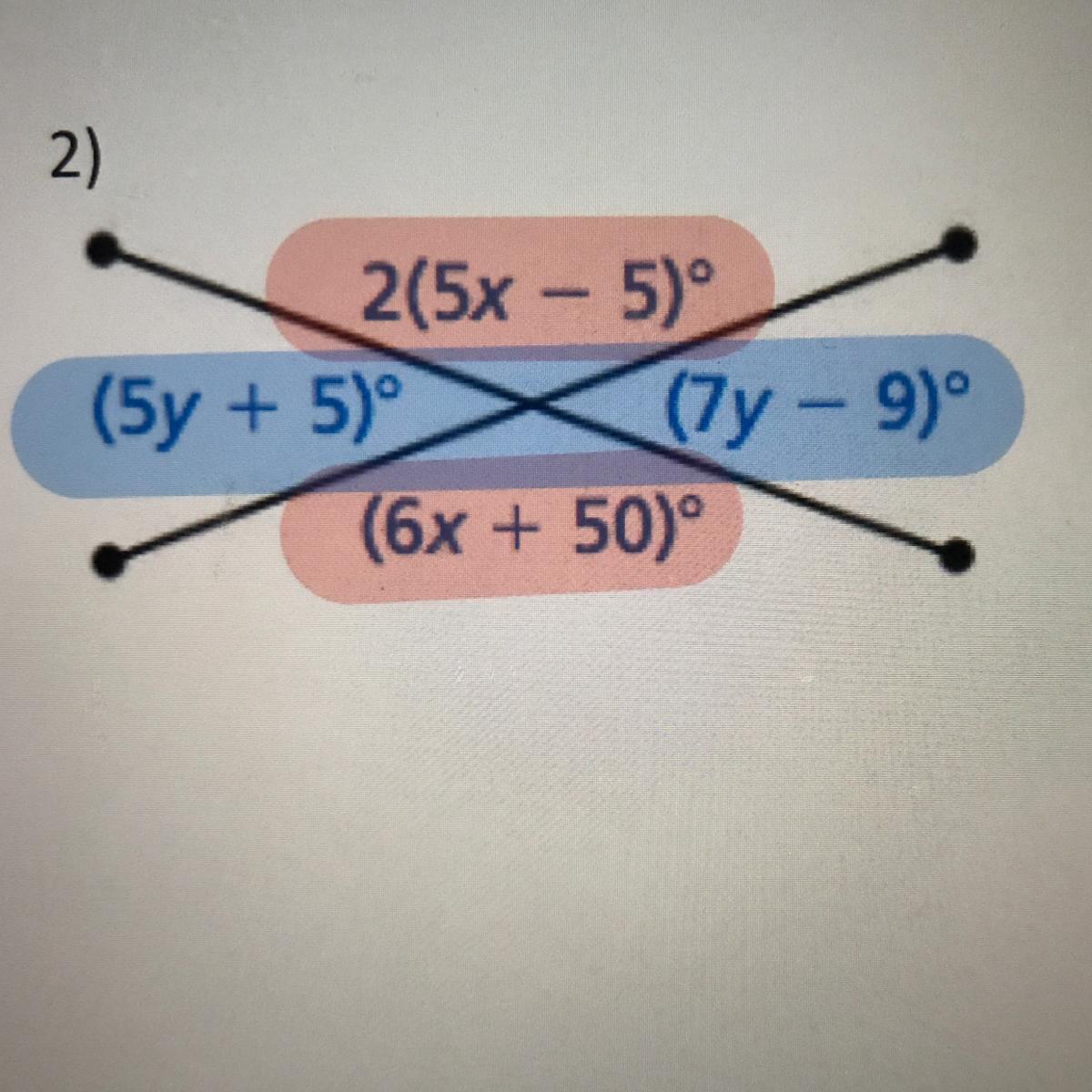
Answers
Answer and Step-by-step explanation:
2(5x - 5) = 6x + 50
Distribute the 2 to the 5x and -5.
10x - 10 = 6x + 50
Add 10 to both sides, and subtract 6x from both sides.
4x = 60
Divide both sides by 4.
x = 15
5y + 5 = 7y - 9
Subtract 5 from both sides, and 7y from both sides.
-2y = -14
Divide both sides by -2.
y = 7
#teamtrees #PAW (Plant And Water)
Solve the following quadratic equation for all values of x in simplest form.
3(x-5)^2=33
Answers
The value of x for the given quadratic expression is x = 5 ± √11.
What is an expression?The mathematical expression combines numerical variables and operations denoted by addition, subtraction, multiplication, and division signs.
Mathematical symbols can be used to represent numbers (constants), variables, operations, functions, brackets, punctuation, and grouping. They can also denote the logical syntax's operation order and other properties.
Given expression is 3(x-5)²=33. The value of x will be calculated as,
3(x-5)²=33
(x - 5 )² = 11
(x - 5 ) = ±√11
x = 5 ± √11
Therefore, the value of x for the given quadratic expression is x = 5 ± √11.
To know more about an expression follow
https://brainly.com/question/27826679
#SPJ1
What information would you need to use the Side-Angle-Side Similarity Theorem to prove that the triangles are similar
Answers
With these three pieces of information, you can apply the SAS Similarity Theorem to conclude that the triangles are similar.
To use the Side-Angle-Side (SAS) Similarity Theorem to prove that two triangles are similar, you would need the following information:
1. Side-Side Ratio: You need to know that the ratio of the lengths of corresponding sides of the triangles is equal.
2. Angle: You need to know that the included angle between the corresponding sides of the triangles is equal.
3. Side: You need to know that one pair of corresponding sides of the triangles is proportional.
To know more about triangles visit;
brainly.com/question/2773823
#SPJ11
Please help look at the picture attached need answers ASAP

Answers
You invest $500 into an account that collects 1% interest each month. If you add an additional $50 each week, what will the balance of the account be after 5 months?
Answers
Investing $500 into account that collects 1% interest each month and add an $50 each week for 5 months, your balance will be $1,275.00.
To start, you invested $500 into an account that collects 1% interest each month. This means that after the first month, your balance will have grown by 1% of $500, or $5.00. So at the end of the first month, your balance will be $505.00.
Next, you add an additional $50 each week. This means that after the first week, your balance will have grown by $50, bringing your balance to $555.00. After the second week, your balance will have grown by another $50, bringing it to $605.00.
We can continue this pattern for 5 months, or 20 weeks. So after the third week, your balance will be $655.00, and after the fourth week, your balance will be $705.00.
Continuing this pattern for the next 16 weeks, your balance will continue to grow with the additional $50 each week and the 1% interest each month. At the end of the 5 months, your balance will be approximately $1,275.00.
Learn more about interest brainly.com/question/30393144
#SPJ11
A spinner for a board game consists of a cardboard circle with two plastic arrows anchored to its center. The arrows are congruent isosceles triangles connected at their bases, as shown. 2 isosceles triangles are connected at their bases. The length of the base is 2 centimeters. The total perimeter of each triangle is 10 centimeters. Heron’s formula: Area = StartRoot s (s minus a) (s minus b) (s minus c) EndRoot The base of each triangle measures 2 centimeters and the perimeter of each triangle is 10 centimeters. What is the approximate total area of the plastic triangles on the spinner? 3. 9 square centimeters 6. 7 square centimeters 7. 7 square centimeters 13. 4 square centimeters.
Answers
A triangle is isosceles triangle when the two sides and two angle of the triangle in equal in measure.
The approximate total area of the plastic triangles on the spinner is 7.7 squared centimetre.
What is isosceles triangle?A triangle is isosceles triangle when the two sides and two angle of the triangle in equal in measure.
The area of the isosceles triangle is given as,
\(A=\dfrac{1}{2}\times b\times \sqrt{a^2-\dfrac{b^2}{4} }\)
Here, \(a\) is the measure of equal sides and \(b\) is the measure of third side.
Given information-
The arrows of board game are congruent isosceles triangles connected at their bases.
isosceles triangles are connected at their bases.
The base of each triangle measures 2 centimetres
The perimeter of each triangle is 10 centimetres.
The perimeter of a triangle is sum of all its sides.
As the given triangle is isosceles triangle. Thus both the sides of the triangle should be equal.
Suppose the equal sides is x centimetres. The measure of third side is given which is equal to 2 centimetres. Thus,
\(x+x+2=10\\2x=10-2\\2x=8\\x=\dfrac{8}{2} \\x=4\)
Hence the length of the equal sides of the isosceles triangle is 4 cm.
Using the above formula as,
\(A=\dfrac{1}{2}\times b\times \sqrt{a^2-\dfrac{b^2}{4} }\\A=\dfrac{1}{2}\times 2\times \sqrt{4^2-\dfrac{2^2}{4} }\\A= \sqrt{16-\dfrac{4}{4} }\\A=3.871\)
Hence the area of one plastic arrow triangle is 3.71 square centimetre. The approximate total area of the plastic triangles on the spinner is twice of it. Thus,
\(A=3.71\times2\\A=7.7\)
Thus the approximate total area of the plastic triangles on the spinner is 7.7 squared centimetre.
Learn more about the isosceles triangle here;
https://brainly.com/question/1475130
Answer:
The correct answer would be option C. 7.7 square centimeters
Step-by-step explanation:
Just got it right on edge. :)
Hope this helps!
Brainliest would be greatly appreciated :D