A die is rolled twice. What is the probability of showing a
three on the first roll and an even number on the second roll?
Answer using a fraction or a decimal rounded to three
places.
Answers
Answer:
\(\frac{2}{3}\)
Step-by-step explanation:
We Know
A die is rolled twice.
There are six faces on a die.
What is the probability of showing a three on the first roll?
The probability will be \(\frac{1}{6}\) because there is only one 3 in the total of 6 faces.
What is the probability of showing an even number on the second roll?
There are 3 even numbers: 2, 4, 6
The probability is \(\frac{3}{6}\) = \(\frac{1}{2}\)
Now we add both probabilities together.
\(\frac{1}{6}\) + \(\frac{3}{6}\) = \(\frac{4}{6}\) = \(\frac{2}{3}\)
So, the probabilities is \(\frac{2}{3}\)
Related Questions
A line passes through the point (10,-8) and has a slope of negative 3/2Write an equation in slope-intercept form for this line.
Answers
Answer:
9383
Step-by-step explanation:
a bag of chocolates is labeled to contain 0.384 pounds of chocolate. the actual weight of the chocolates is 0.3798 pounds. how much lighter is the actual weight?
Answers
The actual weight is 0.0042 pounds lighter than the labeled weight.
The actual weight of the chocolates is 0.3798 pounds, while the label on the bag states it should weigh 0.384 pounds. To determine how much lighter the actual weight is, we can calculate the difference between the two weights.
Subtracting the actual weight from the labeled weight, we get:
0.384 pounds - 0.3798 pounds = 0.0042 pounds.
Therefore, the actual weight is 0.0042 pounds lighter than the labeled weight.
It's important to note that this difference may seem small, but it can be significant depending on the context. Accuracy in labeling is crucial for various reasons, such as complying with regulations, providing precise information to consumers, and ensuring fair trade practices. Even minor discrepancies can impact trust and customer satisfaction.
For more information on actual weight visit: brainly.com/question/31170036
#SPJ11
Susan can run 3.5 miles in 40 minutes. About how many whole minutes would it take her to run 8 miles at this same rate? Set up and solve a
proportion. Show all work and answer the situation.
Answers
Answer:
40min=3.5miles
40/3.5=1mile
40/3.5×8=8miles
91.428 min
1hr and 31 min
Using the concept of a net rate introduced in Problem 2, determine a differential equation governing a population P(t) if the birth rate is proportional to the population present at time t but the death rate is proportional to the square of the population present at time t.
Answers
We may be thinking about either of these choices depending on whether we are talking about immigration or emigration.
\(\frac{d P}{d t}=r_g \pm r\)
This is further explained below.
What is a differential equation?Generally, The rates of births and deaths will be represented accordingly by the superscripts \(r_b\) and \(r_d\).
The model transforms into the following when subjected to the circumstances outlined in the problem:
\(\frac{d P}{d t}=r_b P-r_d P^2\)
In conclusion, You need to submit model 1 in order to get more information.
In the event that the proportionality assumptions about birth and death be loosened up, the model that is necessary for issue 1 would become the following:
\(\frac{d P}{d t}=r_g \pm r\)
Depending on whether we are discussing immigration or emigration, we may be contemplating either of these options.
Read more about differential equation
https://brainly.com/question/14620493
#SPJ1
A 35 foot ladder is set against the side of a house so that it reaches up 28 feet. If Bentley grabs the ladder at its base and pulls it 4 feet farther from the house, how far up the side of the house will the ladder reach now?
Answers
Answer:
Step-by-step explanation:
24.5 ft
DF=28
AB=4
BF= square root 35^2-28^2
BF= 21
EF= square root 35^2-25^2
EF= 24.5
ANSWER= 24.5
If 8x+7y=6 is a true equation, what would be the value of 5+8x+7y?
Answers
If the equation 8x+7y=6 is a true equation, then the value of the equation 5+8x+7y is 11
What are linear equations?Linear equations are equations that have constant average rates of change. Note that the constant average rates of change can also be regarded as the slope or the gradient
How to determine the value of the equation?The equation is given as
8x + 7y = 6
The above equation is said to be true
The next step is to add 5 to both sides of the equation 8x + 7y = 6
So, we have
5 + 8x + 7y = 6 + 5
Evaluate the sum of the like terms
So, we have
5 + 8x + 7y = 11
Hence, if the equation 8x+7y=6 is a true equation, then the value of the equation 5+8x+7y is 11
Read more about linear equations at
https://brainly.com/question/14323743
#SPJ1
solve the system of equations by substitution
3y - 6x = 24
8 + 2x = y
Answers
Answer:
Step-by-step explanation:
y = 8 + 2x
3(8+2x) - 6x = 24
24 + 6x - 6x = 24
24 = 24
This equation is an identity, all real numbers are solutions
Find the mean, median, and mode for the following sample of scores. (8,7,5,7,0,10,2,4,11,7,8,7)
Answers
The mean, median, and mode of the given sample of scores (8, 7, 5, 7, 0, 10, 2, 4, 11, 7, 8, 7) are 6.25, 7, and 7, respectively.
To find the mean, we sum up all the scores and divide by the total number of scores. In this case, the sum of the scores is 78, and there are 12 scores. Dividing 78 by 12 gives us the mean of 6.25.
The median is the middle value of a dataset when arranged in ascending or descending order. To find the median, we first arrange the scores in ascending order: 0, 2, 4, 5, 7, 7, 7, 7, 8, 8, 10, 11. As there are 12 scores, the middle two values are 7 and 7. Therefore, the median is 7.
The mode is the value that appears most frequently in the dataset. In this case, the mode is 7 since it appears four times, more frequently than any other score.
In summary, the mean of the scores is 6.25, indicating the average value. The median is 7, representing the middle value when the scores are ordered. Lastly, the mode is 7, the value that appears most frequently in the dataset.
Learn more about median here:
https://brainly.com/question/300591
#SPJ11
C A N ~ Y O U ~ P L E A S E ~ H E L P ~ M E ~ O U T
What is the area of this trapezoid?
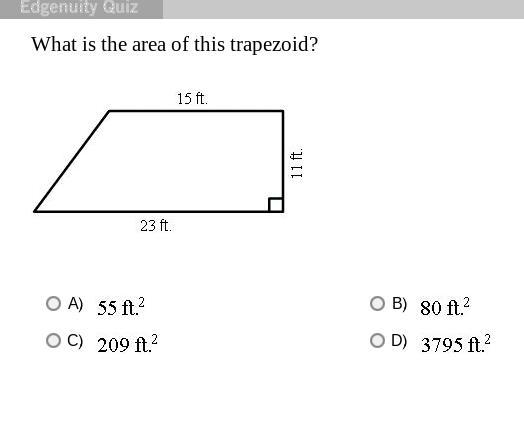
Answers
Answer:
C, 209 ft
Step-by-step explanation:
Firstly, we put it as a rectangle.
A=wl.
Width is 11
Length is 23
23 - 15 = 8. We do this to see how much feet is left in that triangle.
So 209 is our final answer.
Solve 6x+2y=26 for y.
Multiple choice question.
A)
y= –3x – 13
B)
y=3x – 13
C)
y= –3x+13
D)
y=3x+13
Answers
Answer:
The choice C) y = - 3x + 13
Step-by-step explanation:
\(6x + 2y = 26 \\ 2y = 26 - 6x \\ \\ y = \frac{26 - 6x}{2} \\ \\ \\ y = \frac{26}{2} - \frac{6x}{2} \\ \\ \\ y = 13 - 3x\)
y = - 3x + 13
I hope I helped you^_^
Answer:
C
Step-by-step explanation:
6x + 2y = 26 ( subtract 6x from both sides )
2y = - 6x + 26 ( divide terms by 2 )
y = - 3x + 13 → C
what is the slope for (-3,5),(4,-3)
Answers
To calculate the slope of a line that passes through two given points you have to use the following formula
\(m=\frac{y_1-y_2}{x_1-x_2}\)Where
m is the slope
(x₁,y₁) are the coordinates of one of the points
(x₂,y₂) are the coordinates of the second point
For the points (-3,5) and (4,-3) you can calculate the slope as follows
\(m=\frac{-3-5}{4-(-3)}=\frac{-8}{4+3}=-\frac{8}{7}\)The slope is -8/7
Answer:
Step-by-step explanation:
gradient=y2-y1÷x-x1
=-3-5÷4--3
=-8/7
y=mx+
because we have (-3,5)and(4,-3) so you can choose any bracket that you want to use , let's use bracket one that is (-3,5)
5=-8/7(-3)+c
/
scores from the sit and reach test weren't normally distributed. what is the likely cause for this lack of normal distribution?
Answers
A normal distribution can appear utterly erratic due to a lack of data. Results from tests taken in class, for instance, are often regularly distributed.
Given information;
Scores from the sit and reach test weren't normally distributed.
To find what is the likely cause for this lack of normal distribution,
⇒ A normal distribution can appear utterly dispersed due to Insufficient Data. For instance, test scores in the classroom are often regularly distributed.
To learn more about normal distribution click here:
brainly.com/question/29509087
#SPJ4
Please help bro im stuck on this for hours, ill give u so much points

Answers
-2
Explanation:
f = -19.8
17.8 + -19.8 (f) = -2
17.
Is the relation a function? Why or why not?
{(–8, –4), (–2, 0), (1, 3), (–2, 6)}
A. Yes; only one range value exists for each domain value.
B. No; the relation passes the vertical line test.
C. No; two range values exist for domain value –2.
D. Yes; two range values exist for domain value –2.
Answers
Answer:
C. No; two range values exist for domain value –2.
Step-by-step explanation:
The relation is not a function.
The -2 in the domain repeats
Since
x
=
−
2
produces
y
=
0
and
y
=
6
, the relation
(
−
8
,
−
4
)
,
(
−
2
,
0
)
,
(
1
,
3
)
,
(
−
2
,
6
)
is not a function.
The relation is not a function.
Find the distance between the points (-6,5) and (-3,1)
Answers
Answer: the rise over run is 4/3 so 4 units down since its negative and 3 units to the right.
I need help ASAP (will give brainliest)

Answers
Answer:
92°
Step-by-step explanation:
All angles should add up to 360°
Opposite angles are equal so that means two angles are 88°
88+88=176
360 - 176 = 184
184 / 2 = 95
Measure of angle A is 92°
180-88=92
Am not good at explaining
A data set has 10,000 records and 30 predictor variables (columns). Each variablehas 5% of the values missing for that individual variable. The missing values are spread randomly and independently throughout the data set. The analyst uses apredictive model that automatically drops any row (record) that has even a singlemissing values on any of the variables. How many records would be dropped fromthe analysis
Answers
10,000 records have 30 predictor variables, each with 5% of the values missing, resulting in 15,000 missing values. The predictive model drops any row with even a single missing value, so 5,000 records would be dropped from the analysis.
1. There are 30 predictor variables.
2. Each variable has 5% of the values missing.
3. 5% of 10,000 records = 500 records.
4. 30 variables x 500 records = 15,000 missing values.
5. 10,000 records - 15,000 missing values = 5,000 records dropped from the analysis.
The data set has 10,000 records and 30 predictor variables. Each variable has 5% of the values missing, so 500 records are missing for each variable. This results in 15,000 missing values spread randomly and independently throughout the data set. The analyst uses a predictive model that drops any row (record) with even a single missing value, so 5,000 records would be dropped from the analysis.
Learn more about variable here
brainly.com/question/29583350
#SPJ4
mark pushes his broken car 130 m down the block to his friend's house. he has to exert a 120 n horizontal force to push the car at a constant speed.
Answers
The work done by Mark in pushing his broken car 130 meters down the block with a 120 N horizontal force is 15,600 J (joules).
We can use the formula: Force (F) = mass (m) x acceleration (a)
Since the car is not accelerating, the acceleration is 0 m/s^2. Therefore, we can solve for the mass of the car:
120 N = m x 0 m/s^2
m = 0 kg
This is not a realistic value for the mass of a car, but it means that the car is not affected by gravity or any other forces that would cause it to accelerate.
Now, we can calculate the work done by Mark on the car:
Work (W) = force (F) x distance (d)
W = 120 N x 130 m
W = 15,600 J
Therefore, Mark has exerted a total of 15,600 joules of work to push his broken car 130 meters down the block to his friend's house.
To determine the work done by Mark in pushing his broken car 130 meters to his friend's house, we can use the formula for work: Work = Force x Distance x cos(theta). In this case, the horizontal force exerted is 120 N, and the distance is 130 m. Since Mark is pushing the car horizontally, the angle between the force and the displacement is zero degrees. The cosine of zero degrees is 1. So, the work done is Work = 120 N x 130 m x 1.
To know more about horizontal visit :-
https://brainly.com/question/27860688
#SPJ11
Find the measure of the central angle indicated. Assume that lines which appear to be diameters are actual diameters.

Answers
The measure of the central angle indicated is 270 degrees
What is mean by the term Diameter?The term "diameter" refers to a straight line segment that passes through the center of a circle or a sphere, connecting two points on the circumference or surface of the circle or sphere. In other words, it is the longest distance that can be measured between two points on the edge of the circle or sphere, passing through its center.
To find the measure of the central angle indicated, we need to first identify the endpoints of the diameter that contains points W, T, and X. Let's assume that this diameter is WX. Then, we can find the measure of the central angle WTX by finding the measure of the arc WT and dividing it by 2.
We know that the diameter WX passes through the midpoint of segment VT, which we can find by averaging the coordinates of V and T. Using the coordinates given in the diagram, we have:
V: (9x-2, 15x+10)
T: (15x+10, 9x-2)
Midpoint of VT: ((9x-2 + 15x+10)/2, (15x+10 + 9x-2)/2)
= (12x + 4, 12x + 4)
Since this midpoint lies on the diameter WX, we can find the coordinates of point X by reflecting the midpoint across the y-axis:
X: (-12x - 4, 12x + 4)
Now we can find the measure of the arc WT by finding the difference between the angles formed by radii WT and WX. Let's call the center of the circle O:
m∠WOT = 90 degrees (since WT is a diameter)
m∠WOX = 180 degrees (since WX is a diameter)
m∠TOX = m∠WOT - m∠WOX = -90 degrees
To convert this angle to a positive measure, we can add 360 degrees:
m(arc WT) = m∠WOT - m∠TOX + 360 degrees = 90 degrees - (-90 degrees) + 360 degrees = 540 degrees
Finally, we can find the measure of the central angle WTX by dividing the measure of arc WT by 2:
m∠WTX = m(arc WT)/2 = 540 degrees/2 = 270 degrees
Therefore, the measure of the central angle indicated is 270 degrees
Learn more about angle here
https://brainly.com/question/28451077
#SPJ1
~Giving tons of branliest!!~
A city receives 1 inches of snowfall every 4 hours. Write and graph a function that describes the relationship. How long does it take to receive 1 foot of snow? Use x for the independent variable and y for the dependent variable.
A function is y =
It takes _ hours to recieve 1 foot of snow
Answers
Answer:
To receive 1 foot of snow it will take about 18 hours.
Step-by-step explanation:
Given that a city receives 2 inches of snowfall every 3 hours, to write and graph a function that describes the relationship and determine how long does it take to receive 1 foot of snow, the following calculation must be performed:
1 foot = 12 inches
(12/2) x 3 = X
6 x 3 = X
18 = X
Thus, to receive 1 foot of snow it will take about 18 hours.
A third-order homogeneous linear equation and three linearly independent solutions are given below. Find a particular solution satisfying the given initial conditions. xy-3x²y +6xy-6y=0; y(1)-3, y'(1)-11. y''(1) - 20: Y₁-x. ₂-².₂-x² CO The particular solution is ytx) -
Answers
The particular solution satisfying the given initial conditions is y(x) = -5x^3 + 16x^2 - 16x + 8.
To find a particular solution, we need to solve the third-order homogeneous linear equation with the given initial conditions. The differential equation is xy - 3x^2y + 6xy - 6y = 0.
We can assume a particular solution of the form y(x) = Ax^3 + Bx^2 + Cx + D, where A, B, C, and D are constants to be determined.
Substituting this form of y(x) into the differential equation and simplifying, we obtain the following equations:
3Ax^2 + 2Bx + C = 0 (equation 1),
6Ax + 2B - 6 = 0 (equation 2),
6A - 6D = 0 (equation 3).
To determine the values of A, B, C, and D, we use the initial conditions: y(1) = -3, y'(1) = -11, and y''(1) = -20.
Substituting x = 1 into the particular solution, we get the equation -5A + 4B - 4C + D = -3 (equation 4).
Differentiating the particular solution with respect to x and substituting x = 1, we obtain -15A + 4B - 2C = -11 (equation 5).
Differentiating the particular solution again with respect to x and substituting x = 1, we have -30A + 4B = -20 (equation 6).
By solving equations 1, 2, 3, 4, 5, and 6 simultaneously, we find A = -5, B = 16, C = -16, and D = 8. Therefore, the particular solution satisfying the given initial conditions is y(x) = -5x^3 + 16x^2 - 16x + 8.
Learn more about differential equation here: brainly.com/question/32538700
#SPJ11
linear models have a constant growth rate (slope). what is the defining characteristic of exponential models?
Answers
The initial number of dice rolled = 1000
What is exponential modeling?
Based on an equation like where Y = deterioration, T = time, and A and B = parameters to be calculated by the regression approach based on historical data, the exponential model represents the degradation failure process.
Here,
Linear models are used when a phenomenon is changing at a constant rate whereas exponential models are used when a phenomenon is changing in a way that is quick at first, then more slowly, or slow at first and then more quickly. This is the defining characteristic of the exponential model.
(a)
dice(n) = 1000 × (−0.138629436ln)........... (1)
The initial number of dice rolled when n=0
Putting n=0 in (1),
dice(0) = 1000e(0) = 1000
Hence, the initial number of dice rolled = 1000
To learn more about exponential modeling from the given link
https://brainly.com/question/4519423
#SPJ4
use double integrals to find the area inside the curve r = 3 + sin(θ).
Answers
The area inside the curve r = 3 + sin(θ) is 4.5π square units.
To find the area inside the curve r = 3 + sin(θ), we can use double integrals in polar coordinates. The general formula for finding the area inside a polar curve is given by:
A = (1/2) ∫(θ2-θ1) ∫(r1^2)^(r2^2) r dr dθ
where θ1 and θ2 are the limits of integration for the angle θ, and r1 and r2 are the limits of integration for the radius r. In this case, since we want to find the area inside the curve r = 3 + sin(θ), we have r1 = 0 and r2 = 3 + sin(θ), and θ1 = 0 and θ2 = 2π (since we want to cover the full circle). Therefore, the double integral becomes:
A = (1/2) ∫(0)^(2π) ∫(0)^^(3+sinθ) r dr dθ
Evaluating the inner integral, we get:
∫(0)^^(3+sinθ) r dr = [1/2 r^2]_(0)^(3+sinθ) = 1/2 (9 + 6sinθ)
Substituting this into the double integral and evaluating the outer integral, we get:
A = (1/2) ∫(0)^(2π) 1/2 (9 + 6sinθ) dθ
= (1/4) (9(2π) + 6(∫(0)^(2π) sinθ dθ))
= (1/4) (18π) = 4.5π
Therefore, the area inside the curve r = 3 + sin(θ) is 4.5π square units.
Visit here to learn more about the area:
brainly.com/question/27683633
#SPJ11
the difference of triple a number and 8 is at most 12
Answers
The number "x" must be less than or equal to 6.67 to satisfy the given condition.
To start, let's use algebra to represent the information given in the problem. Let's say that the number we're talking about is x. Then the difference of triple a number (3x) and 8 is:
3x - 8
According to the problem, this expression is at most 12. In other words, it's less than or equal to 12. We can write this as:
3x - 8 ≤ 12
Now, let's solve for x. To do this, we'll add 8 to both sides of the inequality:
3x ≤ 20
Finally, we'll divide both sides by 3:
x ≤ 20/3
So the answer to your question is that the number (x) is at most 20/3. If we convert this to a decimal, we get:
x ≤ 6.666...
So if the difference of triple a number and 8 is at most 12, then the number itself is at most 6.666... (rounded to 150 decimal places).
"the difference of triple a number and 8 is at most 12" can be represented as an inequality. Using the terms "long answer" and "150", let me provide a concise explanation.
Let "x" be the number in question. Then, the difference of triple that number and 8 can be written as 3x - 8. Since the difference is "at most 12", the inequality can be represented as:
3x - 8 ≤ 12
To solve this inequality, follow these steps:
1. Add 8 to both sides:
3x ≤ 20
2. Divide both sides by 3:
x ≤ 20/3 ≈ 6.67
To know more about inequality visit:-
https://brainly.com/question/30231190
#SPJ11
if -48=(a), Then a =
Answers
Answer:
A = -48....................
Answer: -48
Step-by-step explanation:
-48 = a, so a = -48
Volume of Composed Figures-Quiz-Level G
Isaac's grandmother is teaching him how to make an egg casserole. Isaac pours the
mixture into the pan shown. Isaac's grandmother tells him he should stop when the
mixture is inch from the top, to allow room for the casserole to rise in the oven.
2
1
What is the volume, V, of the mixture that
Isaac should pour into the pan?
V = ||
in.³
9 in.
9 in.
2 in

Answers
So, Isaac should pour 81 cubic inches of mixture into the pan.
What is volume?Volume is a physical quantity that refers to the amount of space occupied by an object or a substance. It is typically measured in cubic units, such as cubic meters, cubic centimeters, or cubic feet.
The volume of an object or substance can be determined by measuring its length, width, and height (in the case of a solid object), or by measuring the amount of space it occupies (in the case of a liquid or gas).
In mathematical terms, the volume of an object is given by the formula V = l x w x h, where l is the length, w is the width, and h is the height. For irregularly shaped objects, the volume may be determined by displacement - that is, by measuring the amount of water or other fluid displaced by the object when it is immersed in the fluid.
To find the volume of the mixture that Isaac should pour into the pan, we need to find the volume of the pan itself and subtract the volume of the space that needs to be left empty.
The pan is in the shape of a rectangular prism, so its volume is:
V_pan \(= l *w * h = 9 in. * 9 in. * 2 in. = 162 in^{3}\)
To leave room for the casserole to rise, Isaac should only fill the pan to a height of 1 inch. So, the volume of the space that needs to be left empty is:
V_empty \(= l *w * h = 9 in. * 9 in. * 2 in. = 81 in^{3}\)
Therefore, the volume of the mixture that Isaac should pour into the pan is:
V = V_pan - V_empty\(= 162 in^{3} - 81 in^{3} = 81 in^{3}\)
To know more about the volume, visit:
https://brainly.com/question/13338592
#SPJ1
BEDMAS QUESTIONS
Solve the following.
Show your work.
Round you asnwers to the nearest hundredth

Answers
Using BODMAS, the solutions of the expressions are: (1) 9, (2)-12, (3) 0.27, and (4) 0.24
What is BODMAS?We should know that BODMAS is a mathematical approach which means
B=BracketO=OffD=DivisionM=MultiplicationA=Addition S=SubtractionIn each of the expressions, we must follow the approach
1) \(\frac{(6+3)^{2} }{3*9}\)
Simplifying this we have 9²/9=81/9
=9
2) \(\frac{3^{2} -6*2}{(12-8)/2^{2} *10}\)
Using BODMAS we have
(9-12)/4/40
-3÷1/9
⇒-3*4
=-12
(3) \(\frac{4.5+2*2.5^{2}-6 }{8*4+9}\)
Using BODMAS we have
(4.5+2*6.25-6)/32+9
⇒(4.5+12.5-6)/41
=11/41=0.27
4 (\(\frac{3.5+16-2.5^{2} }{10.5-6*11}\))
Using BODMAS we have
(3.5+16-6.25)/10.5-66
Simplifying
(13.25)/-55.5
=0.24
Therefore, the values when simplified are (1) 9, (2)-12, (3) 0.27, and (4) 0.24
Learn more about BODMAS on https://brainly.com/question/29626866
#SPJ1
John took all his money from his savings account. He spent $88 on a radio and 1/4 of what was left on presents for his friends. Of the money remaining, John put 4/11 into a checking account and the last remaining $420 was left to charity. How much money did John originally have in his savings account?
Answers
Answer:
$968
Step-by-step explanation:
x= the money John originally had in his savings account
The statement says that John spent $88 on a radio and 1/4 of what was left on presents for his friends. This means that the remaining money is 3/4 of the total amount minus the money spent on the radio:
3/4(x-88)
Then, it says that of the money remaining, John put 4/11 into a checking account and the last remaining $420 was left to charity. Since, he spent 4/11 of the remining 3/4(x-88), the remaining is 7/11 of that and that remaining is equal to $420 that was the money left at the end:
7/11(3/4(x-88))= 420
21/44(x-88)=420
21/44x-42=420
21/44x=462
x=968
According to this, the money that John originally had in his savings account was $968.
He spent $88 on a radio: 968-88= 880
1/4 of what was left on presents for his friends: 880/4= 220
880-220=660
Of the money remaining, John put 4/11 into a checking account: 660*(4/11)=240
the last remaining $420 was left to charity: 660-240= 420
If I1 ⊇ I2 ⊇ .... In ⊇... is a nested sequence of intervals and if In = [an; bn], show that a1 ≤ a2 ≤ ....... ≤ an ≤ ........ and b1 ≤ b2 ≤..... bn ≤ ......
Answers
The intervals are nested, each subsequent interval is contained within the previous one. Mathematically, this means I₁ ⊇ I₂ ⊇ ... In ⊇ ... . Therefore, we have:
1. I₁ ⊇ I₂ implies [a₁; b₁] ⊇ [a₂; b₂], which means a₁ ≤ a₂ and b₁ ≥ b₂.
2. I₂ ⊇ I₃ implies [a₂; b₂] ⊇ [a₃; b₃], which means a₂ ≤ a₃ and b₂ ≥ b₃.
To show that a1 ≤ a2 ≤ ... ≤ an ≤ ..., we need to use the fact that the sequence of intervals is nested, meaning that each interval is contained within the next one.
First, we know that I1 contains I2, so every point in I2 is also in I1. That means that a1 ≤ a2 and b1 ≥ b2.
Now consider I2 and I3. Again, every point in I3 is also in I2, so a2 ≤ a3 and b2 ≥ b3.
We can continue this process for all the intervals in the sequence, until we reach In. So we have:
a1 ≤ a2 ≤ ... ≤ an-1 ≤ an
and
b1 ≥ b2 ≥ ... ≥ bn-1 ≥ bn
This shows that the endpoints of the intervals are ordered in the same way.
Given that I₁ ⊇ I₂ ⊇ ... In ⊇ ... is a nested sequence of intervals and In = [an; bn], we can show that a₁ ≤ a₂ ≤ ... ≤ an ≤ ... and b₁ ≥ b₂ ≥ ... ≥ bn ≥ ... as follows:
Since the intervals are nested, each subsequent interval is contained within the previous one. Mathematically, this means I₁ ⊇ I₂ ⊇ ... In ⊇ ... . Therefore, we have:
1. I₁ ⊇ I₂ implies [a₁; b₁] ⊇ [a₂; b₂], which means a₁ ≤ a₂ and b₁ ≥ b₂.
2. I₂ ⊇ I₃ implies [a₂; b₂] ⊇ [a₃; b₃], which means a₂ ≤ a₃ and b₂ ≥ b₃.
Continuing this pattern for all intervals in the sequence, we can conclude that a₁ ≤ a₂ ≤ ... ≤ an ≤ ... and b₁ ≥ b₂ ≥ ... ≥ bn ≥ ... .
Visit here to learn more about sequence : https://brainly.com/question/30262438
#SPJ11
Line f has an equation of 6x + y = 7. Perpendicular to line f is line g, which passes through the point (-10, -1). what is the equation of line g
(Write the equation in slope-intercept form. Write the numbers in the equation as simplified proper fractions, improper fractions, or integers.)
Answers
Answer:
y = (1/6)x - 5/3
Step-by-step explanation:
Please find the attachment for detail