x^2
- 3x - 18 < 0
41. \( x^{2}-3 x-18 \leq 0 \)
Answers
41. \( x^{2}-3 x-18 \leq 0 \) gives the solution interval as x∈(−∞,−3]∪[6,∞).
To solve the inequality x² - 3x - 18 < 0, you can factor the quadratic expression and use the sign chart method. Here's the step-by-step process:
Step 1: Factor the quadratic expression
To factor x² - 3x - 18, you need to find two numbers whose product is -18 and whose sum is -3. Those numbers are -6 and 3. Therefore, x² - 3x - 18 = (x - 6)(x + 3).
Step 2: Determine the critical values
The critical values are the values of x that make the expression zero. In this case, the critical values are
x = 6 and
x = -3,
since (x - 6)(x + 3) = 0
when x = 6 or
x = -3.
Step 3: Create a sign chart
To create a sign chart, draw a number line and place the critical values on it. Then test a value in each interval to see if the expression is positive or negative. Write a plus sign (+) above the line if the expression is positive and a minus sign (-) if the expression is negative.
Interval x² - 3x - 18(x - 6)(x + 3) < 0x < -3 (-)(-)(+) < 0-3 < x < 6 (-)(+)(+) > 06 < x (+)(+)(+) > 0
Step 4: The solution is the interval(s) where the expression is negative, which is the interval between the critical values: -3 < x < 6.
Therefore, the inequality x² - 3x - 18 < 0 is equivalent to x ∈ (-3, 6).
Answer: 41. \( x^{2}-3 x-18 \leq 0 \) gives the solution interval as x∈(−∞,−3]∪[6,∞).
To know more about interval visit
https://brainly.com/question/11051767
#SPJ11
Related Questions
ore time on the Internet: A researcher polled a sample of 1012 adults in the year 2010 , asking them how many hours per week they spent on the Internet. The sample mean was 10.01 with a standard deviation of 13.90. A second sample of adults was taken in the year . For this sample, the mean was with a standard deviation of . Assume these are simple random samples from populations of adults. Can you conclude that the mean number of hours per week spent on the Internet differs between and
Answers
Sample 1: Mean=10.01, Standard deviation=13.90 Sample 2: Mean
=11.43, Standard deviation
=14.10
Sample size of 1st year = n1
= 1012Mean of 1st year sample
= X1 = 10.01Standard deviation of 1st year sample
= s1
= 13.90Sample size of 2nd year
= n2
= 1012Mean of 2nd year sample
= X2
= 11.43
Standard deviation of 2nd year sample = s2
= 14.10 Let us assume a significance level of α = 0.05, which implies that the critical region consists of 2.5% in both tails (since it is a two-tailed test).
Therefore, we do not have sufficient evidence to conclude that the mean number of hours per week spent on the Internet differs between 2010 and another year.
To know more about deviation, visit:
https://brainly.com/question/23907081
#SPJ11
ILL GIVE U BRAINLISTTT!!!!!

Answers
Hi
exponnential cannot be negative. So A is out
quadratic function cannot also. So B is out.
C is the only one remaining...
Answer: the new one is A. Sorry i mistaked C but ik A is right. I re-did the problem
I hope i helped and can i be marked brainliest if i got it right?
if i did not get it right, therefore please forgive me please.
Students in 10th and 11th grade were asked whether they have a job. The raw data was converted to relative frequencies and recorded in the table below. A 4-column table with 3 rows. The first column has no label with entries job, no job, total. The second column is labeled tenth grade with entries 0. 14, 0. 86, 1. The third column is labeled eleventh grade with entries 0. 25, 0. 75, 1. The fourth column is labeled total with entries 0. 20, 0. 80, 1. Given the conditional relative frequency table by column, what does the 0. 14 represent? Given that a student is a 10th grader, there is a 14% chance he or she has a job. Given that a student has a job, there is a 14% chance he or she is a 10th grader. 14% of the students surveyed are 10th graders who have a job. 14% of the students are 10th graders.
Answers
Option A is correct because 0.14 represents that if a student is a 10th grader, there is a 14% chance he or she has a job.
It is given that there is a table with 4 columns and 3 rows.
What is a conditional relative frequency table?It is a special type of frequency table that shows relationships between two categories.
From the attached table it is clear that 0.14 is the probability of getting a job if a student is a 10th grader,
Option B is incorrect, stating there is a 14% chance a student is a 10th grader if he/she has had a job because we do not know the number of students.
Option C is also incorrect stating that 14% of the students surveyed are 10th graders who have a job because we do not know the total number of students.
Option D is also incorrect because we do not know the number of students.
Therefore,Option A is correct because 0.14 represents that if a student is a 10th grader, there is a 14% chance he or she has a job.
To get more about conditional relative frequency, refer to the link,
https://brainly.com/question/22728236

Answer: A
Step-by-step explanation:
A) Using Integration By Parts, Find ∫Xsin(2x−1)Dx. (6) (B) Use Substitution Method To Find ∫2x−1x2dx, Giving Your Answer In
Answers
The values of integral:
A) ∫x sin(2x - 1) dx = -1/2 x cos(2x - 1) + 1/4 sin(2x - 1) + C B) ∫(2x - 1)/x^2 dx = -(x - 1)/(2x) + C
A) To find ∫x sin(2x - 1) dx using integration by parts, we can use the formula:
∫u dv = uv - ∫v du
Let's choose u = x and dv = sin(2x - 1) dx.
Differentiating u, we get du = dx, and integrating dv, we get v = -1/2 cos(2x - 1).
Applying the integration by parts formula, we have:
∫x sin(2x - 1) dx = -1/2 x cos(2x - 1) - ∫(-1/2 cos(2x - 1)) dx
Simplifying the integral on the right-hand side, we have:
∫x sin(2x - 1) dx = -1/2 x cos(2x - 1) + 1/4 sin(2x - 1) + C
Therefore, ∫x sin(2x - 1) dx = -1/2 x cos(2x - 1) + 1/4 sin(2x - 1) + C.
B) To find ∫2x - 1/x^2 dx using the substitution method, we can let u = 2x - 1.
Differentiating u with respect to x, we get du = 2 dx.
Rearranging the equation, we have dx = du/2.
Substituting these values into the integral, we have:
∫(2x - 1)/x^2 dx = ∫(u)/(x^2)(du/2)
Simplifying the integral, we have:
∫(2x - 1)/x^2 dx = ∫(u)/(2x^2) d
Breaking the fraction apart, we have:
∫(2x - 1)/x^2 dx = ∫(u)/(2x^2) du = (1/2) ∫(u)/(x^2) du
Integrating with respect to u, we get:
∫(2x - 1)/x^2 dx = (1/2) ∫(u)/(x^2) du = (1/2) (-u/x) + C
Substituting back u = 2x - 1, we have:
(2x - 1)/x^2 dx = (1/2) (-2x + 1)/x + C
Simplifying further, we get:
∫(2x - 1)/x^2 dx = -(x - 1)/(2x) + C
Therefore, ∫(2x - 1)/x^2 dx = -(x - 1)/(2x) + C.
To know more about integral,
https://brainly.com/question/32387684
#SPJ11
Nicole bought a microwave that was originally priced for $950
but it was 30% off. His sales tax is 7%. How much was his total bill
for this microwave?
Answers
665+7%=711.55
Total bill for microwave =711.55
Which of the following equations will have a positive solution?
n+1 1/2= -3/4
n-3/4= -1 1/2
n-3/4= 1 1/2
n+1 1/2= 3/4
Answers
Answer:
Option C; n-3/4= 1 1/2
Step-by-step explanation:
n-3/4= 1 1/2
+3/4 +3/4
n=2 1/4
a concave mirror has a focal length of 16 cm . at what object distance will the magnification be -2.0?
Answers
The object distance is 0, which implies that the object is at the focus of the concave mirror.
For a concave mirror, the magnification is given by the formula:
m = -di/do
where m is the magnification, di is the image distance, and do is the object distance. Since we are given that the magnification is -2.0, we can write:
-2.0 = -di/do
Simplifying this expression, we get:
di = 2do
We can also use the mirror formula for a concave mirror:
1/f = 1/do + 1/di
where f is the focal length of the mirror. Substituting di = 2do and f = -16 cm (since the mirror is concave), we get:
1/-16 = 1/do + 1/(2do)
Multiplying both sides by -16do, we get:
do - 2f = -32
Substituting f = -16 cm, we get:
do - (-32) = -32 + 32
do = 0
This means that the object distance is 0, which implies that the object is at the focus of the concave mirror. This is a valid result, since a concave mirror can form a real, inverted image for an object placed at a distance equal to its focal length. In this case, the magnification would be -1, not -2. So, it is not possible to have a magnification of -2 for an object distance in front of a concave mirror with a focal length of 16 cm.
To learn more about concave mirrorvisit:https://brainly.com/question/3555871
#SPJ11
jessica has four small boxes that are the same size and one large box. Each of the four small boxes is 4 centimeters wide and 2 centimeters long. The large box has a volume of 96 cubic centimeters. jessica puts the four small boxes into the large box. there’s no space leftover.
What is the height of the one small box?
Answers
Answer:
H = 3
Step-by-step explanation:
V of Large box = 4 x (V of Small box)
96/4 = 24
24 = V Small box.
V = L x W x H
24 = 2 x 4 x H
24 = 8 x H
3 = H
Therefore height of small box is 3.
The height of the one small box is 4 cm if Jessica puts the four small boxes into the large box. there’s no space leftover.
What is a cuboid?It is defined as the six-faced shape, a type of hexahedron in geometry.
It is a three-dimensional shape.
Jessica has four small boxes that are the same size and one large box.
The dimensions for the small boxes:
Width w = 4 cm
Length l = 2 cm
Volume of a large box = 96 cubic centimetres
As Jessica puts the four small boxes into the large box. there’s no space leftover, mathematically,
The volume of the large box = 4(Volume of the small boxes)
The volume of the large box = 4(l×w×h)
Where h is the height of the small box.
96 = 4(3×2×h)
24h = 96
h = 4 cm (divide by 48 on both sides)
Thus, the height of the one small box is 4 cm if Jessica puts the four small boxes into the large box. there’s no space leftover.
Learn more about the cuboid here:
brainly.com/question/9740924
a certain job can be done in 20 hours by 4 people. how many people are needed to do the same job in 10 hours?
Answers
To find out how many people are needed to do this job in 10 hours, we can see that we got to 10 hours by dividing 20 by two so, to see how many people are needed to complete this same job in ten hours, we would just divide 4 people by 2 which is 2 people.
So, in conclusion, if it takes a certain job to be done in 20 hours by 4 people, then it would take 10 hours for that same job to be completed by 2 people.
Glad I could help!
Holly picks 125 she spends 35 on gas for her car
which model represtant how much money holly spent
Answers
The second option is the correct representation of the given context.
What is a number line?A number line is a one-dimensional horizontal line where we can represent any real number.
The origin of the number line is represented by zero left to it are all negative real numbers and right to it are all positive real numbers.
Look at the model, it starts at 125 and drops to 90.
If she has $125 and spends $35 on gas, the remaining balance is reduced by that amount.
125 - 35 = 90.
Q. Holly has $125 she spends $35 on gas for her car which model represents how much money holly has left?
learn more about number line here :
https://brainly.com/question/13425491
#SPJ1

PICTURE PROVIDED JUST TELL ME IF IT IS A FUNCTION AND WHY

Answers
Answer:
This is a function because the Y values do not repeat.
Referring to Table 1, what is the predicted consumption level for an economy with GDP equal to $4 billion and an aggregate price index of 150? a. $1.39 billion ...
Answers
The predicted consumption level for an economy with a GDP of $4 billion and an aggregate price index of 150 is $2.07 billion.
Referring to Table 1, the predicted consumption level for an economy with a GDP equal to $4 billion and an aggregate price index of 150 is $1.39 billion.
In Table 1, we can observe the relationship between GDP and the corresponding consumption levels for different aggregate price indexes. To find the predicted consumption level, we need to locate the row in the table that corresponds to an aggregate price index of 150. In this case, we find the row where the aggregate price index is 150.
Looking at the row with an aggregate price index of 150, we can see that the corresponding consumption level is $2.33 billion. However, this value represents the consumption level for an economy with a GDP of $3 billion. Since we need to find the predicted consumption level for an economy with a GDP of $4 billion, we need to adjust the value accordingly.
To adjust the consumption level, we can use the concept of proportionality. We observe that the consumption level increases linearly with GDP. Therefore, we can calculate the predicted consumption level by scaling the consumption level of $2.33 billion proportionally to the change in GDP.
The ratio of the new GDP ($4 billion) to the original GDP ($3 billion) is 4/3. Multiplying this ratio by the consumption level of $2.33 billion, we get:
($4 billion) / ($3 billion) * ($2.33 billion) = $3.11 billion
However, it's important to note that this adjusted consumption level is for an economy with an aggregate price index of 100. Since the given economy has an aggregate price index of 150, we need to adjust the consumption level based on the change in the price index.
The ratio of the new price index (150) to the base price index (100) is 150/100 = 1.5. Dividing the adjusted consumption level by this ratio, we find:
($3.11 billion) / 1.5 = $2.07 billion
Therefore, the predicted consumption level for an economy with a GDP of $4 billion and an aggregate price index of 150 is $2.07 billion.
Please note that the predicted consumption level is an estimate based on the relationship observed in the data provided in Table 1. It assumes a linear relationship between GDP and consumption, and it should be interpreted as a rough prediction rather than an exact value.
Learn more about GDP here
https://brainly.com/question/1383956
#SPJ11
the sum of three consecutive multiples of 2 is 18 .
Answers
Answer:
consecutive multiple of 2 is 2,4,6
this sum is 2+4+6 is 12
Do the ratios
5
3
and
20
12
form a proportion
Answers
Answer:
5:3
20:12 they're not proportional
Answer:
yes i think so
Step-by-step explanation:
5 10 15 20
3 6 9 12
so yes they are even
Multiply 3 by the number of apples in each bag x and add it to 3 times the number of bananas in each bunch y an then multiply that sum by 3
Answers
Answer:
9(x+y)
(3x + 3y) 3
help meeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeee

Answers
Answer: 9.7 seconds
Step-by-step explanation:
\(16t^2=1503\\\\t^2 =\frac{1503}{16}\\\\t=\sqrt{1503/16} \text{ } (t > 0)\\\\t \approx 9.7\)
helpp ill mark u as brainlist

Answers
Answer:
Proportional graph
Step-by-step explanation:
It is proportional because as the x increases, the y also increases. It maintains the same rate of change.
Answer:
non pro becuase it dont pass the origin
Step-by-step explanation:
Find the area of each polygon in square units 7-4

Answers
3×4 + ½ ×3×4 + 1×4 + ½×3×4
12 + 6 + 4 + 6
18+ 10
28units²
What is the difference in the = between (1, 7) and (3, 11)? What is half that difference?
Answers
Answer:
2
1
Step-by-step explanation:
I will assume you want to find the slope [ y2-y1/x2-x1 ]
11-7/3-1
4/2
2
Hald the distance.
2/1 = 1
Best of Luck!
FILL IN THE BLANK. The _______ method first quantizes the object space into a finite number of cells that form a _________ structure and then performs clustering on the ________ structure.
Answers
The grid-based method first quantizes the object space into a finite number of cells that form a grid structure and then performs clustering on the grid structure.
The grid-based methods are used in data mining and are based on a multi-resolution grid data structure. In the grid-based methods the space of instance is divided into a grid structure. Following the division, clustering techniques are employed using the cells of the grid as the base units instead of individual data points. The great advantage of grid-based clustering technique is its significant reduction of the computational complexity, especially for clustering very large data sets and improving the processing time.
Learn more about Data mining:
https://brainly.com/question/14755446
#SPJ4
Solve for d.
85 = 5(d − 68)
Answers
Answer:
Step-by-step explanation:
5(d - 68) = 85
Use distributive property
5*d - 68*5 = 85
5d - 340 = 85
Add 340to both sides
5d = 85 + 340
5d = 425
Divide both side by 5
d = 425/5
d = 85
Can any one please help me in this the teacher did not explain this it have to be in the lowest term also no decimal answer thank you please help me

Answers
Answer:
a. -5 + 3x = -41
3x = -36
x = -12
b. 7x - 4 = -2x + 11
9x = 15
x = 15/9 = 1 and 2/3
c. (-4x) / 5 = 8
-4x = 40
x = -10
d. x / 3 - 1/2 = 1/4
4x - 6 = 3 (multiply by lcm of 3, 2 and 4 which is 12)
4x = 9
x = 9/4 = 2 and 1 / 4
e. -4(6x + 1) + 3 = 11 + 2(x + 2)
-24x - 4 + 3 = 11 + 2x + 4
-24x - 1 = 2x + 15
-26x = 16
x = -8/13
A road running north to south crosses a road going east to west at the point P. Car A is driving north along the first road, and an airplane is flying east above the second road. At a particular time the car is 15 kilometers to the north of P and traveling at 60 km/hr, while the airplane is flying at speed 185 km/hr 10 kilometers east of P at an altitude of 2 km. How fast is the distance between the car and the airplane changing?
Answers
Answer:
D^2 = (x^2 + y^2) + z^2
and taking derivative of each term with respect to t or time, therefore:
2*D*dD/dt = 2*x*dx/dt + 2*y*dy/dt + 0 (since z is constant)
divide by 2 on both sides,
D*dD/dt = x*dx/dt + y*dy/dt
Need to solve for D at t =0, x (at t = 0) = 10 km, y (at t = 0) = 15 km
at t =0,
D^2 = c^2 + z^2 = (x^2 + y^2) + z^2 = 10^2 + 15^2 + 2^2 = 100 + 225 + 4 = 329
D = sqrt(329)
Therefore solving for dD/dt, which is the distance rate between the car and plane at t = 0
dD/dt = (x*dx/dt + y*dy/dt)/D = (10*190 + 15*60)/sqrt(329) = (1900 + 900)/sqrt(329)
= 2800/sqrt(329) = 154.4 km/hr
154.4 km/hr
Step-by-step explanation:
The graph shows the amount of a medicine min milligrams, remaining in a patient's body hours after receiving an injection . The amount of the medicine decreases exponentially
a.By what factor did the medicine decrease in the hour and a half?
b. By what factor did the medicine decrease in the first half hour?
—What about in the first hour?
C. Write an equation relating m, the number of milligrams of the drug in the patient's body, and hthe number of hours since the injection
Answers
An exponential function is a function defined by y = ab^x, where a represents the initial value, and b represents the rate
(a) The factor at which the medicine decreased, in 1.5 hourFrom the graph, we have the following ordered pairs
(x,y) = (0,270) and (1.5,80)
Given that:
\(y = ab^x\)
At point (0,270), we have:
\(ab^0=270\)
\(a= 270\)
At point (1.5,80), we have:
\(ab^{1.5} = 80\)
Substitute 270 for a
\(270b^{1.5} = 80\)
Divide both sides by 270
\(b^{1.5} = 0.2963\)
Take 1.5th root of both sides
\(b =0.44\)
Hence, the medicine decrease in the hour and half at a factor of 0.44
(b) The factor at which the medicine decreased in the first half hour, and the first hourIn (a), we have:
The decay factor (b) to be 0.44
This represents the factor at which the medicine decreased throughout.
Hence, the medicine decrease in the first half hour, and the first hour at a factor of 0.44
(c) The exponential equationIn (a), we have:
\(a= 270\)
\(b = 0.44\)
So, the exponential equation is:
\(y =270 * 0.44^x\)
In terms of m and h, we have:
\(m =270 * 0.44^h\)
Hence, the equation relating m to h is \(m =270 * 0.44^h\)
Read more about exponential equations at:
https://brainly.com/question/11832081
Help me on this final question
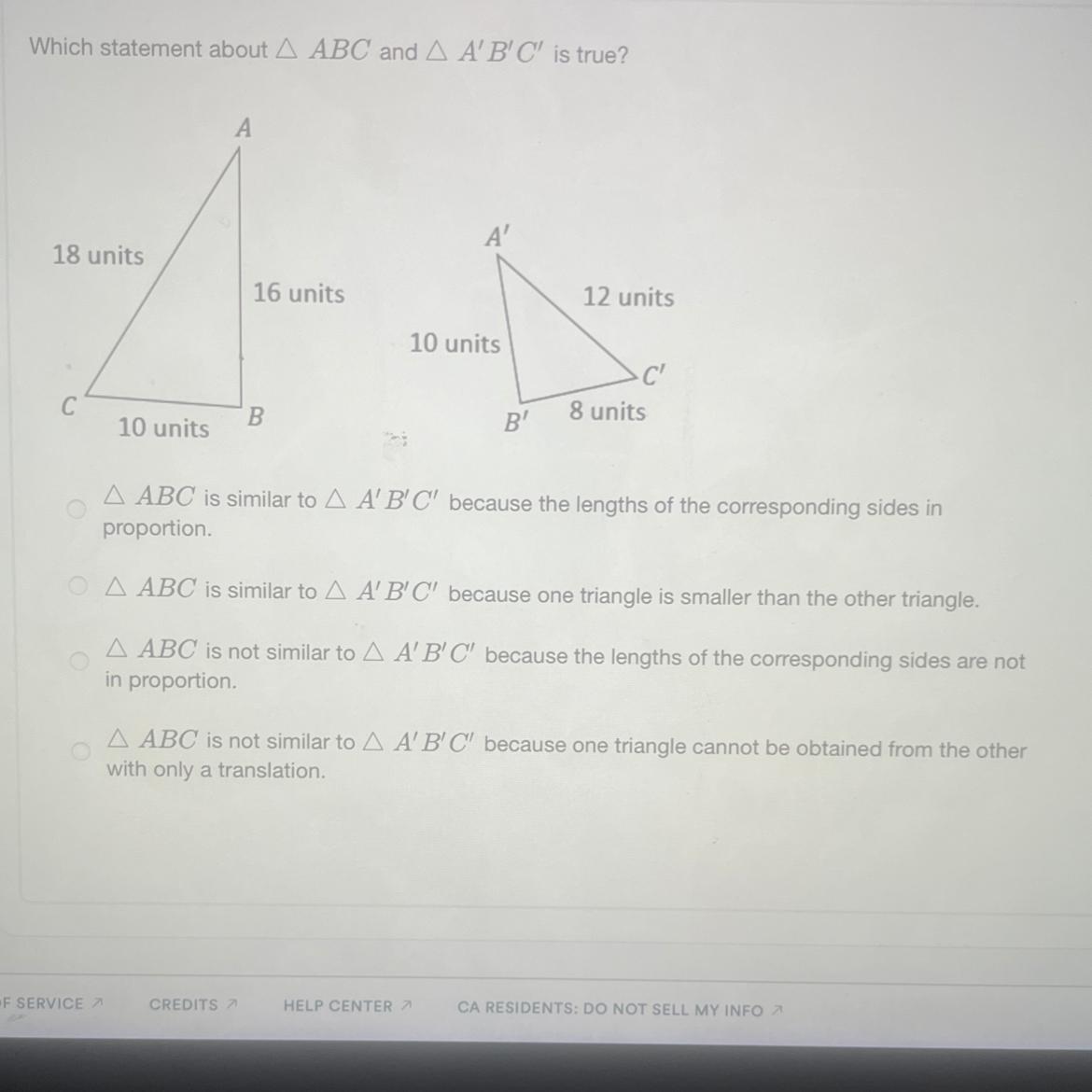
Answers
ΔABC and ΔA'B'C' are not similar because the length of corresponding sides are not in proportion.
We have two Triangles as ΔABC and ΔA'B'C'
Now, In ΔABC and ΔA'B'C'
AB / A'B = 16 /10 = 8/5
CB / C'B' = 10/8 = 5/4
AC / A'C' = 18/12 = 3/2
So, the triangle ΔABC and ΔA'B'C' are not similar.
Thus, ΔABC and ΔA'B'C' are not similar because the length of corresponding sides are not in proportion.
Learn more about Similarity here:
https://brainly.com/question/26451866
#SPJ1
I need help ASAP!!Please make sure u sure the answer is correct.

Answers
Answer:
(x-8)^2+(y-16)^2 = 4
Step-by-step explanation:
The equation of a circle is given by
(x-h)^2+(y-k)^2 = r^2 where (h,k) is the center and r is the radius
(x-8)^2+(y-16)^2 = 2^2
(x-8)^2+(y-16)^2 = 4
Solve the following IVP with the Laplace transform y" – 6y' + 13y = 16te3t y(0) = 4 y'(0) = 8
Answers
The given differential equation is solved using the Laplace transform method. After taking the Laplace transform and simplifying the equation, we find the expression for the Laplace transform of the solution.
To solve the given initial value problem (IVP) using the Laplace transform, we will follow these steps:
Step 1: Take the Laplace transform of both sides of the differential equation.
Applying the Laplace transform to the equation y" - 6y' + 13y = 16te^3t, we get:
s^2Y(s) - sy(0) - y'(0) - 6(sY(s) - y(0)) + 13Y(s) = 16L{te^3t}
Using the initial conditions y(0) = 4 and y'(0) = 8, we can simplify the equation as follows:
s^2Y(s) - 4s - 8 - 6sY(s) + 24 + 13Y(s) = 16L{te^3t}
(s^2 - 6s + 13)Y(s) - 4s - 16 = 16L{te^3t}
Step 2: Solve for Y(s).
Combining like terms and rearranging the equation, we have:
(s^2 - 6s + 13)Y(s) = 4s + 16 + 16L{te^3t}
Dividing both sides by (s^2 - 6s + 13), we get:
Y(s) = (4s + 16 + 16L{te^3t}) / (s^2 - 6s + 13)
Step 3: Find the inverse Laplace transform of Y(s) to obtain the solution y(t).
Taking the inverse Laplace transform of Y(s), we get:
y(t) = L^(-1){(4s + 16 + 16L{te^3t}) / (s^2 - 6s + 13)}
To solve this inverse Laplace transform, we can use tables of Laplace transforms or a Laplace transform calculator to find the expression in terms of t. The resulting expression will be the solution to the given IVP.
Learn more about Laplace transform : brainly.com/question/30759963
#SPJ11
The graph of a function f(x) passes through the following points:
(0,2), (1, 0), (-1,-4)
Which of the following could be f(x)?
O f(x) = 2x - 2
O f(x) = 2x² - 2
○ f(x) = - 2x - 2
○ f(x) = 2√x-2
Answers
The function is f(x) = -2x² + 2 if the function f(x) passes through the following points:(0,2), (1, 0), (-1,0)
What is a function?It is defined as a special type of relationship, and they have a predefined domain and range according to the function every value in the domain is related to exactly one value in the range.
The question is incomplete.
The complete question is in the picture, please refer to the attached picture.
We have:
The graph of a function f(x) passes through the following points: (0,2), (1, 0), (-1,0)
Let
f(x) = ax² + bx + c
Plug x = 0, and y = 2
c = 2 ...(1)
Plug x = 1 and y = 0
a + b + c = 0 ..(2)
Plug x = -1 and y = 0
a - b + c = 0 ...(3)
After solving (1), (2), and (3)
a = -2
b = 0
c = 2
f(x) = -2x² + 2
Thus, the function is f(x) = -2x² + 2 if the function f(x) passes through the following points:(0,2), (1, 0), (-1,0)
Learn more about the function here:
brainly.com/question/5245372
#SPJ1


Kim’s age is one less than twice Mike’s age. If the sum of their ages is 32, what is Mike’s age?
Answers
11•2=22-1=21
21+11=32
How many different 5-digit PIN codes are there that only include the digits 6, 2, 5, 4, 8 and 7?
Answers
Answer:
First, there are NO 5-digit pin codes that include the digits 7, 2, 4, 6, 9, and 3. But, there are 720 pin codes that include 5 out of the 6 digits.