Calculate the mean for the given data set: 3.0 3.5 3.5 4.1 4.8 5.2 7.1 11.2 Round your answer to 1 decimal place.
Calculate the median for the given data set: 3.0 3.5 3.5 4.1 4.8 5.2 7.1 11.2 Round your answer to 2 decimal places.

Answer:

10.9

Step-by-step explanation:

4 1/2 plus 6 2/5=10.9

Answer: 10.9

Step-by-step explanation:

In the first circle, 12 seeds can be planted. And to plant 20 seeds, the diameter must be 12.74ft.

We know that the flower seeds will be planted on a circle of diameter of 8ft. The circumference of said circle will be:

C = pi*D = 3.14*8ft = 25.12ft

And each seed is 2ft apart from seeds on the other side, so the maximum number of seeds that can be planted is:

N = 25.12ft/2ft = 12.56

Which must be rounded to 12, as we can't plant a 0.56 of a seed.

Now, we need to find the diameter of a circle such that 20 seeds can be planted there.

To plant 20 seeds, the circumference needed is:

20*2ft = 40ft

Then, for a circle of diameter D, we need that:

3.14*D = 40ft

D = 40ft/3.14 = 12.74ft

The diameter of the circle must be 12.74ft

If you want to learn more about circles, you can read:

https://brainly.com/question/1559324

We know that the length of a rectangle is twice its width. Then:

The area of a rectangle is the product of its width and length. Then:

Additionally, we know that the area is 162 in². Using the expression above we can obtain the value of x:

Finally, the perimeter (2P) is just twice the sum of the width and the length of the rectangle:

Step-by-step explanation:

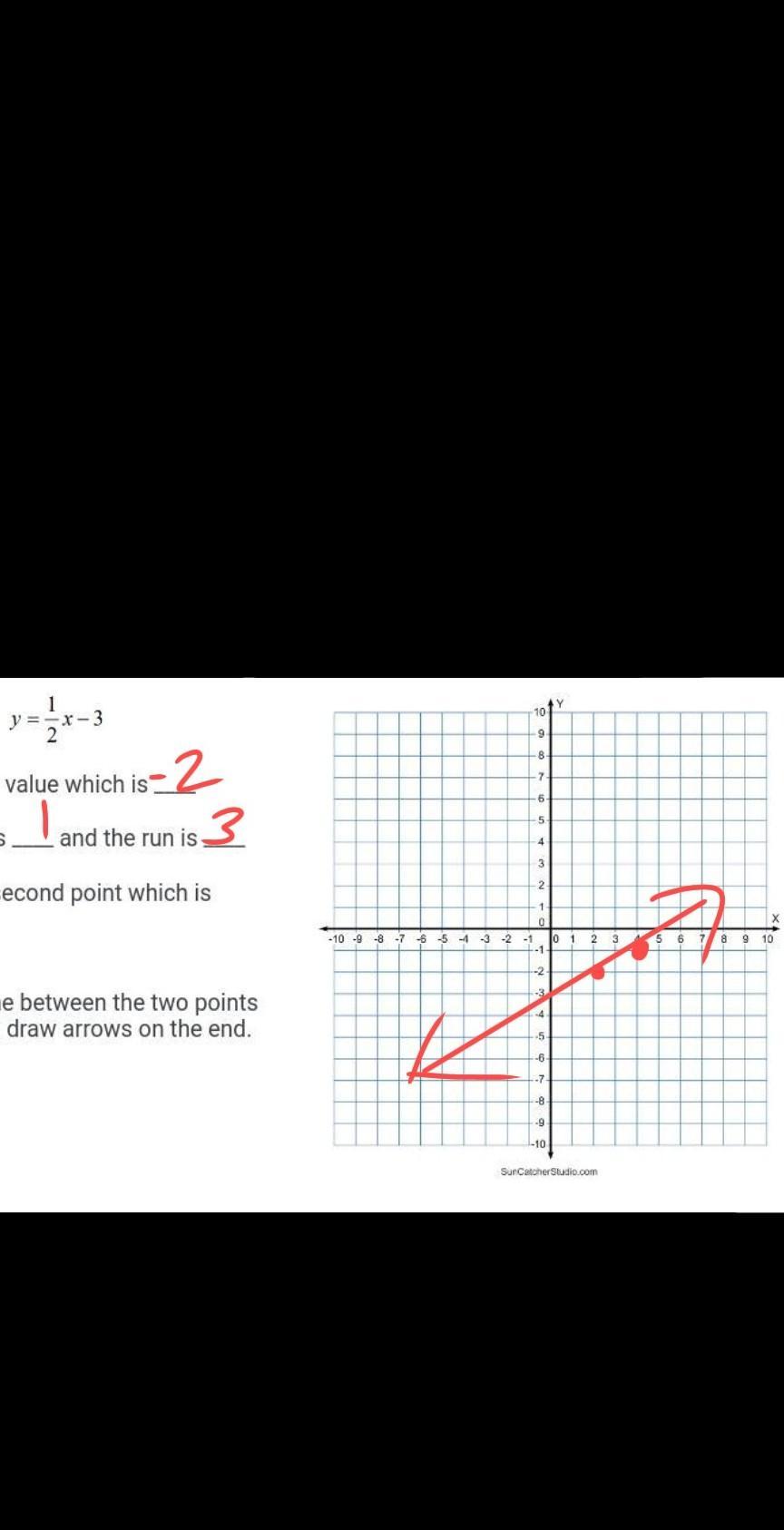
Number 3 is -2,4. ..................

The probability that a box of 40 light bulbs will last for 5 years is very low, due to the short mean lifetime of 2 months and the relatively high standard deviation of 0.25 months.

The mean lifetime of a particular type of light bulb is given as 2 months, and the standard deviation is given as 0.25 months. The mean lifetime represents the average time that the light bulbs will last, while the standard deviation represents how much the lifetimes of the bulbs vary from the mean.

To determine the probability that a box of 40 light bulbs will last for 5 years, we need to convert the given information into a format that we can work with. 5 years is equal to 60 months, and since we have 40 light bulbs, we can assume that the lifetimes of the bulbs are independent and identically distributed. This means that the probability of one bulb lasting for 60 months is the same as the probability of any other bulb lasting for 60 months.

Next, we need to calculate the standard deviation of the sample mean. The standard deviation of the sample mean represents how much the means of different samples of size 40 would vary from the population mean. The formula for the standard deviation of the sample mean is given by the following equation:

standard deviation of the sample mean = standard deviation of the population / square root of the sample size

In this case, the standard deviation of the population is given as 0.25 months, and the sample size is 40. Therefore, the standard deviation of the sample mean is:

0.25 / sqrt(40) = 0.0395

Now that we have the mean lifetime and the standard deviation of the sample mean, we can use the normal distribution to determine the probability that a box of 40 light bulbs will last for 5 years. We can assume that the lifetimes of the bulbs follow a normal distribution with a mean of 2 months and a standard deviation of 0.0395 months (which is the standard deviation of the sample mean).

To find the probability that a bulb will last for 60 months, we can use the following equation:

z = (x - μ) / σ

where z is the standard score, x is the value we want to find the probability for (60 months in this case), μ is the mean, and σ is the standard deviation.

Plugging in the values, we get:

z = (60 - 2) / 0.0395 = 1509.49

To find the probability corresponding to this standard score, we can use a standard normal distribution table or a calculator. The probability is extremely small (close to zero), which means that it is highly unlikely that all 40 light bulbs will last for 5 years.

To know more about probability here.

https://brainly.com/question/11234923

#SPJ4

Answer: D and B

Step-by-step explanation:

Formula for two terms = a + b

Therefore,

D and B have two terms aka one plus sign

D. a + c

B. m^4 + 6m

Hi! ❄

  - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -  

The sum of two terms is what we get after adding these two terms.

If you add two positive terms, you put a + sign in between these terms.

\(\sf{Example\!\!:a+b}\)

If you add two negative terms, you put a - sign in between these terms.

\(\sf{Example\!\!:a-b}\) (this is the same as \(\sf{a+-b}\))

Let's look at the provided choices to see which one works.

It doesn't, because \(\sf{xy\neq x+y}\). In this expression a number x was multiplied by a number y. So this one doesn't check.

One down, three to go.

It does, because \(\sf{m^4+6m\stackrel\checkmark{=}m^4+6m}\). A number m was multiplied by itself 4 times, and then the product of that samee number m and 6 was added to it.

So this one checks.

It doesn't. It is indeed a sum, but we need 2 terms, not 3

So this one doesn't work.

It does, because \(\sf{a+c\stackrel\checkmark{=}a+c}\). So Choice D. also works.

Hope that made sense !!

  - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -  - - - - - - - - - - - - - - - - - - - - - - - - -

\(\star\tiny\pmb{calligraphy}\star\)

Answer:

AAS, I believe.

Step-by-step explanation:

The triangles share the angle (where the meet), and the angles that are marked. Then, there is the sides that are marked as well. So the theorem is angle-angle-side.

The average period of the trials that were conducted with an amplitude of 10 degrees is 1.694 seconds.

What is amplitude ?

In mathematics, amplitude refers to the maximum displacement or distance from the equilibrium position of a periodic function, such as a sine or cosine wave. In other words, if a periodic function oscillates back and forth between two extreme values, the amplitude is the maximum distance from the average value or midpoint of the oscillation to one of the extreme values.

To calculate the average period of the trials that were conducted with an amplitude of 10 degrees, we simply add up all the periods and divide by the total number of trials.

10 degrees: 1.66 s, 1.700 s, 1.71 s, 1.68 s, 1.72 s

Average period = (1.66 + 1.700 + 1.71 + 1.68 + 1.72) / 5 = 1.694 seconds

Therefore, the average period of the trials that were conducted with an amplitude of 10 degrees is 1.694 seconds.

To learn more about periodic function from the given link :

https://brainly.com/question/29277391

#SPJ1

1/3 is not a fraction of 1/2. To find out what fraction of 1/2 is 1/3, you would need to divide 1/3 by 1/2. This would give you 3/6, or 1/2. So 1/3 is one half of 1/2.

A fraction is a way of representing a part of a whole. It consists of two numbers, a numerator and a denominator, separated by a line or a slash. The numerator represents the number of parts of the whole that are being considered, and the denominator represents the total number of parts that make up the whole. For example, in the fraction 1/2, the numerator is 1 and the denominator is 2, so it represents one part of a whole that is divided into two equal parts. Fractions can also be used to represent ratios and division.

Learn more about fraction in brainly.com/question/10354322

#SPJ1

Answer:

The answer is below

Step-by-step explanation:

Describe a rigid motion or composition of rigid motions that maps the rectangular bench at (0,10) and the adjacent flagpole onto the other short rectangular bench and flagpole

Solution:

The other short rectangular bench is at (0, -10), while the short flagpole at (2, 10)

Transformation is the movement of a point from its initial location to a new location. If an object is transformed then all its points are also transformed. Types of transformation are reflection, dilation, rotation and translation.

If a point X(x, y) is reflected across the x axis, the new point is (x, -y)

If a point X(x, y) is reflected across the y axis, the new point is (-x, y)

Therefore the rectangular bench at (0,10) is reflected across the x axis to give the other short rectangular bench at (0, -10) while the adjacent flagpole  at (-2,10) is reflected across the y axis to give the other flagpole at (2, 10)

The given two equations represent that 6x-15y=15  is equal to y=2/5x-1 And they have the same slope 2/5.

Equations are logical statements in mathematics that are denoted by an equals (=) sign and two algebraic expressions on either side of it. It is demonstrated that the expressions on the left and right are equal to one another.

All mathematical equations start with LHS = RHS (left hand side = right hand side). To find the value of an unknown variable, or unknown quantity, you can solve equations. If the statement does not contain a "equal to" symbol, it is not an equation. It will be considered as an expression.

We have been asked to find the value of x and y in 6x-15y=15 and y=2/5x-1

Let solve for y = 2/5x-1

in  6x - 15y = 15

⇒ 6x - 15(2/5x-1) = 15

⇒ 6x - 6x  + 15 = 15

⇒ 15 = 15

⇒ This mans they have slope

⇒ 6x - 15y = 15 in y-intercept form

⇒ 15y = 6x - 15

⇒ 15y = 6x - 15

⇒ y = (6x - 15)15

⇒ y = 2/5x - 1

Thus, 6x-15y=15  is equal to y=2/5x-1 And they have the same slope 2/5.

Learn more about equation

https://brainly.com/question/2972832

#SPJ9

Step-by-step explanation:

Four tosses of the coin result in 2^4 = 16 possible outcomes of which FOUR

will have ONE tails :

HHHT

HHTH
HTHH
THHH      so 4 out of 16  = 4/16 = 1/4    Not very unusual

Answer:

34 years

Step-by-step explanation:

f = s + 28          Eq. 1

f+8 = 3(s+8)      Eq. 2

f = father age

s = son age

replacing Eq. 1 on Eq. 2

(s+28) + 8 = 3(s+8)

s + 36 = 3*s + 3*8

s + 36 = 3s + 24

36 - 24 = 3s - s

12 = 2s

12/2 = s

s = 6

from the Eq. 1

f = 6 + 28

f = 34

Check

from the Eq. 2

34+8 = 3(6+8)

42 = 3*14

The standard deviation of a standard normal distribution (b) is always equal to one. The correct answer is (b) is always equal to one.

The standard deviation of a standard normal distribution is always equal to one. A standard normal distribution is a normal distribution with a mean of zero and a standard deviation of one. This distribution is commonly used in statistical analysis and is characterized by a bell-shaped curve. The curve is symmetric, with the highest point at the mean, and the spread of the distribution is determined by the standard deviation.

The standard deviation is a measure of the variability or spread of the data. In a normal distribution, about 68% of the data falls within one standard deviation of the mean, and about 95% falls within two standard deviations. Therefore, the standard deviation is an important parameter that helps describe the distribution of the data.

for such more question on standard deviation

https://brainly.com/question/475676

#SPJ11

The budgets of Hong Kong, the United States of America, and Korea differ in contents, formats, advantages, and disadvantages. While each budget has its strengths and weaknesses, they all aim to provide a clear and transparent financial plan for their respective countries.

A budget is a financial plan that estimates expected income and expenditure for a specific period. It may include income, expenses, debts, and savings. Budgets may vary from country to country and can be analyzed by comparing their contents, formats, advantages, and disadvantages. Here are the budgets of Hong Kong, the United States of America, and Korea:

United States Budget:

Korean Budget:

Learn more about Budget:

https://brainly.com/question/26894404

#SPJ11

A survey company must contact 136 people via phone calls.  The no.of buttons that must be pushed to make the 136 long-distance calls is

136 ✖ 10 = 1,360 buttons.

A survey is a study approach used for accumulating information from a predefined institution of respondents to benefit records and insights into diverse subjects of the hobby. They can have multiple functions, and researchers can conduct it in lots of approaches relying on the method chosen and have a look at's intention.

A survey can be used to investigate the characteristics, behaviors, or evaluations of a set of human beings. Those research gear may be used to ask questions about demographic information about traits which includes intercourse, faith, ethnicity, and profits.

In studies of human subjects, a survey is a list of questions aimed at extracting precise information from a selected institution of human beings. Surveys may be conducted with the aid of telephone, mail, thru the net, and additionally at road corners or in department stores.

Learn  more about the survey here https://brainly.com/question/12264186

#SPJ4

Answer:

Distance=12 miles

Step-by-step explanation:

d=distance

t=time going

d/t=40 mph going

t-2/60=time returning      (2 minutes/60 minutes per hour)

d/(t-2/60)=45 mph returning

set up equations:

d=40t

d=45(t-2/60)

substitute:

40t=45(t-2/60)

40t=45t-90/60

40t=45t-3/2

0=5t-3/2

5t=3/2

t=0.3 hours or 18 minutes

(time returning=16 minutes)

substitute:

d=40t=40(0.3)

d=12 miles

P and Q are 12 miles far from each other.

Given that

Patrick drives from P to Q at an average speed of 40 mph

Also he drives from Q to P at an average speed of 45  mph

Let "s" be the distance between P and Q in miles.

Let "t" be  the time in minutes

There are 60 minutes in 1 hours

\(\rm So \;in \; "t" \; minutes \; there\; will\; be = \dfrac{t}{60} \ hours\)

By the definition pf average speed we can write that

\(\rm Average \; Speed = \dfrac{Total \; distance }{Total \; time}\\\)

The speed is given in two situations in miles per hour

Case 1  When average speed = 40 mph

\(\rm 40 = \dfrac{s}{t/60} \\\\\rm {40 = \dfrac{60s}{t} .....(1) }\)

Case 2 When  average speed = 45 mph  

Given that Patrick takes two minutes less  

\(\rm 45 = \dfrac{s}{(t-2)/60} \\\\45 = \dfrac{60s}{t-2 } ....(2)\)

Solving for "s" from equations (1) and (2) gives us

\(\rm \bold {s = 12}}\)

So we can conclude that P and Q are 12 miles far from each other.

For more information please refer to the link given below

https://brainly.com/question/21470320

If 5 is subtracted from three times a number, the result is 16. Therefore the number is 07.

Number:

Numbers are mathematical objects used for counting, measuring and marking. The original examples are the natural numbers 1, 2, 3, 4, etc. Numbers can be represented by number words. More generally, individual numbers can be represented by symbols, called numerals; for example, "5" is the number representing the number five.

According to the Question:

Let the number be x.

As per question, we have

3x−5 = 16

⇒ 3x = 16+5

⇒ 3x = 21

⇒ x = 7

Therefore, the number is 7.

Complete Question:

If 5 is subtracted from three times a number, the result is 16. Find the number.

Learn more about Number:

https://brainly.com/question/17429689

#SPJ4

Answer:

Z=8C

If you times Cups C by 8, you get ounces Z. Sorry, not too sure how to explain here.

Answer:

x = 6

Step-by-step explanation:

Given equation,

→ 3x - (2/3)x = 14

Now the value of x will be,

→ 3x - (2/3)x = 14

→ (9/3)x - (2/3)x = 14

→ ((9 - 2)/3)x = 14

→ (7/3)x = 14

→ 7x = 14 × 3

→ x = 42/7

→ [ x = 6 ]

Hence, the value of x is 6.

Answer:

  A. 25 cm^3

Step-by-step explanation:

The volume of a pyramid is given by the formula ...

  V = (1/3)Bh

where B is the area of the base, and h is the vertical height from the base.

For the given pyramid, ...

  V = (1/3)(5 cm)^2(3 cm) = 25 cm^3

Answer:

Step-by-step explanation:

Answer:

Length of the shadow = 20.5 cm

Step-by-step explanation:

Given the 32-meter height of a tall building, and its casted shadow of 38 meters, we can find the unknown length of the shadow by using the Pythagorean Theorem.

The Pythagorean Theorem states that the squared length of the hypotenuse of a right triangle is equal to the sum of the squared lengths of the legs.

The algebraic representation of the Pythagorean Theorem is:  

                     c² (hypotenuse) = a² (leg₁) + b² (leg₂)

To solve for the given problem:

Let c = 38 m (distance from the top of the building to the tip of the shadow)

a = unknown length of the shadow

b = 32m (height of the building)

Since we have to solve for the value of a (unknown length of the shadow), we must algebraically solve for a:

c² = a² + b²

Subtract b² from both sides:

c² - b² = a²  + b² - b²

a² = c² - b²

Substitute the given values into the formula for solving a :

a² = c² - b²

a² = (38)² - (32)²

a² = 1444 - 1024

a² = 1444 - 1024

Next, take the square root of both sides to solve for a:

\(\displaystyle\mathsf{\sqrt{(a)^2}\:=\:\sqrt{420}}\)

a = 20.49 or 20.5 cm

Therefore, the length of the shadow is 20.5 cm.

Answer:

17) m = 35; n = 110

18) b = 90; c = 80; d = 100

Step-by-step explanation:

17)

2m = 70

m = 35

n + 70 + 70 + n = 360

2n + 140 = 360

2n = 220

n = 110

18)

b - 10 + b + 10 = 180

2b = 180

b = 90

b - 10 = c

90 - 10 = c

c = 80

b + 10 = d

90 + 10 = d

d = 100

Answer: The answer is 4

Step-by-step explanation: Firstly you want to convert the whole number into a fraction, the fraction will be 24/1. Then you multiply 1/6 by 24/1 or 1x24/6x1 which equals 26/6. This fractio can be simplified, by finding the greatest common factor. The GCF of 24 and 6 is 6.

24/6 = 4

6/6 = 1

So our full answer is 4/1 which simplifies to 4

Answer:

4

Step-by-step explanation:

take 24 divided by 6 you'll get

Step-by-step explanation:

\( \underline{ \underline{ \text{Given}}} : \)

\( \underline{ \underline { \text{To \: Find}}} : \)

\( \underline{ \underline{ \text{Solution}}} : \)

The new matrix obtained from a given matrix by interchanging it's rows and columns is called the transposition of matrix. It is denoted by \( \sf{ {A}^{T}} \). Again , Interchange it's rows and columns in order to find ' A '.

\( \tt{A = \begin{bmatrix} 2 & 4 \\ - 4 & 3 \\ \end{bmatrix}}\)

Now , LEFT HAND SIDE ( L.H.S )

\( \tt{ {A}^{2} - 5A+ 22I}\)

Here, I refers to identity matrix. A diagonal matrix in which all the elements of leading diagonal are 1 ( unit ) is called unit or identity matrix.

⟼ \(\begin{bmatrix} 2 & 4 \\ - 4 & 3 \\ \end{bmatrix} \times \begin{bmatrix} 2 & 4 \\ - 4 & 3 \\ \end{bmatrix} - 5 \times \begin{bmatrix} 2 & 4 \\ - 4 & 3 \\ \end{bmatrix} + 22 \times \begin{bmatrix} 1 & 0 \\ 0 & 1\\ \end{bmatrix}\)

⟼ \(\begin{bmatrix} 2 \times 2 + 4 \times ( - 4)& 2 \times 4 + 4 \times 3 \\ - 4 \times 2 + 3 \times ( - 4) & - 4 \times 4 + 3 \times 3 \\ \end{bmatrix} - \begin{bmatrix} 10 & 20 \\ - 20& 15 \\ \end{bmatrix} + \begin{bmatrix} 22 & 0 \\ 0 & 22 \\ \end{bmatrix}\)

⟼ \(\begin{bmatrix} 4 + ( - 16) & 8 + 12 \\ - 8 + ( - 12) & - 16 + 9 \\ \end{bmatrix} - \begin{bmatrix} 10 & 20 \\ - 20 & 15 \\ \end{bmatrix} + \begin{bmatrix} 22 & 0 \\ 0 & 22 \\ \end{bmatrix}\)

⟼ \( \begin{bmatrix} - 12 & 20\\ - 20& - 7 \\ \end{bmatrix} - \begin{bmatrix} 10 & 20 \\ - 20 & 15 \\ \end{bmatrix} + \begin{bmatrix} 22 & 0 \\ 0 & 22 \\ \end{bmatrix}\)

⟼ \(\begin{bmatrix} - 22 & 0 \\ 0& - 22 \\ \end{bmatrix} + \begin{bmatrix} 22 & 0 \\ 0 & 22 \\ \end{bmatrix}\)

⟼ \(\begin{bmatrix} - 22 + 22 & 0 + 0 \\ 0 + 0 & - 22 + 22 \\ \end{bmatrix}\)

⟼ \(\begin{bmatrix} 0 & 0\\ 0 & 0 \\ \end{bmatrix}\)

⟼ \( \sf{0}\)

RIGHT HAND SIDE ( R.H.S ) : 0

L.H.S = R.H.S [ Hence , proved ! ]

Hope I helped ! ♡

Have a wonderful day / night ! ツ

▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁

Answer:

Step-by-step explanation:

\underline{ \underline{ \text{Given}}} :

\begin{gathered} \tt{ {A}^{T} = \begin{bmatrix} 2 & - 4 \\ 4 & 3 \\ \end{bmatrix}}\end{gathered}

\underline{ \underline { \text{To \: Find}}} :

\underline{ \underline{ \text{Solution}}} :

The new matrix obtained from a given matrix by interchanging it's rows and columns is called the transposition of matrix. It is denoted by \sf{ {A}^{T}}

. Again , Interchange it's rows and columns in order to find ' A '.

\begin{gathered} \tt{A = \begin{bmatrix} 2 & 4 \\ - 4 & 3 \\ \end{bmatrix}}\end{gathered}

Now , LEFT HAND SIDE ( L.H.S )

\tt{ {A}^{2} - 5A+ 22I}

Here, I refers to identity matrix. A diagonal matrix in which all the elements of leading diagonal are 1 ( unit ) is called unit or identity matrix.

⟼ \begin{gathered}\begin{bmatrix} 2 & 4 \\ - 4 & 3 \\ \end{bmatrix} \times \begin{bmatrix} 2 & 4 \\ - 4 & 3 \\ \end{bmatrix} - 5 \times \begin{bmatrix} 2 & 4 \\ - 4 & 3 \\ \end{bmatrix} + 22 \times \begin{bmatrix} 1 & 0 \\ 0 & 1\\ \end{bmatrix}\end{gathered}

⟼ \begin{gathered}\begin{bmatrix} 2 \times 2 + 4 \times ( - 4)& 2 \times 4 + 4 \times 3 \\ - 4 \times 2 + 3 \times ( - 4) & - 4 \times 4 + 3 \times 3 \\ \end{bmatrix} - \begin{bmatrix} 10 & 20 \\ - 20& 15 \\ \end{bmatrix} + \begin{bmatrix} 22 & 0 \\ 0 & 22 \\ \end{bmatrix}\end{gathered}

⟼ \begin{gathered}\begin{bmatrix} 4 + ( - 16) & 8 + 12 \\ - 8 + ( - 12) & - 16 + 9 \\ \end{bmatrix} - \begin{bmatrix} 10 & 20 \\ - 20 & 15 \\ \end{bmatrix} + \begin{bmatrix} 22 & 0 \\ 0 & 22 \\ \end{bmatrix}\end{gathered}

⟼ \begin{gathered} \begin{bmatrix} - 12 & 20\\ - 20& - 7 \\ \end{bmatrix} - \begin{bmatrix} 10 & 20 \\ - 20 & 15 \\ \end{bmatrix} + \begin{bmatrix} 22 & 0 \\ 0 & 22 \\ \end{bmatrix}\end{gathered}

⟼ \begin{gathered}\begin{bmatrix} - 22 & 0 \\ 0& - 22 \\ \end{bmatrix} + \begin{bmatrix} 22 & 0 \\ 0 & 22 \\ \end{bmatrix}\end{gathered}

⟼ \begin{gathered}\begin{bmatrix} - 22 + 22 & 0 + 0 \\ 0 + 0 & - 22 + 22 \\ \end{bmatrix}\end{gathered}

⟼ \begin{gathered}\begin{bmatrix} 0 & 0\\ 0 & 0 \\ \end{bmatrix}\end{gathered}

⟼ \sf{0}0

RIGHT HAND SIDE ( R.H.S ) : 0

Answer:

n = 6

Rule: Times 3

Step-by-step explanation:

The given statement "a rational expression is undefined if the numerator is zero" is false because a rational expression is undefined if the denominator is zero, not the numerator.

When the denominator of a rational expression becomes zero, the expression becomes undefined because division by zero is not defined in mathematics.

If the numerator of a rational expression is zero, it does not make the expression undefined. Instead, it results in the value of the expression being zero, regardless of the value of the denominator.

To know more about rational expression:

https://brainly.com/question/30488168

#SPJ4

Answer:

Step-by-step explanation:

1. 500

2. 10

3. 25000