Answers
Answer:
Refer to the step-by-step Explanation.
Step-by-step Explanation:
Simplify the equation with given substitutions,
Given Equation:
\(mgh+(1/2)mv^2+(1/2)I \omega^2=(1/2)mv_{_{0}}^2+(1/2)I \omega_{_{0}}^2\)
Given Substitutions:
\(\omega=v/R\\\\ \omega_{_{0}}=v_{_{0}}/R\\\\\ I=(2/5)mR^2\)\(\hrulefill\)
Start by substituting in the appropriate values: \(mgh+(1/2)mv^2+(1/2)I \omega^2=(1/2)mv_{_{0}}^2+(1/2)I \omega_{_{0}}^2 \\\\\\\\\Longrightarrow mgh+(1/2)mv^2+(1/2)\bold{[(2/5)mR^2]} \bold{[v/R]}^2=(1/2)mv_{_{0}}^2+(1/2)\bold{[(2/5)mR^2]}\bold{[v_{_{0}}/R]}^2\)
Adjusting the equation so it easier to work with.\(\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{2} \Big[\dfrac{2}{5} mR^2\Big]\Big[\dfrac{v}{R} \Big]^2=\dfrac12mv_{_{0}}^2+\dfrac12\Big[\dfrac25mR^2\Big]\Big[\dfrac{v_{_{0}}}{R}\Big]^2\)
\(\hrulefill\)
Simplifying the left-hand side of the equation:
\(mgh+\dfrac{1}{2} mv^2+\dfrac{1}{2} \Big[\dfrac{2}{5} mR^2\Big]\Big[\dfrac{v}{R} \Big]^2\)
Simplifying the third term.
\(\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{2} \Big[\dfrac{2}{5} mR^2\Big]\Big[\dfrac{v}{R} \Big]^2\\\\\\\\\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{2}\cdot \dfrac{2}{5} \Big[mR^2\Big]\Big[\dfrac{v}{R} \Big]^2\\\\\\\\\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{5} \Big[mR^2\Big]\Big[\dfrac{v}{R} \Big]^2\)
\(\\ \boxed{\left\begin{array}{ccc}\text{\Underline{Power of a Fraction Rule:}}\\\\\Big(\dfrac{a}{b}\Big)^2=\dfrac{a^2}{b^2} \end{array}\right }\)
\(\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{5} \Big[mR^2\Big]\Big[\dfrac{v^2}{R^2} \Big]\\\\\\\\\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{5} \Big[mR^2 \cdot\dfrac{v^2}{R^2} \Big]\)
"R²'s" cancel, we are left with:
\(\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{5} \Big[mR^2\Big]\Big[\dfrac{v^2}{R^2} \Big]\\\\\\\\\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{5}mv^2\)
We have like terms, combine them.
\(\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{5} \Big[mR^2\Big]\Big[\dfrac{v^2}{R^2} \Big]\\\\\\\\\Longrightarrow mgh+\dfrac{7}{10} mv^2\)
Each term has an "m" in common, factor it out.
\(\Longrightarrow m(gh+\dfrac{7}{10}v^2)\)
Now we have the following equation:
\(\Longrightarrow m(gh+\dfrac{7}{10}v^2)=\dfrac12mv_{_{0}}^2+\dfrac12\Big[\dfrac25mR^2\Big]\Big[\dfrac{v_{_{0}}}{R}\Big]^2\)
\(\hrulefill\)
Simplifying the right-hand side of the equation:
\(\Longrightarrow \dfrac12mv_{_{0}}^2+\dfrac12\cdot\dfrac25\Big[mR^2\Big]\Big[\dfrac{v_{_{0}}}{R}\Big]^2\\\\\\\\\Longrightarrow \dfrac12mv_{_{0}}^2+\dfrac15\Big[mR^2\Big]\Big[\dfrac{v_{_{0}}}{R}\Big]^2\\\\\\\\\Longrightarrow \dfrac12mv_{_{0}}^2+\dfrac15\Big[mR^2\Big]\Big[\dfrac{v_{_{0}}^2}{R^2}\Big]\\\\\\\\\Longrightarrow \dfrac12mv_{_{0}}^2+\dfrac15\Big[mR^2\cdot\dfrac{v_{_{0}}^2}{R^2}\Big]\\\\\\\\\Longrightarrow \dfrac12mv_{_{0}}^2+\dfrac15mv_{_{0}}^2\Big\\\\\\\\\)
\(\Longrightarrow \dfrac{7}{10}mv_{_{0}}^2\)
Now we have the equation:
\(\Longrightarrow m(gh+\dfrac{7}{10}v^2)=\dfrac{7}{10}mv_{_{0}}^2\)
\(\hrulefill\)
Now solving the equation for the variable "v":
\(m(gh+\dfrac{7}{10}v^2)=\dfrac{7}{10}mv_{_{0}}^2\)
Dividing each side by "m," this will cancel the "m" variable on each side.
\(\Longrightarrow gh+\dfrac{7}{10}v^2=\dfrac{7}{10}v_{_{0}}^2\)
Subtract the term "gh" from either side of the equation.
\(\Longrightarrow \dfrac{7}{10}v^2=\dfrac{7}{10}v_{_{0}}^2-gh\)
Multiply each side of the equation by "10/7."
\(\Longrightarrow v^2=\dfrac{10}{7}\cdot\dfrac{7}{10}v_{_{0}}^2-\dfrac{10}{7}gh\\\\\\\\\Longrightarrow v^2=v_{_{0}}^2-\dfrac{10}{7}gh\)
Now squaring both sides.
\(\Longrightarrow \boxed{\boxed{v=\sqrt{v_{_{0}}^2-\dfrac{10}{7}gh}}}\)
Thus, the simplified equation above matches the simplified equation that was given.
Related Questions
Which of the following is the dimension of moment of inertia? (a) ML2 (b) MLT-1 (c) L2T 2 (d) L T-1
Answers
The dimension of the moment of inertia is \(ML^2\). Option A.
What is the moment of inertia?The moment of inertia represents the resistance of a body to rotational motion. It depends on the body's mass and the distribution of that mass around the axis of rotation.
The formula for the moment of inertia involves mass and distance and is expressed as:
I = mr^2
where
m is the mass of the bodyr is the distance from the axis of rotation.The dimension of mass is represented by M, and the dimension of distance is represented by L. Therefore, the dimension of moment of inertia is:
Moment of inertia = mass x distance^2 = M x L^2 = \(ML^2\).
More on moment of inertia can be found here: https://brainly.com/question/15246709
#SPJ1
When the Apollo 11 lunar module Eagle lands on the moon it comes to a stop 10m above the surface of the moon. The last 10m it freely falls to the surface of the Moon.
i) How long does it take for the Eagle to touch down?
ii) What is the velocity of the lunar module when it hits the surface of the moon?
Answers
Answer:
i) 3.514 s, ii) 5.692 m/s
Explanation:
i) We can use Newton's second law of motion to find out how long does it take for the Eagle to touch down.
as the equation says for free-falling
h = ut +0.5gt^2
Here, h = 10 m, g = acceleration due to gravity = 1.62 m/s^2( on moon surface)
initial velocity u = 0
10 = 0.5×1.62t^2
t = 3.514 seconds
Therefore, it takes t = 3.514 seconds for the Eagle to touch down.
ii) use Newton's 1st equation of motion to calculate the velocity of the lunar module when it hits the surface of the moon
v = u + gt
v = 0+ 1.62×3.514
v= 5.692 m/s
Hey, I need help can someone help me out, please?

Answers
Explanation:
6) newton
7) f =ma = 15*15 = 225N
8) a= 100/20 = 5ms^-2
Answer:
6 newton
7) f =ma = 15*15 = 225N
8) a= 100/20 = 5ms^-2
Explanation:
this is right pls mark as brainliest
PLEASE HELP
S
ai
5
The regular polygon has the following measures.
a = 9√3 m
s = 18 m
Segment a is drawn from the center of the polygon
perpendicular to one of its sides.
What is the vocabulary term for segment a?
What is the area of the polygon?
Round to the nearest tenth and include correct units.

Answers
Segment a, which is drawn from the center of the polygon perpendicular to one of its sides, is called the apothem.
The typical hexagon has a surface area of around 874.6 square meters.
How to determine area of a hexagon?To find the area of a regular polygon, use the formula:
Area = (1/2) x Perimeter x Apothem
Find the perimeter of the polygon. Since the polygon has n sides, use the formula:
Perimeter = n x s
where s = length of one side.
Since s = 18m, find n by using the formula:
n = 360° / (180° - (360° / n))
where n = number of sides.
Plugging in the values:
n = 360° / (180° - (360° / n))
n = 360° / (180° - (360° / 6))
n = 6
So the polygon has 6 sides, which makes it a hexagon.
Now find the perimeter:
Perimeter = n x s
Perimeter = 6 x 18
Perimeter = 108m
Next, find the apothem, use the formula:
Apothem = s / (2 x tan(π/n))
Plugging in the values:
Apothem = 18 / (2 x tan(π/6))
Apothem = 9√3 m
Now use the formula for the area:
Area = (1/2) x Perimeter x Apothem
Area = (1/2) x 108 x 9√3
Area ≈ 874.6 m²
Therefore, the area of the regular hexagon is approximately 874.6 square meters.
Find out more on hexagon here: https://brainly.com/question/28821314
#SPJ1
The following graph of velocity versus time represents that of an automobile that travels in a straight line. From this graph, one can conclude that:

Answers
The true statement is that the acceleration in the first two seconds is 6 m/s^2.
What is acceleration?The term acceleration is defined as the rate of change of velocity with time. The graph as shown is a velocity time graph. The graph shows the changes that occur in the velocity over a given time interval.
Now we have;
Initial velocity = 0 m/s
Final velocity = 12 m/s
Time taken = 2s
Acceleration = 12 - 0/2
= 6 m/s^2
Thus the true statement is that the acceleration in the first two seconds is 6 m/s^2.
Learn more about acceleration:https://brainly.com/question/12550364
#SPJ1
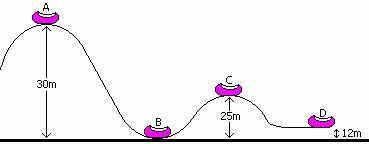
Which letters show moving from KINETIC to POTENTIAL.
Answers
Answer:
B to C
Explanation:
The object height decreases
Potential energy= mass x height x gravitational force
Which means that potential energy is directly proportional to height
Thus, a decrease in height will cause a decrease in potential energy.
ENERGY cannot be wasted, it is transformed to another form, and here this form is kinetic energy as according to the gravitational force the speed increases as the object falls downward.
And kinetic energy = 1/2 mv2
Which again means that speed is directly proportional to kinetic energy
And thus an increase in speed will cause an increase in kinetic energy.
Help ASAP please eheesj

Answers
2. Drought
3. Thunderstorm
4. Hurricane
5. Tsunami
6. Volcano
7. Earthquake
8. Landslides
A wave has an amplitude of 0.0800 m
and is moving 7.33 m/s. One oscillator
in the wave takes 0.230 s to go from
one crest to the next crest. Find the
wavelength of the wave.
(Unit = m)
Answers
If a wave has an amplitude of 0.0800 m and is moving 7.33 m/s. The
wavelength of the wave is 1.69m.
What is the wavelength?The wavelength of a wave can be determined using the equation:
Wavelength = velocity / frequency
To determine the frequency we need to calculate the reciprocal of the time it takes for one complete oscillation.
frequency = 1 / time
frequency = 1 / 0.230
frequency ≈ 4.35 Hz
Substitute the values into the wavelength equation:
wavelength = velocity / frequency
wavelength = 7.33 / 4.35
wavelength ≈ 1.69m
Therefore the wavelength of the wave is approximately 1.69 meters.
Learn more about wavelength here:https://brainly.com/question/10728818
#SPJ1
graph
shows a variety of moving objects and how their distance is related to time what do these objects have in common
Answers
What is common among all the graphs is that they all show an object that is moving.
What is a graph?In the distance time graph, we have the distance on the vertical axis and we have the time on the horizontal axis and the shape of the plots may differ depending on the nature of the motion of the objects.
Graphs of distance vs time help us to examine motion by showing how an object has moved over time. All objects shown on a distance vs. time graph are shifting positions over time, regardless of the graph's specific shape or slope, and the graph reveals information about the direction and speed of their motion.
Learn more about graphs:https://brainly.com/question/848468
#SPJ1
This is a whole quiz please answer ill make as many points as i can. Btw this is pickleball
Question 1 10 pts
The ___________________ rule says the ball must bounce one on each side before either team may start volleying the ball in the air.
Group of answer choices
Double Bounce
Volley Bounce
Bounce
Two Bounce
Flag this Question
Question 2 10 pts
The player standing in the _____________ service court is always the firsts server.
Group of answer choices
right
left
No answer text provided.
No answer text provided.
Flag this Question
Question 3 10 pts
Each game is played to ___________ points, won by _________.
Group of answer choices
11, 2
21,1
15,1
12,2
Flag this Question
Question 4 10 pts
According to one of the inventors, the game was officially named after a family dog, Pickles, who would chase the ball and run off with it.
Group of answer choices
True
False
Flag this Question
Question 5 10 pts
Pickleball is played on a court the size of a Badminton court with what looks like an oversized ping pong paddle and a __________ball.
Group of answer choices
whiffle
tennis
paddle
boccee
Flag this Question
Question 6 10 pts
In Pickleball, there are two non-volley lines, which create an area called the _______ .
Group of answer choices
Kitchen
Ally
Drop Zone
Dead Area
Flag this Question
Question 7 10 pts
Only the receiving team can score a point by winning a rally.
Group of answer choices
True
False
Flag this Question
Question 8 10 pts
Pickleball combines elements of Badminton, Tennis and ____________.
Group of answer choices
Ping Pong
volleyball
Bocce
Four Square
Flag this Question
Question 9 10 pts
Players may not hit the ball while standing in the kitchen unless the ball has already bounced on their side.
Group of answer choices
True
False
Flag this Question
Question 10 10 pts
Before serving, the server must call the score.
Group of answer choices
True
False
Answers
Answer:
1- bounce rule
2- left
3-11,2
4-true
5- whiffle ball
6- kitchen
7-false
8- ping pong
9- true
10- true
Explanation:
I'm pre5sure they are all right. brainliest please
Answer: I had the same quiz too I’m only doing so the other guy can get brainliest
Explanation:Kfkfkfjgjggj
A bike accelerates from 0 m/s to 15 m/s over the span of 5 seconds. How fast is the bike
traveling after 2.5 seconds?
Answers
The bike is travelling at 22.5 m/s after 2.5 s
What is acceleration?This is defined as the rate of change of velocity which time. It is expressed as
a = (v – u) / t
Where
a is the acceleration v is the final velocity u is the initial velocity t is the time How to determine the acceleration Initial velocity (u) = 0 m/sFinal velocity (v) = 15 m/sTime (t) = 5 sAcceleration (a) =?a = (v – u) / t
a = (15 – 0) / 5
a = 3 m/s²
How to determine the final velocity in the first 2.5 s Initial velocity (u) = 15 m/sAcceleration (a) = 3 m/s²Time (t) = 2.5 s Final velocity (v) = ?a = (v – u) / t
3 = (v – 15) / 2.5
Cross multiply
v – 15 = 3 × 2.5
v – 15 = 7.5
Collect like terms
v = 7.5 + 15
v = 22.5 m/s
Learn more about acceleration:
https://brainly.com/question/491732
#SPJ1
Q28. 200cm³ of water (filled to the brim of the beaker) is heated from 21°C to 83°C as shown. If the coefficient of linear expan- sion of Pyrex glass (beaker) is a-3.3x10 K and volume expansion of water as ß = 0.21 x 10³K¹, calculate the excess amount of water (in cm³) that moved up the small tube attached to the lid as shown;
Answers
The excess amount of water that moved up the small tube attached to the lid is 2.60 cm³ in linear expan- sion of Pyrex glass.
What occurs to the water's volume as the beaker heats up?Water expands or gains capacity when it is heated. Water loses density as its volume rises. Water shrinks and loses volume as it cools. Water becomes denser as its volume diminishes.
We can apply the formula:
ΔV = V₀(β₁ΔT - αΔT)
where:
ΔV = excess amount of water that moved up the tube
V₀ = initial volume of water = 200 cm³
β₁ = volume expansion coefficient of water = 0.21 x 10³ K⁻¹
α = linear expansion coefficient of Pyrex glass = 3.3 x 10⁻⁶ K⁻¹
ΔT = change in temperature = 83°C - 21°C = 62°C
Plugging in the values, we get:
ΔV = 200(0.21 x 10³)(62) - 200(3.3 x 10⁻⁶)(62)
= 2.602 cm³ (rounded to three significant figures)
To know more about Pyrex glass visit:-
https://brainly.com/question/14085526
#SPJ1
These waves are traveling at the same speed. Which wave has the highest frequency? A. Wave frequency With line crossing in the middle B. A wave frequency with line crossing in the middle C. A wave frequency with line crossing in the middle D. A wave frequency with line crossing it Reset Next
Answers
These waves are traveling at the same speed. The wave with the highest frequency is option C, "A wave frequency with line crossing in the middle."
Frequency is a measure of the number of complete cycles or oscillations of a wave that occur in one second. It is typically measured in hertz (Hz). The higher the frequency, the more cycles or oscillations occur per unit of time.In the given question, it is stated that all the waves are traveling at the same speed. This means that the speed of propagation is constant for all the waves. However, the frequency of a wave is independent of its speed.By looking at the options, we notice that all the waves have the same wave pattern with a line crossing in the middle. The difference lies in the spacing between the waves, which corresponds to the frequency.The wave with the highest frequency will have the shortest wavelength and the most closely spaced wave crests. Since option C has the shortest spacing between the wave crests, it indicates a higher frequency compared to the other options.Therefore, based on the given information, option C, "A wave frequency with line crossing in the middle," has the highest frequency among the given choices.Please note that the question does not provide specific frequency values or any other information to determine the exact frequencies of the waves. We can only compare the relative frequencies based on the given visual representation.For more such questions on waves, click on:
https://brainly.com/question/26116832
#SPJ8
Hello! Is it possible to get help on this question, I do not understand why it was marked wrong

Answers
To find
Which of the following has units of velocity
Explanation
The unit of velocity is m/s
Conclusion
So the correct option are
-120 m/s
9.8m/s downward
URGENTLY NEED HELP
This is a 2 part question
42) You pull downward with a force of 28 N on a rope that passes over a disk-shaped pulley of mass 1.2 kg and radius 0.075 m. The other end of the rope is attached to a 0.67-kg mass. (a) Is the tension in the rope the same on both sides of the pulley? If not, which side has the greater tension? (b) Find the tension in the rope on both sides of the pulley.
Answers
Answer:
The net force on the hanging mass is thus
2 T Mg 18 6.6 N 11.4 N
, enough to accelerate it upward at
17 m/s2
. The angular acceleration of the pulley is thus
2 2
Explanation:
The tension in the rope on both sides of the pulley 20.21N.
To solve this problem, we'll need to consider the forces acting on both sides of the pulley and apply Newton's second law of motion. Let's break it down step by step:
(a) Is the tension in the rope the same on both sides of the pulley?
No, the tension in the rope is not the same on both sides of the pulley. The side with the greater mass attached will experience a greater tension in the rope.
(b) Find the tension in the rope on both sides of the pulley:
Calculate the gravitational force on each mass:
Gravitational force on the pulley: Fpulley = mpulley * g, where mpulley is the mass of the pulley and g is the acceleration due to gravity (approximately 9.8 m/s²).
Gravitational force on the hanging mass: Fhanging = mhanging * g, where m_hanging is the mass of the hanging mass.
Calculate the net force on the pulley:
Net force on the pulley is the difference between the tension forces on either side: Fnet = TLeft - Tright.
Apply Newton's second law to the pulley:
For the pulley, Fnet = mpulley * a, where a is the acceleration of the pulley. Since the pulley is assumed to be massless, we can use the relationship a = α * r, where α is the angular acceleration and r is the radius of the pulley.
Use the relationship between linear acceleration and angular acceleration:
α = a / r.
Equate the torque due to the tension to the moment of inertia times the angular acceleration:
τ = I * α, where τ is the torque, I is the moment of inertia of the pulley, and α is the angular acceleration.
Substitute the expression for α and solve for the net tension:
Tnet = (τ / r) = (I * α) / r = (1/2 * mpulley * r² * α) / r = (1/2 * mpulley * r * a).
Now, substitute the expression for a from step 3 and solve for the net tension:
Tnet = (1/2 * mpulley * r * α) = (1/2 * mpulley * r * (a / r)) = (1/2 * mpulley * a).
Substitute the expression for a from step 2 (Fnet = mpulley * a) and solve for the net tension:
Tnet = (1/2 * Fnet).
Now, you can find the tensions on each side of the pulley:
Tleft = Tnet + Fhanging
Tright = Tnet - Fpulle
To know more about tension
https://brainly.com/question/16943376
#SPJ2
With fuel prices for combustible engine automobiles increasing, researchers
and manufacturers have given more attention to the concept of an ultralight
car. Using carbon composites, lighter steels and plastics, a fuel-efficient car
can be manufactured at 540 kg. How much less does an ultralight car weigh
compared to a 1450-kg Honda Accord (2007)?
Answers
Answer:
An ultralight car weighs less than Honda at 940kg or about 63% less
5. What is the elevation along the shoreline (sea level)?
O ft
1 ft
10 ft
100 ft
Answers
Because it lies directly on the 10 contour it has and elevation of 10ft.
You are walking on a moving walkway in the airport. The length of the walkway is 59.1 m. If your velocity relative to the walkway is 2.35 m/s, and the walkway moves with a velocity of 1.85 m/s, how long will it take you to reach the other end of the walkway
Answers
Answer:
14.1seconds approx
Explanation:
Given data
Distance= 59.1m
Your velocity= 2.35m/s
Walkway velocity= 1.85m/s
Total velocity= 2.35+1.85= 4.2m/s
We know that
Velocity= distance/time
time= distance/velocity
substitute
time= 59.1/4.2
time= 14.07
time=14.1seconds approx
Hence the time is 14.1seconds approx
The force acting on a body of mass 200 g is equal to 0. Determine the velocity of the body 3 seconds after the start of the observation, if at the initial moment of the observation its velocity is 5 m / s?
Answers
Answer:
5 m/sExplanation:
Formula (Force)
F = maBut, a = v - u / t [First Equation of Motion]F = m (v - u / t)We have the values of :
F = 0m = 200 g = 0.2 kgt = 3 su = 5 m/sSubstitution
0 = 0.2 (v - 5 / 3)v - 5 / 3 = 0v - 5 = 0v = 5 m/sA truck weighing 5 * 10^3 kgf and a cart weighting 500 kgf are moving with the same speed compare their kinetic energies?
Answers
A truck weighing 5 x 10³ kg force.
A cart weighing 500 kg force.
Moving with the same speed complete their
kinetic energies.
As we know that,
Formula of:
→ Kinetic energy = 1/2 x m x v².
Using this formula in the equation, we get.
(1).
→ For truck = 1/2 x 5 x 10³ x v².-----
→ For cart = 1/2 x 500 x v². -----(2).
Divide equation (1) and (2), we get.
⇒ KE₁/KE₂ = 5 x 10³/5 x 10².
➡ KE₁/KE₂ = 10/1.
⇒ KE₁/KE₂ = 10:1.
When several forces act on an object, the forces combine to act as a
Answers
Answer:
Net force
Explanation:
When several forces act on an object, the forces combine to act as a net force.
A 0.0400 kg meter stick is placed on a thin rod at the 30.0 cm mark. What is the minimum mass required to be placed on the 0.00 cm mark on the stick to maintain equilibrium?
Answer in kg
Answers
The minimum mass required to be placed on the 0.00 cm mark of the meter stick to maintain equilibrium is 0.120 kg.
To maintain equilibrium, the torques acting on the meter stick must balance each other. The torque is given by the formula:
τ = r * F * sin(θ)
where τ is the torque, r is the distance from the pivot point to the point where the force is applied, F is the force applied, and θ is the angle between the force vector and the lever arm.
In this case, the meter stick is in equilibrium when the torques on both sides of the pivot point cancel each other out. The torque due to the weight of the meter stick itself is acting at the center of mass of the meter stick, which is at the 50.0 cm mark.
Let's denote the mass to be placed on the 0.00 cm mark as M. The torque due to the weight of M can be calculated as:
τ_M = r_M * F_M * sin(θ)
where r_M is the distance from the pivot point to the 0.00 cm mark (which is 30.0 cm), F_M is the weight of M, and θ is the angle between the weight vector and the lever arm.
Since the system is in equilibrium, the torques on both sides of the pivot point must be equal:
τ_M = τ_stick
r_M * F_M * sin(θ) = r_stick * F_stick * sin(θ)
Substituting the given values:
30.0 cm * F_M = 20.0 cm * (0.0400 kg * 9.8 m/s^2)
Solving for F_M:
F_M = (20.0 cm / 30.0 cm) * (0.0400 kg * 9.8 m/s^2)
F_M = 0.0264 kg * 9.8 m/s^2
F_M = 0.25872 N
Finally, we can convert the force into mass using the formula:
F = m * g
0.25872 N = M * 9.8 m/s^2
M = 0.0264 kg
Therefore, the minimum mass required to be placed on the 0.00 cm mark of the meter stick to maintain equilibrium is 0.120 kg.
For more such questions on equilibrium, click on:
https://brainly.com/question/517289
#SPJ8
which team won the champions league in 2020 2021
Answers
Answer:
Chelsea F.C
Explanation:
Chelsea F.C
Soccer
Can someone explain how to do the algebra for this question? I know everything else, I just don’t know how to rearrange the question to solve for v.

Answers
Answer:
Refer to the step-by-step Explanation.
Step-by-step Explanation:
Simplify the equation with given substitutions,
Given Equation:
\(mgh+(1/2)mv^2+(1/2)I \omega^2=(1/2)mv_{_{0}}^2+(1/2)I \omega_{_{0}}^2\)
Given Substitutions:
\(\omega=v/R\\\\ \omega_{_{0}}=v_{_{0}}/R\\\\\ I=(2/5)mR^2\)\(\hrulefill\)
Start by substituting in the appropriate values: \(mgh+(1/2)mv^2+(1/2)I \omega^2=(1/2)mv_{_{0}}^2+(1/2)I \omega_{_{0}}^2 \\\\\\\\\Longrightarrow mgh+(1/2)mv^2+(1/2)\bold{[(2/5)mR^2]} \bold{[v/R]}^2=(1/2)mv_{_{0}}^2+(1/2)\bold{[(2/5)mR^2]}\bold{[v_{_{0}}/R]}^2\)
Adjusting the equation so it easier to work with.\(\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{2} \Big[\dfrac{2}{5} mR^2\Big]\Big[\dfrac{v}{R} \Big]^2=\dfrac12mv_{_{0}}^2+\dfrac12\Big[\dfrac25mR^2\Big]\Big[\dfrac{v_{_{0}}}{R}\Big]^2\)
\(\hrulefill\)
Simplifying the left-hand side of the equation:
\(mgh+\dfrac{1}{2} mv^2+\dfrac{1}{2} \Big[\dfrac{2}{5} mR^2\Big]\Big[\dfrac{v}{R} \Big]^2\)
Simplifying the third term.
\(\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{2} \Big[\dfrac{2}{5} mR^2\Big]\Big[\dfrac{v}{R} \Big]^2\\\\\\\\\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{2}\cdot \dfrac{2}{5} \Big[mR^2\Big]\Big[\dfrac{v}{R} \Big]^2\\\\\\\\\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{5} \Big[mR^2\Big]\Big[\dfrac{v}{R} \Big]^2\)
\(\\ \boxed{\left\begin{array}{ccc}\text{\Underline{Power of a Fraction Rule:}}\\\\\Big(\dfrac{a}{b}\Big)^2=\dfrac{a^2}{b^2} \end{array}\right }\)
\(\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{5} \Big[mR^2\Big]\Big[\dfrac{v^2}{R^2} \Big]\\\\\\\\\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{5} \Big[mR^2 \cdot\dfrac{v^2}{R^2} \Big]\)
"R²'s" cancel, we are left with:
\(\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{5} \Big[mR^2\Big]\Big[\dfrac{v^2}{R^2} \Big]\\\\\\\\\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{5}mv^2\)
We have like terms, combine them.
\(\Longrightarrow mgh+\dfrac{1}{2} mv^2+\dfrac{1}{5} \Big[mR^2\Big]\Big[\dfrac{v^2}{R^2} \Big]\\\\\\\\\Longrightarrow mgh+\dfrac{7}{10} mv^2\)
Each term has an "m" in common, factor it out.
\(\Longrightarrow m(gh+\dfrac{7}{10}v^2)\)
Now we have the following equation:
\(\Longrightarrow m(gh+\dfrac{7}{10}v^2)=\dfrac12mv_{_{0}}^2+\dfrac12\Big[\dfrac25mR^2\Big]\Big[\dfrac{v_{_{0}}}{R}\Big]^2\)
\(\hrulefill\)
Simplifying the right-hand side of the equation:
\(\Longrightarrow \dfrac12mv_{_{0}}^2+\dfrac12\cdot\dfrac25\Big[mR^2\Big]\Big[\dfrac{v_{_{0}}}{R}\Big]^2\\\\\\\\\Longrightarrow \dfrac12mv_{_{0}}^2+\dfrac15\Big[mR^2\Big]\Big[\dfrac{v_{_{0}}}{R}\Big]^2\\\\\\\\\Longrightarrow \dfrac12mv_{_{0}}^2+\dfrac15\Big[mR^2\Big]\Big[\dfrac{v_{_{0}}^2}{R^2}\Big]\\\\\\\\\Longrightarrow \dfrac12mv_{_{0}}^2+\dfrac15\Big[mR^2\cdot\dfrac{v_{_{0}}^2}{R^2}\Big]\\\\\\\\\Longrightarrow \dfrac12mv_{_{0}}^2+\dfrac15mv_{_{0}}^2\Big\\\\\\\\\)
\(\Longrightarrow \dfrac{7}{10}mv_{_{0}}^2\)
Now we have the equation:
\(\Longrightarrow m(gh+\dfrac{7}{10}v^2)=\dfrac{7}{10}mv_{_{0}}^2\)
\(\hrulefill\)
Now solving the equation for the variable "v":
\(m(gh+\dfrac{7}{10}v^2)=\dfrac{7}{10}mv_{_{0}}^2\)
Dividing each side by "m," this will cancel the "m" variable on each side.
\(\Longrightarrow gh+\dfrac{7}{10}v^2=\dfrac{7}{10}v_{_{0}}^2\)
Subtract the term "gh" from either side of the equation.
\(\Longrightarrow \dfrac{7}{10}v^2=\dfrac{7}{10}v_{_{0}}^2-gh\)
Multiply each side of the equation by "10/7."
\(\Longrightarrow v^2=\dfrac{10}{7}\cdot\dfrac{7}{10}v_{_{0}}^2-\dfrac{10}{7}gh\\\\\\\\\Longrightarrow v^2=v_{_{0}}^2-\dfrac{10}{7}gh\)
Now squaring both sides.
\(\Longrightarrow \boxed{\boxed{v=\sqrt{v_{_{0}}^2-\dfrac{10}{7}gh}}}\)
Thus, the simplified equation above matches the simplified equation that was given.
A geodesic dome constructed with an aluminium framework is a nearly perfect hemisphere; its diameter measures 58.0 m on a winter day at a temperature of -15 ∘C
How much more interior space does the dome have in the summer, when the temperature is 34 ∘C?
Answers
The dome will have approximately 0.1176% more interior space in the summer than it does in the winter.
The dome will have approximately 88.6 m³ more interior space in the summer than it does in the winter.
The dome will have more interior space in the summer due to thermal expansion of the aluminum framework. To calculate the change in volume, we can use the coefficient of thermal expansion for aluminum, which is approximately 2.4 x 10⁻⁵ /°C.
The temperature change from -15°C to 34°C is a difference of 49°C. So, the fractional change in volume is:
ΔV/V = αΔT
ΔV/V = (2.4 x 10⁻⁵/°C) x 49°C
ΔV/V = 0.001176
As a result, the dome will have 0.1176% more internal area in the summer than it does in the winter.
To find the actual change in volume, we can use the formula for the volume of a hemisphere:
V = (2/3)πr³
The diameter of the dome is 58.0 m, so the radius is 29.0 m. Therefore, the volume of the dome is:
V = (2/3)π(29.0 m)³
V = 75344 m³
Increasing the volume by 0.1176% gives us:
ΔV = 0.001176 x 75344 m³
ΔV = 88.6 m³
As a result, the dome will have 88.6 m³ more internal room in the summer than it does in the winter.
To know more about the Geodesic dome, here
https://brainly.com/question/13671923
#SPJ1
5. Hilda is trying to move a 40 kg couch across a level floor and pushes with a horizontal force of
150 N, but the couch does not move. What is the minimum coefficient of static friction with the
floor? Assume the acceleration due to gravity is g = 9.8 m/s2
Answers
The minimum coefficient of static friction with the floor is 0.3846.
To find the minimum coefficient of static friction with the floor, we need to consider the forces acting on the couch. In this case, the force of gravity is pulling the couch downward with a magnitude of mg, where m is the mass of the couch (40 kg) and g is the acceleration due to gravity (9.8 m/s²).
Since the couch does not move, the force of static friction between the couch and the floor must be equal in magnitude but opposite in direction to the horizontal pushing force of 150 N.
Therefore, we have the equation F_friction = F_push, where F_friction is the force of static friction.
The force of static friction can be calculated using the formula F_friction = μ_s * N, where μ_s is the coefficient of static friction and N is the normal force.
Since the couch is on a level floor and is not accelerating vertically, the normal force N is equal in magnitude but opposite in direction to the force of gravity, which is mg.
Substituting the values into the equation, we have μs * mg = 150 N.
Solving for μs, we get μs = 150 N / (mg).
Substituting the given values, we have μ_s = 150 N / (40 kg * 9.8 m/s²).
Simplifying, we find that μs = 0.3846.
For more such questions on friction visit:
https://brainly.com/question/24386803
#SPJ8
Which two things occur when electric charges move through a conductor?
A. The field is perpendicular to the electron flow.
B. The field is parallel to the electron flow.
C. An electric field forms.
D. A magnetic field forms.
The two correct answers are A and D ap3x approved!
Answers
A C and D
C because ofc when electric charge flow electric field is produced
Answer:
A & D
Explanation:
Which is NOT an example of a wave phenomena at a boundary?
Answers
Answer:
Sound waves travel through the air.
Step-by-step explanation:
As a sound wave travels through a medium, it will often reach the end of the medium to encounter an obstacle or perhaps another medium through which it could travel. In a sound wave, a portion of the energy carried by the sound wave will pass across the boundary and out of the transmission, and a portion of the energy carried by the sound wave will reflect off the boundary, remain in the transmission, and travel in the opposite direction.
(a) Calculate the force (in N) the woman in the figure below exerts to do a push-up at constant speed, taking all data to be known to three digits. (You may need to use torque methods from a later chapter.) 401.15
(b)How much work (in J) does she do if her center of mass rises 0.260 m?
(c) What is her useful power output (in W) if she does 30 push-ups in 1 min? (Should work done lowering her body be included? See the discussion of useful work in Work, Energy, and Power in Humans.)

Answers
The force is 400.2 N
The work done is 120 J
The power is 48W
What is Force?Force is a physical concept that describes the influence that one object has on another object, causing it to accelerate or deform. Force can be defined as any influence that changes the motion of an object, such as a push or a pull.
How to solve:
under equilibrium condition
F * 1.45 m =68 kg * 9.81 m/s^2 *0.87 m
F =400.2 N
b)
work done = m*g*h =68 kg*9.81 m/s^2*0.180 m =120.0744 J =120 J
c)
power =120.0744 J *(24 /60 s) =48.02976 W = 48 W
Read more about force here:
https://brainly.com/question/12970081
#SPJ1
state the particles of matter
Answers
Answer:
Matter can exist in one of three main states: solid, liquid, or gas. Solid matter is composed of tightly packed particles. A solid will retain its shape; the particles are not free to move around. Liquid matter is made of more loosely packed particles.