Can someone please help me with this?
The x-values need to go on the horizontal axis and the y-values need to go on the vertical axis (just switch them around).
How do I do that? Could someone please show me how?

Answers
To switch the x and y values and plot the graph with the x-values on the horizontal axis and y-values on the vertical axis, following steps are to be followed.
What is graph?A graph is a visual representation of a set of data or mathematical relationship between variables.
Graphs are used to illustrate patterns, trends, and relationships in the data or functions, making it easier to interpret and communicate the information.
Write down the coordinates of the points given in the format (y, x) instead of (x, y).
(0, 30), (1, 60), (2, 100), (3, 130), (4, 160), (5, 190)
Draw the horizontal x-axis and vertical y-axis on a graph paper. Label the axes as "x-axis" and "y-axis", respectively.
Plot the points (0, 30), (1, 60), (2, 100), (3, 130), (4, 160), and (5, 190) on the graph paper.
To plot each point, locate the x-coordinate on the x-axis and the y-coordinate on the y-axis, and mark a point where they intersect.
Connect the points with a smooth curve to complete the graph.
The resulting graph will have the x-values on the horizontal axis and the y-values on the vertical axis.
To know more about axis visit:
https://brainly.com/question/1851512
#SPJ1
Related Questions
Three side lengths of 6,8 and 15 units will form a triangle
Answers
Answer:
The area would be 360 because 8 x 6 x 15 = 720 divided by 2 = 360
A man gives 1/4 property to his son, 1/3 of the remainder to his daughter and the rest part to hos wife, what portion did his wife get?
Answers
Answer:
5/12 goes to wife I don't really know how to explain it
A partir de un numero k incluso menor de 50
Answers
The required sum of all the even k numbers which are less than 50 is given by 600.
the sum of even k numbers less than 50,
Set of even numbers less than 50.
Even numbers are those that can be divided by 2 without a remainder.
Set of even numbers less than 50 are,
{2, 4, 6, 8, 10, 12, 14, 16, 18, 20, 22, 24, 26, 28, 30, 32, 34, 36, 38, 40, 42, 44, 46, 48}
Sum of the first k even numbers in this set.
k = n/2,
where n is the total number of even numbers in the set
here, n = 24
Use the formula for the sum of the first n natural numbers,
sum = (n /2 ) ( First term + last term )
Plugging in n = 24, we get,
⇒ sum = ( 24 / 2 ) ( 2 + 48 )
⇒ sum = 12 × (50)
⇒ sum = 600
Therefore, the sum of even k numbers less than 50 is equal to 600.
learn more about even here
brainly.com/question/5186943
#SPJ4
The given question is incomplete, I answer the question in general according to my knowledge:
Find the sum of even k numbers less than 50?
Joelle drew a function on a graph by drawing a vertical line. The line she drew passed through _______ point(s).
Answers
When Joelle drew a function on a graph by drawing a vertical line , the line she drew would pass through an infinite number of points.
A vertical line extends vertically without any horizontal displacement. As a result, it intersects the graph at every point with the same x-coordinate. Since the x-coordinate remains constant along the entire length of a vertical line, it passes through an infinite number of points on the graph.
Each point on the graph that shares the same x-coordinate as the vertical line will be intersected by the line. Therefore, the line passes through an infinite number of points. These points are distributed vertically along the y-axis, covering the entire range of the graph.
In conclusion, when Joelle drew a vertical line to represent a function on the graph, the line passed through an infinite number of points. This is because a vertical line intersects the graph at every point with the same x-coordinate, resulting in an infinite number of intersections.
For more such questions on function
https://brainly.com/question/2328150
#SPJ8
9. A police car drives with a constant speed of 52 miles per hour. How far can it travel in
255 minutes?
Answers
Answer:
221 miles
Step-by-step explanation:
1 hour = 60 minutes
52miles/hour = 52miles/60 minutes
then:
52 miles ⇔ 60 minutes
P miles ⇔ 255 minutes
P = 255 * 52 / 60
P = 221 miles
How to find proportional parts in triangles and parallel lines?
Answers
The proportional parts in triangles and parallel lines can be found by similarity and ratios.
The ratio of any related side lengths is always the same when two triangles are similar. The scale factor can be used to identify the triangle's proportionate components. The longer side of one triangle is twice as long as the comparable side in the other. In contrast, the alternate interior angles are identical when two parallel lines are cut by a transverse line. Additionally, the matching angle pairs are all equal. Trigonometry can be used to determine the length of the opposite side of a pair of parallel lines if we know the length of one side and the matching angle.
One can use similarity ratios to determine the proportional components of triangles and parallel lines. Comparable geometries have equivalent sides that are in proportion. To get the missing side length, one can arrange ratios of corresponding sides and utilise cross-multiplication. Further, similar triangles can also be employed. When two triangles are comparable, their corresponding sides and angles are proportionate and equal.
Read more about proportional parts on:
https://brainly.com/question/20291607
#SPJ4
A recent report found that a local creamery has a 35% market share in a certain region. Employees in the marketing
department of the creamery conduct a survey to confirm the results by randomly selecting 300 residents from the regon
The employees asked the question which brand of ice cream do you usually purchase to each resident Use a normal
approximation to calculate the probability that at most 80 of these people will choose the creamery's brand. Use a T1-83,
83 plus, or T-34 calculator to find the probability
- Round your answer to four decimal places
Answers
Using the normal approximation to the binomial, it is found that there is a 0.0015 = 0.15% probability that at most 80 of these people will choose the creamery's brand.
In a normal distribution with mean \(\mu\) and standard deviation \(\sigma\), the z-score of a measure X is given by:
\(Z = \frac{X - \mu}{\sigma}\)
It measures how many standard deviations the measure is from the mean. After finding the z-score, we look at the z-score table and find the p-value associated with this z-score, which is the percentile of X.The binomial distribution is the probability of x successes on n trials, with p probability of a success on each trial. It can be approximated to the normal distribution with \(\mu = np, \sigma = \sqrt{np(1-p)}\).In this problem:
35% market share, hence \(p = 0.35\).300 residents are surveyed, hence \(n = 300\).The mean and the standard deviation are given by:
\(\mu = np = 300(0.35) = 105\)
\(\sigma = \sqrt{np(1-p)} = \sqrt{300(0.35)(0.65)} = 8.26\)
Using continuity correction, the probability that at most 80 of these people will choose the creamery's brand is \(P(X \leq 80 + 0.5) = P(X \leq 80.5)\), which is the p-value of Z when X = 80.5, thus:
\(Z = \frac{X - \mu}{\sigma}\)
\(Z = \frac{80.5 - 105}{8.26}\)
\(Z = -2.97\)
\(Z = -2.97\) has a p-value of 0.0015.
0.0015 = 0.15% probability that at most 80 of these people will choose the creamery's brand.
A similar problem is given at https://brainly.com/question/25298431
Which polynomial degree classic can be used to describe the following expression
Answers
The Degree of a Polynomial is the largest of the degrees of the individual terms. Add the degrees of the variables of each term to decide what is the Degree of the Polynomial. Degree of term 1 is 2 (1+1= 2), Degree of term 2 is 6 (2+4 = 6), Degree of term 3 is 7 (5+2 = 7) 7 is the Degree of the Polynomial.
Step-by-step explanation:The degree of a polynomial is the largest exponent on one of its variables (for a single variable), or the largest sum of exponents on variables in a single term (for multiple variables). Here, the term with the largest exponent is, so the degree of the whole polynomial is 6. Polynomials are algebraic expressions that consist of variables and coefficients. Variables are also sometimes called indeterminates. We can perform arithmetic operations such as addition, subtraction, multiplication, and also positive integer exponents for polynomial expressions but not division by variable. A 1st-degree polynomial is just a straight line also known as a linear equation. It is called linear because it is a straight line. The rate of change is the slope of the line and is constant. A 2nd-degree polynomial is a parabola. A polynomial of degree 4 is called a bi-quadratic polynomial. A cubic polynomial is a name given to a polynomial of degree three.
McKenzie has a 2 quart pitcher.she fills itup two times with juice.how many cups ofjuice was she able to make?
Answers
Since McKenzie fills the 2-quart pitcher 2 times, there are a total of 4 quarts.
2 quarts × 2 times filled = 4 quarts
Recall that
1 quart = 4 cups
Since each quart is 4 cups, having 4 quarts is equivalent to
4 quarts × 4 = 16 cups
Therefore, there are a total of 16 cups of juice that was made.
kenneth, a competitor in cup stacking, claims that his average stacking time is 8.2 seconds. during a practice session, kenneth has a sample stacking time mean of 7.8 seconds based on 11 trials. at the 4% significance level, does the data provide sufficient evidence to conclude that kenneth's mean stacking time is less than 8.2 seconds? accept or reject the hypothesis given the sample data below.
Answers
Do not reject the null hypothesis because the p-value 0.0401 is greater than the significance level \(\alpha = 0.04\)
Given:
\(H_0: \mu = 8.2 \text{ seconds}, \, H_a: \mu < 8.2 \text{ seconds}\)
\(\alpha = 0.04\)
\(z_0 = -1.75\)
\(p = 0.0401\)
If p value is greater than level of significance alpha then we accept null hypothesis otherwise we reject null hypothesis.
Here \(p = 0.0401\) which is greater than the alpha \(0.04\), hence do not reject null hypothesis.
To learn more about hypothesis testing visit: brainly.com/question/17099835
#SPJ4
Solve.
1/2 (y - 12) = -6

Answers
Answer:
y=0
Step-by-step explanation:
Step 1: Simplify both sides of the equation
1/2(y−12)=−6
(
1
2
)(y)+(
1
2
)(−12)=−6(Distribute)
1
2
y+−6=−6
1
2
y−6=−6
Step 2: Add 6 to both sides.
1
2
y−6+6=−6+6
1
2
y=0
Step 3: Multiply both sides by 2.
2*(
1
2
y)=(2)*(0)
y=0
Allan has four and six-fourteenths buckets of paint. He spills three and eleven-twelfths buckets. Estimate how many buckets of paint Allan has left.
one-half bucket
1 bucket
one and one-half buckets
2 buckets
Answers
Answer:
Allan is left with \(\frac{43}{84}\) buckets of paint.
Step-by-step explanation:
How to subtract mixed and unlike fractions?
To perform subtraction on mixed fractions, the fractions are at first converted to improper fractions. The improper and unlike fractions are then converted to like fractions (fractions with the same denominator).According to the given question,
The total quantity of paint that Allan has = 4 + \(\frac{6}{14}\) = 4 \(\frac{6}{14}\)
Converting the above mixed fraction to an improper fraction :
4 \(\frac{6}{14}\)
= \(\frac{(4*14) + 6}{14}\)
= \(\frac{62}{14}\)
Thus Allan has \(\frac{62}{14}\) buckets of paint.
Quantity of paint spilled = 3 + \(\frac{11}{12}\) = 3 \(\frac{11}{12}\)
Converting the above mixed fraction to an improper fraction :
3 \(\frac{11}{12}\)
= \(\frac{( 3 * 12 ) + 11}{12}\)
= \(\frac{47}{12}\)
Therefore, the quantity of paint left with Allan = Total quantity - Quantity spilled
= \(\frac{62}{14}\) - \(\frac{47}{12}\)
To perform this subtraction, these two unlike fractions should first be converted to like fractions (fractions with the same denominator).
Converting to like fractions involves the following steps:
Taking L.C.M of 14 and 12 = 84 Dividing 84 by 14 (gives 6) and multiplying 6 to 62Dividing 84 by 12 (gives 7) and multiplying 47 by 7= \(\frac{372}{84}\) - \(\frac{329}{84}\)
= \(\frac{372 - 329}{84}\)
= \(\frac{43}{84}\)
Thus, Allan is left with \(\frac{43}{84}\) buckets of paint.
To know more about the subtraction of fractions, visit:
https://brainly.com/question/28641578
June was thinking of a number. June doubles it, then adds 18 to get an answer of 90. 7. What was the original number?
Answers
The required original number that June was thinking of is 36.
Let's assume the original number June was thinking of is represented by "x". According to the problem, June doubles the original number (2x) and adds 18 to get an answer of 90. We can write this as the equation:
\(2x + 18 = 90\)
To find the value of x, we need to isolate it on one side of the equation. Let's subtract 18 from both sides:
\(2x = 90 - 18 \\ 2x = 72\)
Now, we divide both sides of the equation by 2 to solve for x:
\(x = 72 / 2 \\ x = 36\)
Therefore, the original number that June was thinking of is 36.
Learn more about equation here,
http://brainly.com/question/2972832
#SPJ4
Someone explain please

Answers
Answer:
SA = 94 ft²
Step-by-step explanation:
To find the surface area of a rectangular prism, you can use the equation:
SA = 2 ( wl + hl + hw )
SA = surface area of rectangular prism
l = length
w = width
h = height
In the image, we are given the following information:
l = 4
w = 5
h = 3
Now, let's plug in the information given to us to solve for surface area:
SA = 2 ( wl + hl + hw)
SA = 2 ( 5(4) + 3(4) + 3(5) )
SA = 2 ( 20 + 12 + 15 )
SA = 2 ( 47 )
SA = 94 ft²
If this answer helped you, please leave a thanks!
Have a GREAT day!!!
One ticket to a show costs $20 at full price. Susan buys 4 tickets using a coupon that gives her 25% discount.
Answers
Answer: \(\$60\)
Step-by-step explanation:
Given
One ticket costs $20
Susan buys 4 tickets with 25% discount
Cost of 4 tickets is \(4\times 20=\$80\)
On applying discount, it costs
\(\Rightarrow 80-80\times 0.25\\\Rightarrow 80(1-0.25)\\\Rightarrow 80\times 0.75=\$60\)
So, total cost of tickets is \(\$60\).
What is the equivalent ratio to 7 : 20
Answers
Answer:
14:40
Step-by-step explanation:
A 40 foot long wire stretches from the top of a vertical pole to a stake in the ground 18 feet from the foot of the pole. find, to the nearest degree, the measure of the acute angle that the wire makes with the ground.
Answers
The measure of the acute angle that the wire makes with the ground is 63.27°.
The situation forms a right angle triangle.
How to find the angle of a right triangle?The length of the wire is the hypotenuse of the right triangle.
The distance from the foot of the pole is the adjacent side of the right triangle.
Therefore,
cos ∅ = adjacent / hypotenuse
cos ∅ = 18 / 40
cos ∅ = 0.45
∅ = cos⁻¹ 0.45
∅ = 63.2563160496
∅ = 63.27°
learn more on right angle triangle here: https://brainly.com/question/14866099
#SPJ1
Find the slope:
Find the slope

Answers
Answer: Undefined
Step-by-step explanation:
A vertical line has an undefined slope.
Slope can be found with change in y over change in x, but in a vertical line, this simplifies to dividing by 0, which comes out as undefined.
Learn more about an undefined slope here:
https://brainly.com/question/20383597
Use elimination to solve for x and y:
- 2x - y = 9
2x - 9y = 1
Answers
Answer:
x=-4, y=-1
Step-by-step explanation:
Given the following system of equations, solve the system using elimination.
\(\left \{ {{-2x-y=9} \atop {2x-9y=1}} \right.\)
(1) - Add the equations together
\(\left\begin{array}{ccc}&-2x-y=9\\+&2x-9y=1\end{array}\right\\\\\Longrightarrow -10y=10\\\\\therefore \boxed{y=-1}\)
Notice how the x term was eliminated, hence the name for this method is "elimination."
(2) - Take the value we just found for y and plug it into either of the two equations and solve for x
\(2x-9y=1\\\\\Longrightarrow 2x-9(-1)=1\\\\\Longrightarrow 2x+9=1\\\\\Longrightarrow 2x=-8\\\\\therefore \boxed{x=-4}\)
(3) - Thus, the system is solved. When x=-4, y=-1.
3 A shop sells pairs of trainers for £52 each.
They make a percentage profit of 100% on each pair.
What was the cost of each pair of trainers to the shop?
Answers
Answer:
26
Step-by-step explanation:
What type of triangle is a 50/50 80?
Answers
50-50-80 is a Isosceles acute triangle.
An isosceles acute triangle is a triangle in which all three angles are less than 90°, and at least two of its angles are equal in measurement. One example of the angles of an isosceles acute triangle is 50°, 50°, and 80°.
Properties of Isosceles Acute Triangle
It is easy to point to an isosceles acute triangle if we know its properties. The properties of the isosceles acute triangle are noted below:
Two angles and two sides opposite those angles are equal.All three angles are less than 90° (acute angles).The summation of all the interior angles is 180°.Read more about the Isosceles acute triangle.:
https://brainly.com/question/8155990
#SPJ4
FOR 14 points! A group of friends are going to see the newest action movie. The price of a ticket is $6.25. As a group, they spent $12 on refreshments. If they spent a total of $30.75 on tickets and refreshments, how many people went to the movies? Which equation represents the scenario? How many people went to the movies?
Answers
x = number of people went to movies
equation
6.25x + 12 = 30.75
6.25x = 30.75 - 12
6.25x = 18.75
x = 18.75 / 6.25
x = 3
answer
3 people
Answer:
6.25x + 12 = 30.75
3 people
Step-by-step explanation:
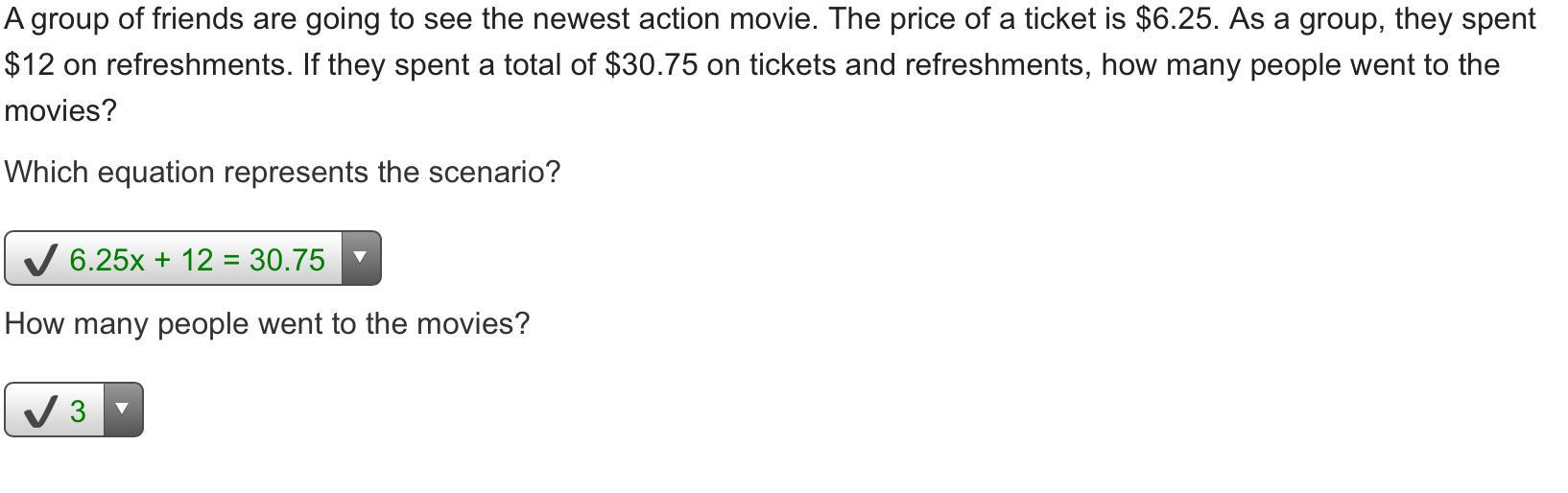
when a number is rounded to 400,000 when rounded to nearest 100 thousand and rounded to 350,000 when rounded to nearest ten thousand what is a possible number
Answers
Let’s call the number we’re looking for “x”. If x is rounded to 400,000 when rounded to the nearest 100,000 and rounded to 350,000 when rounded to the nearest 10,000, then we know that x must be between 375,000 and 424,999.
This is because if we round x down to the nearest 100,000, we get 300,000 (since it rounds down to the nearest hundred thousand), and if we round x up to the nearest 100,000, we get 500,000 (since it rounds up to the nearest hundred thousand). Therefore, x must be between these two numbers.
Similarly, if we round x down to the nearest 10,000, we get 340,000 (since it rounds down to the nearest ten thousand), and if we round x up to the nearest 10,000, we get 359,999 (since it rounds up to the nearest ten thousand). Therefore, x must be between these two numbers as well.
Therefore, a possible number that satisfies these conditions is any number between 375,000 and 424,999 that rounds to 400,000 when rounded to the nearest hundred thousand and 350,000 when rounded to the nearest ten thousand.
I hope that helps!
47. Find the probability that a point chosen at random would land in the triangle. Give your answer as a percent.

Answers
The probability that a point chosen at random would land in the inscribed triangle is 31.831%.
To find the probability that a point chosen at random would land in the inscribed triangle.
we need to compare the areas of the triangle and the circle.
Since the triangle is inscribed in the circle, the base of the triangle is equal to the diameter of the circle, which is twice the radius (2× 6 = 12m). The height of the triangle is equal to the radius of the circle (6m).
Using these values, we can calculate the area of the triangle:
A = (1/2) × 12m×6m = 36m²
The area of the circle can be found using the formula for the area of a circle: A = π ×radius².
Substituting the radius (6m) into the formula:
A = π×(6m)² = 36πm²
Now, to find the probability that a point chosen at random would land in the triangle.
we divide the area of the triangle by the area of the circle and multiply by 100 to express it as a percentage:
Probability = (36m² / 36πm²) × 100
Probability = (1 / π) × 100
Probability = (1 / 3.14159) ×100 = 31.831%
To learn more on probability click:
https://brainly.com/question/11234923
#SPJ1
I need the answer to this problem asap

Answers
Answer: well you need to divid
Step-by-step explanation: well by dividing you get a answer so like you compare if the answer you got is for all of them and if it is not you say it is not proportional
Suppose z=x^2siny, x=−2s^2−5t^2, y=−10st.
A. Use the chain rule to find ∂z/∂s and ∂z/∂t as functions of x, y, s and t.
∂z/∂s=_________________________
∂z/∂t= _________________________
B. Find the numerical values of ∂z/∂s and ∂z/∂t when (s,t)=(−2,−1).
∂z/∂s(−2,−1)= ______________________
∂z/∂t(−2,−1)= ______________________
Answers
(a) Using the chain rule, ∂z/∂s = 2\(x^2\) cos(y) - 40xyt and ∂z/∂t = -20\(x^2\)siny.
(b) When (s, t) = (-2, -1), ∂z/∂s = 722 cos(20) - 320 and ∂z/∂t= -722 sin(20)
(a) To find ∂z/∂s and ∂z/∂t using the chain rule, we differentiate z with respect to s and t while considering the chain rule for each variable.
Let's start with ∂z/∂s:
∂z/∂s = (∂z/∂x)(∂x/∂s) + (∂z/∂y)(∂y/∂s)
Using the given equations for x and y, we substitute them into the expression for ∂z/∂s:
∂z/∂s = (∂z/∂x)(-4s) + (∂z/∂y)(-10t)
Differentiating z with respect to x and y separately, we find:
∂z/∂x = 2xysiny
∂z/∂y = \(x^2\)cosy
Substituting these derivatives back into the expression for ∂z/∂s, we have:
∂z/∂s = 2\(x^2\)cos(y) - 40xyt
Similarly, for ∂z/∂t, we have:
∂z/∂t = (∂z/∂x)(∂x/∂t) + (∂z/∂y)(∂y/∂t)
Using the given equations for x and y, we substitute them into the expression for ∂z/∂t:
∂z/∂t = (∂z/∂x)(-10t) + (∂z/∂y)(-s)
Substituting the derivatives of z with respect to x and y, we find:
∂z/∂t = -20\(x^2\)siny
(b) To find the numerical values of ∂z/∂s and ∂z/∂t when (s, t) = (-2, -1), we substitute these values into the expressions obtained in part (a).
∂z/∂s = 2\(x^2\) cos(y) - 40xy
∂z/∂t = -20\(x^2\) sin(y)
Substituting x = -2\(s^2\) - 5\(t^2\) and y = -10st into the expressions, we get:
∂z/∂s = 2\((-2s^2 - 5t^2)^2\) cos(-10st) - 40(-2\(s^2\) - 5\(t^2\))(-10st)
∂z/∂t = -20\((-2s^2 - 5t^2)^2\) sin(-10st)
Now, substituting (s, t) = (-2, -1) into these expressions, we have:
∂z/∂s(-2, -1) = \(2(4(-2)^4 + 20(-2)^2(-1)^2 + 25(-1)^4) cos(10(-2)(-1)) + 40(-2)^3(-1)^3\)
= 2(256 + 80 + 25) cos(20) - 320
= 2(361) cos(20) - 320
= 722 cos(20) - 320
∂z/∂t(-2, -1) = \(-20(4(-2)^4 + 20(-2)^2(-1)^2 + 25(-1)^4)\) sin(10(-2)(-1))
= -20(256 + 80 + 25) sin(20)
= -20(361) sin(20)
= -722 sin(20)
Therefore, ∂z/∂s(-2, -1) = 722 cos(20) - 320 and ∂z/∂t(-2, -1) = -722 sin(20).
Learn more about chain rule here:
https://brainly.com/question/30764359
#SPJ11
1) f(t)=t2+sin(2t)+2cos(2t)+e−tsin(3t) You must solve the problem manually. You can only use MATLAB or other computer tools to verify your solution.
Answers
The solution to the integral of f(t) = t^2 + sin(2t) + 2cos(2t) + e^(-t)sin(3t) is:
F(t) = (1/3)t^3 - (1/2)cos(2t) + (1/2)sin(2t) - 4e^(-t)cos(3t) + C
where F(t) represents the antiderivative or the indefinite integral of f(t).
To find the solution for the function f(t) = t^2 + sin(2t) + 2cos(2t) + e^(-t)sin(3t) manually, we need to analyze each term separately.
Term: t^2
The integral of t^2 with respect to t is (1/3)t^3.
Term: sin(2t)
The integral of sin(2t) with respect to t is -(1/2)cos(2t).
Term: 2cos(2t)
The integral of 2cos(2t) with respect to t is (1/2)sin(2t).
Term: e^(-t)sin(3t)
To integrate this term, we can use integration by parts. Let's define u = e^(-t) and dv = sin(3t) dt.
Taking the derivatives and integrals:
du = -e^(-t) dt
v = -(1/3)cos(3t)
Using the integration by parts formula:
∫ u dv = uv - ∫ v du
∫ e^(-t)sin(3t) dt = -e^(-t)(1/3)cos(3t) - ∫ -(1/3)cos(3t)(-e^(-t)) dt
= -e^(-t)(1/3)cos(3t) + (1/3)∫ cos(3t)e^(-t) dt
We can apply integration by parts again to the remaining integral:
Let u = cos(3t) and
dv = e^(-t) dt.
Taking the derivatives and integrals:
du = -3sin(3t) dt
v = -e^(-t)
Using the integration by parts formula again:
∫ cos(3t)e^(-t) dt = -e^(-t)cos(3t) - ∫ (-e^(-t))(-3sin(3t)) dt
= -e^(-t)cos(3t) + 3∫ e^(-t)sin(3t) dt
Substituting the value we found for the previous integral:
∫ e^(-t)sin(3t) dt = -e^(-t)(1/3)cos(3t) + (1/3)(-e^(-t)cos(3t) + 3∫ e^(-t)sin(3t) dt)
Now we can solve for the integral:
∫ e^(-t)sin(3t) dt = (-e^(-t)(1/3)cos(3t) - (1/3)e^(-t)cos(3t))/(1 - 1/3)
= -3e^(-t)(1/3)cos(3t) - 3e^(-t)cos(3t)
= -e^(-t)cos(3t) - 3e^(-t)cos(3t)
= -4e^(-t)cos(3t)
Now we can put all the terms together:
∫ f(t) dt = (1/3)t^3 - (1/2)cos(2t) + (1/2)sin(2t) - 4e^(-t)cos(3t)
Let's continue with the expression for the integral:
∫ f(t) dt = (1/3)t^3 - (1/2)cos(2t) + (1/2)sin(2t) - 4e^(-t)cos(3t) + C
where C is the constant of integration.
So, the solution to the integral of f(t) = t^2 + sin(2t) + 2cos(2t) + e^(-t)sin(3t) is:
F(t) = (1/3)t^3 - (1/2)cos(2t) + (1/2)sin(2t) - 4e^(-t)cos(3t) + C
where F(t) represents the antiderivative or the indefinite integral of f(t).
To know more about integral visit
https://brainly.com/question/31433890
#SPJ11
14.1 x 2.7. Show work pls!
Answers
Answer:
14.1 x 2.7
= 141/10 . 27/10
=3807/100
=38,07
Step-by-step explanation:
what is the answer to |2x-12|=-4x ?
Answers
Answer: -6x
Step-by-step explanation: 24=-4x
24/-4 = -6x
Answer: -6
Step-by-step explanation:
|2x-12|=-4x
if 2x-12 ≥0 or x ≥ 6 then|2x-12|=2x-12
2x-12=-4x
6x=12
x=2 to exclude since x ≥6
if 2x-12 <0 then|2x-12|=-(2x-12)
-2x+12 =-4x
2x =-12
x=-6
Answer x=-6
please what's the answer

Answers
Answer: B
Step-by-step explanation: 60 divided by 15 is 4 and 4 is a natural number