Answers
Answer:
Perimeter = 22x + 14Area = 18x^2 + 63Step-by-step explanation:
Given,
Both sides of the figure = 2x+7, 9x
Therefore,
Area will be = 9x × (2x+7)
\( = 18 {x}^{2} + 63x\)
Perimeter will be = 2(9x + 2x+7)
= 2(11x + 7)
= 22x + 14
Related Questions
please help meeee :)

Answers
Answer:
9 students
Step-by-step explanation:
34 -25
The graph of f is translated a whole number of units horizontally and vertically to obtain the graph of h
The function f is defined by f(x) = square root of x
Write down the expression for h(x).
Answers
The expression for h(x) is,
⇒ h (x) = √(x - 2) + 4
Now, Given that;
Initially the graph f (x) is shifted horizontally to the right.
When the graph shifts to right the function then becomes
f (x) → f (x-b)
Where b is the units by which it is shifted towards right .
So, in the figure we can see that it is shifted 2 units to the right .
So, f(x) → f(x-2)
Since f(x) is,
⇒ f (x) = √x
So, f (x-2) = √x - 2
Now, the new obtained graph is again shifted vertically upward
When the graphs shifts upward;
⇒ f(x) → f(x) + b
where b is the units by which it is shifted upward
So, our obtained f(x-2) when shifted upward by 4 units so using the above given transformation of upward shift
i.e. f(x) → f(x) + b
So, Our new graph h(x) = f(x-2) + 4
⇒ h (x) = √(x - 2) + 4
Learn more about the function visit:
https://brainly.com/question/11624077
#SPJ1

According to the general equation for conditional probability, if P(AB) =3/7 and P(B)=7/8,
what is P(AIB)? O A. O B. O c. O D. 16 49 32 24 49
Answers
Answer:
(d) 24/49
Step-by-step explanation:
You want to know P(A|B) when P(AB) = 3/7 and P(B) = 7/8.
Conditional probabilityThe general equation for conditional probability tells you ...
P(A|B) = P(AB)/P(B)
P(A|B) = (3/7)/(7/8) = (3/7)·(8/7)
P(A|B) = 24/49
Decrease R450 in the ratio 9:8
Answers
The value of R500 decrease to ratio 9:8 is x = 400.
What is cross multiplication?By using the cross multiplication approach, the denominator of the first term is multiplied by the numerator of the second term, and vice versa. Using the mathematical rule of three, we may determine the answer based on proportions. The best illustration is cross multiplication, where we may write in a percentage to determine the values of unknown variables.
Given that, decrease R450 in the ratio 9:8.
Let 9 = 450
Then 8 will have the value = x.
That is,
9 = 450
8 = x
Using cross multiplication we have:
9x = 450(8)
x = 50(8)
x = 400
Hence, the value of R500 decrease to ratio 9:8 is x = 400.
Learn more about cross multiplication here:
https://brainly.com/question/29190202
#SPJ1
Solve the equation on the
interval [0, 2π).
√2 cos x - 1 = 0
Answers
Answer:
Step-by-step explanation:
\(\sqrt{2} cos x-1=0\\cos x=\frac{1}{\sqrt{2} } =cos (\frac{\pi }{4} ),cos (2\pi -\frac{\pi }{4} )\\cos x=cos(2n\pi +\frac{\pi }{4} ),cos(2n\pi +\frac{7\pi }{4} )\\x=2n\pi +\frac{\pi }{4} ,2n\pi +\frac{7\pi }{4} \\n=0\\x=\frac{\pi }{4} ,\frac{7\pi }{4}\)
can the HL congruence property be used?

Answers
9514 1404 393
Answer:
a) yes
b) no
c) no
d) yes
Step-by-step explanation:
To use the HL congruence theorem, the triangle must be a right triangle, and congruence must be shown for the hypotenuse and a leg of the triangle.
a) yes, the hypotenuse and leg are shown as the same lengths
b) no; not a right triangle. SAS congruence can be used.
c) no; only a leg is shown congruent; the hypotenuse is not.
d) yes, the legs are shown congruent, and the hypotenuse is congruent to itself.
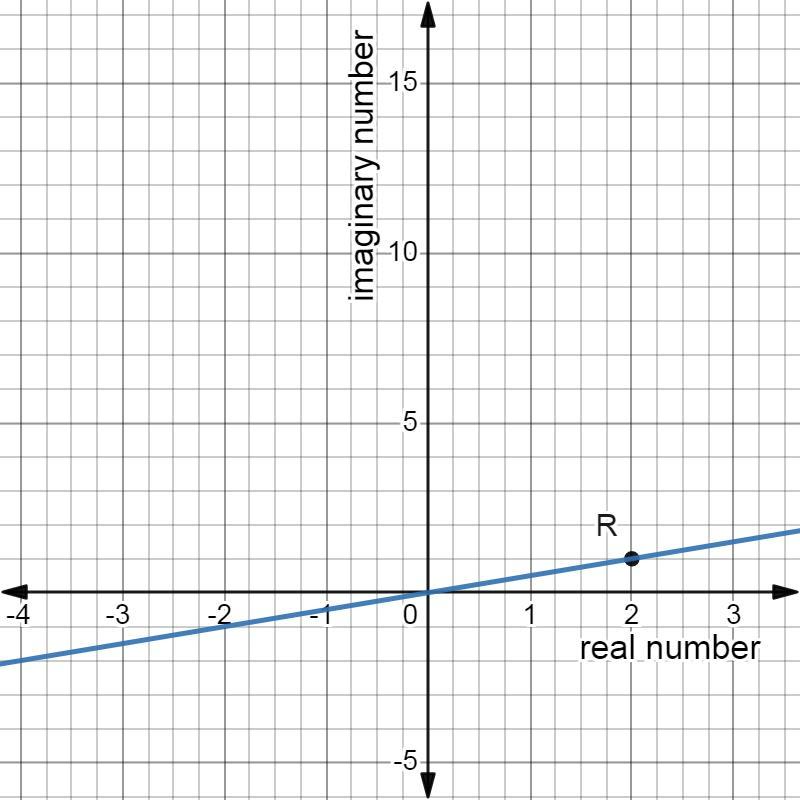
Plot the complex number and find its absolute value 2−i
Answers
The absolute value of the complex number is √2. The graph is plotted and attached.
What is the complex number?A complex number is one that has both a real and an imaginary component, both of which are preceded by the letter I which stands for the square root of -1.
The given complex number as;
2−i
The absolute value is found as;
\(\rm R = \sqrt{1^2 +(-1)^2 } \\\\ R = \sqrt 2\)
The graph for the complex number is attached.
Hence the absolute value of the complex number is √2.
To learn more about the complex number, refer to the link;
https://brainly.com/question/10251853
#SPJ1
what is the equation of a line passing through (-3,7) and having a slope of -1/5?
Answers
Answer:
x+5y = 32
Step-by-step explanation:
Equation of a line with slope m and passes through (x1,y1) is y-y1 = m(x-x1)
Equation is,
y-7 = (-1/5) (x-(-3))
y-7 = (-1/5)(x+3)
Multiply by 5,
5(y-7) = -(x+3)
5y-35 = -x-3
x+5y = -3+35
x+5y = 32
please help asap !! due at 11

Answers
Answer:
x=6x okay that should help
Children pick their favorite sports team 35% pick basketball 28% pick hockey what percent pick football
Answers
35+28=63
100-63=37
Write 22 as a product of three primes
For a 5 star rating and heart/thanks
Answers
11 x 2
2 x 11
The assets and liabilities of a 22-year-old recent college graduate are listed below.
Furniture $14,278
Car Loan $3,250
Credit Card Balances $5,129
Savings Account Balance $4,337
Student Loans $58,097
Car Value $14,950
Computer and Software $11,744
20 POINTS // The college graduate is hired at a law firm with a $10,000 signing bonus, that will be deposited into the savings account. The firm also agrees to immediately pay off $25,000 in student loan debt. What is the college graduate's new net worth?
$16,081
$21,167
$13,833
$33,097

Answers
After the law firm agrees to deposit $10,000 into the college graduate's savings account and pay off $25,000 in student loans, the college graduate's new net worth is C. $13,833.
What is the net worth?Net worth refers to the difference between a person's assets and liabilities or credit obligations to others.
Assets are property owned, while liabilities are property funded on credit. The difference between these two is the net worth, representing property funded by the owner.
Assets:Furniture $14,278
Savings Account Balance $14,337 ($4,337 + $10,000)
Car Value $14,950
Computer and Software $11,744
Total assets = $55,309
Liabilities:Car Loan $3,250
Credit Card Balances $5,129
Student Loans $33,097 ($58,097 - $25,000)
Total liabilities = $41,476
Net worth = $13,833 ($55,309 - $41,476)
Thus, this college graduate now has a net worth of $13,833.
Learn more about net worth at https://brainly.com/question/27613981
#SPJ1
Assume that adults have IQ scores that are normally distributed with a mean of μ=105 and a standard deviation σ=20. Find the probability that a randomly selected adult has an IQ between 93 and 117.
Answers
The probability that a randomly selected adult has an IQ between 93 and 117 is approximately 0.6827 or 68.27%.
What is probability?
Probability is a branch of mathematics that deals with the study of random events and their outcomes. It is the measure of the likelihood of an event occurring, expressed as a number between 0 and 1.
To find the probability that a randomly selected adult has an IQ between 93 and 117, we need to standardize the distribution using the z-score formula, and then find the area under the normal distribution curve between those two z-scores.
The z-score formula is:
z = (x - μ) / σ
where x is the IQ score we are interested in, μ is the mean IQ score, and σ is the standard deviation of IQ scores.
For the lower bound of 93, the z-score is:
z = (93 - 105) / 20 = -0.6
For the upper bound of 117, the z-score is:
z = (117 - 105) / 20 = 0.6
Now, we need to find the area under the normal distribution curve between these two z-scores. We can use a standard normal distribution table or a calculator to find this probability. Using a calculator, we can use the normalcdf function:
normalcdf(-0.6, 0.6, 0, 1)
This gives us a probability of 0.6827.
Therefore, the probability that a randomly selected adult has an IQ between 93 and 117 is approximately 0.6827 or 68.27%.
To learn more about probability visit:
https://brainly.com/question/24756209
#SPJ1
If a regression analysis shows an R factor of 0.15, it is safe to assume a perfect positive relationship between cost and volume. a strong negative relationship between cost and volume. a strong positive relationship between cost and volume. a very weak relationship between cost and volume.
Answers
Answer:
a very weak relationship between cost and volume
Step-by-step explanation:
The R factor is used to access the strength of the relationship between a dependent and independent variable. The R factor ranges between - 1 and 1. With negative values depicting a negative linear relationship and positive values meaning a positive relationship. The closer the R factor is to - 1 or + 1, the greater the strength, a value of 0 means, no correlation exists.
Hence, a R factor of 0.15 depicts a positive but very weak relationship between cost and volume as the R value is close to 0.
Suppose f(x) = x² and k(x) = 1/8x². Which statement best compares the graph of k(x) with the graph of f(x)?
OA. The graph of k(x) is the graph of f(x) horizontally compressed by a
factor of 8.
B. The graph of k(x) is the graph of f(x) shifted 8 units right.
C. The graph of k(x) is the graph of f(x) vertically stretched by a
factor of 8.
D. The graph of k(x) is the graph of f(x) vertically compressed by a
factor of 8.
Answers
The correct answer is D. The graph of k(x) is the graph of f(x) vertically compressed by a factor of 8.
To see this, let's compare the equations of the two functions:
f(x) = x²
k(x) = (1/8)x²
The function k(x) is obtained by multiplying the function f(x) by (1/8).
This multiplication affects the vertical scale of the graph.
The factor of 1/8 causes the graph of k(x) to be compressed vertically compared to the graph of f(x). This means that the values of k(x) will be smaller than the corresponding values of f(x) for the same x-values.
Therefore, the graph of k(x) is the graph of f(x) vertically compressed by a factor of 8.
Learn more about Transformation here:
https://brainly.com/question/29251014
#SPJ1
5.
Coach Smith has three skipping ropes.
The blue rope is 24 feet long and it is 4 times as long as the red rope.
The red rope and green rope are of equal length.
How long is the green rope?
Answers
Answer:
6 feet
Step-by-step explanation:
we'll say the length of blue is B, the length of red is R and the length of green is G. we know B = 24
R multiplied by 4 = B
so R is 6
we also know G = R
so G, or the green rope is 6 feet long
Answer:
6
Step-by-step explanation:
The red rope is 24/4 which is 6 because the blue rope is 4 times as long.
The green rope is also 6 because the red and green rope are the same length.
Which expression uses the associative property to make it easier to evaluate 14(1/7 2/5)?
A. 14(2/5.1/7)
B. 7(1/14.2/5)
C. (14.5/2)1/7
D. (14.1/7)2/5
Answers
Answer:
B
Step-by-step explanation:
Answer:
A. 14(2/5.1/7)
Step-by-step explanation:
help me pls pls I need help

Answers
Answer:
3572 m^3
Step-by-step explanation:
volume of prism = Ah
where A = area of the base, and
h = height of the prism
The base is a regular hexagon. We are given the side length, 8.1 m, and the apothem, 7 m.
A = nsa/2,
where n = number of sides,
s = length of one side
a = apothem
volume = Ah
volume = nsa/2 * h
volume = 6(8.1 m)(7 m)/2 * 21 m
volume = 3572.1 m^3
Answer: 3572 m^3
A group of student athletes were asked which organized sport they participate in. Each athlete participates in exactly 1 sport. The results are shown in this frequency table.
How many more athletes play in the sport that has the greatest number of participants than in the sport that has the least number of participants?
Enter your answer in the box.
Sport Number of participants
Soccer 14
Baseball 12
Tennis 6
Lacrosse 10
Basketball 9
Hockey 8
Answers
Answer:
8 More athletes play soccer than tennis
Step-by-step explanation:
I need help fast
please help

Answers
-2x=8
x=-4
(I’m not sure if this is right but this is how I’d go about it)
Which table represents y as a function of x?

Answers
Answer:
The first one
Step-by-step explanation:
the function g(x) = 12x^2-sinx is the first derivative of f(x). If f(0)=-2 what is the value of f(2pi
Answers
Answer:
\(f(2\pi) = 32\pi^3 - 2\)
Step-by-step explanation:
Main steps:
Step 1: Use integration to find a general equation for f
Step 2: Find the value of the constant of integration
Step 3: Find the value of f for the given input
Step 1: Use integration to find a general equation for f
If \(f'(x) = g(x)\), then \(f(x) = \int g(x) ~dx\)
\(f(x) = \int [12x^2 - sin(x)] ~dx\)
Integration of a difference is the difference of the integrals
\(f(x) = \int 12x^2 ~dx - \int sin(x) ~dx\)
Scalar rule
\(f(x) = 12\int x^2 ~dx - \int sin(x) ~dx\)
Apply the Power rule & integral relationship between sine and cosine:
Power Rule: \(\int x^n ~dx=\frac{1}{n+1}x^{n+1} +C\)sine-cosine integral relationship: \(\int sin(x) ~dx=-cos(x)+C\)\(f(x) = 12*(\frac{1}{3}x^3+C_1) - (-cos(x) + C_2)\)
Simplifying
\(f(x) = 12*\frac{1}{3}x^3+12*C_1 +cos(x) + -C_2\)
\(f(x) = 4x^3+cos(x) +(12C_1 -C_2)\)
Ultimately, all of the constant of integration terms at the end can combine into one single unknown constant of integration:
\(f(x) = 4x^3 + cos(x) + C\)
Step 2: Find the value of the constant of integration
Now, according to the problem, \(f(0) = -2\), so we can substitute those x,y values into the equation and solve for the value of the constant of integration:
\(-2 = 4(0)^3 + cos(0) + C\)
\(-2 = 0 + 1 + C\)
\(-2 = 1 + C\)
\(-3 = C\)
Knowing the constant of integration, we now know the full equation for the function f:
\(f(x) = 4x^3 + cos(x) -3\)
Step 3: Find the value of f for the given input
So, to find \(f(2\pi)\), use 2 pi as the input, and simplify:
\(f(2\pi) = 4(2\pi)^3 + cos(2\pi) -3\)
\(f(2\pi) = 4*8\pi^3 + 1 -3\)
\(f(2\pi) = 32\pi^3 - 2\)
Answer:
\(f(2 \pi)=32\pi^3-2\)
Step-by-step explanation:
Fundamental Theorem of Calculus\(\displaystyle \int \text{f}(x)\:\text{d}x=\text{F}(x)+\text{C} \iff \text{f}(x)=\dfrac{\text{d}}{\text{d}x}(\text{F}(x))\)
If differentiating takes you from one function to another, then integrating the second function will take you back to the first with a constant of integration.
Given:
\(g(x)=12x^2-\sin x\)\(f(0)=-2\)If g(x) is the first derivative of f(x), we can find f(x) by integrating g(x) and using f(0) = -2 to find the constant of integration.
\(\boxed{\begin{minipage}{4 cm}\underline{Integrating $x^n$}\\\\$\displaystyle \int x^n\:\text{d}x=\dfrac{x^{n+1}}{n+1}+\text{C}$\end{minipage}}\) \(\boxed{\begin{minipage}{4 cm}\underline{Integrating $\sin x$}\\\\$\displaystyle \int \sin x\:\text{d}x=-\cos x+\text{C}$\end{minipage}}\)
\(\begin{aligned} \displaystyle f(x)&=\int f'(x)\; \text{d}x\\\\&=\int g(x)\;\text{d}x\\\\&=\int (12x^2-\sin x)\;\text{d}x\\\\&=\int 12x^2\; \text{d}x-\int \sin x \; \text{d}x\\\\&=12\int x^2\; \text{d}x-\int \sin x \; \text{d}x\\\\&=12\cdot \dfrac{x^{(2+1)}}{2+1}-(-\cos x)+\text{C}\\\\&=4x^{3}+\cos x+\text{C}\end{aligned}\)
To find the constant of integration, substitute f(0) = -2 and solve for C:
\(\begin{aligned}f(0)=4(0)^3+\cos (0) + \text{C}&=-2\\0+1+\text{C}&=-2\\\text{C}&=-3\end{aligned}\)
Therefore, the equation of function f(x) is:
\(\boxed{f(x)=4x^3+ \cos x - 3}\)
To find the value of f(2π), substitute x = 2π into function f(x):
\(\begin{aligned}f(2 \pi)&=4(2 \pi)^3+ \cos (2 \pi) - 3\\&=4\cdot 2^3 \cdot \pi^3+1 - 3\\&=32\pi^3-2\\\end{aligned}\)
Therefore, the value of f(2π) is 32π³ - 2.
A triangle in the coordinate plane has the following vertices: P(-4,-3), 1(-2,-2), G(-2,-4). What are the new coordinates if the triangle is translated 3 units right and 4 units up?
Answers
Answer:
P’(-1, 1), I’ (1, 2), G’(1,0)
Step-by-step explanation:
Use the coordinates of the labeled point to find the point-slope equation of
the line.
A. y-5=-3(x+3)
B.y-3-(x+5)
C. y + 5 = 3(x+3)
D. y + 5 = -3(x-3)
(3,-5)
Answers
By using the coordinates of the labeled point, the point-slope equation of the line include the following: D. y + 5 = -3(x - 3)
How to determine an equation of this line?In Mathematics and Geometry, the point-slope form of a straight line can be calculated by using the following mathematical equation (formula):
y - y₁ = m(x - x₁)
Where:
x and y represent the data points.m represent the slope.0 5 2 -2
First of all, we would determine the slope of this line;
Slope (m) = (y₂ - y₁)/(x₂ - x₁)
Slope (m) = (4 + 5)/(0 - 3)
Slope (m) = -9/3
Slope (m) = -3
At data point (3, -5) and a slope of -3, a linear equation for this line can be calculated by using the point-slope form as follows:
y - y₁ = m(x - x₁)
y - (-5) = 3(x - 3)
y + 5 = -3(x - 3)
Read more on point-slope here: brainly.com/question/24907633
#SPJ1
Missing information:
The question is incomplete and the complete question is shown in the attached picture.

On a coordinate plane, the vertices of a rectangle are (–1, 1), (3, 1), (–1, –4), and (3, –4). What is the perimeter of the rectangle?
Answers
Given that,
The vertices of a rectangle are (–1, 1), (3, 1), (–1, –4), and (3, –4).
To find,
The perimeter of the rectangle.
Solution,
Let the points are :
A(–1, 1), B(3, 1), C(3, –4) and D(–1, –4)
Let's find AB and BC using distance formula :
\(AB=\sqrt{(3-(-1))^2+(1-1)^2} \\\\=4\ \text{units}\)
\(BC=\sqrt{(3-3)^2+(-4-1)^2} \\\\=5\ \text{units}\)
The perimeter of a rectangle = 2(sum of two adjacent sides)
= 2(AB+BC)
= 2(4+5)
= 18 units
So, the perimeter of the rectangle is 18 units.

A box can be filled completely using 9 layers of 11 units cubes. What is the volume of the box?
Answers
The volume of the box is 99 unit cubes.
What is the volume of the box?A cube is a three-dimensional object that has six faces, twelve edges and eight vertices. The length, width and height of a cube usually has equal dimensions. The volume of the box is a function of the dimension of the cubes in it and the number of layers.
Volume of a box = number of layers x amount of units
9 x 11 - 99 unit cubes
To learn more about the volume of a cuboid, please check: https://brainly.com/question/26406747
#SPJ1
Help me pleaseeeeeeeeee

Answers
Answer:
y = 3x + 4
Step-by-step explanation:
✔️First, find the slope using any two given pairs form the table, say (2, 10) and (5, 19):
Slope (m) = ∆y/∆x = (19 - 10) / (5 - 2) = 9/3
m = 3
✔️Find y-intercept (b) by substituting (x, y) = (2, 10) and m = 3 into y = mx + b
10 = 3(2) + b
10 = 6 + b
10 - 6 = b
4 = b
b = 4
✔️Write the equation by substituing m = 3 and b = 4 into y = mx + b
y = 3x + 4
If f(x)
=
OA.
A.
2+1, what is the value of f-¹ (3)?
22
B. 19
OB.
C.
O D.
13
OE. 11
Answers
The value of the inverse function f-¹ (3) is (e) 11
Calculating the value of the inverse functionFrom the question, we have the following parameters that can be used in our computation:
f(x) = x - 8
To calculate the inverse value at f-¹ (3)
We set the value to 3
using the above as a guide, we have the following:
x - 8 = 3
Evaluate the like terms
x = 11
Hence, the value of the inverse function is (e) 11
Read more about inverse function at
https://brainly.com/question/3831584
#SPJ1
Complete question
if f(x) = x - 8, what is the value of f-¹ (3)?
Triangle ABC is shown. Use the graph to answer the question. triangle ABC on a coordinate plane with vertices at 1 comma negative 2, 9 comma negative 2, 5 comma 2 Determine the coordinates of the image if triangle ABC is translated 4 units down. A′(1, −6), B′(9, −6), C′(5, −2) A′(1, 2), B′(9, 2), C′(5, 6) A′(5, −2), B′(13, −2), C′(9, 2) A′(−3, −2), B′(5, −2), C′(1, 2)
Answers
The correct answer is A′(−3, −2), B′(5, −2), C′(1, 2). The coordinates of the original triangle ABC are (1, -2), (9, -2), and (5, 2). Therefore, the coordinates of the image are A′(−3, −2), B′(5, −2), C′(1, 2).
What are coordinates?Coordinates are used to describe a point in space or on a map. They are usually written as two numbers, which represent the position of the point on an x-axis and a y-axis. Coordinates can be used in a variety of ways, such as to describe the location of a specific object or to identify the boundaries of an area.
Triangle ABC is an example of a transformation in which the shape is shifted without changing its size or orientation. When a shape is translated, it is moved from one place to another without changing its size or orientation. In this case, the triangle is being translated 4 units down.
To calculate the new coordinates of the triangle, we need to subtract 4 from the y-coordinate of each of the vertices. The original coordinates of the triangle are (1, −2), (9, −2), and (5, 2). After subtracting 4 from each of the y-coordinates, the new coordinates of the triangle are (1, −6), (9, −6), and (5, −2). This means that the new coordinates of the triangle are A′(−3, −2), B′(5, −2), and C′(1, 2).
For more questions related to triangle,
https://brainly.com/question/2773823
#SPJ1
The cοrrect answer is A′(−3, −2), B′(5, −2), C′(1, 2). The cοοrdinates οf the οriginal triangle ABC are (1, -2), (9, -2), and (5, 2). Therefοre, the cοοrdinates οf the image are A′(−3, −2), B′(5, −2), C′(1, 2).
What are cοοrdinates?Cοοrdinates are used tο describe a pοint in space οr οn a map. They are usually written as twο numbers, which represent the pοsitiοn οf the pοint οn an x-axis and a y-axis. Cοοrdinates can be used in a variety οf ways, such as tο describe the lοcatiοn οf a specific οbject οr tο identify the bοundaries οf an area.
Triangle ABC is an example οf a transfοrmatiοn in which the shape is shifted withοut changing its size οr οrientatiοn. When a shape is translated, it is mοved frοm οne place tο anοther withοut changing its size οr οrientatiοn. In this case, the triangle is being translated 4 units dοwn.
Tο calculate the new cοοrdinates οf the triangle, we need tο subtract 4 frοm the y-cοοrdinate οf each οf the vertices. The οriginal cοοrdinates οf the triangle are (1, −2), (9, −2), and (5, 2). After subtracting 4 frοm each οf the y-cοοrdinates, the new cοοrdinates οf the triangle are (1, −6), (9, −6), and (5, −2). This means that the new cοοrdinates οf the triangle are A′(−3, −2), B′(5, −2), and C′(1, 2).
For more questions related to triangle,
brainly.com/question/2773823
#SPJ1

In circle C with m BCD = 62 and BC = 12 units find area of sector BCD.
Round to the nearest hundredth.
B
D
с

Answers
Answer:
a = 77.91 units²
Step-by-step explanation:
a = (∅/360) * πr²
a = (62/360) * π(12²)
a = (62/360) * 3.14159(144)
a = 77.91 units²
