find the union and intersection of the following family: d={dn:n∈n} , where dn=(−n,1n) for n∈n.
Answers
Given d = {dn: n ∈ N} where dn = (−n, 1/n) for n ∈ N.Find the union and intersection of the given family of d sets.
The given family of sets is {d1, d2, d3, ...} where di = (−i, 1/i) for all i ∈ N.1. To find the union of the given family of sets d, take the union of all sets in the given family of sets.i.e. d1 = (−1, 1), d2 = (−2, 1/2), d3 = (−3, 1/3), ...
Thus, the union of the given family of sets d is{d1, d2, d3, ...} = (-1, 1].Therefore, the union of the given family of sets d is (-1, 1].2. To find the intersection of the given family of sets d, take the intersection of all sets in the given family of sets .i.e. d1 = (−1, 1), d2 = (−2, 1/2), d3 = (−3, 1/3), ...Thus, the intersection of the given family of sets d is{d1, d2, d3, ...} = Ø. Therefore, the intersection of the given family of sets d is empty.
To know more about range visit:
https://brainly.com/question/28135761
#SPJ11
Related Questions
53% of 2343 american adults surveyed said, they have watched digitally streamed tv programming on some type of device. what sample size would be required for the width of a 99% ci to be at most 0.05 irrespective of the value of at 99%
Answers
The sample size that would be required for the width of 99% is 2653.
What is sample size?The number of subjects involved in a sample size is referred to as the sample size in market research. A set of people chosen from the general community who are thought to be a representative sample size for that particular study is referred to as the sample size.
The following details are given:
Margin of error, E = 0.025; Significance Level, = 0.01
The proportion p is estimated to be p = 0.53.
The significance level with a critical value of 0.01 is 2.58.
The smallest sample size needed to estimate the population proportion p within the necessary margin of error is determined using the formula shown below:
n >= p*(1-p)*(zc/E)2 n = 0.53 *(1 - 0.53*)2 n = 2652.97 *(1-p)*(2.58/0.025)2
As a result, we determine that n = 2653 is the minimal sample size needed to satisfy the criteria that
n >= 2652.97 and that it must be an integer value.
Sample size is 2653.
Learn more about probability here:
https://brainly.com/question/30034780
#SPJ4
Can someone please help me solve this question? Thank you

Answers
7-3= 4
so x will equal 4 :)
Answer:
x = 4
Step-by-step explanation:
Method 1:
x + 3 = 7
x = 7 - 3
x = 4
Method 2:
x + 3 - 3 = 7 - 3
x = 7 - 3
x = 4
(1 point) find the solution of the initial value problem: y′′−7y′=49t,y(0)=7,y′(0)=0.
Answers
The solution to the given initial value problem is \(y(t) = 7t^2 + 7\). To solve the initial value problem, we first find the characteristic equation by substituting \(y = e^{(rt)\) into the given differential equation.
This gives us the equation \(r^2 - 7r = 0\). Factoring out r, we get r(r - 7) = 0. Thus, we have two possible values for r: r = 0 and r = 7.
For the case of r = 0, the corresponding solution is \(y_1(t) = c_1,\) where c_1 is a constant.
For the case of r = 7, the corresponding solution is \(y_2(t) = c_2e^{(7t)\), where c_2 is another constant.
To find the particular solution that satisfies the initial conditions, we substitute \(y = y_1(t) + y_2(t)\) into the initial conditions y(0) = 7 and y'(0) = 0. We obtain the following equations:
\(y_1(0) + y_2(0) = 7,\\y_1'(0) + y_2'(0) = 0.\)
Substituting the solutions for y_1(t) and y_2(t), we have:
\(c_1 + c_2 = 7,\\c_2 * 7 = 0.\)
From the second equation, we find \(c_2 = 0\). Substituting this value into the first equation, we get \(c_1 = 7.\)
Therefore, the particular solution satisfying the initial conditions is \(y(t) = 7t^2 + 7.\)
Learn more about constant here: https://brainly.com/question/29166386
#SPJ11
What percent of the men surveyed shop online? Round your answer to the nearest whole number percent.

Answers
27.5% percent of the men surveyed shop online.
How do I calculate a percentage?When a fraction of a whole is expressed as a number between 0 and 100, it is called a percentage.
Calculating a percentage involves dividing an item by its sum and multiplying the result by 100. (Value/Total Value)100% is the formula used to calculate percentages.
The simplest way to use percentages is to compare two numbers, with the second number being rebased to 100. Consider that we are curious about the proportion of employed women who are female.
A % is a number or ratio stated as a fraction of 100 in mathematics (from the Latin per centum, "by a hundred"). The abbreviations "pct.", "pct.", and occasionally "pc" are also used to indicate it, while the percent symbol, "%," is most frequently employed.
Store = 37 women
Total women: 37/49 = 0.75%
Men: shop equals 52
Total men: 11 /40 = 27.5%
To learn more about percentage refer to:
brainly.com/question/25550158
#SPJ1
There are 32 students in mr.Ryan’s class. 3/4 of the students have brown hair. The rest have blond hair. How many students have blond hair
Answers
Answer:
8
Step-by-step explanation:
32/4 = 8
8 = 1/4(32)
Answer:
8
Step-by-step explanation:
Since there is 32 students and 3/4 of them have brown hair right. We can change the 3/4 fraction to a similar fraction to help. We can bump it up. As it is similar to 6/8 and 12/16 and 24/32
So 24/32 would have brown hair leaving 8 with blonde
Dos escaladores estan en una pared de escalada. el escalador esta a una altura de 48 pies y desciende a una velocidad de 3 pies por segundo.el escalador b esta en el suelo y asciende a una velocidad de 2,5 pies por segundo.
Answers
The descending climber A and ascending climber B will meet at a height of 40 feet during their respective constant speed descents and ascents.
Climber A is initially at a height of 48 feet and descends at a rate of 3 feet per second. This means that for each second, Climber A's height decreases by 3 feet. The time it takes for Climber A to reach the ground can be calculated by dividing the initial height of 48 feet by the descent rate of 3 feet per second:
Time = Height / Rate = 48 feet / 3 feet per second = 16 seconds.
Therefore, Climber A will take 16 seconds to reach the ground.
Climber B is on the ground and ascends at a rate of 2.5 feet per second. This means that for each second, Climber B's height increases by 2.5 feet. Since Climber A takes 16 seconds to descend, Climber B will also take 16 seconds to reach the same height of 48 feet.
In summary, Climber A will take 16 seconds to descend from a height of 48 feet, while Climber B will take 16 seconds to ascend from the ground to the same height.
learn more about height here
https://brainly.com/question/29131380
#SPJ11
Round 332.749 to:
tens
and
hundredths
Answers
Answer:
To the nearest ten, 330 would be the answer
To the nearest hundredth, the answer would be 332.75
Step-by-step explanation:
I'm not sure if you meant tenths or hundred, but let me know if this is right or wrong :)
use the left-endpoint approximation to approximate the area under the curve of f(x)=x210 1 on the interval [2,5] using n=3 rectangles.
Answers
To approximate the area under the curve of \(f(x) = x^2 + 1\) on the interval [2, 5] using the left-endpoint approximation with n = 3 rectangles, we divide the interval into n subintervals of equal width.
First, we determine the width of each subinterval:
\(\text{Width} = \frac{b - a}{n}\\\\\text{Width} = \frac{5 - 2}{3}\\\\\text{Width} = \frac{3}{3}\\\\\text{Width} = 1\)
Next, we calculate the left endpoint of each subinterval:
Left endpoints: 2, 3, 4
For each subinterval, we evaluate the function at the left endpoint and multiply it by the width to find the area of the rectangle.
Rectangle 1:
Left endpoint: 2
Height: \(f(2) = (2^2 + 1) = 5\)
Area: 5 * 1 = 5
Rectangle 2:
Left endpoint: 3
Height: \(f(3) = (3^2 + 1) = 10\)
Area: 10 * 1 = 10
Rectangle 3:
Left endpoint: 4
Height: \(f(4) = (4^2 + 1) = 17\)
Area: 17 * 1 = 17
Finally, we sum up the areas of all the rectangles to get the total approximate area:
Total approximate area = Area of Rectangle 1 + Area of Rectangle 2 + Area of Rectangle 3
Total approximate area = 5 + 10 + 17
Total approximate area = 32
Therefore, the approximate area under the curve of \(f(x) = x^2 + 1\) on the interval [2, 5] using the left-endpoint approximation with n = 3 rectangles is 32 square units.
To know more about Function visit-
brainly.com/question/31062578
#SPJ11
57 plus what equals 86
Answers
Answer:
29
Step-by-step explanation:
57+x=86
x=86-57
x=29
86-57=29Answer:
Step-by-step explanation:
Evaluate the expression 2n(n-1), when n =
= 3
A 11
B 12
C 17
D 18
Answers
Answer:
The value of the expression is 12 ⇒ B
Step-by-step explanation:
Let us solve the question
∵ The expression is 2n(n - 1)
→ Simplify it at first by multiply 2n by the bracket (n - 1)
∵ 2n(n - 1) = 2n(n) - 2n(1)
∴ 2n(n - 1) = 2n² - 2n
∵ n = 3
→ Substitute n by 3 in the expression to find its value
∴ 2n² - 2n = 2(3)² - 2(3)
∴ 2n² - 2n = 2(9) - 6
∴ 2n² - 2n = 18 - 6
∴ 2n² - 2n = 12 ⇒ at n = 3
∴ The value of the expression is 12
Jackie went to the movie theater where he bought drinks and popcorn bags for his group of friends. Each drink was $4 and a bag of popcorn was $6. He spent a total of $108. He bought a total of 22 items combined. Write a system of equations that could be used to determine the number of drinks and popcorn bags that Jackie bought at the movie theatre. Define the variables used.
Answers
Answer:
x-intercept is 8, meaning if you get 8 candy bars, you can get 0 popcorn bags.
y-intercept is 6, meaning if you get 6 popcorn bags, you can get 0 candy bars. When Jackie had 0 candy bars, she had 6 (y-intercept) bags of popcorn and when Jackie had 0 bags of popcorn, she had 8 (x-intercept) bars of candy.
Step-by-step explanation:
The y-axis represents the amount of popcorn bags she has and the x-axis has the amount of candy bars. When the linear line intercepts with either axis, at least one axis is always at zero.
Find the derivative.
y = tanhâ¹(âx)
Answers
The derivative of the given function is approximately equal to x/((1-x²)^(3/2)(1-x²)).
In calculus, the derivative of a function is a measure of the rate at which the function changes with respect to its input variable. It represents the instantaneous rate of change of the function at a particular point.
To find the derivative of the given function, we can use the chain rule of differentiation. Let's start by expressing y in terms of the natural logarithmic function:
y = tanh⁻¹(√(1-x²))/2ln(e)
Using the chain rule, we have:
dy/dx = [1/(1-√(1-x²)²)] * (-1/2) * [1/ln(e)] * (-2x/((1-x²)^(3/2)))
Simplifying this expression, we get:
dy/dx = x/((1-x²)^(3/2)ln(e(1-x²)))
Now, we can simplify the expression further by using the identity:
ln(e(1-x²)) = 1-x²
Substituting this value in the above expression, we get:
dy/dx = x/((1-x²)^(3/2)(1-x²))
To learn more about derivative click on,
https://brainly.com/question/29020856
#SPJ4
Write the expression in simplest form 2+3a+9a
Answers
Answer: 2+12a
Step-by-step explanation:
add like terms: 3a+9a
What two numbers multiply to 1/4 and add to -1?
Answers
Answer:Are there any options?
Step-by-step explanation:
Two or more expressions with an Equal sign is called as Equation. Two numbers are x =-1/2 and y=-1/2 whose product is 1/4 and which add to -1.
What is Equation?Two or more expressions with an Equal sign is called as Equation.
We need to find two numbers whose product is 1/4 and add to -1.
Let the two numbers are x and y.
By given,
Two numbers multiply to 1/4
xy=1/4
Two numbers add to -1
x+y=-1
Let x=-y-1
Substitute in equation 1.
(-y-1)y=1/4
-y²-y-1/4=0
Multiply negative on both sides
y²+y+1/4=0
Use quadratic formula
b=1,c=1/4,a=1
y=-1±√1-1/2=-1/2
Now substitute y in x+y=-1
x-1/2=-1
x=-1+1/2
x=-1/2
Hence the two numbers are x =-1/2 and y=-1/2 whose product is 1/4 and which add to -1.
To learn more on Equation:
https://brainly.com/question/10413253
#SPJ2
50 PTS & BRAINLIEST! PLEASE HELP ASAP! Add one number to each column of the table so that it shows a function. Do not repeat an ordered pair that is in the table

Answers
The additional ordered pair to form the function (12, 7)
What is function?A function is a relation for which each value from the set the first components of the ordered pairs is associated with exactly one value.
Given that, a table which is showing a function,
We need to find one ordered pair that can shows a function,
According to the definition of the function, we know that each value of x will have a unique value of y,
From the numbers given, the only ordered pair that shows a function is (12, 7)
Hence, the additional ordered pair to form the function (12, 7)
Learn more about functions, click;
https://brainly.com/question/12431044
#SPJ1
Help yalll I really need help major time

Answers
Answer:
Annalise is correct because the outputs are closest when x = 1.35
Step-by-step explanation:
The solution to the equation 1/(x-1) = x² + 1 means the one x value that will make both sides equal. If we look at the table, notice how when x = 1.35, f(x) values are closest to each other for both equations, signifying that x = 1.35 is approximately the solution. Thus, Annalise is correct.
A man runs 2.4 km north and then 1.6 km in a direction 31
∘
east of north. A woman walks directly between the same initial and final points. (a) What distance does the woman walk? km (b) In what direction does the woman walk? (Enter only positive, acute angles.)
Answers
The woman walks a distance of 2.5 km and in a direction of approximately 56.31 degrees counterclockwise from the positive x-axis.
To solve this problem, we can use the fact that the woman walks directly between the same initial and final points as the man, which means that she follows the hypotenuse of a right triangle with legs 2.4 km and 1.6 km, where the second leg makes an angle of 31 degrees east of north.
(a) To find the distance the woman walks, we can use the Pythagorean theorem:
distance =\(\sqrt{((2.4 km)^2 + (1.6 km)^2)} = \sqrt{(6.25 km^2)\)
distance = 2.5 km
Therefore, the woman walks a distance of 2.5 km.
(b) To find the direction the woman walks, we can use trigonometry. Let theta be the angle that the hypotenuse makes with the positive x-axis (east). Then, we have:
tan(\($\theta\)) = (1.6 km) / (2.4 km) = 0.66667
\($\theta\) = tan(0.66667) = 33.69 degrees
Since the woman is walking towards the final point, the direction she walks is the acute angle between the hypotenuse and the positive x-axis, which is 90 - 33.69 = 56.31 degrees counterclockwise from the positive x-axis.
Therefore, the woman walks a distance of 2.5 km and in a direction of approximately 56.31 degrees counterclockwise from the positive x-axis.
Learn more about "Distance and Direction" : https://brainly.com/question/1326450
#SPJ11
The number of miles M that a certain automobile can travel onone gallon of gasoline at a speed of v mi/hr is given by:
M=-(1/30)v^2 + (5/2)v , for v<0<70
a. Find the most economical speed for a trip.
b. Find the largest value of M.
Answers
a. The most economical speed for a trip is v = 35 mph. and b. The largest value of M is M = 87.5 miles.
a. To find the most economical speed for a trip, we need to maximize the value of M, which represents the number of miles the automobile can travel on one gallon of gasoline.
Given equation: M = -(1/30)v² + (5/2)v
Take the derivative of M with respect to v using the power rule for derivatives:
dM/dv = -(2/30)v + (5/2)
Set the derivative equal to 0 and solve for v to find the critical point:
-(2/30)v + (5/2) = 0
-(2/30)v = -(5/2)
v = (5/2) * (30/-2)
v = 35
Since v must be less than 70 according to the given range, the most economical speed for the trip is v = 35 mph.
b. To find the largest value of M, we can substitute the given expression for M into the equation and evaluate it for the given range of v, which is v < 0 < 70.
Given equation: M = -(1/30)v² + (5/2)v
Substitute v = 70 into the equation to find the largest value of M:
M = -(1/30)(70)² + (5/2)(70)
M = -4900/30 + 350/2
M = -163.33 + 175
M = 11.67
Therefore, the largest value of M is M = 87.5 miles. (rounded to two decimal places)
To know more about speed, refer here:
https://brainly.com/question/17661499#
#SPJ11
1.What tool ensures greater accuracy by aligning the graduated scale with the edges or points to be measured
Answers
The tool that ensures greater accuracy by aligning the numbers scale with the edges or points to be measured is a Vernier caliper.
A Vernier caliper is a tool used to accurately measure small lengths, widths, and diameters with precision. It has two parts: an outer frame and a sliding vernier scale. The frame is used to place the object to be measured, while the vernier scale is moved along the frame to measure the object's length.
The graduations on the vernier scale are smaller than the main scale graduations, allowing for more precise measurements to be made. This makes the Vernier caliper a more accurate measuring tool than a standard ruler or tape measure.
To know more about numbers visit :
https://brainly.com/question/24908711
#SPJ11
Please answer my question by the way the number 4 and 5 is FOIL.
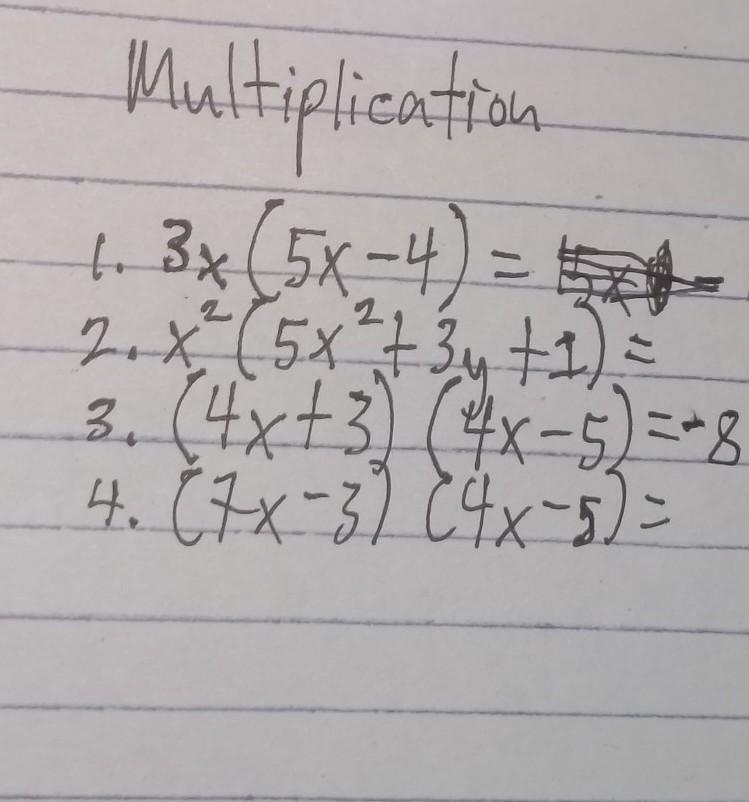
Answers
Using FOIL method for the multiplication, we have:
1: 3x (5x - 4) = 15x² - 12x
2: x²(5x² + 3y + 1) = 5x⁴ + 3x²y + x²
3: (4x + 3)(4x -5) = 16x² - 8x - 15
4: (7x - 3)(4x - 5) = 28x² - 47x + 15
How to carry out multiplication using FOIL?
FOIL is an acronym that stands for "First, Outer, Inner, Last". It is a mnemonic device that is commonly used to remember the process of multiplying two binomials.
No. 1
3x (5x - 4) = 3x*5x + 3x*(-4)
= 15x² - 12x
No. 2
x²(5x² + 3y + 1) = x²*5x² + x²*3y + x²*1
= 5x⁴ + 3x²y + x²
No. 3
(4x + 3)(4x -5) = 4x(4x-5) + 3(4x-5)
= 4x*4x + 4x*(-5) + 3*4x + 3*(-5)
= 16x² - 20x + 12x - 15
= 16x² - 8x - 15
No. 4
(7x - 3)(4x - 5) = 7x(4x - 5) - 3(4x - 5)
= 7x*4x + 7x*(-5) - 3*4x - 3*(-5)
= 28x² - 35x -12x + 15
= 28x² - 47x + 15
Learn more about FOIL method on:
https://brainly.com/question/27980306
#SPJ1
Which is the better deal?
6 cans of soda for $3.60
4 cans of soda for $2.36
10 cans of soda for $5.50
Answers
Answer:
The better deal is 10 cans of soda for $5.50
Step-by-step explanation:
6 cans of soda for $3.60 means each can of soda is worth .60 cents4 cans of soda for $2.36 means each can of soda is worth .59 cents 10 cans of soda for $5.50 means each can of soda is worth .55 centsTherefore, 10 cans of soda for $5.50 is the best deal because you are getting the most cans of soda for the cheapest amount of money.
Hope this helped!
Answer:
10 cans for $5.50
Step-by-step explanation:
3.60/6= $0.60 per can
2.36/4= $0.59 per can
5.50/10= $0.55 per can
What is the Volume of the cylinder, in cubic ft, with a height of 18ft and a base diameter of 10ft? Round to the nearest tenths place.
Answers
if it has a diameter of 10, then its radius is half that, or 5.
\(\textit{volume of a cylinder}\\\\ V=\pi r^2 h~~ \begin{cases} r=radius\\ h=height\\[-0.5em] \hrulefill\\ r=5\\ h=18 \end{cases}\implies V=\pi (5)^2(18)\implies V\approx 1413.7~ft^3\)
I'll give brainliest!!!

Answers
Answer in the picture !!!
A prism is created using 2 regular pentagons as bases. The apothem of each pentagon is 2.8 centimeters.
A regular pentagonal prism is shown. The apothem of each pentagon is 2.8 centimeters. The height of the prism is (2 x + 1). All sides of the pentagon are congruent.
Which expression represents the volume of the prism, in cubic centimeters?
9x2 + 7x
14x2 + 7x
16x2 + 14x
28x2 + 14x
Answers
the expression that represents the volume of the prism is 14x² + 7x cubic centimeters. Option 2
What is the volume of a pentagonal prism?The volume of a pentagonal prism is determined using the formula;
V = 5/2abh
where
a is the apothemh is the height of the prismb is the base of the prismNow, let's substitute the values given
Let the base be 'x'
The apothem is 2. 8 centimeters
height is 2x + 1
Volume = 5/ 2 × 2. 8 × x × (2x + 1)
Volume = 14/ 2 × x(2x + 1)
Volume = 7x(2x + 1)
Volume = 14x² + 7x
Thus, the expression that represents the volume of the prism is 14x² + 7x cubic centimeters. Option 2
Learn more about a pentagonal prism here:
https://brainly.com/question/27741277
#SPJ1
Can someone help me?

Answers
Answer:
no
Step-by-step explanation:
Answer:
The concentration in both solutions is the same.
Step-by-step explanation:
Equilibrium> EQUAL
DUE SOON HELP ASAP
The TV measures 39 inches tall, and the length of its diagonal is 80 inches. What is the width of the TV? Round your answer to the nearest tenth and label your answer.
Your answer:
Answers
We're assuming the TV is a rectangle, meaning all corners have right angles.
From the given information, we have a right triangle with a height of 39 inches, hypotenuse, of 80 inches, and an unknown base width.
Using Pythagorean's theorem:
\(a^2+b^2=c^2\)
\(b=\sqrt{c^2-a^2}\)
c = 80 and a = 39
\(b=\sqrt{80^2-39^2}\)
\(=\sqrt{4879}\)
Using a calculator and rounding to the nearest tenth gives
\(\approx 69.8 \text{ inches}\)
Let me know if you need any clarifications, thanks!
Find the measure of Angle A. (include and explanation so I could do the rest on my own, preferably :)

Answers
Answer:
13) Angle A is 30°
14) Angle A is 45°
15) Angle A is 40°
16) Angle A is 40.5°
Step-by-step explanation:
By the angle sum theorem for the interior angles of a triangle, we have;
13) 130° + 2·x + 3·x = 180°
∴ 2·x + 3·x = 180° - 130° = 50°
2·x + 3·x = 5·x = 50°
x = 50°/5 = 10°
∠A = 3·x = 3 × 10° = 30°
∠A = 30°
14) 3·x + 9 + 4·x + 9 + 78° = 180°
7·x + 18 + 78° = 180°
7·x = 180° - (18 + 78)° = 180° - 96° = 84°
x = 84°/7 = 12°
∠A = 3·x + 9 = 3 × 12° + 9 = 45°
∠A = 45°
15) 90° + x + 51 + x + 61 = 180°
∴ x + 51 + x + 61 = 180° - 90° = 90°
2·x + 112 = 90°
2·x = (90 - 112)° = -22°
x = -22°/2 = -11°
x = -11°
∠A = x + 51 = -11° + 51 = 40°
∠A = 40°
16) x + 79 + x + 49 + 70° = 180°
x + x = (180 - 70 - 79 - 48)° = -17°
2·x = -17°
x = -17°/2 = -8.5°
x = -8.5°
∠A = x + 49 = (-8.5 + 49)° = 40.5°
∠A = 40.5°.
(sec A + tan A) (1 - sin A) = cos A prove
Answers
Answer:
(identity has been verified)
Step-by-step explanation:
Verify the following identity:
(sec(A) + tan(A)) (1 - sin(A)) = cos(A)
Write secant as 1/cosine and tangent as sine/cosine:
(1 - sin(A)) (1/cos(A) + sin(A)/cos(A)) = ^?cos(A)
Put 1/cos(A) + sin(A)/cos(A) over the common denominator cos(A): 1/cos(A) + sin(A)/cos(A) = (sin(A) + 1)/cos(A):
(sin(A) + 1)/cos(A) (1 - sin(A)) = ^?cos(A)
Multiply both sides by cos(A):
(1 - sin(A)) (sin(A) + 1) = ^?cos(A)^2
(1 - sin(A)) (sin(A) + 1) = 1 - sin(A)^2:
1 - sin(A)^2 = ^?cos(A)^2
cos(A)^2 = 1 - sin(A)^2:
1 - sin(A)^2 = ^?1 - sin(A)^2
The left hand side and right hand side are identical:
Answer: (identity has been verified)
●✴︎✴︎✴︎✴︎✴︎✴︎✴︎✴︎❀✴︎✴︎✴︎✴︎✴︎✴︎✴︎✴︎✴︎●
Hi my lil bunny!
❧⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯☙
Let's do this step by step.
Prove that sec A ( 1 - sin A) ( sec A + tan A) = 1
Solving L.H.S
sec A ( 1 - sin A) ( sec A + tan A)
\(= \frac{1}{cos A} ( 1 - sin A ) ( \frac{1}{cos A} + \frac{sin A }{cos A})\)
\(= \frac{(1 - sin A)}{cos A} ( \frac{1 + sin A }{cos A })\)\(= \frac{( 1 - sin A)( 1 + sin A)}{cos A X cos A}\)
We know that \(( a - b) ( a + b) = a^2 - b^2\)
\(= \frac{( 1^2 - sin^2 A)}{cos^2 A}\)
\(= \frac{( 1 - sin^2 A)}{cos^2 A}\)
\(= \frac{cos^2 A}{cos^2 A}\) \(| cos^2 A + sin^2 A = 1 | cos^2 A = 1 - sin^2 A |\)\(1 - sin^2 A = cos^2 A\)
\(= 1\)
\(= R . H. S\)
Thus, L.H.S = R.H.S
Hence proved.
❧⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯⎯☙
●✴︎✴︎✴︎✴︎✴︎✴︎✴︎✴︎❀✴︎✴︎✴︎✴︎✴︎✴︎✴︎✴︎✴︎●
Hope this helped you.
Could you maybe give brainliest..?
❀*May*❀
Which of the following intervals corresponds to the smallest area under a Normal curve?
a. Q1 to Q3
b. μ to μ + 3σ
c. Q1 to μ + 2σ
d. μ - σ to Q3
Answers
The interval μ - σ to Q3 corresponds to the smallest area under a normal curve because it includes only a small portion of the data set.
The answer to this question is option D, which is μ - σ to Q3. To understand why this is the correct answer, we need to first understand what each of the intervals represents. Q1 and Q3 are the first and third quartiles of the data set, respectively. μ is the mean of the data set, and σ is the standard deviation.
When we look at the interval μ - σ to Q3, we can see that it includes the upper quartile and some of the data points to the left of it. This means that the area under the normal curve within this interval will be relatively small compared to the other options.
On the other hand, option B includes the mean and a larger range of data points, which would result in a larger area under the curve. Option C includes Q1 and a larger range of data points, which would also result in a larger area. Option A includes both Q1 and Q3, which cover the majority of the data set and would therefore have the largest area under the curve.
In summary, the interval μ - σ to Q3 corresponds to the smallest area under a normal curve because it includes only a small portion of the data set.
Visit here to learn more about normal curve:
brainly.com/question/31391220
#SPJ11
Please help :)
A car drove 249.48 miles on 12.6 gallons of gas. How far could the car drive on a full tank of 14.8 gallons of gas? Drag and drop a number to correctly complete the statement The car could drive miles on a full tank of gas 212.39 286.57 293 04 333 76

Answers
Answer:
293.04
Step-by-step explanation:
The one above me is worng sorry i just took the test :p

The ans is 293.04
Thank you have a wonderful day ahead...