Four circles, each with a radius of 2 inches, are
removed from a square. What is the remaining area of
the square?
(16 – 4pi) in.2
(16 – pi) in.2
(64 – 16pi) in.2
(64 – 4pi) in.?

No, 9 erasers in each box are not possible.

An expression is a way of writing a statement with more than two variables or numbers with operations such as addition, subtraction, multiplication, and division.

Example: 2 + 3x + 4y = 7 is an expression.

We have,

Number of erasers = 50

Number of box = 6

Number of erases in each box.

= 50/6

= 8.33

= 8

So,

9 erasers in each box are not possible.

Thus,

9 erasers in each box are not possible.

Learn more about expressions here:

https://brainly.com/question/3118662

#SPJ1

Answer: 913,256

6-ones place

5-tens place

2-hundreds place

3- thousands place

1-ten thousands place

9-hundred thousands place

Soooo ur answer is 1, 1 is in the ten thousands place.

Answer: the ten-thousands place is the 5th digit from the left. This digit is 1

Step-by-step explanation:

Answer:

No

Step-by-step explanation:

Answer:

i think it is a but i am not shore. Hope this helps

Step-by-step explanation:

Answer:

0

Step-by-step explanation:

x < 4 means all numbers less than 4, excluding 4 itself.

4.5 > 4.

5 > 4.

so 0 is the solution

Answer:

4

Step-by-step explanation:

interval notation ( negative infinity, 4)

The fraction that Roberto is thinking of is \(\mathbf{\dfrac{x}{2+x} = \dfrac{3}{9}}\) and the value of x = 1

A fraction is that part of a whole number that is used to express figures into two parts which are the numerator(upper part) and the denominator(lower part) and these two parts are separated by a division line.

From the given information, we have:

\(\mathbf{\dfrac{x}{2+x} = \dfrac{3}{9}}\)

Cross multiply

9x = 3(2+x)

9x = 6 + 3x

9x - 3x = 6

6x = 6

x = 6/6

x = 1

Learn more about fractions here:

https://brainly.com/question/78672

Answer:

no se

perdon

Step-by-step explanation:

Answer:

Arithmetic Sequence

Step-by-step explanation:

Using the cross product for the proff of Pythagoras theorem, the correct step is

By the cross product property, AB² =  BC multiplied by BD

The cross product property is typically used to solve equations with two values, where the product of the extremes (the outer terms) is equal to the product of the means (the inner terms).

For the similar triangles, the ratio is as follows

BD / BA = BA / BC

BA² = BD * BC

and AB = BA, hence

BA² = BD * BC

Learn more about cross product property at

https://brainly.com/question/14308624

#SPJ1

When substituting y = 11 into the equation y = 1/2(x) - 25, we find that x = 72, confirming that (23.5, 11) is a valid point of intersection.

Given that x is 23.5, it is required to prove that it is an intersection point for the equation y = 1/2(x) - 25 when y is equal to 11.

The equation is given as y = 1/2(x) - 25

When y = 11, we can substitute the value of y in the equation to obtain 11 = 1/2(x) - 25

This can be simplified as 11 + 25 = 1/2(x)36 = 1/2(x)

On solving, x = 72Thus, when y is equal to 11 and x is equal to 72, the given point of intersection is valid.

Therefore, it can be concluded that x = 23.5 is a point of intersection for the equation y = 1/2(x) - 25 when y is equal to 11.

In summary, when given an equation with two variables, we can find the point of intersection by setting one of the variables to a given value and solving for the other variable. In this case, when y is equal to 11, we can solve for x and obtain the point of intersection as (72,11).

For more questions on intersection

https://brainly.com/question/30429663

#SPJ8

Answer:

386.75

Step-by-step explanation:

added in the picture

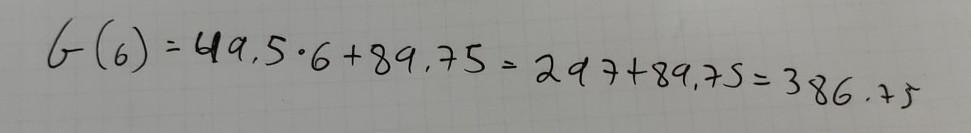
The cost of joining the gym for 6 months is 386.75.

Given,

The function G(m) = 49.5m + 89.75 represents the cost of joining the gym in addition to the one-time membership fee.

The cost, G, is measured in dollars for m months.

We need to find the value of G(6).​

Here,

The one-time membership = 89.75.

Now,

Putting m = 6 months in:

G(m) = 49.5m + 89.75

G(6) = 49.5 x 6 + 89.75

       = 297 + 89.75

       = 386.75

Thus the cost of joining the gym for 6 months is G(6) = 386.75.

Learn more about finding the value of a function here:

https://brainly.com/question/8822000

#SPJ2

The question is incomplete. Here is the complete question.

Find the measurements (the lenght L and the width W) of an inscribed rectangle under the line y = -\(\frac{3}{4}\)x + 3 with the 1st quadrant of the x & y coordinate system such that the area is maximum. Also, find that maximum area. To get full credit, you must draw the picture of the problem and label the length and the width in terms of x and y.

Answer: L = 1; W = 9/4; A = 2.25;

Step-by-step explanation: The rectangle is under a straight line. Area of a rectangle is given by A = L*W. To determine the maximum area:

A = x.y

A = x(-\(\frac{3}{4}.x + 3\))

A = -\(\frac{3}{4}.x^{2} + 3x\)

To maximize, we have to differentiate the equation:

\(\frac{dA}{dx}\) = \(\frac{d}{dx}\)(-\(\frac{3}{4}.x^{2} + 3x\))

\(\frac{dA}{dx}\) = -3x + 3

The critical point is:

\(\frac{dA}{dx}\) = 0

-3x + 3 = 0

x = 1

Substituing:

y = -\(\frac{3}{4}\)x + 3

y = -\(\frac{3}{4}\).1 + 3

y = 9/4

So, the measurements are x = L = 1 and y = W = 9/4

The maximum area is:

A = 1 . 9/4

A = 9/4

A = 2.25

Check the picture below.

Make sure your calculator is in Degree mode.

\(\cos(25^o )=\cfrac{\stackrel{adjacent}{x}}{\underset{hypotenuse}{12}}\implies 12\cos(25^o)=x\implies \boxed{10.876\approx x} \\\\[-0.35em] ~\dotfill\\\\ \sin(25^o )=\cfrac{\stackrel{opposite}{z}}{\underset{hypotenuse}{12}}\implies 12\sin(25^o)=z \\\\[-0.35em] ~\dotfill\\\\ \sin(50^o )=\cfrac{\stackrel{opposite}{z}}{\underset{hypotenuse}{y}}\implies y=\cfrac{z}{\sin(50^o)}\implies y=\cfrac{12\sin(25^o)}{\sin(50^o)}\implies \boxed{y\approx 6.62}\)

Answer:

A and C and E

Step-by-step explanation:

Answer:

Step-by-step explanation:

A)correct

B)incorrect

C)incorrect

D)incorrect

F)correct

Answer :

1619kg 750g

Explanation :

mass of wheat in the morning : 2510kg 350g

mass of sold out wheat during: 890kg 600g

the day

mass of wheat left in the shop: 1619kg 750g

in the evening

Dylan should choose Ball B which having lowest density.

What is volume of spere?

The volume of a sphere is calculated using the formula volume = 4/3πr³ where r is the sphere's radius.

Given that:

Ball A = diameter 7cm, mass 1.742kg

Ball B = diameter 6cm, mass 1.040kg

As we know that,

volume of a sphere =4/3πr³

1) For Ball A,

volume of a Ball A = 4/3π(3.5)³

volume of a Ball A = 179.6 cm³

given mass for Ball A is 1.742 kg = 1742 g.

So, Density = \(\frac{mass}{volume}\)

     Density of Ball A will be 9.7 g/cm³.

2) For Ball B,

volume of a Ball B = 4/3π(3)³

volume of a Ball B = 113.11 cm³

given mass for Ball B is 1.040 kg = 1O40 g.

So, Density = \(\frac{mass}{volume}\)

     Density of Ball B will be 9.19 g/cm³.

By comparing density of both balls,

Density of Ball A > Density of Ball B

Hence, Dylan should choose Ball B which having lowest density.

To know more about Volume of sphere visit,

https://brainly.com/question/22807400

#SPJ1

Check the picture below.

The value of f(x) at x=4 is 2.

A function is an expression, rule, or law in mathematics that describes a relationship between one variable (the independent variable) and another variable (the dependent variable). Functions are common in mathematics and are required for the formulation of physical relationships in the sciences.

We have to find the value of function for x=4.

This means that from the figure we have to find the value of y when x= 4.

Now, looking into the figure we have

f(4)= 2

f(0)= -2

f(-1)= -1

f(3)= 4

So, the required value is 2.

Learn more about Function here:

https://brainly.com/question/12431044

#SPJ7

Answer:

In order to find the values of k and m in a parallelogram, you would need to have additional information such as the coordinates of the points that make up the parallelogram and the formulas or equations that relate k and m to these points.

Here is one way to find the values of k and m in a parallelogram:

Label the four vertices of the parallelogram as A, B, C, and D with the opposite sides parallel to each other.

Find the slope of the line segment AB by using the formula (y2-y1)/(x2-x1), where (x1, y1) and (x2, y2) are the coordinates of the two points.

Let the slope of AB be represented by k.

Find the slope of the line segment AD by using the same formula and let it be represented by m.

The values of k and m are now found.

Please note that, this method is only applicable if the Parallelogram is not degenerate i.e, it is not a rectangle or a line.

It is also important to keep in mind that different formulas or equations may be used depending on the specific context of the problem. It is also important to check that the slopes of opposite sides are equal.

Step-by-step explanation:

I think you might be referring to the definite integral,

\(\displaystyle \int_{-1}^2|x-1|\,\mathrm dx\)

Recall the definition of absolute value:

\(|x| = \begin{cases}x&\text{if }x\ge0\\-x&\text{if }x<0\end{cases}\)

Then \(|x-1|=x-1\) if \(x\ge1\), and \(|x-1|=1-x\) is \(x<1\). So spliting up the integral at x = 1, we have

\(\displaystyle \int_{-1}^2|x-1|\,\mathrm dx = \int_{-1}^1(1-x)\,\mathrm dx + \int_1^2(x-1)\,\mathrm dx\)

The rest is simple:

\(\displaystyle \int_{-1}^2|x-1|\,\mathrm dx = \left(x-\dfrac{x^2}2\right)\bigg|_{-1}^1 + \left(\dfrac{x^2}2-x\right)\bigg|_1^2 \\\\ = \left(\left(1-\frac12\right)-\left(-1-\frac12\right)\right) + \left(\left(2-2\right)-\left(\frac12-1\right)\right) \\\\ = \boxed{\frac52}\)

The parabola x = √(y - 9) opens upwards.The given parabolic equation is x = √(y - 9). Let's identify the direction of opening of this parabola.The general form of the equation of a parabola is y = a(x - h)² + k.

Comparing this to the given equation, we can see that h = 0 and k = 9. The vertex is therefore (h, k) = (0, 9). Now, let's determine whether the parabola opens upwards or downwards.

If the coefficient of (x - h)² is positive, the parabola opens upwards, and if it's negative, the parabola opens downwards. In this case, since the coefficient of (x - h)² is 1, which is positive, the parabola opens upwards.

Therefore, the parabola x = √(y - 9) opens upwards.

For more question on equation

https://brainly.com/question/29174899

#SPJ8

Presumably, both δ and ρ represent density of some object. Considering the context, ρ is likely a function of 3 variables, ρ = ρ(x, y, z). Then the mass of the sphere with the prescribed density δ(x, y, z) = k ρ(x, y, z) is

\(\displaystyle \iiint_B k \rho(x,y,z) \, dx\, dy\, dz\)

where B is the set

\(B = \left\{(x,y,z) ~ : ~ x^2+y^2+z^2 \le 7^2\right\}\)

If you have all the details at hand, you can compute the integral by converting to spherical coordinates, substituting

\(\begin{cases}x = u \cos(v) \sin(w) \\ y = u \sin(v) \sin(w) \\ z = u \cos(w)\end{cases} \text{ and } dx\,dy\,dz = u^2 \sin(w) \, du \, dv \, dw\)

The integral then transforms to

\(\displaystyle k \int_0^{2\pi} \int_0^\pi \int_0^7 \rho(u\cos(v)\sin(w),u\sin(v)\sin(w),u\cos(w)) \, u^2 \sin(w) \, du \, dw \, dv\)

Without any additional information, there's not much more to say...

Answer:

x = 90

y = 30°

∠ABC = 75°

Step-by-step explanation:

               ΔDAB is an isosceles triangle.

         ⇒ ∠ADB ≅ ∠ABD = y

In ΔABD ,

     120 + y + y = 180 {Angle sum property of triangle}

            120 + 2y = 180

                     2y = 180 - 120

                     2y = 60

                       y = 60 ÷ 2

                       \(\sf \boxed{y = 30^\circ}\)

ΔDCB is an isosceles triangle.

      ∠CDB ≅ ∠CBD = 45

    x + 45 + 45 = 180

              x + 90 = 180

                       x = 180 - 90

                       \(\sf \boxed{x = 90^\circ}\)

∠ABC = ∠ABD + ∠DBC

          = 30 + 45

           = 75°

The parameters that are needed to calculate a probability are listed as follows:

Then the probability is calculated as the division of the number of desired outcomes by the number of total outcomes, hence it is the same as a relative frequency.

The total number of periods is given as follows:

5 + 6 + 4 = 15.

The frequency of each class is given as follows:

Learn more about the concept of probability at https://brainly.com/question/24756209

#SPJ1

If he wants an average of 84, he needs to get at least 93 points.

Remember that the average value between 3 values A, B, and C is:

(A + B + C)/3

Here we know that the first two scores are 76 and 83 points, let's say that the third score is x, if we want to have an average of 84 or more, then we need to solve:

(76 + 83 + x)/3 = 84

159 + x = 252

x = 252 - 159

x = 93

So he needs to get at least 93 points in the next exam.

Learn more about averages at:

https://brainly.com/question/20118982

#SPJ1

The slope of the inequality y > x - 3 is 1

The slope of a line is the ratio of the amount that y increases as x increases some amount

To change the inequality y > x - 3 into slope,

substract x from both sides of the inequality

y- x > x - 3-x

= y-x>-3

= y<-3+x

From the inequality y<-3+x the co-efficient of x is 1

Hence, The slope of the inequality is 1

Learn more about the slope of an inequality at: https://brainly.com/question/24256400

#SPJ1

The width cannot be negative, the width of the walkway is 6 feet. The total area of the garden and the walkway is given as 648 square feet. We know the area of the garden is length multiplied by width, which in this case is 12 feet by 15 feet, so the garden area is\(12 \times 15 = 180\) square feet.

To find the area of the walkway, we subtract the garden area from the total area. Therefore, the area of the walkway is 648 - 180 = 468 square feet.

The walkway surrounds the garden on all sides, so its length and width will be greater than the corresponding dimensions of the garden.

To calculate the width of the walkway, we can use the formula for the area of a rectangle, which is length multiplied by width. In this case, the length is 12 + 2x (twice the width of the walkway) and the width is 15 + 2x.

So, we have the equation\((12 + 2x) \times (15 + 2x) = 468\).

Expanding and rearranging the equation, we get\(4x^2 + 54x - 228 = 0.\)

Solving this quadratic equation using factoring, completing the square, or the quadratic formula, we find that x = 6 or x = -9/2.

For more such questions area

https://brainly.com/question/25292087

#SPJ8

The range, in centimetres (cm), of the following lengths is 20.5 cm

What is the range?

The difference between the lowest and highest numbers is referred to as the range. For instance, the range will be 10 - 2 = 8 if the given data set is 2, 5, 8, 10, and 3.

As a result, the range may alternatively be thought of as the distance between the highest and lowest observation. The range of observation is the name given to the outcome. Statistics' range reflects the variety of observations.

Given, 15 cm, 0.5 cm, 10.3 cm, 16.7 cm, 21 cm, 8.6 cm

So, the highest value of length = 21 cm

the lowest value of length = 0.5 cm

Then, range = the highest value - the lowest value

                    = 21 - 0.5 = 20.5 cm

Hence, the range, in centimetres (cm), of the following lengths is 20.5 cm

To learn more about range

https://brainly.com/question/29794774

#SPJ1

Answer:

The 98% confidence interval for the true proportion of women shoppers who shop on impulse is  

              \(0.1391 < p < 0.2009\)

Step-by-step explanation:

From the question we are  told that

     The  sample size is n = 800

      The sample  proportion is  \(\r p = 0.17\)

Given that the confidence level is  98%  

The level of significance is evaluated as

      \(\alpha = 100 -98\)

     \(\alpha = 2\)%

      \(\alpha = 0.02\)

given that this is a two tailed test

    \(\frac{\alpha }{2} = \frac{0.02}{2} = 0.01\)

The critical values obtained from the normal distribution table is  

   \(z_{\frac{\alpha }{2} } = 2.33\)  

Now the the margin of error is mathematically evaluated as

         \(MOE = 2.33 * \sqrt{\frac{0.17 (1-0.17)}{800} }\)

          \(MOE = 0.0309\)

the 98% confidence interval for the true proportion of women shoppers who shop on impulse is mathematically evaluated as

      \(0.17 - 0.0309 < p < 0.17 + 0.0309\)

       \(0.1391 < p < 0.2009\)

The 98% confidence interval for the true proportion of women shoppers will be:

"0.1391 < p < 0.2009".

According to the question,

Sample size, n = 800

Sample proportion, \(\hat p\) = 0.17

Confidence level = 98%

Now,

The level of significance will be;

→ α = 100 - 98

      = 2% or,

      = 0.02

Two-tailed be:

→ \(\frac{\alpha}{2}\) = \(\frac{0.02}{2}\)

     = 0.01

The critical value be:

\(z_{\frac{\alpha}{2} }\) = 2.33

then, The margin of error be:

= 2.33 × \(\sqrt{\frac{0.17(1-0.17)}{800} }\)

= 2.33 × \(\sqrt{\frac{0.17\times 0.83}{800} }\)

= 2.33 × \(\sqrt{\frac{0.1411}{800} }\)

= 0.0309

hence,

The 98% confidence level be:

= 0.17 - 0.0309 < p < 0.17 < 0.0309

= 0.1391 < p < 0.2009

Thus the above approach is correct.

Find out more information about critical value here:

https://brainly.com/question/16112320

Answer:

5/33

Step-by-step explanation:

Probability=10/66

P=5/33

Answer:

5/33

Step-by-step explanation:

Remove the parentheses and combine the like terms:

7 - -9 = 7+ 9 = 16

6i - -8i = 6i +8i = 14i

The answer is 16 + 14i