I need quick help with this!! 15 PTS POSSIBLE!!! i know for sure its not 3/50, but i think its 3/20? and explain your reasoning.

Answers
Answer:
3/20
Step-by-step explanation:
Given:
Probability that it is Monday and that a student is absent is 3/100
Probability that a randomly selected day of the school week is monday is 1/5
To Find:
What is the probability that a student is absent given that today is Monday.
Solve:
It may be different if it were on a Wednesday. Because we expect fewer absentees on Wednesday than on Monday/ Friday. In these cases the conditional probability, P(absent/weekday) would differ depending on the day of the week.
3/100 ÷ 1/5 = 3/20
The probability that a student is absent on a Monday is 3/20
[RevyBreeze]
Related Questions
please help i’m unsure how to do this

Answers
Answer:
5
Step-by-step explanation:
theres 5 gaps. just count
Answer: OPTION A. 5
Step-by-step explanation:
The value of MN is the distance between M and N.
Distance formula on number line: | value 1-value 2 |Since distance is always positive we use absolute value. This means, that in contents of this question, M-N or N-M does not make any difference in result.
M=-3
N=2
-------------------------------------------------------------
| value 1-value2 |
=| M-N |
=| -3-2 |
=| -5 |
=5
--------------------------------------------------------------
As a matter of fact, it is a number line, so you can just count the number of gaps in between.Which of the following best described the line that is passing through the ordered pairs given below? Select all that apply
(-4, 6) & (-4, 1)

Answers
The slope of the line is undefined.
What is Equation of line?The equation of line in point-slope form passing through the points
(x₁ , y₁) and (x₂, y₂) with slope m is defined as;
⇒ y - y₁ = m (x - x₁)
Where, m = (y₂ - y₁) / (x₂ - x₁)
Given that;
Two points on the line are (-4, 6) and (-4, 1)
Now,
Since, The equation of line passes through the points (-4, 6) and (-4, 1)
So, We need to find the slope of the line.
Hence, Slope of the line is,
m = (y₂ - y₁) / (x₂ - x₁)
m = (1 - 6) / (-4 - (-3))
m = - 5 / 0
m = ∞
Thus, The slope of the line is undefined.
Learn more about the equation of line visit:
https://brainly.com/question/18831322
#SPJ1
What fraction form have 6 letters
Answers
Answer:
tenth
Step-by-step explanation:
the side lengths of a triangle are 5, 3 and 4. is this a right triangle?
A. Yes, because 3 ^2 + 4^2 = 5^2
B. No, because 3^2 + 4^2 not equal
C. yes, because 3^2 + 4^2 > 5^2
D. No, because 3 + 4 not equal to 5
Answers
Answer:
A. Yes, because 3 ^2 + 4^2 = 5^2
Step-by-step explanation:
A. Yes, because 3 ^2 + 4^2 = 5^2
find all values of x such that (6, x, −11) and (5, x, x) are orthogonal. (enter your answers as a comma-separated list.)x = ___
Answers
The values x such that (6, x, −11) and (5, x, x) are orthogonal is 6,5.
The orthogonal vectors have dot product to be zero. Thus, the formula to be used is -
a . b = a1b1 + a2b2 + a3b3, where a1, a2 and a3 are components a vector and b1, b2 and be are components of b vector.
Keep the values in formula -
a . b = 6(5) + x² + (-11)x
a . b = 30 + x² - 11x = 0
So, x² - 11x + 30 = 0
x(x - 6) - 5(x - 6) = 0
(x - 6) (x - 5) = 0
So, the value of x is 6,5.
Learn more about orthogonal vector -
https://brainly.com/question/15587050
#SPJ4
How do you solve inequality questions?
Answers
An inequality like the statement, x + 3 > 5, can be solved by finding the value of the variable, "x", which is x > 2.
How to Solve an Inequality Question?Inequalities are used to express relationships between quantities or values that are not equal. They can be used to describe constraints or limitations on a solution to a problem, or to represent a range of possible values.
Inequality can also be used to describe relationships between variables in algebraic expressions or equations.
To solve an inequality, we need to isolate the variable in the given statement to determine the value of the variable.
For example, given the inequality, x + 3 > 5, in order to solve this inequality, we need to find the value of x by subtracting 3 from both sides:
x + 3 - 3 > 5 - 3
x > 2.
Learn more about solving inequality on:
https://brainly.com/question/24372553
#SPJ1
Please help asap!! I'll give you brainliest!!
What is the slope of the function, represented by the table of values below?

Answers
Answer:
-4
Step-by-step explanation:
To find the slope, you can use the formula y2-y1/x2-x1. Plugin any set of values. Say 13 is y2, and 5 is y1. 13-5=8. We now have 8/x2-x1. You have to use the x-values of the y-values that you already used. -2 is x2 and 0 is x1. -2-0=-2. 8/-2 = -4.
Bro please help ima fail


Answers
In the picture there are 10 question.
1. Identify the 1st 10 perfect squares?
The 1st 10 perfect squares are 1,4,9,16,25,36,49,64,81 and 100.
\(1^{2} =1\\2^{2} =4\\3^{2} =9\\4^{2} =16\\5^{2} =25\\6^{2} =36\\7^{2} =49\\8^{2} =64\\9^{2} =81\\10^{2} =100\\\)
2. What does it mean for a number to a perfect square? provide an example.
By squaring a whole number or an integer, one can create perfect squares. Let's examine an illustration to get the idea behind perfect squares. We can use a set of four and a second set of six marbles for this. Let's set the marbles in order.
See the figure(1).
With four marbles, we can create a square with two rows and two marbles in each row. We can create a rectangle with 6 marbles that has 2 rows with 3 marbles in each row. It signifies that 4 = 2\(\times\)2 and 6 = 3\(\times\)2 mathematically. Let's concentrate just on the square-shaped numerals. Here, 4 = 2 × 2 = \(2^{2}\).
Square A is smaller then Square B. Square B is smaller then Square C.
The 3 Square side length are3,4.2 and\(5\frac{1}{2}\).
complete the equation each of the square shown.
3. Square A=Area=\(3^{2}\)=9
4. Square B= Area=\(4.2^{2}\)=17.64
5. Square C=Area= \(\frac{5}{2} =\frac{25}{4}\)
Find the length of the side of the square for each area is given
6.81 square inches
Area=81=\(s^{2}\)
s=9
7. \(\frac{4}{25} cm\)
area=\(\frac{4}{25} cm\)=\(s^{2}\)
s=\(\frac{2}{5}\)
8.0.49 Square unit
area=0.49=\(s^{2}\)
s=0.7
The graph represent the equation \(y=x^{2}\).
9.complete the table of values to find corresponding y-values.
if x =-1.4
Substituting in the equation \(y=x^{2}\)
Then y=\(-1.4^{2}\)
y=1.96.
Therefore, (x,y)=(-1.4,1.96)
if x =-1
Substituting in the equation \(y=x^{2}\)
Then y=\(-1^{2}\)
y=1.
Therefore, (x,y)=(-1,1)
if x =0
Substituting in the equation \(y=x^{2}\)
Then y=\(0^{2}\)
y=0.
Therefore, (x,y)=(0,0)
if x =\(\frac{2}{5}\)
Substituting in the equation \(y=x^{2}\)
Then y=\((\frac{2}{5}) ^{2}\)
y=\(\frac{4}{25}\).
Therefore, (x,y)=(\(\frac{2}{5}\),\(\frac{4}{25}\))
if x =2
Substituting in the equation \(y=x^{2}\)
Then y=\(2^{2}\)
y=4.
Therefore, (x,y)=(2,4)
10. why the graph of equation \(y=x^{2}\) is above x-axis.
Because the \(y=x^{2}\\\) the x values are always positive because x as a square. So, the equation is always on x- axis or above x-axis.
To learn more about squares visit: https://brainly.com/question/14198272
#SPJ9

- 1 2/7x-1=26
Show work
Answers
The expression \($1\frac{2}{7}x -1=26\) gives {x} value as equivalent to 21.
What is expression?In mathematics, an expression or mathematical expression is a finite combination of symbols that is well-formed according to rules that depend on the context.
Mathematical symbols can designate numbers (constants), variables, operations, functions, brackets, punctuation, and grouping to help determine order of operations and other aspects of logical syntax.
Given is the expression as -
\($1\frac{2}{7}x -1=26\)
We can write the expression as -
(9/7)x - 1 = 26
(9/7)x = 27
x = (27 x 7)/9
x = 3 x 7
x = 21
Therefore, the expression \($1\frac{2}{7}x -1=26\) gives {x} value as equivalent to 21.
To solve more questions on expressions, visit the link below -
brainly.com/question/1041084
#SPJ1
Help plz i hate this but everytime I do this I get it worng

Answers
Answer:
The answer is 19.25
Step-by-step explanation:
Key skills needed: Fraction operations, decimal conversion
1) We are given the hint to use 9 as "b", and we get:
---> \(a = \frac{16}{8}(9) + \frac{10}{8}\)
Let's do \(\frac{16}{8}(9)\)
--> \(\frac{16}{8}\) is the same as \(16\) ÷ \(8\)
16 divide by 8 is 2.
2) This means that it would be ---> \(2(9)\) which is simply 2 times 9.
2 times 9 = 9 + 9 = 18
3) With that result, it would be ---> \(a = 18 + \frac{10}{8}\)
Now, do \(\frac{10}{8}\) (10 divided by 8) ---> Get 1.25
4) 18 + 1.25 = 19.25, so --> \(a = 19.25\) is the answer
Hope you understood and have a nice day!! :D
PLS HELP 30 POINTS
simplify
(2√2)(3√3)
pls show your work!!!!
Answers
Answer: 6√6
Multiply the numbers outside of the square root and then multiply the numbers inside of the square root.
:)
Step-by-step explanation:
(2√2)(3√3)
= 2×3 (√2×√3)
= 6√6
giving brainliest!!! ill help on whatever you need!!

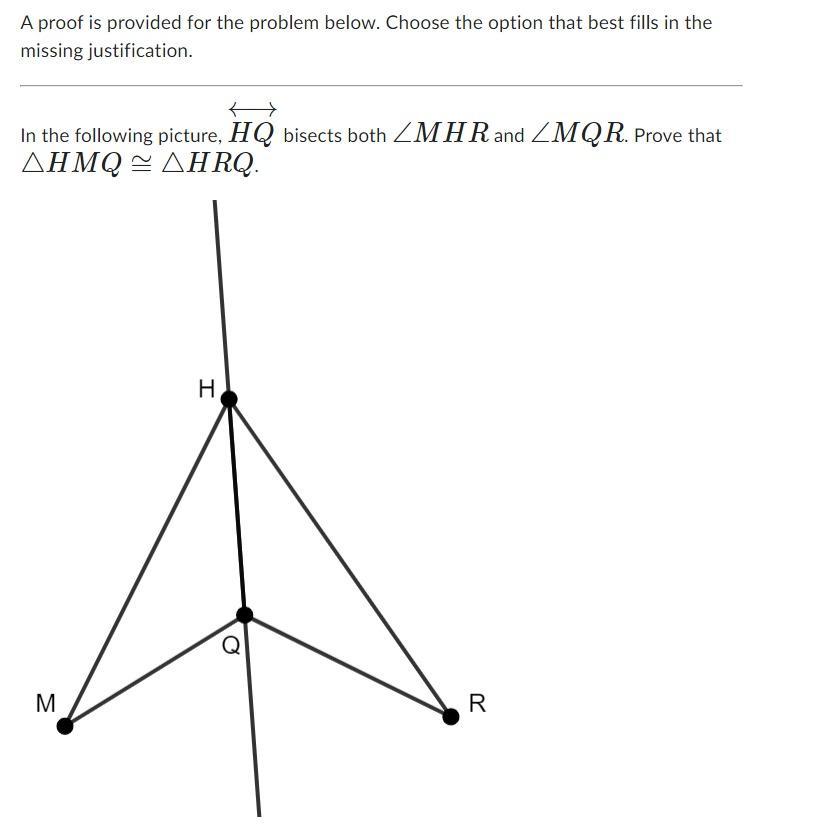
Answers
Answer:
ASA
Step-by-step explanation:
Given: HQ bisects both ∠MHR and ∠MQR Prove: △HMQ ≅ △HRQ
Statement Reason
HQ bisects both ∠MHR and ∠MQR | Given
∠MHQ = ∠HRQ and ∠MQH = ∠RQH | Definition of angle bisector
HQ = HQ | Reflexive property of equality
△HMQ ≅ △HRQ | AAS rule
6x - 2 (x + 2) > 2 - 3 (x + 3)?
Answers
Answer:
x > - 3/7
Step-by-step explanation:
6x - 2x - 4 > 2 - 3 (x + 3)
6x - 2x - 4 > 2 - 3x - 9
4x - 4 > 2 - 3x - 9
4x - 4 > - 7 - 3x
4x - 4 + 3x > - 7
4x + 3x > - 7 + 4
7x > - 7 + 4
7x > - 3
Please help
There are 135 people in a sport centre. 73 people use the gym. 59 people use the swimming pool. 31 people use the track. 19 people use the gym and the pool. 9 people use the pool and the track 16 people use the gym and the track. 4 people use all three facilities. Given that a randomly selected person uses the gym and the track, what is the probability they do not use the swimming pool?
Answers
Answer:
Answer:
1/9
Step-by-step explanation:
4 people use all three facilities, then
16 - 4 = 12 people use the gym and the track and do not use the pool;
9 - 4 = 5 people use the pool and the track and do not use the gym;
19 - 4 = 15 people use the gym and the pool and do not use the track.
At least two facilities use 4 + 12 + 5 + 15 = 36 people, 4 of them use all three facilities. Thus, the probability that a randomly selected person which uses at least two facilities, uses all the facilities is 4/36=1/9
H= Substitute the given values into the given formula and solve for the unknown variable. If necessary round to one decimal place

Answers
We need to evaluate the following expression:
\(S=4LW+2WH\)Where S is equal to 108, L is equal to 7 and W is equal to 3. We have:
\(\begin{gathered} 108=4\cdot7\cdot3+2\cdot3\cdot H \\ 108=84+6\cdot H \\ 84+6\cdot H=108 \\ 6\cdot H=108-84 \\ 6\cdot H=24 \\ H=\frac{24}{6}=4 \end{gathered}\)Can you please solve this?!?

Answers
\(\bold{\huge{\blue{\underline{ Solution }}}}\)
Given :-Here, We have,
\(\sf{\dfrac{a}{b}}{\sf{=}}{\sf{\dfrac{b}{c}}}{\sf{=}}{\sf{\dfrac{c}{d}}}\)To Prove :-We have to prove that √(a - b - c)(b-c-d) = √ab-√bc-√cdLet's Begin :-\(\sf{√(a-b-c)(b-c-d)=√ab-√bc-√cd}\)
Let \(\sf{\dfrac{a}{b}}{\sf{=}}{\sf{\dfrac{b}{c}}}{\sf{=}}{\sf{\dfrac{c}{d}}}{\sf{ = k}} \)Therefore,From above, We can conclude that,
a = bk, b = ck, c = dk
a = dk³ , b = dk² , c = dk
By solving RHS :-
\(\sf{√(a - b - c)(b-c-d) }\)
\(\sf{√(dk³ - dk² - dk) (dk² - dk - d) }\)
\(\sf{√d(k³- k² - k) d(k² - k - 1)}\)
\(\sf{d√(k³- k² - k) d(k² - k - 1)}\)
\(\sf{d√k(k²- k - 1)(k² - k - 1)}\)
\(\sf{d(k²- k - 1)√k ...eq(1)}\)
By solving LHS :-
\(\sf{√ab - √bc - √cd}\)
\(\sf{√dk³(dk²) - √dk²(dk)-√dk(d)}\)
\(\sf{√d²k^5 - √ d²k³ - √d²k}\)
\(\sf{dk²√k - dk√k - d√k}\)
\(\sf{(dk² - dk - d)√k}\)
\(\sf{d(k²- k - 1)√k...eq(2)}\)
From eq(1) and eq(2)
\(\bold{\red{ LHS = RHS }}\)
That is,
\(\sf{√(a-b-c)(b-c-d)=√ab-√bc-√cd}\)
Hence proved .Yes, the RHS and the LHS are equal/
Calculations and Parameters:Given:
\(a/b= b/c = c/d\)
We have to prove that √(a - b - c)(b-c-d) = √ab-√bc-√cd
Hence, after we expand the brackets and evaluate, we see that:
a = bk, b = ck, c = dka = dk³ , b = dk² , c = dkAfter the evaluation of the RHS and LHS, we can see that
RHS= LHS .
Read more about maths expressions here:
https://brainly.com/question/2164351
The mean exam score for 49 male high school students is 239 and the population standard deviation is 47 The mean exam score for 53 female high school students is 21.1 and the population standard deviation is 4.3. At α=001, can you reject the claim that male and female high school students ha equal exam scores? Complete parts (a) through (e). Click here to view page 1 of the standard normal distribution table. Click here to view. page 2 of the standard normal distribution table. A. Male high school students have lower exam scores than female students B. Male and temale high school students have different exam scores. C. Male and female high school students have equal exam scores D. Male high school students have greater exam scores than female students
Answers
Comparing the means of the two samples, we find that the difference between the means is significant. Therefore, we can reject the claim and conclude that male and female high school students have different exam scores.
To perform the two-sample t-test, we first calculate the standard error of the difference between the means using the formula:
SE = sqrt((s1^2 / n1) + (s2^2 / n2))
Where s1 and s2 are the population standard deviations of the male and female students respectively, and n1 and n2 are the sample sizes. Plugging in the values, we have:
SE = sqrt((47^2 / 49) + (4.3^2 / 53))
Next, we calculate the t-statistic using the formula:
t = (x1 - x2) / SE
Where x1 and x2 are the sample means. Plugging in the values, we have:
t = (239 - 21.1) / SE
We can then compare the t-value to the critical t-value at α = 0.01 with degrees of freedom equal to the sum of the sample sizes minus 2. If the t-value exceeds the critical t-value, we reject the null hypothesis.
In this case, the t-value is calculated and compared to the critical t-value using the provided standard normal distribution table. Since the t-value exceeds the critical t-value, we can reject the claim that male and female high school students have equal exam scores.
Therefore, the correct answer is:
B. Male and female high school students have different exam scores.
Learn more about hypothesis testing here: brainly.com/question/17099835
#SPJ11
Suppose that Y is a discrete random variable with mean μ and variance σ 2 and let W = 2Y .
a Do you expect the mean of W to be larger than, smaller than, or equal to μ = E(Y )?Why?
b Use Theorem 3.4 to express E(W ) = E(2Y ) in terms of μ = E(Y ). Does this result agree with your answer to part (a)?
c Recalling that the variance is a measure of spread or dispersion, do you expect the variance of W to be larger than, smaller than, or equal to σ 2 = V (Y )? Why?
d Use Definition 3.5 and the result in part (b) to show that
V ( W ) = E{[W − E(W )]2} = E[4(Y − μ)2] = 4σ 2;
that is, W = 2Y has variance four times that of Y ..
Reference
Answers
The solution is found below
What is discrete random variable ?Generally, From the information, observe that Y is the discrete random variable with mean\($\mu_{\text {and variance }} \sigma^2$\)Consider W is the random variable and it is defined as W=2 Y
a)
Yes.
The mean of W is larger than the mean of Y.
That is, E(W)>E(Y)
Since in general \($(2 \times 2) > 2,(3 \times 3) > 3,(4 \times 4) > 4, \ldots, 2 Y > Y$,\)
2 Y >Y
W >Y
E(W) >E(Y)
b)
Express \(E(W)=E(2 Y)_{\text {in terms of }} \mu=E(Y)$\)
\(\begin{aligned}E(W) & =E(2 Y) \\& =2 E(Y) \quad\left(\begin{array}{l}\text { Since } E(Y)=\mu \text { and } \\E(\{cg}(Y))=c E(g(Y))\end{array}\right) \\& =2 \mu\end{aligned}\)
Yes. Since the anticipated value of W is twice as great as the expected value of Y, this conclusion is consistent with section (a).
c)
Yes.
The Variance of W is larger than the variance of $Y$.
That is, V(W)>V(Y)
Since in general\((2 \times 2) > 2,(2 \times 3) > 3, \ldots, 2 Y > Y$\)
\(\begin{aligned}2 Y & > Y \\V(2 Y) & > Y \\V(W) & > V(Y)\end{aligned}\)
d)
Prove that \($V(W)=4 \sigma^2$\)
\(\begin{array}{rlrl}V(W) & =E\left\{\left(W-\mu_W\right)^2\right\} & & \\& =E\left\{(2 Y-2 \mu)^2\right\} & \left(\begin{array}{l}\text { Since } W=2 Y \text { and } \\E(W)=2 \mu\end{array}\right) \\& =E\left\{2^2(Y-\mu)^2\right\} & & \\& =E\left\{4(Y-\mu)^2\right\} & & \\& =4 E\left\{(Y-\mu)^2\right\} & & (\text { Since } E(c g(X))=c E(g(X))) \\& =4 \sigma^2 & & \left(\text { Since } E\left\{(Y-\mu)^2\right\}=\sigma^2\right)\end{array}\)
Read more about a discrete random variable
https://brainly.com/question/17238189
#SPJ1
will mark brainliest

Answers
Answer:
photos is not
Step-by-step explanation:
clearly posted
if there is a very weak correlation between two variables, then the coefficient of determination must be group of answer choices
Answers
Answer:
If there is a very weak correlation between two variables, then the coefficient of determination (R-squared) must be low as well.
This is because the coefficient of determination measures the proportion of variance in the dependent variable that can be explained by the independent variable(s).
When the correlation between the variables is weak, there is less variance in the dependent variable that can be explained by the independent variable(s), resulting in a lower R-squared value. So, the answer is "low".
To know more about coefficient of determination refer here
https://brainly.com/question/28975079#
#SPJ11
PLEASE HELP FAST!!!!!
Lucy received a $1300 bonus. She decided to invest it in a 3-year certificate of deposit (CD) with an annual interest rate of 1.27% compounded monthly.
Answer the questions below. Do not round any intermediate computations, and round your final answers to the nearest cent. If necessary, refer to the list of financial formulas.
(a) Assuming no withdrawals are made, how much money is in Lucy's account after 3 years?
$____
(b) How much interest is earned on Lucy's investment after 3 Years?
$____
Answers
Answer:
(a) $1350.46
(b) $50.46
Step-by-step explanation:
You want to know the future value of a $1300 CD earning 1.27% compounded monthly for 3 years, along with the amount of interest earned.
Future valueThe compound interest formula tells you the future value is ...
FV = P(1 +r/12)^(12·t)
where r is the annual interest rate and t is the number of years.
ApplicationUsing the given values, we have ...
FV = $1300(1 +0.0127/12)^(12·3) ≈ $1350.46
(a) The value after 3 years is $1350.46.
The interest earned is the difference between the final value and the amount of the original investment:
Interest = $1350.46 - 1300.00 = $50.46
(b) The interest earned by the CD is $50.46.
<95141404393>

Write the expression in expanded form (1 + 3b)(6)
Answers
Answer:
Answer Expert Verified
4.0/5
170
nikitasingh79
Genius
15.5K answers
114.1M people helped
Identity:
An identity is an equality which is true for all values of a variable in the equality.
(a + b)³ = a³+ b³+ 3ab(a + b)
In an identity the right hand side expression is called expanded form of the left hand side expression.
---------------------------------------------------------------------------------------------------
Solution:
(i) (2x + 1)³
Using identity
(a + b)³ = a³+ b³+ 3ab(a + b)
(2x + 1)³
= (2x)³ + 1³ + (3×2x×1)(2x + 1)
= 8x³+ 1 + 6x(2x + 1)
= 8x³ + 1 + 12x² + 6x
(ii) (2a – 3b)³
Using identity,
(a – b)³ = a³–b³ – 3ab(a – b)
(2a – 3b)³ = (2a)³– (3b)³ – (3×2a×3b)(2a – 3b)
=8a³–27b³–18ab(2a –3b)
= 8a³–27b³–36a²b + 54ab²
(iii) [3x/2 + 1]³
Using identity,
(a + b)³ = a³+ b³+ 3ab(a + b)
[3x/2 +1]³
=(3x/2)³+1³+ (3×(3x/2)×1)(3x/2+ 1)
=27x³/8+1+9/2x×(3x/2+1)
= 27x³/8 + 1 + 27/4 x² + 9/2x
= (27/8)x³ + (27/4) x² + 9/2 x + 1
(iv) [x–2/3 y]³
Using identity,
(a - b)³=a³-b³-3ab(a-b)
(X+ 2/3y)³
= (x)³–(2/3 y)³– (3×x×2/3 y)(x – 2/3 y)
= x³– 8y³/27–2xy(x – 2/3 y)
= x³– (8/27)y³–2x²y+ 4/3xy²
=========================================================
Hope this will help you....
Step-by-step explanation:
Write an equation in slope-intercept form for the line that passes through (3,1) and (1,2).
Answers
Answer:
y=-1/2x+2.5
Step-by-step explanation:
The slope is negative so the line would be ing to left side of the coordinate plane. the next point would be (-1,3) meaning that the line would pass the y-axis at 2.5.
Please help me out!!!

Answers
The cylindrical can of oatmeal shown is made of cardboard with diameter of 3cm and height of 10.5cm, except for the lid, which is plastic. Find the surface area of the label for the oatmeal container to the nearest hundredth.
Answers
Answer:31.5 cm
Step-by-step explanation:
3 x 10.5
i need help again on math ):

Answers
Answer:
D. 10x-20
Step-by-step explanation:
The perimeter is just all four sides added together
(3x-2)+(3x-2)+(2x-8)+(2x-8)
add together like terms
3x+3x+2x+2x=10x
-2+-2+-8+-8=-20
write in order
10x-20
The current world population is about 7.6 billion, with an
annual growth in population of 1.2%. At this rate, in how many
years will the world's population reach 10 billion?
Answers
The annual growth rate in population of 1.2% means that the population is increasing by 1.2% of the current population each year. To find the time it will take for the population to reach 10 billion, we need to use the following formula:P(t) = P0 × (1 + r)^twhere P0 is the initial population, r is the annual growth rate, t is the time (in years), and P(t) is the population after t years.
We can use this formula to solve the problem as follows: Let \(P0 = 7.6 billion, r = 0.012 (since 1.2% = 0.012)\), and P(t) = 10 billion. Plugging these values into the formula, we get: 10 billion = 7.6 billion × (1 + 0.012)^t Simplifying the right side of the equation, we get:10 billion = 7.6 billion × 1.012^tDividing both sides by 7.6 billion, we get:1.3158 = 1.012^tTaking the natural logarithm of both sides,
we get:ln\((1.3158) = ln(1.012^t)\) Using the property of logarithms that ln \((a^b) = b ln(a)\), we can simplify the right side of the equation as follows:ln(1.3158) = t ln(1.012)Dividing both sides by ln(1.012), we get:t = ln(1.3158) / ln(1.012)Using a calculator to evaluate the right side of the equation, we get:t ≈ 36.8Therefore, it will take about 36.8 years for the world's population to reach 10 billion at an annual growth rate of 1.2%.
In conclusion, It will take approximately 36.8 years for the world's population to reach 10 billion at an annual growth rate of 1.2%. The calculation was done using the formula P(t) = P0 × (1 + r)^t, where P0 is the initial population, r is the annual growth rate, t is the time (in years), and P(t) is the population after t years.
To know more about logarithms visit -
brainly.com/question/30226560
#SPJ11
Find the value of X in the parallelogram below.

Answers
Answer:
28
Step-by-step explanation:
In paralellograms, the lengths of the parallel sides are equal to each other
As seen in the figure, the side that is parallel to the side that is represented with x has a length of 28 units, so the value of x is also equal to 28
Answer:
x = 28 units
Explanation:
Properties of a parallelogram:
Opposite sides are equal.Opposite angles are equal.Opposite sides are parallel.-------------------------------------------------------
As said above, opposite sides in a parallelogram are equal. Thus, the value of x must be 28 units, as the measure of the opposite side of "x" is 28 units.
Learn more about parallelograms: https://brainly.com/question/27537537
Montrer que le cube d’un nombre pair est un multiple de 8
Answers
Answer:
so the answer is 16? I dont know , need a better explanation on the question. ill answer until then.
Step-by-step explanation:
Answer:
56
Step-by-step explanation:
the answer might be 56
Refer to the picture framing problem. A new picture with the dimensions 25 in by 10 in will be framed with fancy that is 15 in by 10 in. What will be the dimensions of frame that will surround the picture? Show all work in finding the solution.
Answers
The difference between values is gotten by subtracting the values
The dimension of the frame that will surround the picture is 10 inches by 0 inches
How to determine the dimension of the pictureThe given parameters are:
Picture = 25 inches by 10 inchesFancy = 15 inches by 10 inchesThe dimension of the frame is the difference between the dimension of the fancy, and the picture.
So, we have:
Length = 25 inches - 15 inches
Length = 10 inches
Width = 10 inches - 10 inches
Width = 0 inches
Hence, the dimension of the frame that will surround the picture is 10 inches by 0 inches
Read more about differences at:
https://brainly.com/question/2475734