If 39 is 10% of an number what is the number? How did you find your answer?
Answers
Answer:
390
Step-by-step explanation:
10% = 39
39 x 10 = 390
390 = 100%
Answer:
x=390
Step-by-step explanation:
x of 10% equal to 39
x10/100 = 39
x10 =3900
x = 3900/10
= 390
Related Questions
How many solutions are there to the inequality x1 + x2 + x3 ≤ 11, where x1, x2, and x3 are nonnegative integers? [Hint: Introduce an auxiliary variable x4 such that x1 + x2 + x3 + x4 = 11.]
Answers
The number of nonnegative integer solutions to the inequality x1 + x2 + x3 ≤ 11 is C(14,3) = 364.
We can solve this inequality by introducing an auxiliary variable x4, such that x1 + x2 + x3 + x4 = 11. Here, x1, x2, x3, and x4 are all nonnegative integers.
We can interpret this equation as follows: imagine we have 11 identical objects and we want to distribute them among four boxes (x1, x2, x3, and x4). Each box can contain any number of objects, including zero. The number of solutions to this equation will give us the number of nonnegative integer solutions to the original inequality.
We can use a technique known as stars and bars to count the number of solutions to this equation. Imagine we represent the 11 objects as stars: ***********.
We can then place three bars to divide the stars into four groups, each group representing one of the variables x1, x2, x3, and x4. For example, if we place the first bar after the first star, the second bar after the third star, and the third bar after the fifth star, we get the following arrangement:
| ** | * | ****
This arrangement corresponds to the solution x1=1, x2=2, x3=1, and x4=7. Notice that the number of stars to the left of the first bar gives the value of x1, the number of stars between the first and second bars gives the value of x2, and so on.
We can place the bars in any order, so we need to count the number of ways to arrange three bars among 14 positions (11 stars and 3 bars). This is equivalent to choosing 3 positions out of 14 to place the bars, which can be done in C(14,3) ways.
Therefore, the number of nonnegative integer solutions to the inequality x1 + x2 + x3 ≤ 11 is C(14,3) = 364.
Click the below link, to learn more about solutions of the inequality :
https://brainly.com/question/22010462
#SPJ11
A political polling agency wants to take a random sample of registered voters and ask whether or not they will vote for a certain candidate. One plan is to select 400 voters, another plan is to select 1,600 voters. If the study were conducted repeatedly (selecting different samples of people each time), which one of the following would be true regarding the resulting sample proportions of "yes" responses?
A. Different sample proportions would result each time, but for sample size 400 they would be centered (have their mean) at the true population proportion, whereas for sample size 1,600 they would not.
B. Different sample proportions would result each time, but for sample size 1,600 they would be centered (have their mean) at the true population proportion, whereas for sample size 400 they would not.
C. Different sample proportions would result each time, but for either sample size, they would be centered (have their mean) at the true population proportion.
D. For either sample size, using the same size each time, as long as the samples are drawn with replacement, they would be centered (have a mean) at 0.
Answers
Answer:
C. Different sample proportions would result each time, but for either sample size, they would be centered (have their mean) at the true population proportion.
Step-by-step explanation:
From the given information;
A political polling agency wants to take a random sample of registered voters and ask whether or not they will vote for a certain candidate.
A random sample is usually an outcome of any experiment that cannot be predicted before the result.
SO;
One plan is to select 400 voters, another plan is to select 1,600 voters
If the study were conducted repeatedly (selecting different samples of people each time);
Different sample proportions would result each time, but for either sample size, they would be centered (have their mean) at the true population proportion. This is because a sample proportion deals with random experiments that cannot be predicted in advance and they are quite known to be centered about the population proportion.
solve for x
x²+11²+(x+5)²
Answers
Answer:
2 • (x2 + 5x + 73)
Step-by-step explanation:
Step-1 : Multiply the coefficient of the first term by the constant
Step-2 : Find two factors of 73 whose sum equals the coefficient of the middle term, which is 5
Pulling out like terms/factors
Trying to factor by splitting the middle term
Answer: 2 • (x2 + 5x + 73)
Hope this helps.
Answer:
2x^2 + 10x + 146
Step-by-step explanation:
x^2 + 121 + (x+5)^2
x^2 +121 + (x+5)(x+5)
x^2 + 121 + x^2 + 5x + 5x + 25
2x^2 + 10x + 146
I also need help on this one too

Answers
Answer:
4x-9= 3x+29
+9 +9
4x=3x+38
-3x -3x
x=38
Now that we have x we can substitute it into one of the equations
3(38)+29=
114+29=
143 <- this is the angle
Answer: x=38
Step-by-step explanation:
(4x-9)=(3x+29)
4x-9=3x+29
combine like terms: subtract 3x from both sides
x-9=29
combine like terms: add 9 to both sides
x=38
Check answer:
4*38=152
152-9=143
3*38=114
114+29=143
part 2 of important test, all solutions need to be the same
helppp

Answers
The solution of the quadratic equation using the quadratic formula is
x = 4 + 2√6 or 4 - 2√6
How to solve a quadratic equation using quadratic formula?The quadratic formula is a formula used to solve quadratic equations, which are equations of the form ax² + bx + c = 0 where a, b, and c are constants. The quadratic formula is:
x = (-b±√(b²-4ac))/(2a)
Given: x² - 8x - 84 = 0
Here, a = 1, b = -8 and c = -84
x = (-b±√(b²-4ac))/(2a)
x = [-(-8)±√((-8)²-[4×1×(-8)])]/(2a)
x = [8±√(96)]/2
x = (8± 4√6)/2
x = 4 ± 2√6
x = 4 + 2√6 or 4 - 2√6
Thus, the solution is x = 4 + 2√6 or 4 - 2√6
Learn more about quadratic formula on:
https://brainly.com/question/1214333
#SPJ1
Help Please!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!

Answers
Answer:
4/8 = 1/2
Step-by-step explanation:
hope it thepled u
A paratrooper bails out of an airplane at altitude 15,000 ft, then falls freely for 30 seconds, then opens a parachute.
Answers
The speed of the parachute is 50ft/s
How to calculate the speed of an objectThe formula for calculating speed is expressed as:
Speed = Distance/Time
Given the following parameters
Distance = 15,000 feet
Time = 30 seconds
Substituting into the formula;
Speed = 1500ft/30s
Speed = 50ft/s
Hence the speed of the parachute is 50ft/s
Learn more on speed here: https://brainly.com/question/4931057
The perimeter of a triangle is 44 inches. The length of one side is twice the length of the shortest side, and the length of the other side is eight inches longer than the length of the shortest side.
Choose a variable and tell what that variable represents.
Answers
Answer:
side a = Smallest = 9 inches
side b = 18 inches
side c = 17 inches
Step-by-step explanation:
The formula for the perimeter of a triangle = side a + side b + side c
side a = Smallest
The perimeter of a triangle is 44 inches.
The length of one side is twice the length of the shortest side
Hence:
b = 2a
The length of the other side is eight inches longer than the length of the shortest side.
Hence,
c = 8 + a
Hence, we substitute into the Intial formula
a + 2a + 8 + a = 44 inches
4a + 8 = 44
4a = 44 - 8
4a = 36
a = 36/4
a = 9 inches
Solving for b
b = 2a
b = 2 × 9 inches = 18 inches
Solving for c
c = a + 8
c = 9 inches + 8 = 17 inches
Using the equation ŷ = 5.4 + 1.7x, what is the predicted value if x is 1.2?
3.36
−3.36
7.44
−7.44
−2.47
Answers
Answer:
Using the equation ŷ = 5.4 + 1.7x, we can say that if x is 1.2, the predicted value will be 7.44.
Step-by-step explanation:
y=5.4+1.7(1.2)
Multiply 1.7 and 1.2 to get 2.04.
y=5.4+2.04
Add 5.4 and 2.04 to get 7.44.
y=7.44
There you go!
(Sources cited from Microsoft Math Solver.)
There is a line through the origin that divides the region bounded by the parabola y=5x−3x^2 and the x-axis into two regions with equal area. What is the slope of that line?
Answers
The slope of the line that divides the region bounded by the parabola \(y=5x-3x^2\)and the x-axis into two regions with equal area is 5.
To find the slope of the line that divides the region into two equal areas, we need to determine the point of intersection between the parabola and the x-axis. Since the line passes through the origin, its equation will be y = mx, where m represents the slope.
Setting the equation of the parabola equal to zero, we find the x-values where the parabola intersects the x-axis. By solving the equation\(5x - 3x^2 = 0\), we get x = 0 and x = 5/3.
To divide the region into two equal areas, the line must pass through the midpoint between these x-values, which is x = 5/6. Plugging this value into the equation of the line, we have y = (5/6)m.
Since the areas on both sides of the line need to be equal, we can set up an equation using definite integrals. By integrating the equation of the parabola from 0 to 5/6 and setting it equal to the integral of the line from 0 to 5/6, we can solve for m. After performing the integration, we find that m = 5.
Therefore, the slope of the line that divides the region into two equal areas is 5.
Learn more about: Parabola
brainly.com/question/11911877
#SPJ11
find the volume of the parallelepiped with adjacent edges pq, pr, and ps. p(−2, 1, 0), q(4, 4, 4), r(1, 4, −1), s(3, 6, 1)
Answers
The volume of the parallelepiped with adjacent edges PQ, PR, and PS is 24 cubic units.
What is parallelepiped?Six parallelograms come together to form a three-dimensional shape called a parallelepiped (the term rhomboid is also sometimes used with this meaning). It is comparable to a parallelogram in the same way that a cube is comparable to a square. In Euclidean geometry, the four concepts—parallelepiped and cube in three dimensions, parallelogram, and square in two dimensions—are specified; however, only parallelograms and parallelepipeds exist in the setting of a more generic affine geometry, where angles are not discriminated.
There are three similar descriptions of a parallelepiped:
A polyhedron called a hexahedron, which has six parallelogram-shaped facesThree pairs of parallel faces on a hexahedron, andA prism with a parallelogram as its base.We have been that p(−2, 1, 0), q(4, 4, 4), r(1, 4, −1), and s(3, 6, 1) are the adjacent edges of parallelepiped
PQ = Q-P = <6,3,4>
PR = R-P = <3,3.-1>
PS= S-P = <5,5,1>
[ PQ PR PS] = \(\begin{vmatrix}6& 3 & 4 \\ 3& 3 &-1 \\ 5 & 5 & 1 \\ \end{vmatrix}\\ \notag&& \\\)
= 6(3+5)-3(3+5)+4(15-15)
= 6×8-3×8+0
=48-24
=24
To learn more about parallelepiped, visit:
https://brainly.com/question/28174749
#SPJ4
The unit rate for a gym membership is $?
per month.

Answers
Answer:
let mw know if im wrong but i think the answer would most likely be 150
Answer:
9,15
Step-by-step explanation:
3x3=9
3x5=15
both timed by 3 and they are both rates so there you go hope this helps!
first test(x)=86,82,47,69,73,47,63,50,59,77,88,87second test (y)=81,71,51,75,69,44,48,48,58,68,83,77find a equation of the least squares regression line. Round the answer to three decimal places, if necessary.
Answers
To find the equation of the line, we will use the formula:
y=mx + b
The formula for calculating the slope is given as :
\(m=\frac{N\text{ }\Sigma(xy)-\Sigma x\Sigma y}{N\text{ }\Sigma(x^2)-(\Sigma x)^2}\)Whats the answer y’all

Answers
The expressions that will give you a difference of 5 are: -3 - (-8) and 1 - (-4).
What is the Difference of Two Expressions?The difference of two expressions is determined by subtracting one from the other.
Find the difference of each of the expressions given to determine which will give us 5.
-3 - (-8)
= -3 + 8
= 5
-2 - 3 = -5 [this is not the same as 5]
1 - (-4)
= 1 + 4
= 5
7 - (-2)
= 7 + 2
= 9
-3 - (-8) and 1 - (-4) will give us a difference of 5.
Learn more about Difference of Two Expressions on:
https://brainly.com/question/28986379
#SPJ1
Solve this quadratic equation using the quadratic formula
(3 - y)(y + 4) = 3y - 5

Answers

Complete the problem to represent the situation given by the equation 0.40e + 10 + 15 = 0.20e + 5 + 25.
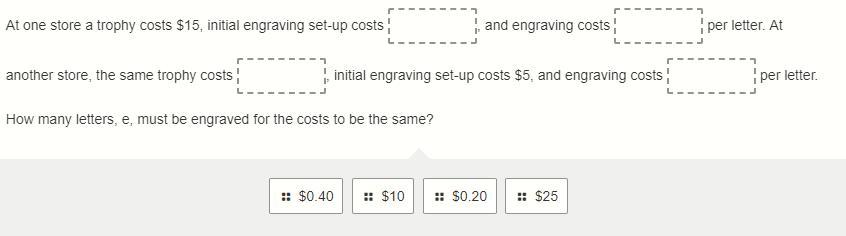
Answers
The number of letters that must be engraved for the costs to be the same is 25.
What is an expression?
Expression in mathematics is defined as the collection of the numbers variables and functions by using signs like addition, subtraction, multiplication, and division.
Since we have given that :
0.40e + 10 + 15 = 0.20e + 5 + 25.
We need to find the number of letters by rearranging the like terms and simplifying for e value :
0.40e + 25 = 0.20e + 30
0.40e - 0.20e = 30-25
0.20e = 5
e = 5/0.20
e = 50/2
e = 25
Hence, the number of letters that must be engraved for the costs to be the same is 25.
Read more about additive identity at:
https://brainly.com/question/11363695
#SPJ1
Evaluate the following.
5 + 3x5-16 + 4
Answers
Answer:
8
Step-by-step explanation:
Order of operations
Look below

Mr. Yoshi has 75 papers. He graded 60 papers and he had a student teacher grade the rest. What percent of the papers did each grade
Answers
Answer:
Student=20%
Teacher=80%
Step-by-step explanation:
60/75 x100
= 80
100-80
=20
Using the side splitter theorem, which segment length would complete the proportion?
Answers
As per the side splitter theorem, the segment length would complete the proportion is GJ
In math, the side splitter theorem states that "if a line is parallel to a side of a triangle and the line intersects the other two sides, then this line divides those two sides proportionally".
Here we have to find the segment length that would complete the proportion by using the side splitter theorem.
According to this theorem and by using the following diagram, we can elaborate the following proportion
=> GH/HE = GJ/JF
Here we have identifies that the segment that completes the proportion is GJ, because it must be used according to the theorem.
To know more about side splitter theorem here.
https://brainly.com/question/9793212
#SPJ4

Ok so 0.5 x 10^5 = ? What is the answer? The 5 is little and its on top please i don't know how to explain.
Answers
Answer:
50,000
Step-by-step explanation:
The little 5 is called an exponent, so it would be 0.5*10^5.
Ten to the fifth power. you can take the ten and do this. 10x10x10x10x10. The answer would be fifty thousand
What's the reciprocal of 3 3/4?
Answers
Answer:
154
Step-by-step explanation:
334=(4×3)+34=154
I NEED HELP QUICKLY for both X

Answers
The solution of the quadratic equation is x = 2. Therefore, \(\frac{4+\sqrt{-4^{2}-4(1)(4) } }{2(1)}\) or \(\frac{4-\sqrt{-4^{2}-4(1)(4) } }{2(1)}\)
How to solve quadratic equation?The quadratic formula can be use to solve the quadratic equation as follows:
x² - 4x + 4 = 0
Modelling it to quadratic equation, ax² + bx + c
Hence,
using quadratic formula,
\(\frac{-b+\sqrt{b^{2}-4ac } }{2a}\) or \(\frac{-b-\sqrt{b^{2}-4ac } }{2a}\)
where
a, b and c are the coefficient in the equationHence,
a = 1
b = -4
c = 4
Therefore,
\(\frac{4+\sqrt{-4^{2}-4(1)(4) } }{2(1)}\) or \(\frac{4-\sqrt{-4^{2}-4(1)(4) } }{2(1)}\)
Finally
x = 2
learn more on quadratic equation here: https://brainly.com/question/30456542
#SPJ1
1.2m xm The box is put on a table. The face of the box in contact with the table has length 1.2 metres and width The force exerted by the box on the table is 27 newtons. The pressure on the table due to the box is 30 newtons/m² pressure = force Diagram NOT accurately draw area
Answers
The value of x, given that a force of 27 newtons was exerted by the box and the pressure on the table due to the box is 30 newtons/m² is 0.75 m
How do i determine the value of x?First, we shall obtain the area of the cuboid. This is obtained as shown below:
Force exerted (F) = 27 newtonsPressure due to the box (P) = 30 newtons/m²Area (A) =?Pressure (P) = Force (F) / Area (A)
30 = 27 / Area
Cross multiply
30 × A = 27
Divide both sides by 30
A = 27 / 30
Area = 0.9 m²
Finally, we shall obtain the value of x. This is shown below:
Area = 0.9 m²Base length = 1.2 mValue of x =?Area = base length × x
0.9 = 1.2 × x
Divide both sides by 1.2
x = 0.9 / 1.2
x = 0.75 m
Thus, we can conclude from the above calculation that the value of x is 0.75 m
Learn more about force, area and pressure:
https://brainly.com/question/31995496
#SPJ1
Complete question:
See attached photo

Lindsey bought a picnic basket originally priced at $40 but on sale for 50% off. After 10% sales tax, what was the total cost?
$
Answers
Answer:
Step-by-step explanation:
I got 22 my friend hope i helped.
Answer:
$18
Step-by-step explanation:
50% of 40 is 20, so 40-20=20
10% of 20 is 2, so 20-2=18
total price=$18
If (7²)x = 1 what is the vaule of x
Answers
Answer:
x=4
Step-by-step explanation:
1 Add 11 to both sides.
2x=7+12x=7+1
2 Simplify 7+17+1 to 88.
2x=82x=8
3 Divide both sides by 22.
x=\frac{8}{2}x=
2
8
4 Simplify \frac{8}{2}
2
8
to 44.
x=4x=4
cheek:
1 Let x=4x=4.
2\times 4-1=72×4−1=7
2 Simplify 2\times 42×4 to 88.
8-1=78−1=7
3 Simplify 8-18−1 to 77.
7=7 7=7
(or -3)
Answer:
x=1/49
Step-by-step explanation:
Rearrange the equation by subtracting what is to the right of the equal sign from both sides of the equation
Add 1 to both sides of the equation :
49x = 1
Divide both sides of the equation by 49:
x = 1/49 = 0.020
Hope this helps....
The inside diameter of a randomly selected piston ring is a random variable with mean value 10 cm and standard deviation 0.07 cm.
(a) If
X
is the sample mean diameter for a random sample of n = 16 rings, where is the sampling distribution of
X
centered and what is the standard deviation of the
X
distribution? (Enter your standard deviation to five decimal places.)
center cm
standard deviation cm
(b) Answer the questions posed in part (a) for a sample size of n = 64 rings. (Enter your standard deviation to five decimal places.)
center cm
standard deviation cm
(c) For which of the two random samples, the one of part (a) or the one of part (b), is
X
more likely to be within 0.01 cm of 10 cm? Explain your reasoning.
X
is more likely to be within 0.01 cm of 10 cm in sample (a) because of the increased variability with a smaller sample size.
X
is more likely to be within 0.01 cm of 10 cm in sample (b) because of the increased variability with a larger sample size.
X
is more likely to be within 0.01 cm of 10 cm in sample (b) because of the decreased variability with a larger sample size.
X
is more likely to be within 0.01 cm of 10 cm in sample (a) because of the decreased variability with a smaller sample size.
Answers
We are given that the inside diameter of a piston ring is a random variable with mean value 10 cm and standard deviation 0.07 cm.
We are asked to find the sampling distribution of the sample mean diameter for two different random samples of n=16 and n=64 piston rings, respectively, and to calculate the standard deviation of each sampling distribution.
The sampling distribution of the sample mean diameter is the distribution of all possible sample means that could be obtained from a given sample size n.
The central limit theorem tells us that for large enough sample sizes (typically n ≥ 30), the sampling distribution of the sample mean is approximately normal, regardless of the shape of the underlying population distribution.
The mean of the sampling distribution of the sample mean is equal to the population mean, and the standard deviation of the sampling distribution is equal to the population standard deviation divided by the square root of the sample size.
For the first random sample of n=16 piston rings, the center of the sampling distribution of the sample mean diameter is still 10 cm, as this is the population mean.
However, the standard deviation of the sampling distribution is equal to the population standard deviation of 0.07 cm divided by the square root of 16, which is 0.0175 cm. Therefore, the standard deviation of the sampling distribution of the sample mean diameter for the first random sample is 0.0175 cm.
For the second random sample of n=64 piston rings, the center of the sampling distribution of the sample mean diameter is still 10 cm, but the standard deviation of the sampling distribution is equal to the population standard deviation of 0.07 cm divided by the square root of 64, which is 0.00875 cm.
Therefore, the standard deviation of the sampling distribution of the sample mean diameter for the second random sample is 0.00875 cm.
Finally, we are asked which of the two random samples is more likely to have a sample mean diameter within 0.01 cm of 10 cm. Since the standard deviation of the sampling distribution of the sample mean decreases as the sample size increases, the second random sample of n=64 piston rings is more likely to have a sample mean diameter within 0.01 cm of 10 cm.
This is because the decreased variability of the sampling distribution means that the sample means are more tightly clustered around the population mean of 10 cm. Therefore, we can conclude that the second random sample is more precise than the first random sample.
In statistics, a random sample is a subset of a larger population that is selected in such a way that each member of the population has an equal chance of being included in the sample.
Random sampling is a crucial tool for drawing conclusions about a population based on a smaller subset of data. In this problem, we will explore the sampling distribution of the sample mean for two different random samples of piston rings.
To know mare about random sample refer here:
https://brainly.com/question/29852583#
#SPJ11
Angie earns $45 a day. she spends $16 each day and saves the rest. how much will angie save in 4 weeks?
Answers
To find how much Angie will save in 4 weeks, we multiply her daily savings ($29) by the number of days in 4 weeks (28). $812 in 4 weeks.Angie will save $812 in 4 weeks.
To find out how much Angie will save in 4 weeks, we need to calculate her savings per day and then multiply it by the number of days in 4 weeks.
Angie earns $45 a day and spends $16 each day, so her daily savings would be $45 - $16 = $29.
Now, we need to find the number of days in 4 weeks. Since there are 7 days in a week, we multiply 4 weeks by 7 days, which gives us 4 * 7 = 28 days.
Finally, to find how much Angie will save in 4 weeks, we multiply her daily savings ($29) by the number of days in 4 weeks (28).
$29 * 28 = $812.
Therefore, Angie will save $812 in 4 weeks.
To know more about number visit:
https://brainly.com/question/3589540
#SPJ11
Gary engages in skin picking. Jerry calculates how many times per hour, on average, Gary engages in skin picking during the session Rate Frequency/ Count Latency Inter response time Duration
Answers
Gary's skin picking behavior, rate/frequency is determined by counting the number of times it occurs per hour. Count refers to the total number of occurrences during the session, latency measures time between cue and behavior, inter-response time is the time between behaviors and duration refers to how long each behavior lasts.
Rate or frequency refers to how often an event occurs within a given time period. The session lasts for one hour and Gary engages in skin picking 10 times during that hour, his rate or frequency would be 10 times per hour.
Count refers to the total number of times the event occurs during the entire session. Latency refers to the time between a specific event or cue and the start of the behavior. Inter-response time refers to the time between the end of one behavior and the start of the next. Duration refers to how long the behavior lasts.
Gary engages in skin picking behavior 50 times during a 2-hour session, his count would be 50.
If a specific trigger leads to Gary beginning to engage in skin picking behavior 10 seconds later, his latency would be 10 seconds.
Inter-response time will be if Gary engages in skin picking behavior, stops for 20 seconds, and then starts again, his inter-response time would be 20 seconds.
If Gary's skin picking behavior lasts an average of 30 seconds each time he engages in it, his duration would be 30 seconds.
To know more about Rate Frequency:
https://brainly.com/question/14972647
#SPJ4
Which one of the following is NOT an assumption for a linear regression model? a. All the variables are normally distributed. b. Y and X have a linear relationship. c. The observations are independent. d. The variance of residual is the same for all values of X.
Answers
The assumption that is NOT applicable for a linear regression model is option (a) "All the variables are normally distributed." The other assumptions for a linear regression model are (b) Y and X have a linear relationship, (c) the observations are independent, and (d) the variance of the residual is the same for all values of X.
Linear regression models have several assumptions that need to be met for the model to be valid and reliable. Let's go through each of the given options to determine which one is not an assumption for a linear regression model:
a. All the variables are normally distributed: This assumption is not necessary for a linear regression model. The response variable (Y) does not need to follow a normal distribution, but rather the residuals should be normally distributed.
b. Y and X have a linear relationship: This is a fundamental assumption for linear regression models. It assumes that there is a linear relationship between the response variable (Y) and the predictor variable(s) (X).
c. The observations are independent: This assumption states that the observations in the dataset are independent of each other. The independence assumption helps ensure that the model's estimates are not biased or influenced by any correlation or dependency between the observations.
d. The variance of the residual is the same for all values of X: This assumption, known as homoscedasticity, assumes that the variability of the residuals is constant across different values of the predictor variable(s) (X). It means that the spread of the residuals should be consistent across the entire range of X values.
Therefore, the assumption that is NOT applicable for a linear regression model is option (a) "All the variables are normally distributed."
To learn more about linear regression click here : brainly.com/question/13328200
#SPJ11
40) On a balanced seesaw, a boy three times as heavy as his partner sitsA) less than 1/3 the distance from the fulcrum.B) 1/3 the distance from the fulcrum.C) more than 1/3 the distance from the fulcrum.
Answers
The correct option for the given question is 1/3 distance from the fulcrum which is option B according to the balance rule.
On a balanced seesaw, the torques around the fulcrum calculated on one side and on another side must be equal. This means that the:\(W_1 d_1 = W_2 d_2\)
where we label the things here,
\(W_1\) is the weight of the boy
\(d_1\) is its distance from the fulcrum
\(W_2\) is the weight of his partner
\(d_2\) is the distance of the partner from the fulcrum
We know that the boy is three times heavier than his companion in this situation, so
\(W_1 = 3 W_2\)
If we plug this into the equation, we get:
\((3 W_2) d_1 = W_2 d_2\)
and by simplifying:
\(3 d_1 = d_2\\\\d_1 = \frac{1}{3}d_2\)
From the above equation, we get that the boy sits at 1/3 the distance from the fulcrum.
Learn more about torques:
https://brainly.com/question/17230318
#SPJ4