if the hole is 5.7 m from a 1.8- m tall person, how tall will the image of the person on the film be?
Answers
If the hole is 5.7 m from a 1.8- m tall person, using similar triangles and the concept of magnification in optics, the height of the image of the person on the film will be 3.6 meters.
To determine the height of the image of the person on the film, we can use similar triangles and the concept of magnification in optics.
Let's denote the height of the person as P, the height of the image as I, and the distance from the person to the film as D.
According to similar triangles, we can set up the following proportion:
P / D = I / (D + 5.7)
Given that the height of the person (P) is 1.8 m and the distance from the person to the hole (D) is 5.7 m, we can plug in these values into the equation:
1.8 / D = I / (D + 5.7)
To find the height of the image (I), we need to solve for I. By cross-multiplying and rearranging the equation, we get:
I = (1.8 * (D + 5.7)) / D
Now, let's plug in the value of D (distance from the person to the hole) as given, which is 5.7 m:
I = (1.8 * (5.7 + 5.7)) / 5.7
Simplifying the equation further, we have:
I = (1.8 * 11.4) / 5.7
I = 3.6 m
Therefore, the height of the image of the person on the film will be 3.6 meters.
Learn more about similar triangles here:
https://brainly.com/question/29378183
#SPJ11
Related Questions
10.メ-2(x-y)-2 Fx = -3,y=4
and z = -1
problem in the photo
algebra

Answers
The value of the expression x²-2(x-y)-z³ is 24.
What is an expression?One mathematical expression makes up a term. It might be a single variable (a letter), a single number (positive or negative), or a number of variables multiplied but never added or subtracted. Variables in certain words have a number in front of them. A coefficient is the number used before a phrase.
Given;
Expression x²-2(x-y)-z³
To find the value of the expression:
If x = -3, y=4 and z = -1.
Substituting the values to the expression,
x²-2(x-y)-z³
= (-3)²-2(-3-4)-(-1)³
= 9 + 14 - (-1)
= 9+14+1
= 24
Therefore, 24 is the value of the expression.
To learn more about the expression;
brainly.com/question/24242989
#SPJ1
prove that if g is a finite group, the index of z(g) cannot be prime
Answers
if G is a finite group, the index of Z(G) cannot be prime.
Let's consider a finite group G with the center Z(G). We want to prove that the index of Z(G) in G cannot be a prime number.
Assume, for the sake of contradiction, that the index of Z(G) in G is a prime number, say p. By definition, the index [G:Z(G)] is equal to the number of distinct cosets of Z(G) in G, which would be p. Since G is a finite group, we can apply the Lagrange's theorem which states that the order of any subgroup (in this case, Z(G)) divides the order of the group (|G|). So, |Z(G)| divides |G| and |G| = p * |Z(G)|.
Now, let's consider the action of G on the set of left cosets of Z(G) by left multiplication. This action gives rise to a homomorphism from G to the symmetric group on p elements, S_p. By the First Isomorphism Theorem, we know that the image of this homomorphism, denoted as Im(φ), is isomorphic to G/Ker(φ), where Ker(φ) is the kernel of the homomorphism.
Observe that Z(G) is a subgroup of the kernel, as any element from Z(G) will fix each coset. This means |Ker(φ)| ≥ |Z(G)|. Furthermore, Ker(φ) is a normal subgroup of G, so the index [G:Ker(φ)] must divide |G| = p * |Z(G)|.
Since |G/Ker(φ)| = |Im(φ)| divides |S_p| = p!, and |Im(φ)| = [G:Ker(φ)], we must have either |Im(φ)| = p or |Im(φ)| = 1. If |Im(φ)| = p, then [G:Ker(φ)] = p, and Ker(φ) = Z(G). However, this would imply that the action is trivial, which is a contradiction. Thus, |Im(φ)| = 1, meaning that the action is trivial, and G = Z(G), which contradicts our initial assumption that the index of Z(G) in G is prime.
Hence, if G is a finite group, the index of Z(G) cannot be prime.
To learn more about Lagrange's theorem click here
brainly.com/question/31637769
#SPJ11
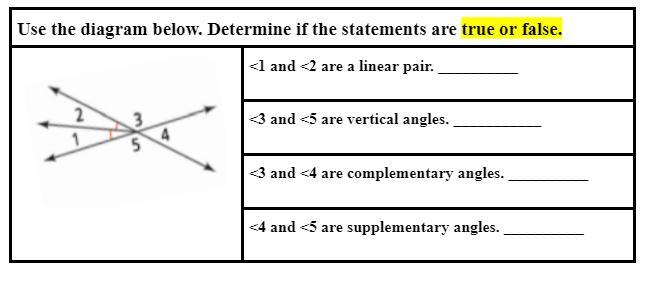
Please fill our the graphs in the images


Answers
Angle refers to the point at which a vertical line meets with a horizontal line.
What are angles?
The term angle refers to the point at which a vertical line meets with a horizontal line. Given that angles could be related to each other, we have been asked to obtain the kind of relationship that exists among the angles shown.
The relationships are as follows;
<1 and <2 are linear pair - True<3 and <5 re vertical angles - True<3and <4 are complimentary angles - False<4 and <5 are supplementary angles - TrueLearn more about angles:https://brainly.com/question/13954458
#SPJ1
The multiplicity of a root r of the characteristic equation of A is called the algebraic multiplicity of r as an eigenvalue of A. T/F
Answers
True. The multiplicity of a root r of the characteristic equation of matrix A is indeed called the algebraic multiplicity of r as an eigenvalue of A.
The characteristic equation of a square matrix A is obtained by subtracting λI (where λ is an eigenvalue and I is the identity matrix) from A and taking its determinant. The roots of this equation are the eigenvalues of matrix A.
The algebraic multiplicity of an eigenvalue r refers to the number of times r appears as a root of the characteristic equation. In other words, it represents the multiplicity of r as a solution of the equation.
The algebraic multiplicity provides information about the behavior of the eigenvalue r within the matrix A. If the algebraic multiplicity of r is greater than 1, it means that r is a repeated eigenvalue and there exist multiple linearly independent eigenvectors associated with it. On the other hand, if the algebraic multiplicity is 1, r is a simple eigenvalue, indicating that there is only one linearly independent eigenvector corresponding to r.
Learn more about square matrix here:
https://brainly.com/question/27927569
#SPJ11
Need Help ASAP PLZ And Thank You No Files...

Answers
Answer:
152 feet^2
Step-by-step explanation:
1: Set up the formula of the surface area of a rectangular prism:
\(2(wl+hl+hw)\)2: (Solving)
\(2(2*8+6*8+6*2)\) \(2(16+48+12)\) \(2(76)\) 152Therefore, the answer is 152 feet^2.
The minute hand is 12 feet long. How far does the tip of the minute sweep between 9:00 and 9:50?
Answers
50/60 * 24π = 20π ≈ 62.8 ft
Last year, there were 123 pies baked for the bake sale. This year, there were b pies baked. Using b, write an expression for the total number of pies baked in the two years.
Answers
A recent study was conducted on the development of forests following wildfires in the Pacific Northwest. Researchers studied the tree diameters at breast height 110 years after the fire. The population of Douglas Fir trees was shown to have a diameter of approximately Normal distribution with mean =49 centimeters and sigma =10 cm. a.) What proportion of the population of Douglas Fir trees have a diameter between 55 and 65 cm ? (Leave answer in decimal form.) Round to three decimal points. b.) If three Douglas Fir trees were selected at random, the probability that exactly 2 of them had diameters between 55 and 65 cm is Round to three decimal points. c.) Determine the diameters that are symmetric about the mean that include 80% of all Douglas Fir trees. and Round to one decimal point.
Answers
a) Proportion of the population of Douglas Fir trees have a diameter between 55 and 65 cm = 0.219
b) Probability that exactly 2 of them had diameters between 55 and 65 cm = 0.034
c) The diameters that are symmetric about the mean that include 80% of all Douglas Fir trees are between 36.8 cm and 61.2 cm.
a.) Proportion of the population of Douglas Fir trees have a diameter between 55 and 65 cm
To find the proportion of the population of Douglas Fir trees that have a diameter between 55 and 65 cm, we need to standardize the values of 55 and 65 using the formula:
z = (x - μ)/σwhere, x = 55 and μ = 49, σ = 10z = (55 - 49) / 10= 0.6
Using the z-score table, the area to the left of the z-score is 0.7257.
z = (x - μ)/σz = (65 - 49) / 10= 1.6
Using the z-score table, the area to the left of the z-score is 0.9452.
Proportion of the population of Douglas Fir trees have a diameter between 55 and 65 cm = P(0.6 ≤ z ≤ 1.6)
P(0.6 ≤ z ≤ 1.6) = 0.9452 - 0.7257= 0.2193
Rounding it to three decimal points, we get, Proportion of the population of Douglas Fir trees have a diameter between 55 and 65 cm = 0.219
b.) Probability that exactly 2 of them had diameters between 55 and 65 cm
For calculating the probability that exactly 2 of the 3 trees had diameters between 55 and 65 cm, we will use the Binomial probability formula, which is:
P(X = k) = nCk . p^k . q^(n-k)where, n = 3, k = 2, p = 0.219 and q = 1 - p = 0.781
Now,P(X = 2) = 3C2 . 0.219^2 . 0.781^(3-2)P(X = 2) = 3 . 0.219^2 . 0.781P(X = 2) = 0.0340
Rounding it to three decimal points, we get, Probability that exactly 2 of them had diameters between 55 and 65 cm = 0.034
c.) Determine the diameters that are symmetric about the mean that include 80% of all Douglas Fir trees.
To determine the diameters that are symmetric about the mean that include 80% of all Douglas Fir trees, we need to find the z-scores associated with the 10th and 90th percentiles of the normal distribution.
Since the distribution is symmetric, the z-score at the 10th percentile is -1.28 and the z-score at the 90th percentile is 1.28.
The corresponding values of x can be found using the formula for z-score.
z = (x - μ)/σ-1.28 = (x - 49)/10
Solving for x, we get:
x = 36.8 (rounded to one decimal point)
1.28 = (x - 49)/10
Solving for x, we get:
x = 61.2 (rounded to one decimal point)
Therefore, the diameters that are symmetric about the mean that include 80% of all Douglas Fir trees are between 36.8 cm and 61.2 cm.
To learn more about probability
https://brainly.com/question/13604758
#SPJ11
Simplify (7x2 + 3)(7x2 – 3) using the difference of squares formula.
Answers
The Solution:
Given:
\(\left(7x^2+3\right)\left(7x^2-3\right)\)Required:
Simplify the given expression using the difference of two squares.
The difference of two squares formula:
\(\)
Hey Guys ~
I need help on my math please...
Which of the following is the solution of \(2x~+~14=22\) ?
A. \(x = 4\)
B. \(x = 8\)
C. \(x = 11\)
D. \(x = 14\)
Explain it your own words ~
Or, you will be reported.
Answers
Well this is ez Answer:
A. IS THE CORRECT ANSWER I HAD TO FIND IT OUT FOR A WHILE
Step-by-step explanation:
So first you see r = 4 that is correct the answer we will get from that is 8 so next you multiply every number that r = for A. B. C. And D. so after that you multiply the correct one which is 2 x 4 so that will be 8 next you add 14 + 8 which you get the answer 22 so A. is the correct answer (SMart variable)
![Hey Guys ~I need help on my math please...Which of the following is the solution of [tex]2x~+~14=22[/tex]](https://i5t5.c14.e2-1.dev/h-images-qa/answers/attachments/W2YSHD7KYvWzDwXI2umlR1LbKcyrxNwo.jpeg)
![Hey Guys ~I need help on my math please...Which of the following is the solution of [tex]2x~+~14=22[/tex]](https://i5t5.c14.e2-1.dev/h-images-qa/answers/attachments/QjRMVAKqi1MGliDwUTExT2OM1dv37Ok8.jpeg)
![Hey Guys ~I need help on my math please...Which of the following is the solution of [tex]2x~+~14=22[/tex]](https://i5t5.c14.e2-1.dev/h-images-qa/answers/attachments/gCfP5ScslNMuI3b5xFtZSl0OHxT20xbc.jpeg)
Can someone pass help!!:(

Answers
Answer:
proofs attached to answer
Step-by-step explanation:
proofs attached to answer

Isabella draws a mystery number from a bag with various integers inside and then describes it to the class read the clues below and then Circle all of the integers that it could be
1. The opposite of the integer is positive
2. The absolute value of the integer is positive
3. The integer is not zero
-8
11
15
-21
Answers
An integer exists a whole number that can be positive, negative, or zero and is not a fraction.
What is meant by an integer?Unlike fractional numbers, integers can only be positive, negative, or zero. On integers, we can carry out all arithmetic operations, including addition, subtraction, multiplication, and division. An integer is a whole number that can be positive, negative, or zero and is not a fraction.
Any number that exists a part of a collection of all the aforementioned number categories is considered an integer. Zero, a positive natural number, or a negative integer denoted by a minus sign are all examples of integers. The inverse additives of the equivalent positive numbers are the negative numbers.
To learn more about integers refer to:
https://brainly.com/question/17695139
#SPJ9
Given the equation -2x+3y=12, find the missing value in the ordered pair 3,
Noo linkss plz
Answers
Answer:
(3,6)
Step-by-step explanation:
-2x+3y=12
Let x = 3
-2(3) +3y = 12
-6+3y = 12
Add 6 to each side
-6+6 +3y = 12+6
3y = 18
Divide each side by 3
3y/3 = 18/3
y = 6
Which ordered pair is a solution to the system of linear equations One-half x minus three-fourths y = StartFraction 11 Over 60 EndFraction and Two-fifths x + one-sixth y = StartFraction 3 Over 10 EndFraction?
Answers
The ordered pair (x, y) = (3/5, 1/2) satisfies both equations in the given system, making it the solution.
The solution to the given system of linear equations is the ordered pair (x, y) = (3/5, 1/2). This means that when x is equal to 3/5 and y is equal to 1/2, both equations are satisfied simultaneously.
In the first equation, One-half x minus three-fourths y equals 11/60.
By substituting x = 3/5 and y = 1/2 into this equation, we have (1/2)(3/5) - (3/4)(1/2) = 11/60, which simplifies to 3/10 - 3/8 = 11/60. By further simplifying, we get 24/80 - 30/80 = 11/60, which is true since both sides are equal to 11/60.
Similarly, in the second equation, Two-fifths x plus one-sixth y equals 3/10.
Substituting x = 3/5 and y = 1/2, we have (2/5)(3/5) + (1/6)(1/2) = 3/10, which simplifies to 6/25 + 1/12 = 3/10.
By finding a common denominator and simplifying, we get 144/600 + 50/600 = 180/600, which is also equal to 3/10.
Thus, the ordered pair (x, y) = (3/5, 1/2) satisfies both equations in the given system, making it the solution.
For more such answers on linear equations
https://brainly.com/question/2030026
#SPJ8
Write the equation of a line in slope intercept form that has a slope of -3 and passes through the point (0,7)
Answers
How do you do this? I'll give brainliest, please help.

Answers
Answer:
Domain is all values of X or (-∞,∞)
Range is all the possible values of Y. Since it goes up to 3, and then it goes down, it's all values less than or equal to y, or (-∞,3] or y ≤ 3 (depending on how you need to enter the response.
Step-by-step explanation:
How do you find the angles of a 5/12/13 triangle?
Answers
The angles of a triangle with side lengths 5 , 12 and 13 are 90°, 22.63°, 67.38°.
What is Law of cosines formula?In trigonometry, the law of cosines, also known as the law of cosines or cosine formula, basically relates the length of a triangle to one cosine of its angle. It states that if we know the lengths of two sides of a triangle and the angle between them, we can determine the length of the third side. c² = a²+ b² – 2ab cosγ
where a, b, c are the sides of the triangle and γ is the angle between a and b, see image above.
To find the length of a side of a triangle, take △ABC according to the cosine formula, which can be written as follows.
a² = b²+ c²– 2bc cos αb²= a² + c²– 2ac cos βc²= b² + a² – 2ba cos γWe have , a = 5 , b = 12 , c= 13
cos α =( 12² + 13² - 5² )/2×12×13= 288/312
=> α = cos⁻¹(0.923 ) = 22.63°
cos β = (5² + 13² - 12² )/2×13×5 = 50/130
=> β = cos⁻¹(5/13) = 67.38°
cos γ = (12² + 5² - 13² )/2×12×5= 0
=> γ = cos⁻¹(0) = 90°
Hence, the required angles are 90°, 22.63°, 67.38°.
To learn more about law of cosines formula, refer:
https://brainly.com/question/4372174
#SPJ4

Ben is paid £9.60 per hour.
If he works for more than 7 hours in a day, he is paid overtime for the additional hours at a rate of 1.5 times his normal rate of pay.
Yesterday, Ben worked for 12 hours.
How much money did he earn
Answers
Answer:
u liar>>>>>>
Step-by-step explanation:
(Your answer will be a fraction. In the answer box write is
as a decimal rounded to two place.)
2x+8+4x = 22
X =
Answer
Answers
The value of x is 7/3, which can be rounded to two decimal places as approximately 2.33.
To solve the equation 2x + 8 + 4x = 22, we need to combine like terms and isolate the variable x.
Combining like terms, we have:
6x + 8 = 22
Next, we want to isolate the term with x by subtracting 8 from both sides of the equation:
6x + 8 - 8 = 22 - 8
6x = 14
To solve for x, we divide both sides of the equation by 6:
(6x) / 6 = 14 / 6
x = 14/6
Simplifying the fraction 14/6, we get:
x = 7/3
Therefore, the value of x is 7/3, which can be rounded to two decimal places as approximately 2.33.
for such more question on decimal places
https://brainly.com/question/24015908
#SPJ8
I need help please show your work

Answers
Answer:
The 2nd equation is false.
Step-by-step explanation:
You don't even have to solve. DE is not 58, it's 40.
The 2nd equation is false.
I know that this is simple algebra but I am asking for your help step by please will mark brainliest for who gets this right.

Answers
Answer: Y= -9 + 3x/2
Step-by-step explanation: Down below Have a brilliant day-Lily ^_^


Use the table to determine a reasonable estimate for lim 2x^2 please HURRY

Answers
Answer:
\(\frac{1}{2}\)
Step-by-step explanation:
The expression \(\lim_{x\rightarrow 3} \frac{2x^2-x+15}{x^3-5x-12}\) is used to denote the function's behavior as \(x\) approaches 3.
From the graph we see that the closer we get to \(x=3\), the closer we get to \(0.5\). Therefore, as \(x\) approaches 3, \(f(x)\) approaches 0.5, hence \(\implies \boxed{\frac{1}{2}}\)
Hey guys!!
Need a little help with this!!
My questions are on the attachment..
All you can do is to do them as a Simplest Form..
Thanks if someone answer this!!
(≧▽≦)(ㆁωㆁ)

Answers
3. 1/2
4. 8/15
5. 1 1/8
\(\sf 2. \: \frac{7}{8} - \frac{4}{16}\)
\(\sf = \frac{7}{8} + \frac{- 4}{16}
\(\sf = \frac{7}{8} + \frac{- 1}{4}\)
\(\sf = \frac{7}{8} + \frac{- 2}{8} \)
\(\sf = \frac{7 + - 2}{8} \)
\(\sf = \frac{5}{8 }\)
\(\sf3.\)
\( \sf= \frac {1}{2} \)
\(\sf4.\)
\(\sf \frac{8}{15}\)
\(\sf5.\)
\(\sf= \frac{11}{8}\)
Becky and Meredith are selling wrapping paper for a school fundraiser. Customers can buy rolls of plain wrapping paper and rolls of shiny wrapping paper.
Becky sold 7 rolls of plain wrapping paper and 1 roll of shiny wrapping paper for a total of $39. Meredith sold 7 rolls of plain wrapping paper and 4 rolls of shiny wrapping paper for a total of $72.
What is the cost each of one roll of plain wrapping paper and one roll of shiny wrapping paper?
Answers
Answer:
111
Step-by-step explanation:
72+39+1=111
PLEASE HELP WILL GAVE BRAINLIEST AND 100 POINTS
Draw yourself a number line. Order the numbers from least to greatest: pi, -3, 49√
, 9√
Answers
Answer: -3, 9√, pi, 49√
Step-by-step explanation:
Having the check beside a number, ex: 49√, shows that something squared, in other words multiplied by itself, equals this. In this example it would be 7. For, 7*7=49. For 9√ it would be 3. Pi is 3.14. Listing these out we have:
-3, 3.14, 3, 7. Let's order this...
-3 is the least, then 3, then 3.14 (slightly bigger than 3), lastly, 7.
a jet airliner travels 1680 miles in 3 hours with a tail wind. the return trip, into the wind, takes 3.5 hours. write a system of equations whose solution is the ground speed of the airplane and the wind speed.
Answers
A system of equation is 1680mi3hr = p−w×600×2hr -p + w for a jet airliner travels 1680 miles in 3hours with a tail wind.
Let p be the speed of the jet airliner
and w be the speed of the tail wind
It takes the plane 3 hours to go 1680 miles when a jet airliner travels with a tail wind and and 3.5 hours to go 1680 miles against the wind.. So, using system of equations we get
1680mi3hr = p−w×600×2hr = p + w
Solving for the left sides we get:
200mph = p - w
300mph = p + w
Now solve for one variable in either equation, we use
200mph = p - w
Add w to both sides:
p = 200mph + w
Using the value of x, we can found the value of w using system of equations.
300mph = (200mph + w) + w
Combine like terms:
300mph = 200mph + 2w
Subtract 200mph on both sides:
100mph = 2w
On dividing by 2:
50mph = w
So the speed of the tail wind is 50mph.
Therefore, 200mph = p - 50mph
Add 50mph on both sides:
250mph = p
Hence, speed of jet airliner is 250mph.
To know more about System of equation, visit here:
https://brainly.com/question/21620502
#SPJ4
someone please help me with the perimeter and area of this problem

Answers
Answer: Perimeter = 40
Area = 204
Step-by-step explanation:
Perimeter is the sum of all the sides:
11 + 12 + 17 = 40
The area of a triangle is 1/2 base x height
1/2( 17 x 24)
1/2( 408) = 204
need assistance with algebra 2

Answers
Answer:
A. Quadratic, degree 2
Step-by-step explanation:
The degree is found by simply finding the term with the power of, that is the highest. Which would be 2x^2. It is raised to the 2nd power, so the degree is 2.
given that p(a ∩ d)=2/5, find p(~ (a ∩ d)).
Answers
The probability of the event "~ (a ∩ d)", or the probability that either "a" OR "d" does not occur, is 3/5.
The symbol "∩" represents the intersection of two events.
For example, "a ∩ d" means the event where both "a" and "d" occur together.
The symbol "~" means "not" or "complement." So, "~ (a ∩ d)" means the event where "a ∩ d" does NOT occur, or in other words, where "a" OR "d" does not occur.
Now, onto the question.
We are given that p(a ∩ d) = 2/5, which means that the probability of both events "a" and "d" occurring together is 2/5.
We want to find the probability of the event "~ (a ∩ d)", or the probability that either "a" OR "d" does not occur.
To find this probability, we need to use some basic probability rules.
One of these rules is that the probability of an event happening (let's call it "E") is equal to 1 minus the probability of the complement of that event not happening (~E).
In other words, p(E) = 1 - p(~E).
So, if we apply this rule to the event "~ (a ∩ d)", we get:
p(~ (a ∩ d)) = 1 - p(a ∩ d)
Now we can substitute in the value we were given for p(a ∩ d):
p(~ (a ∩ d)) = 1 - 2/5
Simplifying this gives:
p(~ (a ∩ d)) = 3/5
Therefore, the probability of the event "~ (a ∩ d)", or the probability that either "a" OR "d" does not occur, is 3/5.
Know more about probability here:
https://brainly.com/question/251701
#SPJ11
help me with this please!!!!!

Answers
Answer:
Step-by-step explanation:
Ok: So A is similar to B put different ratios
1. Look at sides
A has one side 12cm while B has the same side 9 cm
9:12 is the ratio or 9/12= 3/4
Sides B is 3/4 of A
2. Caculate
W= 3/4*7=
w=21/4cm
or
w= 5 1/4 cm