. In 1940 the average size of a U.S. farm was 174 acres. The standard deviation was 45 acres. Define the random variable x in words. X = _________.
Answers
Answer:
232343
Step-by-step explanation:
4343
Answer:
its 102002i45-08-5983-958y7 jnskp;xjgbna;xjgna['hg[ohj who cares about you
Step-by-step explanation:
Related Questions
Question The following frequency table summarizes a set of data. What is the five-number?

Answers
We can see the following given data table:
And from this table, we have to find the five-number summary.
The five-number summary gives us:
• The minimum value for the data set
,• The first quartile (Q1): this is a value for which 25% of the observations are below it, and 75% of the observations are above it.
,• The median (50% of the observations are above (and below) this value.
,• The third quartile (Q3): this is a value for which 75% of the observations are below it, and 25% of the observations are above it
,• The maximum value for the data set
Then to find those numbers, we can proceed as follows:
1. We have to rewrite the information from the data as a list, and we have to take into account the frequencies for each observation:
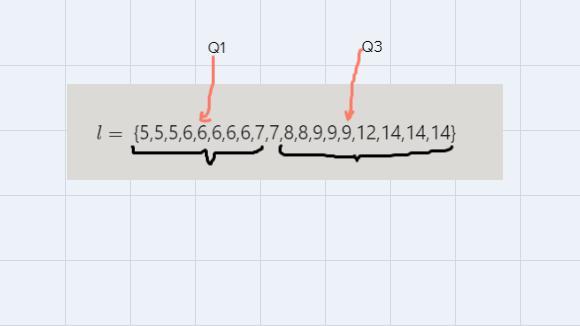
\(\begin{gathered} l=\text{ \textbraceleft5,5,5,6,6,6,6,6,7,7,8,8,9,9,9,12,14,14,14\textbraceright} \\ \end{gathered}\)And we already have the observations ordered in ascending order. The total of observations is 19 cases.
2. Now we can find the minimum and the maximum values as follows:
• Minimum value = 5
,• Maximum value = 14
3. We have to find the median as follows:
Since we have that the total number of observations is 19, and this is an odd number, then the median is the middle value. In this case, below the median, we have 9 values, and above it, 9 values too.
Therefore, the median is equal to 7.
4. Find the first quartile (Q1) and the third quartile (Q3)
The first quartile, roughly speaking, is the median for the first half of the values, and we can say that the third quartile is the median for the second half of the values. Then we have:
We use here the Method of Moore and McCabe in which we do not include the median in finding both values.
Therefore, Q1 = 6, and Q3 = 9.
Therefore, in summary, the five-number summary can be written as follows:
Min = 5
Q1 =


Please help thank uuuuu

Answers
Answer:
12
Step-by-step explanation:
You simply add the coefficients together.
3 + 4 + 5 = 12
Find the third iterate x3 of f(x) = 2x + 3
for an initial value of x0 = 2
a. 7
b. 15
c. 17
d. 37
Answers
For the function f(x) = 2x + 3 the third iterate x₃ is 37
To find the third iterate, x3, of the function f(x) = 2x + 3, given an initial value of x₀ = 2,
we can apply the function repeatedly.
Starting with x₀ = 2:
x₁ = f(x₀)
= 2(2) + 3
= 7
x₂ = f(x₁)
= 2(7) + 3 = 17
x₃ = f(x₂)
= 2(17) + 3
= 37
Therefore, the third iterate x₃ is 37.
To learn more on Functions click:
https://brainly.com/question/30721594
#SPJ1
help with horizontal asymptotes


Answers
Answer:
y = 3. last option is correct answer for 2nd question.
Step-by-step explanation:
f(x) just means y.
horizontal asymptote is y = 'something.'
the graph crosses y = 4, so y = 4 cannot be asymptote.
y = 3 is since the graph never quite touches it.
as for ∞, we see that as x approaches -∞, y almost reaches 3. but not quite. also, as x approaches +∞, y approaches +∞.
so the last option is the correct answer.
Determine the number of compounding periods for the following investment
Principal: $1832
Future value $2193
Interest rate 8.1%
Frequency of conversion monthly
Answers
Answer: There is 1 compounding period for this investment.
Step-by-step explanation: To determine the number of compounding periods, we can use the following formula:
n = (t / p) * m
where:
n = number of compounding periods
t = time period in years
p = payment frequency per year
m = compounding frequency per payment period
In this case, we have:
Principal = $1832
Future value = $2193
Interest rate = 8.1%
Payment frequency = 12 (monthly)
First, let's calculate the time period in years:
t = 1 year / 12 months = 0.0833 years
Next, let's determine the compounding frequency per payment period:
m = 12 (since interest is compounded monthly)
Now we can calculate the number of compounding periods:
n = (t / p) * m = (0.0833 / 1) * 12 = 1
Therefore, there is 1 compounding period for this investment.
To find the number of compounding periods, we can use the formula:
n = (t x f)
where:
n = number of compounding periods
t = time in years
f = frequency of conversion
We are given that the principal is $1832, the future value is $2193, the interest rate is 8.1%, and the frequency of conversion is monthly. We need to find the time in years.
To find the time in years, we can use the formula:
FV = PV x (1 + r/n)^(nt)
where:
FV = future value
PV = present value
r = interest rate
n = number of compounding periods per year
t = time in years
Substituting the given values, we get:
$2193 = $1832 x (1 + 0.081/12)^(12t)
Simplifying this equation, we get:
1.1971^(12t) = 1.1971
Taking the natural logarithm of both sides, we get:
12t x ln(1.1971) = ln(1.1971)
Solving for t, we get:
t = ln(1.1971) / (12 x ln(1.1971))
t = 3 years
Now that we know the time in years, we can find the number of compounding periods:
n = (t x f)
n = (3 x 12)
n = 36
Therefore, the number of compounding periods is 36.
Does the line appear to be a line of
symmetry of the triangle? Explain.
Please and thank you

Answers
Answer:
No, the two sides are not congruent.
I flipped my whole laptop around to check loll
. Write an expression equivalent to 3w - 3w + 3w with just one term. - What property or properties applies?
Answers
Answer:
w(3-3+3)
Step-by-step explanation:
distibutitve property
select all that are integers
9, -17, 1/4, 0, -3.6
Answers
Answer:
9,-17,0
Step-by-step explanation:
An integer is a whole number
What is the meaning of "\( \varphi (x,y)\) be \( y\wedge \phi (x)\) "?
![What is the meaning of "[tex] \varphi (x,y)[/tex] be [tex] y\wedge \phi (x)[/tex] "?](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/qBCO5ypO9m13MpdV66LMvZB6FUp8SIQM.png)
Answers
The reasoning presented lacks explicit explanations and logical connections between the steps, making it difficult to fully understand the intended proof strategy.
The given proof aims to show that the Separation Axioms can be derived from the Replacement Schema using a particular construction involving a formula p(x, y). Let's analyze the proof step by step:
Define the formula p(x, y) as x = yo(x).
This formula states that for each x, y pair, x is equal to the unique object y such that y is obtained by applying the operation o to x.
Define the set F as {(x, x) (x)}.
This set F contains pairs (x, x) where x is the unique object obtained by applying the operation (x) to x.
Claim: F(X) = {y (x = X)p(x, y)} = {y: (x = X)x = y^o(x)} = {x: (3x € X)o(x)} = {x X: (x)}.
This claim asserts that F(X) is equivalent to {y (x = X)p(x, y)}, which is further equivalent to {y: (x = X)x = y^o(x)}, and so on.
The proof states that since (x, y) satisfies the functional formula VaVyVz(p(x, y)^(x, z) y = z), it follows that (x, y) is a functional formula.This step emphasizes that the formula p(x, y) satisfies certain properties that make it a functional formula, which is relevant for the subsequent deductions.
Finally, the proof concludes that the Separation Axioms follow from the Replacement Schema, based on the previous steps.
For more such questions on explicit
https://brainly.com/question/30394385
#SPJ8
What are the main properties of a parallelogram?
use the parallelogram (ABEF) to help you

Answers
Answer:
It must be number 2.
1 and 3 number is wrong as parallelogram don't need have right angle
A coffee place is selling coffees for $2.50 each and cappuccinos for $3.75 each.
Today the coffee place sold a total of 70 drinks (coffees and cappuccinos) for a total of $222.50.
a) Write an equation that represents the information.
b) Solve the equation in (a) to find how many coffees and how many cappuccinos the coffee place sold today.
Answers
Answer:
Step-by-step explanation:
a) Let's denote the number of coffees sold as 'x' and the number of cappuccinos sold as 'y'.
The equation that represents the given information is:
2.50x + 3.75y = 222.50
b) To solve the equation, we need to find the values of 'x' and 'y' that satisfy the equation.
Since we have two variables and only one equation, we cannot determine the exact values of 'x' and 'y' independently. However, we can find possible combinations that satisfy the equation.
Let's proceed by assuming values for one of the variables and solving for the other. For example, let's assume 'x' is 40 (number of coffees):
2.50(40) + 3.75y = 222.50
100 + 3.75y = 222.50
3.75y = 222.50 - 100
3.75y = 122.50
y = 122.50 / 3.75
y ≈ 32.67
In this case, assuming 40 coffees were sold, we get approximately 32.67 cappuccinos.
We can also assume different values for 'x' and solve for 'y' to find other possible combinations. However, keep in mind that the number of drinks sold should be a whole number since it cannot be fractional.
Therefore, one possible combination could be around 40 coffees and 33 cappuccinos sold.
he polynomial of degree 5, P ( x ) has leading coefficient 1, has roots of multiplicity 2 at x = 3 and x = 0 , and a root of multiplicity 1 at x = − 1 Find a possible formula for P ( x ) .
f]
Answers
The possible formula for the polynomial in discuss whose roots are described as; having roots of multiplicity 2 at x = 3 and x = 0 , and a root of multiplicity 1 at x = − 1 is; P(x) = x^5 -5x⁴-6x³+18x².
What is the polynomial in discuss whose roots and leading coefficient are as discussed?The polynomial which is as described in the task content whose roots are as given can be written in its factorised form as follows;
P(x) = (x-3) (x-3) (x) (x) (x+1)
The expanded form is therefore;
P(x) = x^5 - 5x⁴- 6x³+ 18x².
Therefore, the polynomial having roots of multiplicity 2 at x = 3 and x = 0 , and a root of multiplicity 1 at x = − 1 is P(x) = x^5 - 5x⁴- 6x³+ 18x².
Read more on polynomials;
https://brainly.com/question/13793580
#SPJ1
The dimensions of a cylinder are shown in the diagram
Round to the nearest whole number , what is the total surface area of the cylinder in cubic centimeters

Answers
Answer:
S = 2π(3^2) + 2π(3)(8.2) = 67.2π = 211 cm^3
HELPPPPPPPPPPPPPPPPPPPPPPPPPPPPPPPPPPPPPP

Answers
c = 8
d = -5
3 (c + d) [Substitute the values of c & d]
= 3 (8 - 5)
= 3 - 3
= 0
Hope it helps!
꧁✿ ᴿᴬᴵᴺᴮᴼᵂˢᴬᴸᵀ2222 ✬꧂
Answer:
9
Step-by-step explanation:
3(8+(-5))=3 X (8-5)
=3x3
=9
what is the largest of 3 consecutive positive integers if the product of the two is smaller integers is 8 more times the largest integer
Answers
The three successive positive numbers are 3, 4, and 5.
The largest of these 5.
What are integers?Integers are made up of zeros, natural numbers, and their additive inverses. Except for the fractional part, it can be represented on a number line.
Let's call the smallest of three successive positive numbers x. The following two consecutive integers would then be x + 1 and x + 2.
The product of the two smaller integers (x and x + 1) is eight times that of the largest integer (x + 2). This can be written as an equation:
x(x + 1) = 8 + (x + 2)
By enlarging and simplifying the left side, we get:
\(x^2 + x = x + 10\)
When we subtract x and 10 from both sides, we get:
\(x^2 - 9 = 0\)
After factoring in, we get:
(x - 3)(x + 3) = 0
As a result, x = 3 or x = -3. We can disregard the negative solution because we're seeking positive integers and infer that x = 3.
As a result, the three successive positive numbers are 3, 4, and 5.
The largest of these 5.
Learn more about integers here:
https://brainly.com/question/15276410
#SPJ1
Write an equation to represent the measures of the angle

Answers
139°+x=180°
Subtract 139° from both sides
X=180°-139°
X=41°
find the measure of a complementary angle, a supplementary angle, and a vertical angle for the following measures:• m(
Answers
Supplementary angles are those that when summed, add up 180°. Two angles are complementary when they add up 90°.
Vertical angles are opposed by the vertex when two lines intersect each other. Vertical angles have always the same measure.
Now, following that logic, we can evaluate the two options.
1. m(∠A) = 45:
A supplementary angle will sum 180° when added to 45. Let's call that supplementary angle S1:
\(\begin{gathered} 45^o+m(\angle S_1)=180^o \\ m(\angle S_1)=180^o-45^o \\ m(\angle S_1)=135^o \end{gathered}\)The measure of a supplementary angle will be 135°.
Similarly, let's call that complementary angle C1:
\(\begin{gathered} 45^o+m(\angle C_1)=90^o \\ m(\angle C_1)=90^o-45^o \\ m(\angle C_1)=45^o \end{gathered}\)The measure of a complementary angle will be also 45°.
For the vertical angle, we stated that vertical angles are equal; then it will also be 45°.
For the second option we follow exactly the same process:
1. m(∠A) = 45:
A supplementary angle S2:
\(\begin{gathered} w^o+m(\angle S_2)=180^o \\ m(\angle S_2)=180^o-w^o \end{gathered}\)180° - w° will be a supplementary angle.
A complementary angle C2:
\(\begin{gathered} w^o+m(\angle C_2)=90^o \\ m(\angle C_2)=90^o-w^o \end{gathered}\)90° - w° will be a complementary angle.
And for the vertical angle, just like before, it will have exactly the same measure: w°.
Statement: If an angle's measure is 20°, then it is not obtuse.
What is the converse of the following statement?
A
If an angle is not obtuse, then its measure is 20°.
O
B
If an angle is obtuse, then its measure is not 20°.
O
C
If an angle's measure is not 20°, then it is obtuse.
D
If an angle is not obtuse, then its measure is not
O
20°.
Answers
Answer:
Samantha has 8 quarts of milk. The ratio of quarts to gallons is 4:1. How many gallons of milk does Samantha have? x a. 1 over 32 x b. begin mathsize 16px style 1 half end style x c. 2 x d. 32
Step-by-step explanation:Samantha has 8 quarts of milk. The ratio of quarts to gallons is 4:1. How many gallons of milk does Samantha have? x a. 1 over 32 x b. begin mathsize 16px style 1 half end style x c. 2 x d. 32vSamantha has 8 quarts of milk. The ratio of quarts to gallons is 4:1. How many gallons of milk does Samantha have? x a. 1 over 32 x b. begin mathsize 16px style 1 half end style x c. 2 x d. 32Samantha has 8 quarts of milk. The ratio of quarts to gallons is 4:1. How many gallons of milk does Samantha have? x a. 1 over 32 x b. begin mathsize 16px style 1 half end style x c. 2 x d. 32
7 cameras cost $308. The cost of each camera is the same. What is the cost of each camera?
Answers
Answer:
$44
Step-by-step explanation:
Hello!
It said that 7 cameras cost $308, right?
If each camera costs the same, it means that 7 cameras with the same value equal $308.
If we use this in an equation, and if x is a variable we want to solve, it would be:
7x = 308.
If 7x = 308, it would be
308/7 =
44.
So, $44 is the answer.
(q1) Find the length of the curve described by the function

Answers
The value of the Integral at the lower limit from the value of the integral at the upper limit to get the length of the curve.
The length of the curve described by the function f(x) = 1 + 3x^2 + 2x^3 is to be found. The formula used to find the length of a curve is:
L = ∫(sqrt(1 + [f'(x)]^2))dx where f'(x) is the derivative of f(x)We have to first find f'(x):f(x) = 1 + 3x^2 + 2x^3f'(x) = 6x + 6x^2
The integral becomes:L = ∫(sqrt(1 + [6x + 6x^2]^2))dx = ∫(sqrt(1 + 36x^2 + 72x^3 + 36x^4))dx The integral appears to be difficult to evaluate by hand.
Therefore, we use software like Mathematica or Wolfram Alpha to solve the problem. Integrating the expression using Wolfram Alpha gives:
L = 1/54(9sqrt(10)arcsinh(3xsqrt(2/5)) + 2sqrt(5)(2x^2 + 3x)sqrt(9x^2 + 4))The limits of integration are not given. Therefore, the definite integral be solved.
We can, however, find a general solution. We use the above formula and substitute the limits of integration.
Then, we subtract the value of the integral at the lower limit from the value of the integral at the upper limit to get the length of the curve.
To know more about Integral.
https://brainly.com/question/27419605
#SPJ8
just look at the picture to answer it

Answers
The areas of rectangles D, E and F are 24p units², 14m units² and (15x + 40) units² respectively
How to find the areas of the rectangles?Given: rectangles D, E and F as labeled
For rectangle D, the length will be the addition of the four p's
length(L) = p + p + p + p = 4p
width (W) = 6
Area = L × W
Area = 4p × 6 = 24p units²
For rectangle E:
It should be noted that the length will be the addition of 6 and 8. The width is also represented by m.
length(L) = 6 + 8 = 14
width (W) = m
Area = L × W
Area = 14 × m = 14m units²
For rectangle F:
length(L) = 3x + 8
width (W) = 5
Area = L × W
Area = (3x + 8) × 5 = (15x + 40) units²
Learn more about area of rectangle on:
https://brainly.com/question/25292087
#SPJ1
help pls now points are 30

Answers
Answer:
4 AU
Step-by-step explanation:
20.8 ÷ 5.2 = 4
5.2 increased by 4.
Scale means to get bigger or smaller evenly.
-kiniwih426
Jolene invests her savings in two bank accounts, one paying 3 percent and the other paying 9 percent simple
interest per year. She puts twice as much in the lower-yielding account because it is less risky. Her annual
interest is 3120 dollars. How much did she invest at each rate?
Amount invested at 3 percent interest is $____
Amount invested at 9 percent interest is $___
Answers
Let's denote the amount Jolene invested at 3 percent interest as 'x' dollars. Since she put twice as much in the lower-yielding account, the amount she invested at 9 percent interest would be '2x' dollars.
To calculate the interest earned from each account, we'll use the formula: Interest = Principal × Rate × Time.
For the 3 percent interest account:
Interest_3_percent = x × 0.03
For the 9 percent interest account:
Interest_9_percent = 2x × 0.09
We know that the total annual interest is $3120, so we can set up the equation:
Interest_3_percent + Interest_9_percent = 3120
Substituting the above equations, we have:
x × 0.03 + 2x × 0.09 = 3120
Simplifying the equation:
0.03x + 0.18x = 3120
0.21x = 3120
Dividing both sides of the equation by 0.21:
x = 3120 / 0.21
x = 14857.14
Therefore, Jolene invested approximately $14,857.14 at 3 percent interest and twice that amount, $29,714.29, at 9 percent interest.
Answer:
Step-by-step explanation:
X is the amount invested at 6%
Y is the amount invested at 9%
0.06X + 0.09Y = 4998
X = 2Y
0.06(2Y) + 0.09Y = 4998
.12Y + 0.09Y = 4998
0.21Y = 4998
21Y = 499800
Y = 499800/21 = 23800
So X = 2*23800 = 47600
$47,600 is invested at 6% and $23800 is invested at 9%
A group of friends wants to go to the amusement park. They have $100 to spend on
parking and admission. Parking is $13, and tickets cost $14.50 per person, including
tax. How many people can go to the amusement park?
Answers
Answer:
3
Step-by-step explanation:
14.50 + 13.00 = 27.50
27.50 x 3 = 82.50
Answer:
6
Step-by-step explanation:
\color{red}{14.5}x+\color{blue}{13}=
14.5x+13=
\,\,\color{purple}{100}
100
-\color{blue}{13}\phantom{=}
−13=
\,\,-\color{blue}{13}
−13
Subtract 13 from both sides
\color{red}{14.5}x=
14.5x=
\,\,87
87
\frac{\color{red}{14.5}x}{\color{red}{14.5}}=
14.5
14.5x
=
\,\,\frac{87}{\color{red}{14.5}}
14.5
87
Divide both sides by 14.5
x=
x=
\,\,\boxed{6}
6
\text{6 people can go to the amusement park.}
6 people can go to the amusement park.
14 points!! PLEASE HELP MARKING BRAINLIST

Answers
Answer:
x = 58.03°
Step-by-step explanation:
Given:
17 cm is the length of the hypotenuse9 cm is the length of the adjacent sideSolve for x:
cos x = adjacent / hypotenusex = \(cos^{-1}(\frac{adjacent}{hypotenuse})\)1. \(x=cos^{-1}(\frac{9}{17})=58.03\)
Answer:
So, the measure of angle x is equal to 58.03.
HELP PLEASE DONT HAVE MUCH TIME

Answers
Answer:
4.8*10^7
Step-by-step explanation: You chose the right answer. Hint plug it exactly like it is in the calculator and change your mode to scientific notation. That’s what I did
Can you explain to me how did we get the answer

Answers
The end behavior of the polynomials are as follows;
(a) y = x³ - 9·x² + 8·x - 14
End behavior; y → ∞ as x → ∞
\({}\) y → -∞ as x → -∞
(b) y = -8·x⁴ + 13·x + 800
End behavior; y → -∞ as x → -∞
\({}\) y → -∞ as x → ∞
What is the end behavior of a a polynomial?The end behavior of a polynomial is the characteristics of the graph of the polynomial as the input (x-values), tends to plus and minus infinity.
The factors that effect the end behavior of a polynomial are;
The degree of the polynomial, (even or odd)
The sign of the leading coefficient of the polynomial (positive or negative)
The leading coefficient is the coefficient of the term with the highest degree.
(a) The polynomial, function, y = x³ - 9·x² + 8·x - 14
The specified polynomial is a third degree polynomial, with a positive leading coefficient of 1, the end behavior is therefore;
y tends to positive infinity as x tends to positive infinity
y tends to negative infinity as x tends to negative infinity
End behavior;
y → ∞ as x → ∞
y → -∞ as x → -∞
(b) The polynomial function can be expressed as follows;
y = -8·x⁴ + 13·x + 800
The above polynomial of degree 4 is an even degree polynomial
The leading coefficient of the polynomial is -8, therefore, the leading coefficient is negative
The shape of the graph of the polynomial is therefore ∩ shaped, such that the end behavior is as follows;
y-values approaches negative infinity as x approaches negative infinity
y-values approaches negative infinity as x approaches positive infinity
End behavior;
y → -∞ as x → -∞
y → -∞ as x → ∞
Learn more on the end behavior of polynomials here: https://brainly.com/question/8245713
#SPJ1
determine whether the following graphs are functions using the vertical line test. what is the vertical line test?

Answers
The vertical line test is a method that is used to determine whether a given relation is a function or not. The approach is rather simple. Draw a vertical line cutting through the graph of the relation, and then observe the points of intersection.
The vertical line test supports the definition of a function. That is, every x-value of a function must be paired to a single yy-value. If we think of a vertical line as an infinite set of x-values, then intersecting the graph of a relation at exactly one point by a vertical line implies that a single x-value is only paired to a unique value of y.
If a vertical line intersects the graph in all places at exactly one point, then the relation is a function.
If a vertical line intersects the graph in some places at more than one point, then the relation is NOT a function.
With the above illustration,
Graph 3 is not a function using vertical test because it has infinitely many solutions
Graph 4 is not a function because the vertical cuts the graph and intersect at a points
Graph 5 is a constant function because it has a repeated value of x and a constant y value
Graph 6 is not a function because the x-values which is the domain is repeated for different values of y
Graph 7 is not a function because the vertical line cuts the graph at two-point
Graph 8 is a function because it has one point intersection and each value of x have a corresponding value of y




-Ꮞk +1 = 1+k + 3k
What is this answer I need it quick thanks
Answers
Answer:
0
Step-by-step explanation:
Step 1:
- 4k + 1 = 1 + k + 3k Equation
Step 2:
- 4k + 1 = 1 + 4k Add
Step 3:
1 = 1 + 8k Add 4k on both sides
Step 4:
0 = 8k Subtract 1 on both sides
Answer:
k = 0
Hope This Helps :)
I need the answers for the table below.

Answers
The values of f(x) for the given x - values rounded to 4 decimal places are 0.0078, 0.0078, 0.0020, 0.0020, 0.0019 and 0.0013 respectively
Given the function :
tan(πx)/7xSubstitute the given value of x to obtain the corresponding f(x) values :
x = -0.6
f(x) = (tanπ(-0.6))/7(-0.6) = 0.0078358
x = -0.51
f(x) = (tanπ(-0.51))/7(-0.51) = 0.0078350
x = -0.501
f(x) = (tanπ(-0.501))/7(-0.501) = 0.001967
x = -0.5
f(x) = (tanπ(-0.5))/7(-0.5) = 0.001959
x = -0.4999
f(x) = (tanπ(-0.4999))/7(-0.4999) = 0.001958
x = 0.499
f(x) = (tanπ(-0.499))/7(-0.499) = 0.001951
x = -0.49
f(x) = (tanπ(-0.49))/7(-0.49) = 0.00188
x = -0.4
f(x) = (tanπ(-0.4))/7(-0.4) = 0.00125
Therefore, values which complete the table are 0.0078, 0.0078, 0.0020, 0.0020, 0.0019 and 0.0013
Learn more on functions : https://brainly.com/question/12634120
#SPJ1