Let X be a topological space and A ⊂ X. The closure of A, denoted
A¯, is the intersection of all closed sets containing A.
(a) Show that A¯ is the smallest closed subset of X containing A, in
the following sense: if A ⊂ F ⊂ X and F is closed, then A¯ ⊂ F.
Answers
The "closure(A⁻)" of "subset-A" in a topological-space X is the intersection of all "closed-sets" containing A. It is smallest closed subset of X that contains A, means that if A⊂F⊂X and F is closed, then A⁻ is a subset of F.
In order to show that the closure(A⁻) is the smallest closed subset of X which contains "A", we need to prove that if A ⊂ F ⊂ X and F is closed, then A⁻ ⊂ F,
We know that, A⁻ is intersection of all "closed-sets" containing A. Since F is "closed-set" which contains "A", it is one of the sets in the intersection defining A⁻. So, A⁻ is contained in F.
To show that A⁻ ⊂ F, we consider an arbitrary point x ∈ A⁻, which means that x is in the intersection of all closed sets containing A. Since, F is one such "closed-set" containing A, x must be in F as well.
Since x was an "arbitrary-point" in A⁻, we have shown that every point in A⁻ is also in F, So, A⁻ ⊂ F.
Therefore, we have proved that if A ⊂ F ⊂ X and F is closed, then A¯ ⊂ F.
Learn more about Topological Space here
https://brainly.com/question/31392876
#SPJ4
Related Questions
What is the sign of the product (–7)(–2)(–5)(1)?
Answers
Answer:
Negative
Step-by-step explanation:
When an even number of negatives are multiplied together, the product will be positive (because two negatives cancel out). When an odd number of negatives are multiplied together, the product will be negative.
In the given expression, there is also a singular (1) at the end, which we can ignore since anything multiplied by 1 is itself. The other numbers are all negative and there are 3 of them, and since 3 is an odd number, the product will be negative.
I hope this helps!
Answer:
The third choice
Step-by-step explanation:
Negative, because the product (–7)(–2) is positive, and the product (–5)(1) is negative and the product of a positive and a negative number is negative,
pls mark as brainliest hope you have a nice day!
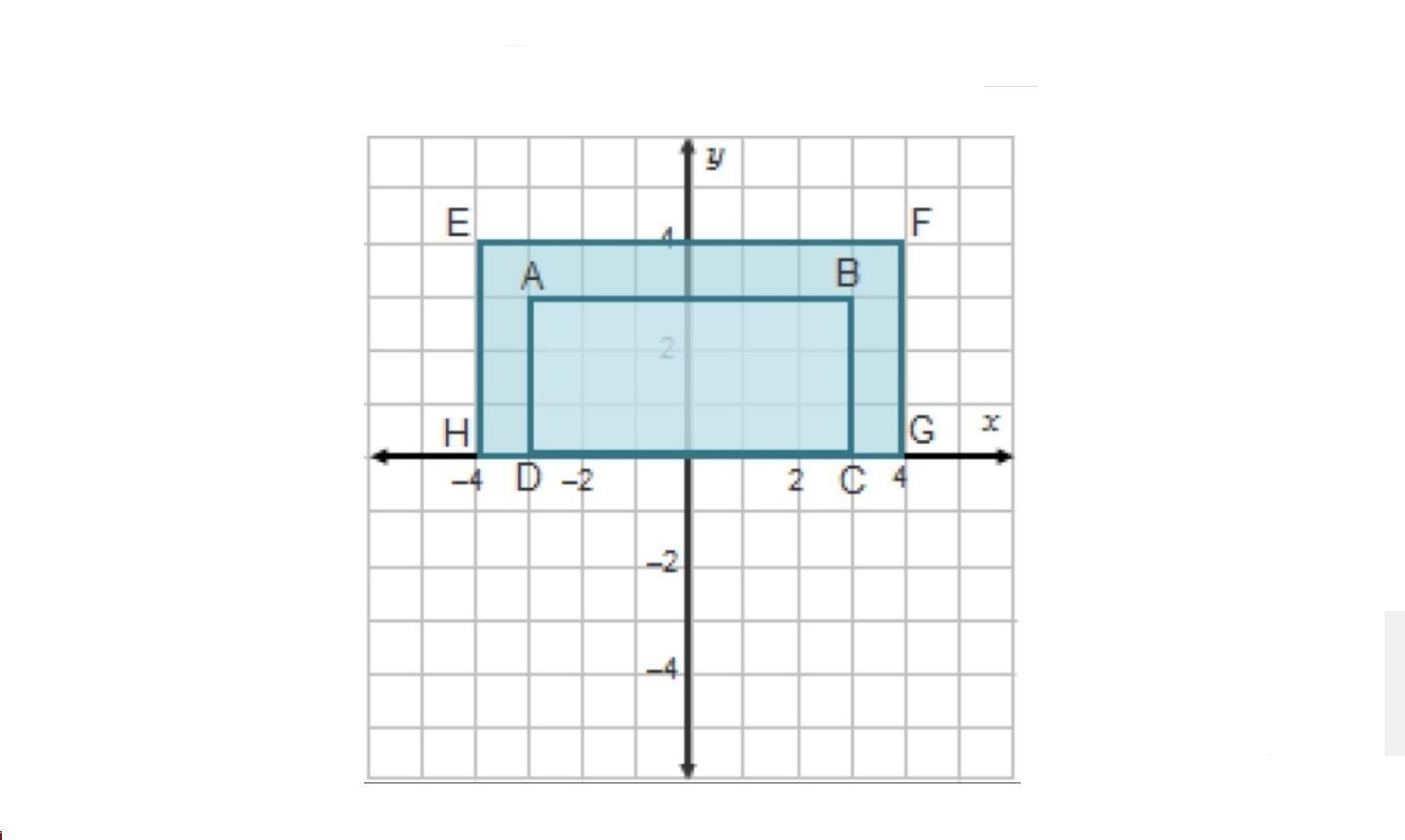
Is rectangle EFGH the result of a dilation of rectangle ABCD with a center of dilation at the origin
Answers
Yes, because both figures are rectangles and all rectangles are similar. Option B is correct option.
According to the statement
We have given that the two rectangles EFGH and ABCD and we have show that the these rectangles Are dilation at origin or not.
So, According to the given diagram
Yes, the rectangle EFGH is a result of dilation of rectangle ABCD with a center of dilation of rectangle at the origin.
Also The scale factor of the dilation is greater than one as the image is bigger than the pre-image i.e. there is a stretch.
The scale factor could be calculated by the ratio of the sides of the image to the pre-image rectangle.
According to the diagram In Rectangle ABCD:
Its vertices have coordinates A(-3,3), B(3,3), C(3,0) and D(-3,0).
Now consider rectangle EFGH:
Its vertices have coordinates E(-4,4), F(4,4), G(4,0) and H(-4,0).
Hence the scale factor is becomes from these values is:
EF/AB = EH/AD = FG/BC = HG/DC = 4/3.
Hence the scale factor becomes 4/3.
Also
∠A=∠B=∠C=∠D=∠E=∠F=∠G=∠H=90°
( When a shape is dilated the two shapes are similar.And similar shapes have equal interior angles , corresponding sides are proportional ).
So, Yes, because both figures are rectangles and all rectangles are similar. Option B is correct option.
Learn more about center of dilation of rectangle here https://brainly.com/question/17986580
#SPJ4
The width of a rectangle is 5 feet less than the length. The perimeter is 62. Find the length and width of the rectangle.
Variables: ___________________________
Model equation: ______________________________
Length of the rectangle: ______________________________
Width of the rectangle: ______________________________
Answers
Perimeter formula: p = 2(w + l)
The perimeter is 62.
p = 62
The width of a rectangle is 5 feet less than the length.
w = l - 5
Substitute and solve.
62 = 2[(l - 5) + l)]
62 = 2l + 2l - 10
62 = 4l - 10
72 = 4l
18 = l
Substitute and solve for the width
w = 18 - 5
w = 13
Therefore, the length is 18 and the width is 13.
Best of Luck!
Answer:
see below and attached.
Step-by-step explanation:
Perimeter (P) = 2 L (Length) + 2 W (Width)
where P = 62
W = L - 5
P = 2L + 2W
62 = 2L + 2(L -5)
62 = 2L + 2L - 10
72 = 4L
L = 72/4
L = 18
W = L - 5
W = 18 - 5
W = 15
Variables : P, L, W
Model equation: P = 2L + 2W
Length of the rectangle: 18
Width of the rectangle: 13

Find the sum of the interior angles for a hexagon. 540° 720° 900° 1,080°
Answers
The sum of interior Angles of Hexagon is always 720°
Any hexagon's internal angles add up to 720° in all cases. By dividing 720° by 6, we may get the size of each interior angle of a regular hexagon. As a result, we have:
720°÷6 = 120°
In a regular hexagon, each inside angle is 120°.
An example of a regular hexagon with equal-length sides and angles is shown in the diagram below. By multiplying the six 120° angles together, we can demonstrate that the result is 720°.
Therefore, the interior angles of a hexagon are always added up to 720°.
Learn more about hexagon :
brainly.com/question/3295271
#SPJ4

what is the distance in units between point A(5,-8) and point B(-2,4 on the coordinate plane
Answers
Answer:
13.89
Step-by-step explanation:
Answer:13
Step-by-step explanation:
Can someone message me I need help ASAP by tmr
Answers
What is the minimum order of the Taylor polynomial centered at 0 for cos x required to approximate the following quantity with an absolute error no greater than 10 -5?cos (-0.34)The minimum order of the Taylor polynomial is n =
Answers
1. The Taylor polynomial at x = -0.34. 10^-5 ≤ |Pn(-0.34)| ≤ 0.34^n / n!
2. The minimum order of the Taylor polynomial is n = 4.
1. To find the minimum order of the Taylor polynomial centered at 0 for cos(x) required to approximate cos(-0.34) with an absolute error no greater than 10^-5, we can use the error bound formula for Taylor polynomials.
The error bound formula states that for a function f(x) and its nth-degree Taylor polynomial Pn(x) centered at a, the absolute error between f(x) and Pn(x) is bounded by:
|f(x) - Pn(x)| ≤ M * |x - a|^n / (n!)
where M is the maximum value of the (n+1)th derivative of f(x) on the interval of interest.
For cos(x), the maximum value of the (n+1)th derivative is 1 for all n.
Let's solve the inequality:
10^-5 ≤ |cos(-0.34) - Pn(-0.34)| ≤ |0 - Pn(-0.34)|
Since cos(0) = 1, we only need to consider the error of the Taylor polynomial at x = -0.34.
10^-5 ≤ |Pn(-0.34)| ≤ 0.34^n / n!
2. To find the minimum value of n, we can start with a small value of n and increase it until the right side of the inequality is less than or equal to 10^-5.
Let's calculate the right side for n = 1, 2, 3, ...
For n = 1: 0.34^1 / 1! ≈ 0.34
For n = 2: 0.34^2 / 2! ≈ 0.057
For n = 3: 0.34^3 / 3! ≈ 0.006
For n = 4: 0.34^4 / 4! ≈ 0.001
We can see that for n = 4, the right side is less than 10^-5.
Therefore, the minimum order of the Taylor polynomial is n = 4.
So, the answer is n = 4.
To learn more about Taylor polynomial
https://brainly.com/question/15581839
#SPJ11
if you work 14 hours a week but have 6 hour shifts how many days would you work
Answers
The correct answer is 3.
A multiple-choice quiz has 5 questions. Each question has 3 possible answers. A student guesses the answer to each question. Find the probability that the student answers exactly 1 question correctly.
Answers
The probability that the student answers exactly 1 question correctly is
\($\frac{135}{243}=\boxed{\frac{5}{9}}$\)
What is the binomial coefficient?
In combinatorics, the binomial coefficient is used to denote the number of possible ways to choose a subset of objects of a given numerosity from a larger set.
There are a total of \($3^5=243$\) possible ways that the student can answer the quiz. To count the number of ways that the student can answer exactly 1 question correctly, we can use the binomial coefficient formula:
\(\binom{5}{1}=5\)
This counts the number of ways that the student can choose which 1 question to answer correctly.
For each of these 5 ways, there are 3 ways to choose the incorrect answer for each of the other 4 questions, so the total number of ways that the student can answer exactly 1 question correctly is
\($5\cdot 3^4=135$\).
Therefore, the probability that the student answers exactly 1 question correctly is
\($\frac{135}{243}=\boxed{\frac{5}{9}}$\)
To learn more about binomial coefficients, visit:
https://brainly.com/question/14216809
#SPJ1
What's the total charge for a meal that cost $47.5 if the tax it 8%?
Answers
To determine the total charge we first need to calculate how much we pay of taxes. This can be done by using a rule of three:
\(\begin{gathered} 47.5\rightarrow100 \\ x\rightarrow8 \end{gathered}\)Then we have:
\(x=\frac{8\cdot47.5}{100}=3.8\)Hence charge for the tax is $3.8.
Therefore the total charge of the meal is $51.3
Multiply.
(-0.3)(-0.85)
Answers
Answer:
O.225
Step-by-step explanation:
Two negatives = positive
Answer:
.255
Step-by-step explanation:
.255
in exercises 1-8 solve the inequality graph the solution
1. 6x < -30
Answers
Step-by-step explanation:
x<-5 is the answer
1.
6x=-30
2.
x=-5
3.
x<-5
Match the word to its description. The difference between the lowest and highest value 1. mode The middle value 2. mean 3. median The most frequent value PP 4. range The average
Answers
Answer:
range
Step-by-step explanation:
The difference between the lowest and the highest number is called the range. The middle value is the median (it's in the "mid"dle, median) The mean is the average. And the mode is the most frequent value.
Can someone help me with 11 and 12 plss

Answers
Answer:
Q11: x = 3
Q12: x = -4
Step-by-step explanation:
Q11
We have the equation as
\(\:64^{2x\:+\:4}=16^{5x}\)
Convert the bases in terms of the common base 4
64 = 16 x 4 = 4 x 4 x 4 = 4³
16 = 4 x 4 = 4²
Therefore the original equation becomes:
\(\mbox {\large 4^{3\left(2x+4\right)}=4^{2\cdot \:5x}}\)
Since the bases are the same in this equation, the exponents must also be the same
Equation the two exponents we get
3(2x + 4) = 2·5x
==> 6x + 12 = 10x
Move 12 to the right side
6x = -12 + 10x
Move 10x to the left:
6x - 10x = -12
-4x = -12
x = -12/-4 = 3
Answer to Q11
-------------------------------------------------------------
Q12
We can solve this in a similar manner to Q12
27 = 3 x 3 x = 3³
9 = 3 x 3 = 3²
Plugging these in we get
\(\mbox {\large 27^x = 3^{3(x)} = 3^{3x}}\)
\(\mbox {\large 9^ {(x - 2)} = 3^{2(x-2)} = 3^{2x -4} }\\\\\)
Thus we get
\(\mbox {\large 3^{3x} = 3^{2x -4} }\\\\\)
Equating the exponents we get
\(3x = 2x - 4\\\\3x - 2x = -4\\\\x = -4\)
ANSWER to 12
if you give me the correct answer then I will mark as brainliest

Answers
It's is b cause it is and yeah
Answer:
a
Step-by-step explanation:
List six consecutive numbers
Answers
Answer:
90, 91, 92, 93, 94, 95, 96
Determine the length

Answers
The length of KI is 10 cms.
What is Length?The skill of measuring the lengths of objects and understanding the units of length is very crucial as it helps us to interact with our environment in a more organized manner.
Here, ΔRJS ≈ Δ IJK
then JR / JI = SR/KI
1/2 =5 /ki
kI = 5 X 2
kI = 10 units.
Thus, The length of KI is 10 cms.
Learn more about Length from:
https://brainly.com/question/8552546
#SPJ1
B=cos-1(16) as a decimal
Answers
Hope it helps!
A bacterium was found to have a mass of
2
×
10
−
12
gram. After 30 hours, one bacterium was replaced by a population of 480,000,000 bacteria. What is the mass of the population of bacteria after 30 hours? Write your answer in scientific notation.
Answers
The mass of the population of bacteria after 30 hours is 9.6×10⁻⁴ grams in expressions.
What does a math expression mean?
Mathematical expressions consist of at least two numbers or variables, at least one arithmetic operation, and a statement. It's possible to multiply, divide, add, or subtract with this mathematical operation. An expression's structure is as follows: Expression: (Math Operator, Number/Variable, Math Operator)Mass of 1 bacterium =2×10-¹². Then the mass after 30 hours will be calculated as below:-
Mass of 480,000,000 bacteria = 2×10-¹²×480,000,000
Mass of 480,000,000 bacteria =0.00096
Mass of 480,000,000 bacteria =9.6×10⁻⁴
Therefore, the mass of the population of bacteria after 30 hours is 9.6×10⁻⁴ grams.
Learn more about expression
brainly.com/question/14083225
#SPJ9
Rhonda completed her homework in 1 1/2 hours jerry took 2 1/4 to do the same homework how much longer did jerry take than Rhonda did to complete the homework?
Answers
Using improper fractions we know that Jerry took 3/4 hours longer than Rhonda to complete the homework.
To find out how much longer Jerry took than Rhonda to complete the homework, we need to subtract Rhonda's time from Jerry's time. Here's a step-by-step explanation:
1. Convert the mixed numbers to improper fractions:
Rhonda: 1 1/2 = (1 * 2 + 1)/2 = 3/2
Jerry: 2 1/4 = (2 * 4 + 1)/4 = 9/4
2. Find a common denominator for both fractions, which in this case is 4.
3. Convert both fractions to have the common denominator:
Rhonda: 3/2 * 2/2 = 6/4
Jerry: 9/4 (already has the common denominator)
4. Subtract Rhonda's time from Jerry's time:
9/4 - 6/4 = 3/4
So, Jerry took 3/4 hours longer than Rhonda to complete the homework.
Know more about improper fractions here:
https://brainly.com/question/387381
#SPJ11
What is the relationship between

Answers
The relationship between ∠ a and ∠ b is that they are B. Complementary angles.
What are complementary angles ?Complementary angles have a sum that equates to 90 degrees, making them vital concepts in geometrical and trigonometrical problem-solving concerning right-angled triangles. Employed primarily in these two fields of study, their addition when combined engenders formation of a perfect right angle.
As we can see from the image, ∠ a and ∠ b would add up to 90 degrees because they are by a straight line.
Find out more on complementary angles at https://brainly.com/question/1358595
#SPJ1
the wronskian of the function e^x and e3x is?
Answers
The Wronskian of the functions e and e(3x) is 2e(4x).
The Wronskian of the functions e^x and e^(3x) is found by computing the determinant of the matrix formed by their derivatives. In this case, the Wronskian is:
W(e^x, e^(3x)) = |(d/dx)e^x (d/dx)e^(3x)|
|e^x 3e^(3x)|
Now, compute the determinant:
W(e^x, e^(3x)) = e^x(3e^(3x)) - e^(3x)e^x
W(e^x, e^(3x)) = 2e^(4x)
Know more about Wronskian of the functions here:
https://brainly.com/question/8472385
#SPJ11
until 2005 the entire postawko creek watershed in fremont county consisted of a national forest interspersed with hay meadows and houses on lots with a minimum size of five acres. in 2005 farmer cobb opened a dairy farm in the watershed, and the citizens of fremont county became concerned that the runoff from the dairy farm would negatively impact the water quality of the watershed. scientists employed by the county collected water quality data at five sites in the watershed; some of the data are compiled in the table above. based on these data, which site is most likely immediately downstream from the dairy farm?
Answers
As we can conclude that Site 5 is most likely immediately downstream from the dairy farm.
When we talk about the impact of the dairy farm's runoff on water quality, we must consider the concept of downstream. Downstream refers to the direction in which water flows. In a watershed, water flows from higher elevations to lower elevations. Thus, the site downstream of the dairy farm would be the one that receives water after it has passed through the farm.
Looking at the table provided, we can see that Site 5 has the highest values for nitrate, ammonia, and total phosphorus, which are all indicators of pollution. This indicates that Site 5 is likely to be immediately downstream of the dairy farm.
This conclusion is supported by the fact that Site 5 has the highest levels of pollutants, and it is the last site listed in the table. This means that the water flowing through Site 5 has already passed through the other four sites, including the dairy farm, before reaching Site 5.
To know more about downstream here
https://brainly.com/question/28904568
#SPJ4
Two functions, f and g are defined on R, the set of real numbers, by f(x)=x²-5 and g(x) = 3x - 1. Find f(g(x)).
Answers
Answer:
f(g(x)) = 9x² - 6x - 4
Step-by-step explanation:
f(g(x) )
= f(3x - 1)
= (3x - 1)² - 5 ← expand factor using FOIL
= 9x² - 6x + 1 - 5
= 9x² - 6x - 4
Please help me! I need an answer before 3:30!

Answers
Answer:
2
Step-by-step explanation:
Answer:
LCM: 1
Step-by-step explanation:
1/2 and 1/4
so we want to find a multiple.
double both we get:
1 and 1/2
but then what if we just double 1/2?
1 and 1 :OOOOO
LCM IS 1
but it's 6:18 P:M for me?
Tri-County G\$T selis 150,000 MWh per yeat of electrical power to Boulder at $80 per MWh, has fixed costs of $80.1 milion per yoar, and has varistele costs of $20 por MWh. If Tri-County has 1,000,000MW h of demand from its customers (other than Boulder), what will Tri-County have to charge to break even? Tri-County wit have to charge? to break oven. (Enter your response rounded to the nearest penny.)
Answers
Tri-County will have to charge approximately $83.1 per MWh to break even.
To calculate the break-even price that Tri-County will have to charge to cover its costs, we need to consider both the fixed costs and the variable costs. The fixed costs are given as $80.1 million per year.
The variable costs are calculated by multiplying the quantity of electrical power sold (150,000 MWh per year to Boulder) by the variable cost per MWh ($20). Therefore, the variable costs amount to 150,000 MWh/year * $20/MWh = $3 million per year.
To cover both fixed and variable costs, Tri-County needs to charge a total amount that equals the sum of these costs. The total cost is $80.1 million + $3 million = $83.1 million per year.
Now, let's calculate the break-even price per MWh. Since Tri-County has a demand of 1,000,000 MWh from its customers (other than Boulder), we can divide the total cost by this quantity to find the break-even price.
Break-even price = Total cost / Quantity of electrical power demanded
Break-even price = $83.1 million / 1,000,000 MWh = $83.1/MWh
Therefore, Tri-County will have to charge approximately $83.1 per MWh to break even.
Learn more about charge here:-
https://brainly.com/question/29017802
#SPJ11
The unit vectors on x, y and z axes of Cartesian coordinates are denoted i, j and k, respec- tively. Answer the following questions. (1) Let the scalar field = ez sin y + e* cos y and the vector field A = (2x - z)i - 2j+2k. Evaluate the component of the gradient of o in the direction of A at the point (1,0,1). (2) Evaluate the surface integral for the vector field A = zi-3j+ 4xyk, along the following surface S. S: 6x + 3y + z = 3 (x ≥ 0, y ≥ 0, z ≥ 0)
Answers
(1) The correct option is (A) Scalar field = ez sin y + e* cos y, and the vector field A = (2x - z)i - 2j+2k. We must find the component of the gradient of o in the direction of A at the point (1, 0, 1).The gradient of the scalar function φ (x, y, z) is defined as ∇φ = (∂φ / ∂x)i + (∂φ / ∂y)j + (∂φ / ∂z)k.
So, we have to find the gradient of the scalar field φ = ez sin y + e* cos y.∇φ = (∂φ / ∂x)i + (∂φ / ∂y)j + (∂φ / ∂z)k= 0i + ez cos y j + e* sin y kNow, at point (1, 0, 1), the gradient of the scalar field is given by,∇φ = 0i + e cos 0j + e sin 0k= e j + e* kAnd, at the point (1, 0, 1), the vector field A = (2x - z)i - 2j + 2k = 2i - 2j + 2kSo, we need to find the component of ∇φ along A, i.e.,∇φ . A / |A|∇φ . A = (e j + e* k) . (2i - 2j + 2k)= 0 + 0 + 4e* / 2= 2e*Hence, the required component is 2e*/√3. So, the correct option is (A).(2) We have to evaluate the surface integral for the vector field A = zi - 3j + 4xyk, along the following surface S, where S: 6x + 3y + z = 3 (x ≥ 0, y ≥ 0, z ≥ 0).
So, we need to find the unit normal vector of S at (x, y, z) and the limits of integration for x and y.The gradient of S is given by,∇S = 6i + 3j + kHence, the unit normal vector of S is given by,n = ∇S / |∇S|n = 6i + 3j + k / √46n = (2 / √46)i + (1 / √46)j + (1 / √46)k.We have to evaluate the surface integral for A along the surface S.S: 6x + 3y + z = 3 (x ≥ 0, y ≥ 0, z ≥ 0)The given surface is a plane that cuts through the positive x, y, and z axes. To perform the surface integral of A, we need to find a unit vector normal to the surface.6x + 3y + z = 3implies z = 3 - 6x - 3y.The normal vector is therefore N = (∂z/∂x)i + (∂z/∂y)j - k= -k.The surface integral of A is given by∬S A · dS where dS is an infinitesimal element of surface area.The surface S is a rectangle of sides 2 and 1. Therefore, its area is 2.The surface integral of A over S is∬S A · dS= ∬S (0)i - (0)j + (z)k · (-k) dS= -∬S (z) dS= -z(x, y) dxdy where z(x, y) = 3 - 6x - 3y. The limits of integration arex = 0 to x = 1- y = 0 to y = 1-xThe surface integral of A over S is therefore∬S A · dS= -∫[0,1]∫[0,1-x] (3 - 6x - 3y) dy dx= -[3x - 3x² - 3x(1 - x) + 3/2(1 - x)²]dx= -[3x - 9/2x² + 3/2x³ - 3/2x² + 3/2x³ - 1/2x⁴]dx= -[3/2x⁴ - 9x² + 6x]dx= -[3/10]Therefore, the surface integral of A over S is -3/10.Answer:1. The component of the gradient of ϕ in the direction of A at the point (1,0,1) is \($\frac{2e^{*}+2}{3\sqrt{3}}$\).2. The surface integral of A over S is -3/10.
To know more about scalar visit:
https://brainly.com/question/28518744
#SPJ11
a rectangular rug has an area of 160 square feet the lengut of the rug is 6 feet longer than the width
Answers
The width of the rug is 10 feet, and the length is 16 feet.
Let's assume the width of the rectangular rug is "x" feet.
According to the given information, the length of the rug is 6 feet longer than the width, so the length would be "x + 6" feet.
The formula for the area of a rectangle is length multiplied by width.
We can set up an equation using this formula to solve for the width:
Area = Length × Width
160 = (x + 6) × x
Now, let's simplify the equation:
\(160 = x^2 + 6x\)
Rearranging the equation:
\(x^2 + 6x - 160 = 0\)
Now we have a quadratic equation.
We can solve it by factoring, completing the square, or using the quadratic formula.
In this case, let's solve it by factoring:
(x + 16)(x - 10) = 0
Setting each factor to zero:
x + 16 = 0 or x - 10 = 0
Solving for x:
x = -16 or x = 10
Since we are dealing with measurements, we can disregard the negative value.
Therefore, the width of the rug is 10 feet.
Now, we can find the length by adding 6 to the width:
Length = x + 6 = 10 + 6 = 16 feet.
For similar question on width.
https://brainly.com/question/19634537
#SPJ8
what is 3856 times 4656 equal
Answers
(4656) (3856) = 17,953,536
What is the degree of the power function represented in the table

Answers
Answer:
2
Step-by-step explanation:
i took the quizz