Ms. Johnson had 3/4 of a tank of gas when she started a trip. When she arrived home, she had 1/8 of a tank left.What fraction of a tank of gas was used during the trip
Answers
Answer:
5/8
Step-by-step explanation:
3/4 = 6/8
6/8 - x = 1/8
x = used gas = 5/8
x is the fraction used
3/4 - 1/8 = x
(8)3/4 - 1/8(4) = x
24/32 - 4/32 = x
20/32 = x
Simplify
20/32 (/4) = 5/8
Hence she used 5/8 of the tank
To check the answer
3/4 - 5/8 = 1/8
ANSWER:
5/8
Related Questions
who is from india and speaks malyalam i do but i was born in america
Answers
Answer:
i don't sorry thanks for the points though
Step-by-step explanation:
Answer:
I do, not from India though.
Step-by-step explanation:
y = -x^4 + 25x³ - 150x²
graph
Answers
Answer:
Step-by-step explanation:
i thing its 125
Elizabeth is returning to the United states from Canada. She charges the remaining 300 canadian dollars she has to $250 US dollars. What was $1 US dollar worth in canadian dollars? I got 75000 what did I do wrong?
Answers
Answer:
1 us dollar would be worth $0.8333333 Canadian dollars
Step-by-step explanation:
i don't know what you did wrong but you would divide 250/300 to get the amount it's worth. I guess you tried to multiply it, don't do that.
FIRST TO ANSWER GETS BRAINLYEST WHATEVER ITS CALLED, PLEASE I NEED THIS DONE TODAY. JUST LOOK AT THE PICTURE

Answers
Answer:
27.9
Step-by-step explanation:
3*3.1 = height = 9.3
9.3 * 3
Answer the questions below to find the total surface area of the can.

Answers
Ab=3.14xRadious to the power of 2
then to the area of the rectange you do B x H = YOUR ANSWER
. Write the equation of a line with a slope of 4 passing through the point (3, 1). Write the
equation in slope-intercept form.
Answers
Answer:
y = 4x - 11
Step-by-step explanation:
The general equation of the slope-intercept form of a line is given by:
y = mx + b, where
(x, y) are any point on the line,m is the slope,and b is the y-intercept.We can find b, the y-intercept of the line, by plugging in 4 for m and (3, 1) for (x, y) in the slope-intercept form:
1 = 4(3) + b
1 = 12 + b
-11 = b
Thus, the y-intercept is -11.
Thus, the equation of the line with a slope of 4 passing through the point (3, 1) in slope-intercept form is y = 4x - 11
The answer is:
y = 4x - 11Work/explanation:
First, we will write the equation in point slope:
\(\sf{y-y_1=m(x-x_1)}\)
where m = slope;
(x₁,y₁) is a point on the line.
Plug in the data:
\(\sf{y-1=4(x-3)}\)
Simplify
\(\sf{y-1=4x-12}\)
Add 1 on each side
\(\sf{y=4x-12+1}\)
\(\sf{y=4x-11}\)
Hence, the equation is y = 4x - 11.A faucet leaks at a rate of 2 milliliters per second. What is the rate in liters per minute?
A
12 -
B
0.12 L/min
12LT
D
120 L/min
Answers
Answer: 0.12 per minute
Step-by-step explanation: I actually had to reread this one a couple times, as the setup is a bit confusing. Here's how I would solve it personally:
1. Since it's leaking 2ml per second, and there are 60 seconds in a minute, multiply 2ml times 60 seconds to get 120ml every minute.
2. 1ml equals 0.001 liters, so multiply 120ml by 0.001 to find out how many liters that is. 120(0.001)=0.12.
Hope this helps,
Lacia
what is 2043.666666 rounded to 2 decimal places
Answers
Answer:
\(2043.67\)
Step-by-step explanation:
Hundredths is at 2 decimal places.
The thousandths place is higher than 5, so add 1 to the hundredths place.
Answer:
2043.67
Step-by-step explanation:
If you’ve ever rounded a number, you would know that if it’s 5 or higher, round it up, and if it’s 4 or lower, round it down. In this case, the second decimal place reads ’6’ which is higher that 5, so we round up. The rest of the numbers stay the same
2043.67
calculate the mean deviation of the following numbers 8,5,7,10,3,4,31,12.
Answers
Answer:
5.75.
Step-by-step explanation:
The mean = (8+5+7+10+3+4+31+12)/ 8
= 80/8
= 10.
Now we calculate the difference of each value from the mean We take the absolute ( positive) differences
10 - 8 = 2
10 - 5 = 5
10 - 7 = 3
10 - 10 = 0
10-3 = 7
10 = 4 = 6
10 - 31 = 21
10 - 12 = 2
Sum of the differences = 46
and the mean deviation is 46/8 = 5.75.
Consider the equation that gives the volume of a square pyramid, where
• V represents the volume in cubic feet,
• b represents the length of each side of the pyramid's base in feet, and
• h represents the height of the pyramid in feet.
V= 1²
3
Enter an equation for which the solution is the height of a pyramid, in feet, when its volume is 48 cubic feet and the
length of each side of its base is 4 feet.
Answers
An equation for which the solution is the height of a pyramid, in feet, when its volume is 48 cubic feet and the length of each side of its base is 4 feet is h = 3(48)/4².
How to calculate the volume of a square pyramid?In Mathematics and Geometry, the volume of a square pyramid can be calculated by using the following formula:
Volume of a square pyramid, V = 1/3 × a² × h
Where:
h represent the height of a square pyramid.a represent the side length of the base of a square pyramid.By making "h" the subject of formula, the side length of the base of a square pyramid can be determined as follows;
V = 1/3 × a² × h
3V = a²h
Height, h = 3V/a²
Height, h = 3(48)/4²
Read more on pyramid here: brainly.com/question/16315790
#SPJ1
4. Emily, Ashley and Peter can clean a warehouse in 2 hours. If Emily does the job alone, she can finish it in 6 hours. If Ashley does the job alone she can finish it in 8 hours.
How long will it take for Peter to finish the job alone?
How long will it take for Emily and Peter to finish the job together.
Answers
According to the unitary method,
A) The time taken for Peter to finish the job alone is 4 hours, 48 minutes.
B) The time taken for Emily and Peter to finish the job together is 2 hours, 36 minutes.
Unitary method:
In math, unitary method refers the process of finding the value of a single unit, and based on this value.
Given,
Emily, Ashley and Peter can clean a warehouse in 2 hours. If Emily does the job alone, she can finish it in 6 hours. If Ashley does the job alone she can finish it in 8 hours.
Here we need to find the following:
A) The time take for Peter to finish the job alone
B) The time taken for Emily and Peter to finish the job together
Let us consider x be the time taken for Peter to finish the job alone.
So, based on the given question we can write it as,
=> 1/6 + 1/8 + 1/x = 1/2
=> (8 + 6)/48 + 1/x = 1/2
=> 14/48 + 1/x = 1/2
=> 7/24 + 1/x = 1/2
=> 1/x = 1/2 - 7/24
=> 1/x = 12 -7/24
=> 1/x = 5/24
=> x = 24/5
=> x = 4.8
So, the time taken for Peter to finish the job alone is 4 hours, 48 minutes.
Then the time taken for Emily and Peter to finish the job together is calculated as,
=> 5/24 + 1/6
=> 5 + 4/ 24
=> 9/24
=> 3/8
Therefore, the time taken for Emily and Peter to finish the job together 2 hours, 36 minutes.
To know more about Unitary method here.
https://brainly.com/question/28276953
#SPJ1
Each airline passenger and his or her luggage must be checked to determine whether he or she is carrying weapons on to the airplane. Suppose that at O’hare International Airport, an average of 10 passengers per minute arrive (interarrival times are exponential). To check passengers for weapons, the airport must have a checkpoint consisting of a metal detector and baggage X-ray machine. Whenever a checkpoint is in operation, two employees are required. A checkpoint can check an average of 12 passengers per minute (the time to check a passenger is exponential). Under the assumption that the airport has only one checkpoint, answer the following questions:
a. What is the probability that a passenger will have to wait before being checked for weapons?
b. On the average, how many passengers are waiting in line to enter the checkpoint?
c. On the average, how long will a passenger spend at the checkpoint?
Answers
Answer:
a
\(P_k = 0.83\)
b
\(N_{\mu} \approx 4 \ passengers\)
c
\(T_{\lambda} = 0.5 \ minutes\)
Step-by-step explanation:
From the question we are told that
The average number of passengers that arrive per minute is \(\lambda = 10\)
The average number of check that can be carried out in one minute is \(\mu= 12\)
Generally the probability that a passenger will have to wait before being checked for weapons is mathematically represented as
\(P_k = \frac{\lambda }{ \mu }\)
=> \(P_k = \frac{10 }{ 12}\)
=> \(P_k = 0.83\)
Generally the number of passengers are waiting in line to enter the checkpoint is mathematically represented as
\(N_{\mu} = \frac{\lambda^2}{\mu (\mu -\lambda) }\)
=> \(N_{\mu} = \frac{10^2}{12 (12 -10) }\)
=> \(N_{\mu} \approx 4 \ passengers\)
Generally the average time a passenger spend at the checkpoint is mathematically represented as
\(T_{\lambda} = \frac{ \frac{\lambda}{(\mu - \lambda)} }{ \lambda}\)
=> \(T_{\lambda} = \frac{ \frac{ 10}{(12 - 10)} }{10}\)
=> \(T_{\lambda} = 0.5 \ minutes\)
Find the mass of lamina bounded by circles x2 + y2 = 1 and x2 + y2 = 4in the first quadrant if the density is (x2 + y2). Could please anyone solve this...
Answers
Since density is equal to mass per unit volume, mass is equal to density times volume. So we split up the lamina into tiny regions with "volume" (area) equal to dA, multiplied by the density, and integrated over the entirety of the lamina.
This is best done in polar coordinates:
\(\begin{cases}x=u\cos v\\y=u\sin v\end{cases}\implies\mathrm dA=\mathrm dx\,\mathrm dy=u\,\mathrm du\,\mathrm dv\)
so that \(x^2+y^2=u^2\cos^2v+u^2\sin^2v=u^2\).
The lamina is then the set of points
\(L=\left\{(u,v)\mid1\le u\le2\land0\le v\le\dfrac\pi2\right\}\)
Now compute the integral: the mass of the lamina is
\(\displaystyle\iint_L(x^2+y^2)\,\mathrm dA=\int_0^{\pi/2}\int_1^2u^3\,\mathrm du\,\mathrm dv=\frac\pi2\int_1^2u^3\,\mathrm du=\frac{15\pi}8\)
Convert as indicated. When necessary round to the nearest tenth of a degree At company headquarters the room temperature is to be set at 70F but the thermostat is calibrated Celsius find the temperature to be set

Answers
Given:
Temperature value =70 f
Required:
To convert this value in celsius f
Explanation:
We have to covert f into celsius.
\((70\mathring{\text{ }}F-32)\times\frac{5}{9}\mathring{\text{ = 21.111}}\)we just simply place given value in equation,
Required answer:
21.11 celsius
If a driver uses of a tank of gas every day, what fraction of a tank will he use in a) 3 days? b) 1 week?
Answers
Answer:
Step-by-step explanation:
a) If a driver uses a full tank of gas every day, the fraction of a tank he will use in 3 days is 3/1, or 3/1 of a tank.
b) If a driver uses a full tank of gas every day, the fraction of a tank he will use in 1 week is 7/1, or 7/1 of a tank.
It's worth noting that tanks of gas come in different sizes, so the amount of gas consumed in a day, week, or any other period of time will depend on the size of the tank, and the consumption of the car, so this answer is based on the assumption that a full tank is used every day.
PLEASE HELP ME!! ILL GIVE BRAINLIEST!!
Kate is making hamburgers for her friends and has 7 1/2. pounds of hamburger. How many 1/4 pound of hamburgers can she make?
A. 15
B. 4
C. 30
D. 120
Answers
Answer:
C
Step-by-step explanation:
1/4 is .25
4 fourths is 1. 4 times 7 equals 28 hamburgers. one half is two fourths. 28+2=30 hamburgers.
No of hamburgers she can make
\(\\ \sf\longmapsto 7\dfrac{1}{2}\div \dfrac{1}{4}\)
\(\\ \sf\longmapsto \dfrac{15}{2}\times \dfrac{4}{1}\)
\(\\ \sf\longmapsto \dfrac{60}{2}\)
\(\\ \sf\longmapsto 30\)
The table compares the average daily temperature and ice cream sales each day.
Temperature (°F) Ice Cream Sales
58.2 $112
64.2 $135
64.3 $138
66.8 $146
68.4 $166
71.6 $180
72.7 $188
76.2 $199
77.8 $220
82.8 $280
Using technology, determine the line of fit, where x represents the average daily temperature and y represents the total ice cream sales. Round values to the nearest tenth.
A) ŷ = 3.8x − 109.2
B) ŷ = −3.8xx − 109.2
C) ŷ = 6.5x − 279.1
D) ŷ = −6.5x − 279.1
Answers
The linear regression equation is defined as follows:
C. ŷ = 6.5x − 279.1
How to find the equation of linear regression?To find the regression equation, which is also called called line of best fit or least squares regression equation, we need to insert the points (x,y) in the calculator.
The points for this problem are given on the table as follows:
(58.2, 112), (64.2, 135), (64.3, 138), (66.8, 146), (68.4, 166), (71.6, 180), (72.7, 188), (76.2, 199), (77.8, 220), (82.8, 280).
Inserting these points into a calculator, the line of best fit is given as follows:
ŷ = 6.5x − 279.1
Meaning that the correct statement is given by option C.
More can be learned about linear regression at https://brainly.com/question/29613968
#SPJ1
There are 8 ounces in a cup, 2 cups in a pint, 2 pints in a quart, and 4 quarts in a gallon.
1 L≈ 0.26 gallons
7 KL= cups
Answers
Since there are 1000 L in a kL, we have to multiply 0.26 gallons by 1000 to get gallons in a kL. 0.26 x 1000 = 260
Now we have to change gallons to cups. Since there are 16 cups in a gallon (2 x 2 x 4), you have to multiply 260 by 16 to get cups in a gallon. 260 x 16 = 4160.
That means there are 4160 cups in a kL, using those conversion factors.
Pls hurry I’m being timed

Answers
Answer:
C
Step-by-step explanation:
C explains what is currently happening.
Answer:
its b
Step-by-step explanation:
A sales person starts working 40 hours per week at a job with 2 options for being paid . Option A is an hourly wage of $19. Option B is a commission rate of 8% on weekly sales.
How much does the sales person need to sell in a given week to earn the same amount with each option?
A. $9,500
B. $4,750
C. $760
D. $320
Answers
Given, Option A: Hourly wage is $19 and the salesperson works 40 hours per week. So, he will earn in a week \(\sf = 19 \times 40 = \$760\)
Now, according to option b, he will get 8% commission on weekly sales.
Let. x = the amount of weekly sales.
To earn the same amount of option A, he will have to equal the 8% of x to $760
So, \(\sf \dfrac{8x}{100}=760\)
Or, \(\sf 8x= 76000\)
Or, \(\sf x= \dfrac{76000}{8}=9500\)
the salesman needs to make a weekly sales of $9,500 to earn the same amount with two options.
It takes Miranda 30 minutes to paint 6 chairs. If Miranda paints at a constant rate, what is the constant of proportionality that relates y, the number of chairs painted in x minutes?
Answers
Answer:
5
Step-by-step explanation:
Divide 30 by 6, since this will find the number of chairs she can paint in 1 minute:
30/6
= 5
So, the constant of proportionality is 5, since she can paint 5 chairs in a minute.
Answer:
1/5
Step-by-step explanation:
You want k so that ...
y = kx
You are told that y=6 when x=30, so ...
6 = k·30
6/30 = k = 1/5
The constant of proportionality is 1/5.
y = (1/5)x
there is a graph with 36 possible outcomes. What is the probability of rolling a sum of 10 when rolling 2 number cubes.
A. 1/12
B. 1/9
C. 1/3
D. 5/12
Answers
Answer: A
Step-by-step explanation:
Answer:
A. 1/12
Step-by-step explanation:
please help me answer this question thank you

Answers
Answer:
A
Step-by-step explanation:
Match the expression on the left with its simplified form on the right. Answer options on the right may be used more than once.

Answers
Answer:
-/3/=-3
/-3/=3
Step-by-step explanation:
qwarsrdzxcftgvyhubjnjbjonkklpnvgutcuhsdbdjdjsnd
Evaluate-5X 6 =
(Need help)
Answers
Answer:
x=7
Step-by-step explanation:
i think
The sum of an A.P. is 340, the first term is 7 and the common difference is 6.
Calculate the number of terms in the sequence
Answers
The number of terms (n) in the sequence = 10.
We know that,
S = \(\frac{n}{2\\}\) {2a + (n-1)d}
Where,
S = Sum of A. P. series
n = number of terms in A. P. series
a = first term of the series
d = common difference
According to the question,
Sum of the series, S = 340
First term, a = 7
Common difference, d = 6
S = \(\frac{n}{2\\}\) {2a + (n-1)d}
340 = \(\frac{n}{2}\) {2*7 + (n-1)6}
340 = \(\frac{n}{2}\) {14 + 6n - 6}
340*2 = n( 6n + 8)
680 = 6n^2 + 8n
6n^2 + 8n - 680 = 0
2 (3n^2 +4n - 340) = 0
3n^2 +4n - 340 = 0
3n^2 + 34n - 30n - 340 = 0
n (3n+ 34) - 10 (3n + 34) = 0
(3n + 34) (n - 10) = 0
if we take, 3n + 34 = 0
3n = - 34
n = -34/3
which is not possible, as the number of terms can not be negative
if we take, n- 10 = 0
n = 10
Hence, the number of terms in the given A. P. series is 10.
Learn more about Arithmetic Progression (A. P.) Series:
https://brainly.com/question/20375979
I need help figuring out any details that are in this graph and what you can make of it.

Answers
The graph shows the comparison of the human welfare and ecological footprints.
What is a graph?A graph simply means a diagram that shows the relationship between variables.
From the graph, it can be seen that countries such as Norway, Canada, USA, and Australia meet the minimum criteria for sustainability.
The graph also shows that Sierra Leone is the least developed country among the countries compared as it has the lowest human development index.
The threshold for high human development as given as 0.8. Most African countries had a human development index of 0.5 and below.
Learn more about graphs on:
brainly.com/question/19040584
#SPJ1
Question 3 of 10How many solutions does the nonlinear system of equations graphed belowhave?10X10
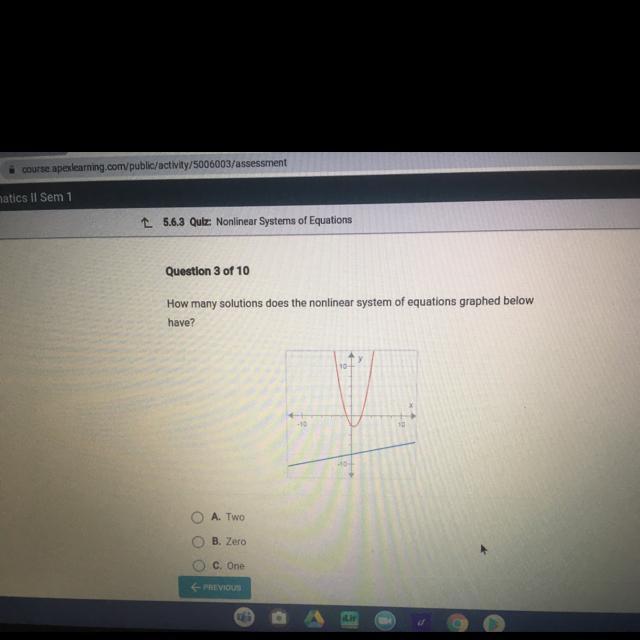
Answers
Answer:
B. Zero
Explanation:
The solutions of the system of equation are the points where the graph intersect. In this case, the red function and the blue function doesn't cross each other. So, the system formed by these non linear equations has no solutions.
Therefore, the answer is:
B. Zero
Answer:two
Step-by-step explanation:
Find the volume of the cubes or rectangular prisms in cubic centimeters.
SS
8
125 cm
126cm
ਹੈ।
135cm
rectangular prism with a length,
width and height of 5 cm. 5 cm.
and 5 cm, respectively
cube with a side length of 5 cm
of a
cube with a side length of 5 cm
rectangular prism with a length,
width and height of 5 cm 5 cm.
and 5 cm, respectively

Answers
Answer:
ans : it is order wise
a) 6/125 cm³
b) 8/125 cm³
c) 1/125 cm³
d) 36/125 cm³
Step-by-step explanation:
a)
for rectangular prism = length × width × height
= (1/5) × (2/5) × (3/5)
= 6/125 cm³
b)
for cube = length³
= (2/5)³
= 8/125 cm³
c)
for cube = (1/5)³
= 1/125 cm³
d)
for rectangular prism = (3/5) × (4/5) × (3/5)
= 36/125 cm³
The percent defective for parts produced by a manufacturing process is targeted at 4%. The process is monitored daily by taking samples of sizes n = 160 units. Suppose that today’s sample contains 14 defectives. Determine a 88% confidence interval for the proportion defective for the process today. Place your LOWER limit, rounded to 3 decimal places, in the first blank. For example, 0.123 would be a legitimate answer. Place your UPPER limit, rounded to 3 decimal places, in the second blank. For example, 0.345 would be a legitimate entry.
Answers
Answer:
The 88% confidence interval for the proportion of defectives today is (0.053, 0.123)
Step-by-step explanation:
In a sample with a number n of people surveyed with a probability of a success of \(\pi\), and a confidence level of \(1-\alpha\), we have the following confidence interval of proportions.
\(\pi \pm z\sqrt{\frac{\pi(1-\pi)}{n}}\)
In which
z is the zscore that has a pvalue of \(1 - \frac{\alpha}{2}\).
For this problem, we have that:
\(n = 160, \pi = \frac{14}{160} = 0.088\)
88% confidence level
So \(\alpha = 0.12\), z is the value of Z that has a pvalue of \(1 - \frac{0.12}{2} = 0.94\), so \(Z = 1.555\).
The lower limit of this interval is:
\(\pi - z\sqrt{\frac{\pi(1-\pi)}{n}} = 0.088 - 1.555\sqrt{\frac{0.088*0.912}{160}} = 0.053\)
The upper limit of this interval is:
\(\pi + z\sqrt{\frac{\pi(1-\pi)}{n}} = 0.088 + 1.555\sqrt{\frac{0.088*0.912}{160}} = 0.123\)
The 88% confidence interval for the proportion of defectives today is (0.053, 0.123)