PLEASE HELP!! I WILL MARK THE FIRST CORRECT ANSWER BRAINLIEST!!!!)) A cylinder has a radius of 8 centimeters. Its volume is 3,818.24 cubic centimeters. What
is the height of the cylinder?
Use na 3.14 and round your answer to the nearest hundredth.
(1) ha
centimeters

Answers
Answer:
h≈18.99 cm
Step-by-step explanation:
Related Questions
8cm
20cm
What is the volume of this cylinder?

Answers
Answer:
4021.2386 cm ^3
Step-by-step explanation:
V = πr2h = π·82·20 = 4021.2386 cm ^3
Someone help me with this

Answers
Answer:
81
Step-by-step explanation:
Any number, wether it is negative or postive, will always be postive when there is a even exponent.
In this case, we have a -9, and a exponent thats 2.
Since 2 is a even number, -9^2 will be a postive value.
Now, what is the actual value of this?
Well, a exponent of 2 basiclly means 2 numbers being multiplied together.
Or in this case, 2 negative 9s being multiplied together.
THis will be:
-9*-9
=
As I said above, a even exponent makes a negative number postive.
You see here that a negative 9 is being multiplied by -9. When two negative numbers multiply together, they make a postive.
THis is why a even exponent makes a postive.
Hope this helps!
Which is the equation of the line shown in the graph?
y
(-3, 4)
2
o
2|(50)
07-2
5
- 2x +
Oy-x+5
Oy

Answers
I think the right answer is A
Answer:
Option 3 is the correct answer.
Plssss help me plssss

Answers
Answer:
5 courage 1 classic
Step-by-step explanation:
So you already have 5 of the courage’s. Then, one fifth of them is 5/5=1. So 1 classic
set-1 consists of 10 elements. what is the total number of ways in which elements from s1 can be arranged in a line?
Answers
The total number of ways in which elements from set-1 can be arranged in a line is given by the permutation of 10 elements, denoted by P(10), which is 10!. So, the total number of ways to arrange 10 elements in a line is 10! = 3,628,800.
If set-1 consists of 10 elements, the total number of ways in which elements from s1 can be arranged in a line is given by the permutation of 10 elements, denoted by P(10), which is:
P(10) = 10!
This means that there are 10 ways to choose the first element, 9 ways to choose the second element (since one element has already been chosen), 8 ways to choose the third element (since two elements have already been chosen), and so on, until there is only 1 way to choose the last element.
Therefore, the total number of ways to arrange 10 elements in a line is 10! = 3,628,800.
Learn more about permutations here: brainly.com/question/30649574
#SPJ4
Find the exact location of all the relative and absolute extrema of the function. (Order your answers from smallest to largest x.) f(x) = 2x2 – 3x + 2 with domain [0, 3] f has --Select-- at (x, y) =
Answers
The function \(f(x) = 2x^2 - 3x + 2\) has a relative minimum at (3/4, 5/8) and an absolute minimum at (3/4, 5/8), and an absolute maximum at (3, 11).
To find the relative and absolute extrema of the function \(f(x) = 2x^2 - 3x + 2\) with domain [0, 3], we need to follow these steps:
Find the critical points of f(x) by solving f'(x) = 0.
Test the critical points and the endpoints of the domain to determine the relative and absolute extrema.
Step 1: Find the critical points of f(x) by solving f'(x) = 0.
\(f(x) = 2x^2 - 3x + 2\)
f'(x) = 4x - 3
Setting f'(x) = 0, we get:
4x - 3 = 0
4x = 3
x = 3/4
So, the critical point of f(x) is x = 3/4.
Step 2: Test the critical points and the endpoints of the domain to determine the relative and absolute extrema.
\(f(0) = 2(0)^2 - 3(0) + 2 = 2\)
\(f(3) = 2(3)^2 - 3(3) + 2 = 11\)
\(f(3/4) = 2(3/4)^2 - 3(3/4) + 2 = 5/8\)
To determine the relative and absolute extrema, we need to examine the sign of f'(x) around each critical point and at the endpoints of the domain.
When x < 3/4, f'(x) < 0, which means that f(x) is decreasing. When x > 3/4, f'(x) > 0, which means that f(x) is increasing. Therefore, the function has a relative minimum at x = 3/4.
Since f(3/4) is the lowest point on the function, it is also an absolute minimum.
The function has an absolute maximum at x = 3, and no other relative extrema.
Therefore, the function \(f(x) = 2x^2 - 3x + 2\) has a relative minimum at (3/4, 5/8) and an absolute minimum at (3/4, 5/8), and an absolute maximum at (3, 11).
To learn more about relative minimum visit: https://brainly.com/question/29088643
#SPJ11
Ray has tea. He has 2.671 liters of tea he pours 0.47 liters in a glass. how many liters did he pour? Solve.
Answers
On solving the given problem by help of mathematical operations, we got - After Ray poured 0.47L, he was left with 2.201L.
What does the term "mathematical operations" mean?The term "operation" in mathematics refers to the process of calculating a value using operands and a math operator. For the given operands or numbers, the math operator's symbol has predetermined rules that must be followed.
What are the five operations in mathematics?In mathematics, there are five basic operations: addition, subtraction, multiplication, division, and modular forms.
Total amount of tea Ray has - 2.671L
Amount of tea Ray poured - 0.47
Therefore,
amount he was left with was = 2.671- 0.47 = 2.201L
To know more about mathematical operations visit:
https://brainly.com/question/8959976
#SPJ9
Simplified fraction 8 (5/6) =
Answers
hi
technique is to decompose both part of the fraction ( in prime numbers if possible )
8 * 5/6 = 2*4*5 / 3*2 = 20/3
STEP BY STEP EXPLANATION
can someone justify my answer for me -

Answers
Answer:
Step-by-step explanation:
You are correct in what you are doing. All you need do is show that what you got can be expressed algebraically.
Let x be the time in hours.
Let y be the snowfall in inches.
The first one does not work.
y = k * x
12 = k * 6 Divide both sides by 6
12/6 = k/6
k = 2
All the rest will fit into this equation.
try x = 2.5
y = k * x
y = 2 * 2.5
y = 5 which is just the amount of snowfall you should get.
Evaluate the expression for f=-10 9f+39
Answers
Answer:
The answer is 129.
Step-by-step explanation:
If f = 10, we would have to multiply 9 x 10, which is 90.
Add that to 39, and you will get 129.
Hope you can understand this
Answer:
-51
Step-by-step explanation:

Write down the two inequalities that define the shaded region in the diagram

Answers
The two inequalities that define the shaded region in the diagram are:
y ≥ 4 and y < x
How to Write Inequalities that define the Shaded Region?For the solid vertical line, the slope (m) is 0. The inequality sign we would use would be "≥" because the shaded region is to the left and the boundary line is solid.
The y-intercept is at 4, therefore, substitute m = 0 and b = 4 into y ≥ mx + b:
y ≥ 0(x) + 4
y ≥ 4
For the dashed line:
m = change in y / change in x = 1/1 = 1
b = 0
the inequality sign to use is: "<"
Substitute m = 1 and b = 0 into y < mx + b:
y < 1(x) + 0
y < x
Thus, the two inequalities are:
y ≥ 4 and y < x
Learn more about Inequalities on:
https://brainly.com/question/24372553
#SPJ1
It costs $2.80 to make a sandwich at the local deli shop. To make a profit, the deli sells it at a price that is 170% of the cost. The sandwich sells for $___. (Make sure to enter the answer as a decimal number only. Do not enter special characters such as the dollar symbol.)
Answers
Answer:
$4.76
Step-by-step explanation:
It costs $2.80 to make a sandwich at the local deli shop and the deli sells it at a price that is 170% of the cost.
We have to find 170% of the cost of making each sandwich ($2.80):
170/100 * 2.80 = $4.76
The sandwich sells for $4.76
Answer:
ben
Step-by-step explanation:
a hat company charges a design fee plus $4 per hat. the total cost of 5 hats is $30. how much will it cost for 3 hats?
Answers
Using Algebraic expression solution ,
The cost of three hats is $18 .
We have given that,
A hat company charge for design fee $4 per hat .
i.e design fee of one hat = $4
total cost of 5 hats = $30
let the cost of one hat without design fee be $x and total cost of one hat is $(x+4) .
using the above statement, we get an algebraic expression,
5( x+ 4)= 30
we solve the above algebra expression,
=> 5x + 20 = 30
=> 5x = 10
=> x = 2
so, cost of a hat without design fee is $2
and total cost of one hat is $6.
we have to calculate cost of 3 hats .
cost of one hat in hat company= $ 6
cost of three hats in hat company= $(6×3)
= $18
Hence, the total cost of 3 hats is $18.
To learn more about Algebraic expression , refer:
https://brainly.com/question/22763064
#SPJ4
Write a fraction and a percent to represent the shaded portion of the model
Help, NOW PLSSS ITS DUE TOMORROW SNNSDJJDJSJKSMSMSKDKDKKDKSK

Answers
Answer:
1. 1/4 - 25%
2. 3/6 - 50%
3. 2/10 - 20%
4. 1/4 - 25%
5. 3/5 - 60%
6. 2/3 - 66.66%
7. 2/4 - 50%
8. 2/5 - 40%
Step-by-step explanation:
Evaluate the function when x=3, x=0, x=-2
f(x)=x
Answers
The value of the function at, x = 3, x = 0 and x = - 2 are 3, 0, and - 2.
What is a function?A function can be defined as the outputs for a given set of inputs.
The inputs of a function are known as the independent variable and the outputs of a function are known as the dependent variable.
Given, A function f(x) = x, Now this is an identity function as y = x.
Now, At x = 3,
f(3) = 3.
At x = 0,
f(0) = 0.
At, x = - 2,
f(-2) = - 2.
learn more about functions here :
https://brainly.com/question/12431044
#SPJ1
The price of products may increase due to inflation and decrease due to depreciation. Marco is studying the change in the price of two products, A and B, over time.The price f(x), in dollars, of product A after x years is represented by the function below:f(x) = 72(1.25)xPart A: Is the price of product A increasing or decreasing and by what percentage per year? Justify your answer. (5 points)Part B: The table below shows the price f(t), in dollars, of product B after t years:t (number of years)1234f(t) (price in dollars)6584.5109.85142.81Which product recorded a greater percentage change in price over the previous year? Justify your answer. (5 points)
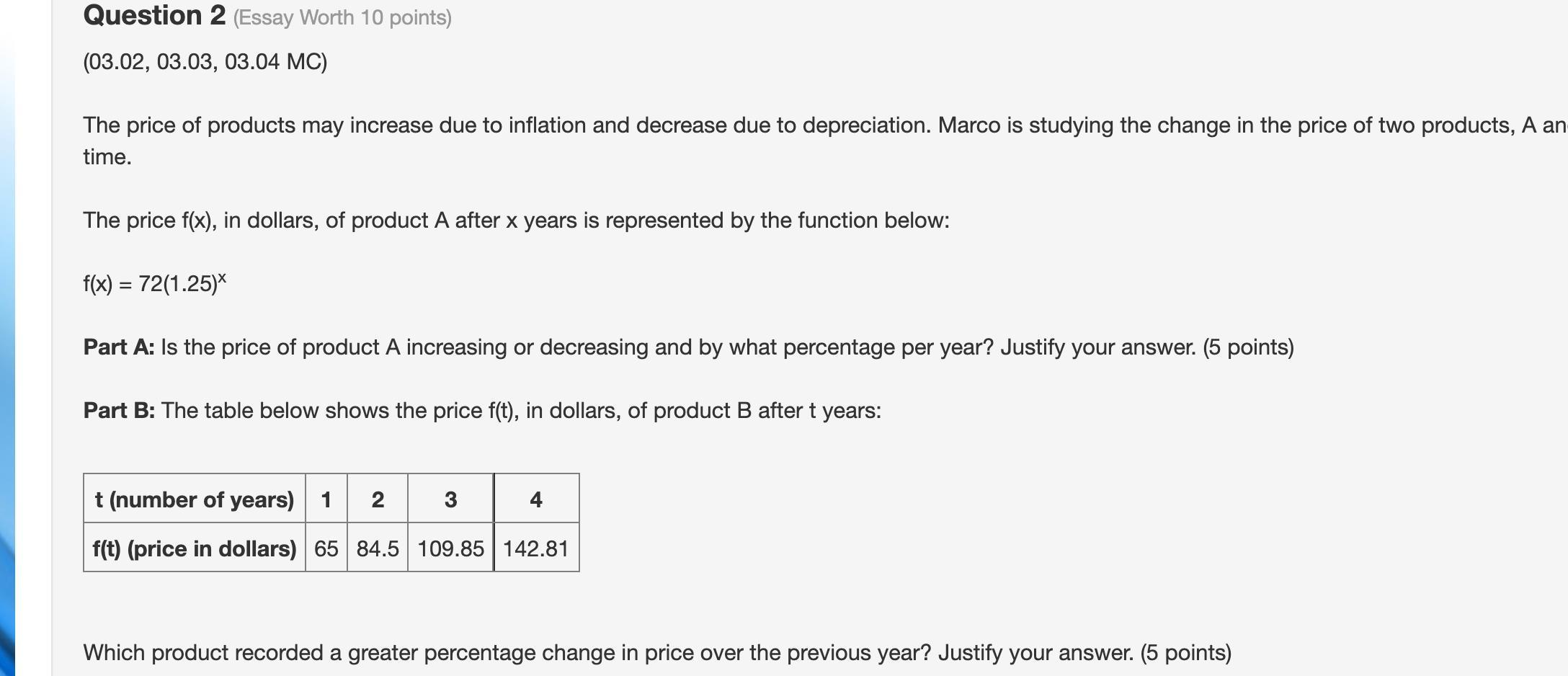
Answers
Part A.
According to the given equation, the price of product A is increasing, this is because the number that is being raised to x is greather than 1, which means that each year the price will be greater.
It is increasing by a 25% per year (we know this because the number is 1.25, which means that each year it increases 0.25 of its value).
Part B.
We can use the given values to find the value of the percentage change in price of product B:
\(\begin{gathered} 84.5=65(x) \\ \frac{84.5}{65}=x \\ 1.3=x \\ \end{gathered}\)It means that the percentage change in price of product B is 30%. In conclusion, product B has a greater percentage change in its price.
Which number produces a rational number when added to o ? Α. π= pie
B. square root 12
C. 0.314
D. 4.35889894...
Answers
Explain why two variables must both be quantitative in order to find the correlation between them
Answers
If the variables are not quantitative we cannot do the arithmetic required in the formulas for r.
What is a variable?A variable in mathematics is a symbol and placeholder for a changing quantity or any mathematical object.A variable can specifically represent a number, a vector, a matrix, a function, a function's argument, a set, or an element of a set.Quantitative order:
Quantitative methods emphasize objective measurements and statistical, mathematical, or numerical analysis of data gathered through polls, questionnaires, and surveys, as well as by manipulating pre-existing statistical data using computational techniques. Ordinal-level measurement data can be quantitative or qualitative. They can be arranged in ranked order, but differences between entries are meaningless. Measurement data at the interval level are quantitative. They can be arranged in any order, and meaningful differences between data entries can be calculated. We can't do the arithmetic required in the r formulas if the variables aren't quantitative.Therefore, if the variables are not quantitative we cannot do the arithmetic required in the formulas for r.
Know more about quantitative data here:
https://brainly.com/question/24492737
#SPJ4
probality of the impossible things in always ______
Answers
The probability of an impossible event is 0. Because it cannot occur in any situation.
What is the slope of the line that passes through the points (5,11) and (14,-10)
Answers
Answer:
the picture has the answer on it
Step-by-step explanation:


The slope of the straight line that passes through the points (5,11) and (14,-10) is \(\frac{-7}{3}\).
What is a straight line?''A straight line is an endless one-dimensional figure that has no width. A straight line is a combination of endless points joined on both sides of a point.''
Given, two points are (5, 11) and (14, -10).
Let, the line that passes through both these points has a slope = m.
Where, m = \(\frac{y_2 - y_{1} }{x_2- x_{1} }\).
Here, (y₂ - y₁) = Change in the value of y.
(x2 - x₁) = Change in the value of y.
Therefore, \(\frac{y_2 - y_{1} }{x_2- x_{1} }\) = \(\frac{-10 - 11}{14 - 5}\) = \(\frac{-21}{9}\) = \(\frac{-7}{3}\)
Hence, slope of the straight line is \(\frac{-7}{3}\).
Learn more about straight line here: https://brainly.com/question/11132221
#SPJ2
manova tests whether mean differences among groups on a combination of dvs is ... if there is at least 20 cases in the smallest cell the test is robust to ...
Answers
Manova (Multivariate Analysis of Variance) tests whether mean differences among groups on a combination of dependent variables (DVs) are statistically significant. If there is at least 20 cases in the smallest cell, the test is robust to violations of the assumption of multivariate normality.
In Manova, multiple dependent variables are analyzed simultaneously to determine if there are significant differences among groups. The test examines whether the mean vectors of the groups are equal across the DVs. The assumption of multivariate normality implies that the data should follow a multivariate normal distribution.
Know more about Multivariate Analysis of Variance here:
https://brainly.com/question/30847840
#SPJ11
50-(7•8)+9 please help! I think it is 3 but I am not positive
Answers
Find the midpoint of the line segment with endpoints (-3, 2) and (1.-2)
A)
(-1,0)
B)
(0, -1)
(-2,0)
D)
(0, -2)
Answers
Answer: They answer is A (-1,0)
Step-by-step explanation:
I plotted (-3,2) and (1,-2) and found they midpoint of it.
Can someone pls help me on this. I'll give BRAINLIEST

Answers
Answer:
i think the answer is 9
Step-by-step explanation:
(2x-1)=17
2x=17+1
x=18/2
x=9
consider the first order separable equation y′=(1−y)54 an implicit general solution can be written as x =c find an explicit solution of the initial value problem y(0)=0 y=
Answers
The explicit solution to the given initial value problem
y′=(1−y)5/4 with y(0)=0 is
y(x) = \(1 - (1 - e^x)^4/5\)
What is the explicit solution to the initial value problem y′=(1−y)5/4 with y(0)=0?The given first-order differential equation is separable, which means that we can separate the variables and write the equation in the form
\(dy/(1-y)^(5/4) = dx.\)
Integrating both sides, we get \((1-y)^(-1/4)\) = 5/4 * x + C, where C is the constant of integration. Solving for y, we get y(x) = 1 -\((1 - e^x)^4/5\).
Using the initial condition y(0) = 0, we can solve for C and get C = 1. Therefore, the explicit solution to the initial value problem is
\(y(x) = 1 - (1 - e^x)^4/5.\)
Learn more about differential equation
brainly.com/question/31583235
#SPJ11
help meeeeeeeeeeeeeeeeeeeeee

Answers
Answer:
To the nearest tenth, the length is 6.4 meters, and the width is 3.4 meters.
Step-by-step explanation:
Let w be the width of the rectangle. Then l = w + 3, and we have:
\( w(w + 3) = 22\)
\( {w}^{2} + 3w = 22\)
\( {w}^{2} + 3w - 22 = 0\)
Calculating the discriminant:
\( \sqrt{ {3}^{2} - 4(1)( - 22)} = \sqrt{9 + 88} = \sqrt{97} \)
So by the quadratic formula, and discarding the negative solution, we then obtain:
\(w = \frac{ - 3 + \sqrt{97} }{2} = 3.4\)
\(l = w + 3 = 3.4 + 3 = 6.4\)
Simplify the expression:
-(5r^3)^2
Answers
Testing more properties of the Cobb-Douglas utility function Check if the Cobb-Douglas utility function u(x
1
,x
2
)=x
i
α
x
2
β
, where α,β>0, satisfies the following properties: (a) local nonsatiation, (b) decreasing marginal utility for both goods 1 and 2, (c) quasi-concavity, and (d) homotheticity.
Answers
The Cobb-Douglas utility function satisfies the properties of local non-satiation, decreasing marginal utility for both goods, quasi-concavity, and homotheticity.
The Cobb-Douglas utility function u(x1, x2) = xi^(α) * x2^(β), where α and β are both greater than zero, satisfies the following properties:
(a) Local non-satiation:
This property states that at each point of the consumption set, there is always another bundle that is arbitrarily close and strictly preferred. Thus, the function has local non-satiation.
(b) Decreasing marginal utility for both goods 1 and 2: The marginal utility of a good measures the utility obtained by consuming one more unit of it. The marginal utility of x1 can be obtained as:
MU1 = α * xi^(α−1) * x2^(β)
The marginal utility of x2 can be obtained as:
MU2 = β * xi^(α) * x2^(β−1)
Therefore, both marginal utilities are decreasing in x1 and x2, satisfying this property.
(c) Quasi-concavity:
The Cobb-Douglas function is quasi-concave. This means that the upper contour set of any level set of the function is convex. This can be proved by taking the second partial derivative of the function and checking whether it is negative or not.
(d) Homotheticity:
The Cobb-Douglas function is homothetic. This means that its shape is independent of the total level of utility. The proof can be achieved by checking whether the function is homogeneous of degree one or not. This is true, since multiplying the inputs by any positive scalar λ leads to a proportional increase in the output.
In conclusion, the Cobb-Douglas utility function satisfies all four properties - local non-satiation, decreasing marginal utility for both goods 1 and 2, quasi-concavity, and homotheticity.
Learn More About marginal utility from the below link:
https://brainly.com/question/15050855
#SPJ11
Sam made 30% of goals in his last 6 games. The points his team scored in the games were 112, 89, 95, 77 and 103 points. On average how many goals did Sam score in each of the last 6 games.
Answers
Answer:
28.56
Step-by-step explanation:
First we need to calculate the mean average of the numbers
Average = 112+89+95+77+103/5
Average = 476/5
Average = 95.2
If Sam made 30% of goals in his last 6 games, then on average, amount made is expressed as;
Average of the last six games = 30% of 95.3
Average of the last six games = 0.3 * 95.2
Average of the last six games = 28.56
Hence the required point average is 28.56
a 127 foot tower is located on a hill a guy-wire is to be attached to the top of the tower and anchored at a point 64 feet downhill from the base of the tower the guy-wire is 175 feet long find the angle the tower makes with the hill
Answers
The angle the tower makes with the hill is approximately 44.77 degrees.
To find the angle the tower makes with the hill, we can use trigonometry. Let's denote the angle the tower makes with the hill as θ.
Using the given information, we can form a right triangle with the tower, the guy-wire, and the horizontal distance between the anchor point and the base of the tower.
The vertical leg of the triangle represents the height of the tower, which is 127 feet.
The hypotenuse of the triangle represents the length of the guy-wire, which is 175 feet.
The horizontal leg of the triangle represents the distance from the anchor point to the base of the tower, which is 64 feet.
We can use the sine function to find the angle θ:
sin(θ) = opposite/hypotenuse
sin(θ) = 127/175
To find θ, we can take the inverse sine (sin^(-1)) of both sides:
\(θ = sin^(-1)(127/175)\)
Using a calculator, we find that θ is approximately 44.77 degrees
Therefore, the angle the tower makes with the hill is approximately 44.77 degrees.
Learn more about trigonometric ratios on:
brainly.com/question/10417664
#SPJ4