please help me!!
In the first month of the new year, the Midwest region secured sales orders from 362 current stores and 66 new stores. Is this data consistent with the sales director’s model?
In the first month of the new year, the South region secured sales orders from 197 current stores and 115 new stores. Is this data consistent with the sales director’s model?


Answers
In the first month of the new year, the Midwest region secured sales orders, the data in comparison to the sales director’s model is mathematically given as
S=85%S'=15%What is the data in comparison to the sales director’s model?Generally, the equation for sale percentage for current stores is mathematically given as
\(S=\frac{Current stores sale}{Total store sale}\)
Therefore
S=362/428
S=85%
In conclusion, the equation for sale percentage for new stores is mathematically given as
\(S=\frac{new stores sale}{Total store sale}\)
Therefore
S=66/428
S'=15%
Read more about sale percentage
https://brainly.com/question/26765608
#SPJ1
Related Questions
PLEASE HELP!!! i can’t find the answer

Answers
Answer:
the correct answer is 2nd hope this will help u i have find the value of x also hope it will help
Step-by-step explanation:
\( {4}^{x + 3} = 64 \\ {2}^{2(x + 3)} = {2}^{6} \\ 2{ }^{2x + 6} = 2 {}^{6 } \\ 2x + 6 = 2 \\ 2x = 2 - 6 \\ x = \frac{ - 4}{2} \\ x = - 2\)
what are the properties of a diamond plane shape
Answers
Step-by-step explanation:
A diamond is a two-dimensional flat quadrilateral with four closed straight sides. A diamond is also called a rhombus because it's sides are of equal measure and because the inside opposite angles are equal. Diamonds are also considered to be parallelograms because their opposite sides are parallel to each other
A machine produces 264 bolts in 42 minutes. At the same rate, how many minutes would it take to produce 308 bolts?
minutes
Answers
Answer:
49.6 minutes to create 308 bolts
Step-by-step explanation:
hope this helps :)
what is the range of the function f(x)= -(x+3)^2+7
Answers
Answer:
thats the answer to rhjs problem

Find the general solution of the given differential equation. y dx − 6(x + y8) dy = 0
x(y) = 3y8+Cy6
Give the largest interval over which the general solution is defined. (Think about the implications of any singular points. Enter your answer using interval notation.)
?
Determine whether there are any transient terms in the general solution. (Enter the transient terms as a comma-separated list; if there are none, enter NONE.)
?
Answers
1) Rewrite the given differential equation: The given differential equation is y dx - 6(x + y^8) dy = 0. We can rewrite it as: y dx = 6(x + y^8) dy
2. Separate variables:
Now, separate the variables x and y:
(y/6) dx = (x + y^8) dy
3. Integrate both sides:
Integrate both sides of the equation with respect to their corresponding variables:
∫(y/6) dx = ∫(x + y^8) dy
(1/12)xy = (1/2)xy + (1/9)y^9 + C
4. Solve for x(y):
To find the general solution in form x(y), rearrange the equation: x(y) = 3y^8 + Cy^6
5. Find the largest interval:
To find the largest interval over which the general solution is defined, consider the domain of y.
The general solution is defined for all y except y = 0 (since this would result in a division by zero). Therefore, the largest interval is: (-∞, 0) ∪ (0, ∞)
6. Determine transient terms:
Since the general solution does not contain any terms that tend to zero as y approaches infinity, there are no transient terms. So the answer is: NONE
To know more about term click here
brainly.com/question/19774850
#SPJ11
4. [8] Convert the following numbers from binary to octal and hexadecimal. a. 1010101110₂ b. 101010011100₂ 5. [4] Convert the following numbers from octal to hexadecimal. a. 746128 b. 2746358 6. [
Answers
4. Conversion of binary to octal and hexadecimal.
1010101110₂
OCTAL: Group the binary digits into groups of three, starting from the right.
Add leadings 0's if necessary.
So, 1010101110 is 001 010 101 110.
Now, substitute each group with an octal digit.
001 010 101 110₂ = 2 5 5 6₈
HEXADECIMAL: Group the binary digits into groups of four, starting from the right.
Add leading 0's if necessary.
So, 1010101110 is 0101 0101 1100.
Now, substitute each group with a hexadecimal digit.
0101 0101 1100₂ = 5 5 C₁₆
b. 101010011100₂
OCTAL: Group the binary digits into groups of three, starting from the right.
Add leadings 0's if necessary.
So, 101010011100 is 001 010 100 111 000.
Now, substitute each group with an octal digit.
001 010 100 111 000₂ = 2 5 4 7 0₈
HEXADECIMAL: Group the binary digits into groups of four, starting from the right.
Add leadings 0's if necessary.
So, 101010011100 is 0101 0100 1110.
Now, substitute each group with a hexadecimal digit.
0101 0100 1110₂ = 5 4 E₁₆.
5. Conversion of octal to hexadecimala.
746128:
Group the octal digits into groups of three, starting from the right.
Add leadings 0's if necessary.
So, 746128 is 007 461 028.
Now, substitute each group with a hexadecimal digit.
007 461 028₈ = 0 3 1 4 0 2₁₆
b. 2746358:
Group the octal digits into groups of three, starting from the right.
Add leadings 0's if necessary.
So, 2746358 is 010 111 100 110 101 100.
Now, substitute each group with a hexadecimal digit.
010 111 100 110 101 100₈ = 2 7 C 6 5 4₁₆.
6. Conversion of hexadecimal to octal.
To know more about hexadecimal visit:
https://brainly.com/question/28875438
#SPJ11
5. A pole has to be erected at a point on the boundary of a circular park of diameter 13 metres in
such a way that the differences of its distances from two diametrically opposite fixed gates A
and B on the boundary is 7m. Is it possible to do so? If yes, at what distances from the two gates
should the pole be erected?
Answers
Answer:
Thus, it is possible to erect a pole at a point p on the boundary of the park at distances 5m and 12m respectively from gates B and A.
Step-by-step explanation:
Let P be the required location of pole .
A and B are the two gates.
Let the distance of pole at p from gate be 'x' meters
i.e. PB = x m
•°• AP = (x + 7)m and AB = 13 m
•°• AP² + BP² = AB² => (x +7)² + x =13²
Rejecting x =-12, we get x = 5
•°• PB = 5m
and
AP = 12m
(I hope this helped :)
Derek needs 2 gallons water to mix a sport drink , but he only has a 1 cup measuring cup.
Answers
Answer:
He can fill up his fill up his measuring cup 8 times that is pretty simple
Step-by-step example
4 cups is in one gallon
Suppose that people who own a NumberKrunch computer for home use will purchase another NumberKrunch with a probability of 0.6 and will switch to a QuickDigit computer with a probablify of 0.4. Those who own a QulckDigit will pairchase another with probability 0.7 and cwitch to a Numberkrunch with a probabilty of 0.3. Find the probability that if a person has a Numberkrunch computer, two computer purchases later he or she will also buy a Numberikrinch coenputer. The probability is (Type an integer or a decimal)
Answers
The probability that a person who initially owns a NumberKrunch computer will also buy another NumberKrunch computer after two purchases is 0.36
To solve this problem, we can use a Markov chain to model the computer purchasing behavior. Let's define the states as follows:
State 1: Owns a NumberKrunch computer
State 2: Owns a QuickDigit computer
The transition matrix for this Markov chain is:
P = | 0.6 0.3 |
| 0.4 0.7 |
The element P[i, j] represents the probability of transitioning from State i to State j. For example, P[1, 1] = 0.6 represents the probability of staying in State 1 (NumberKrunch) when currently in State 1.
To find the probability that after two computer purchases a person who initially owns a NumberKrunch computer will also buy a NumberKrunch computer, we need to calculate the probability of transitioning from State 1 to State 1 after two transitions:
P(X = 1) = P[1, 1] * P[1, 1]
Substituting the values from the transition matrix:
P(X = 1) = 0.6 * 0.6 = 0.36
Therefore, the probability is 0.36.
Learn more about matrix from the given link:
https://brainly.com/question/29132693
#SPJ11
Compute derivatives and solve application problems involving derivatives: Differentiate f(x) = x³ + 4x² CALEDON 9x + 8.
Answers
The derivative of f(x) = x³ + 4x² is f'(x) = 3x² + 8x.
To differentiate the function f(x) = x³ + 4x², we can use the power rule for differentiation.
According to the power rule, the derivative of \(x^n\) with respect to x is \(nx^{(n-1)\), where n is a constant.
Applying the power rule to each term in the function f(x) = x³ + 4x², we get:
f'(x) = d/dx (x³) + d/dx (4x²)
\(= 3x^{(3-1)} + 2(4x^{(2-1)})\)
= 3x² + 8x
So, the derivative of f(x) = x³ + 4x² is f'(x) = 3x² + 8x.
Now, let's solve an application problem involving this derivative.
Application problem: A particle moves along a straight line with a velocity given by v(t) = 3t² + 8t, where t represents time in seconds.
Find the acceleration of the particle.
Solution: The acceleration of the particle is given by the derivative of the velocity function v(t) with respect to time.
v'(t) = d/dt (3t² + 8t)
\(= 2(3t^{(2-1)}) + 8(1t^{(1-1)})\)
= 6t + 8
So, the acceleration of the particle is a(t) = 6t + 8.
Learn more about applications of derivatives click;
https://brainly.com/question/31583544
#SPJ4
The length of a banner is 4.7 feet long. The banner is too short and needs to be extended by 2.3 feet. What is the length of the banner after the extension?
Answers
Answer:
7.0
Step-by-step explanation:
4.7+2.3=7.0
Hi!
Your answer would be: 7 Feet Long.
Reason being: 4.7 ft + 2.3 ft = 7 ft
Hope this helps you!
Let me know if you need any more help, I'd be delighted to help you!
Yours truly,
~~~PicklePoppers~~~Reinforced concrete beam having a width of 500 mm and an effective depth of 750 mm is reinforced with 5 – 25mm p. The beam has simple span of 10 m. It carries an ultimate uniform load of 50 KN/m. Use f'c = 28 MPa, and fy = 413 MPa. Determine the ultimate moment capacity in KN- m when two bars are cut at a distance from the support. Express your answer in two decimal places.
Answers
The ultimate moment capacity of the reinforced concrete beam when two bars are cut at a distance from the support is approximately 157.10 kN-m, expressed in two decimal places.
To determine the ultimate moment capacity of the reinforced concrete beam when two bars are cut at a distance from the support, we need to consider the bending moment and the reinforcement provided.
Given:
Width of the beam (b): 500 mm
Effective depth (d): 750 mm
Reinforcement diameter (ϕ): 25 mm
Span (L): 10 m
Ultimate uniform load (w): 50 kN/m
Concrete compressive strength (f'c): 28 MPa
Steel yield strength (fy): 413 MPa
First, we need to calculate the neutral axis depth (x) based on the given dimensions and reinforcement.
For a rectangular beam with tension reinforcement only, the neutral axis depth is given by:
\(x = (A_{st} * fy) / (0.85 * f'c * b)\)
Where:
\(A_{st\) = Area of steel reinforcement
\(A_{st\) = (number of bars) × (π × (ϕ/2)²)
Given that there are 5 - 25 mm diameter bars, the area of steel reinforcement is:
\(A_{st\) = 5 × (π × (25/2)²)
= 5 × (π × 6.25)
= 98.174 mm²
Converting \(A_{st\) to square meters:
\(A_{st\) = 98.174 mm² / (1000 mm/m)²
= 0.000098174 m²
Now we can calculate the neutral axis depth:
x = (0.000098174 m² × 413 MPa) / (0.85 × 28 MPa × 0.5 m)
= 0.025 m
Next, we calculate the moment capacity (Mu) using the formula:
Mu = (0.85 × f'c × b × x × (d - 0.4167 × x)) / 10 + (A_st × fy × (d - 0.4167 × x)) / 10
Plugging in the values:
Mu = (0.85 × 28 MPa × 0.5 m × 0.025 m × (0.75 m - 0.4167 × 0.025 m)) / 10 + (0.000098174 m² × 413 MPa × (0.75 m - 0.4167 × 0.025 m)) / 10
Calculating the above expression, we get:
Mu ≈ 157.10 kN-m
Therefore, the ultimate moment capacity of the reinforced concrete beam when two bars are cut at a distance from the support is approximately 157.10 kN-m, expressed in two decimal places.
To know more about bending moment, visit
https://brainly.com/question/31862370
#SPJ11
please help with the question asked

Answers
Answer:
C, None of the above
Step-by-step explanation:
Step 1: Expand the brackets
-7 - 3(-4e-3)
-7 + 12e + 9
Step 2: Collect like terms
12e + 2
Because the answer is not a or b the answer is 'None of the Above'
Hey there! I'm happy to help!
Let's use the distributive property to undo the parentheses. We multiply the number next to the parentheses by each number inside of the parentheses.
-7+3(-4e-3)
-7-12e-9
We combine like terms.
-16-12e
This means that Answer B is incorrect. Answer A could still be correct though as it could have equal value to -16-12e.
-4(3e+4)
We use distributive property.
-12e-16
We see that these have the same value, so the correct answer is A.
Have a wonderful day! :D
Y=X-2
-X+Y=10
A)Parallel
B)neither
C)perpendicular
Answers
Answer:]]
The slope of these two linear equations is the same so the would be Parallel.
y = mX + B where m is the slope.
In this case the slope is 1 for both equations.
y = x -2
y = x + 10
They would be exactly 12 units apart too.
Step-by-step explanation:
Find the shortest distance from the point (2,0,-3) to the plane x+y+z=1
Answers
Shortest distance from the point (2, 0, -3) to the plane x + y + z = 1 is √3 units.
How to find the shortest distance from a point to a plane?To find the shortest distance between a point and a plane, we can use the formula:
distance = |ax + by + cz + d| / √(a² + b² + c²)
where a, b, and c are the coefficients of the plane's equation, d is the constant term, and (x, y, z) is the coordinates of the point.
In this case, the plane is x + y + z = 1, so a = 1, b = 1, c = 1, and d = -1. The point is (2, 0, -3), so x = 2, y = 0, and z = -3. Plugging in these values, we get:
distance = |1(2) + 1(0) + 1(-3) - 1| / √(1² + 1² + 1²)
= 3 / √3
= √3
Therefore, the shortest distance from the point (2, 0, -3) to the plane x + y + z = 1 is √3 units.
Learn more about constant term.
brainly.com/question/26099230
#SPJ11
Please help im desperate. :)

Answers
The U.S. Senate consists of 100 senators, with 2 from each of the 50 states. There are 50 Democrats in the Senate. A committee of size 10 is formed, by picking a random set of senators such that all sets of size 10 are equally likely. a) Find the expected number of Democrats on the committee. b) Find the expected number of states represented on the committee (by at least one senator) c) Find the expected number of states such that both of the state's senators are on the committee.
Answers
Note that based on probabilities,
The expected number of Democrats on the committee is 5.
The expected number of states represented on the committee is 26.
The expected number of states such that both of the state's senators are on the committee is 9.09.
How is this so ?a) The probability that a randomly chosen senator is a Democrat is 50/100 = 1/2.
So, the expected number of Democrats on a committee of size 10 is
1/2 * 10 = 5.
b) The minimum number of states that can be represented on a committee of size 10 is 2, and the maximum numberis 50.
The expected number of states representedon the committee is the average of these two values, which is (2 + 50)/ 2 = 26.
c) There are 50 states, and each state has 2 senators. So, there are a total of 100 pairs of senators.
The probability that a randomly chosen pair of senators both belong to the same state is 50/100 * 49/99
= 1/11.
The expected number of states such that both of the state's senators are on the committee is 100 * 1/11 = 9.09.
Learn more about probability at:
https://brainly.com/question/30390037
#SPJ4
list the first five terms of the sequence. an = [(−1)^n−1 / 3n] a1 = ___
a2 = ___
a3 = ___
a4 = ___
a5 = ___
Answers
The first five terms of the sequence. an = [(−1)^n−1 / 3n] a1 = 1/3, a2 = -1/6, a3 = 1/9, a4 = -1/12 and a5 = 1/15
\(a1 = (-1)^0 / (3*1) = 1/3\\a2 = (-1)^1 / (3*2) = -1/6\\a3 = (-1)^2 / (3*3) = 1/9\\a4 = (-1)^3 / (3*4) = -1/12\\a5 = (-1)^4 / (3*5) = 1/15\\\)
The sequence is given by the formula an = [(−1)^(n−1) / 3n]. To find the first five terms, simply plug in the values of n from 1 to 5:
a1 = [(−1)^(1-1) / 3(1)] = [1 / 3] = 1/3
a2 = [(−1)^(2-1) / 3(2)] = [-1 / 6] = -1/6
a3 = [(−1)^(3-1) / 3(3)] = [1 / 9] = 1/9
a4 = [(−1)^(4-1) / 3(4)] = [-1 / 12] = -1/12
a5 = [(−1)^(5-1) / 3(5)] = [1 / 15] = 1/15
So, the first five terms of the sequence are:
a1 = 1/3
a2 = -1/6
a3 = 1/9
a4 = -1/12
a5 = 1/15
To learn more about terms visit;
brainly.com/question/26099230
#SPJ11
A(1,5), B(-4,-3), C(3,-3), and find the area of the triangle it forms.
Answers
Answer:
first calculate the lenght of a triangle with the distance formula
1. Let the distribution of X be the normal distribution N (μ, σ2) and let Y = aX + b. Prove that Y is distributed as N (aμ + b, a2σ2).
2. Let X and Y be two independent random variables with E|X| < [infinity], E|Y| < [infinity] and E|XY| < [infinity]. Prove that E[XY] = E[X]E[Y].
Answers
1 Y is distributed as N(aμ + b, a^2σ^2), as desired.
2 We have shown that under these conditions, E[XY] = E[X]E[Y].
To prove that Y is distributed as N(aμ + b, a^2σ^2), we need to show that the mean and variance of Y match those of a normal distribution with parameters aμ + b and a^2σ^2, respectively.
First, let's find the mean of Y:
E(Y) = E(aX + b) = aE(X) + b = aμ + b
Next, let's find the variance of Y:
Var(Y) = Var(aX + b) = a^2Var(X) = a^2σ^2
Therefore, Y is distributed as N(aμ + b, a^2σ^2), as desired.
We can use the definition of covariance to prove that E[XY] = E[X]E[Y]. By the properties of expected value, we know that:
E[XY] = ∫∫ xy f(x,y) dxdy
where f(x,y) is the joint probability density function of X and Y.
Then, we can use the fact that X and Y are independent to simplify the expression:
E[XY] = ∫∫ xy f(x) f(y) dxdy
= ∫ x f(x) dx ∫ y f(y) dy
= E[X]E[Y]
where f(x) and f(y) are the marginal probability density functions of X and Y, respectively.
Therefore, we have shown that under these conditions, E[XY] = E[X]E[Y].
Learn more about distributed here:
https://brainly.com/question/29664127
#SPJ11
can there be a rectangular prism with the surface area of 120
Answers
Yes there can be rectangular prism with the surface area of 120.
What is Surface Area?A 3D object's surface area is the entire area that all of its faces cover. For instance, the surface area of a cube is its surface area if we need to determine how much paint is needed to paint it. It is consistently expressed in square units.
We have to find the dimension for rectangular prism whose Surface area 120.
let the length be x cm, width be y cm and height be z cm
So, the Surface Area
= 2( lw + wh + lh)
= 2(xy + yz + zx)
120=2( xy + yz + zx)
60 = xy + yz + zx
Now, we have to choose dimension such that they satisfy 60 = xy + yz + zx.
Yes this is possible to have a rectangular prism.
Learn more about Surface area here:
https://brainly.com/question/29298005
#SPJ1
Help me please please

Answers
Answer:
a is the answer I hope you have a great day
Answer:
A.)
B.)
Step-by-step explanation:
i looked it up and only a and b show 10
In electromagnetic theory, the magnetic potential u at a point on the axis of a circular coil is given by u = Ar dx/ (r2 +x2)(3/2)
where A,r,a are constants Compute
U:___
Hint: The integration is a little tricky: Substitute x =rtan 0.
Answers
The magnetic potential U at a point on the axis of a circular coil is U = A / r (2/3).
How magnetic potential calculated?To compute the magnetic potential U at a point on the axis of a circular coil, we can use the given formula:
u = Ar dx/ (r²+ x²)\(^(3/2)\)
where A, r, and x are constants. To perform the integration, we can use the substitution x = r tan θ. This gives:
dx = r sec²θ dθ
r²+ x²= r² + r² tan²θ = r² sec² θ
(r²+ x²)\(^(3/2)\) = (r² sec²θ)\(^(3/2)\)= r³ sec³θ
Substituting these expressions into the formula for u, we get:
u = Ar dx/ (r² + x²)\(^(3/2)\)
= Ar r sec²θ dθ / (r²sec² θ)\(^(3/2)\)
= A / r (cos θ)³dθ
Integrating this expression with respect to θ from 0 to π/2 gives:
U = ∫u dθ from 0 to π/2
= A / r ∫cos³θ dθ from 0 to π/2
To evaluate this integral, we can use the reduction formula:
∫cos\(^n\) θ dθ = (1/n) cos\(^(n-1)\) θ sin θ + ((n-1)/n) ∫cos\(^(n-2)\) θ dθ
Applying this formula with n = 3 gives:
∫cos³θ dθ = (1/3) cos²θ sin θ + (2/3) ∫cos θ dθ
= (1/3) cos²θ sin θ + (2/3) sin θ
Substituting this back into the expression for U and evaluating it gives:
U = A / r ∫cos³θ dθ from 0 to π/2
= A / r [(1/3) cos²θ sin θ + (2/3) sin θ] from 0 to π/2
= A / r (2/3)
Therefore, the magnetic potential U at a point on the axis of a circular coil is U = A / r (2/3).
Learn more about magnetic potential
brainly.com/question/30120799
#SPJ11
5|x +9| < 10
x< ?
x> ?
Answers
Answer:
x<−7 and x>−11
Step-by-step explanation:
Let's solve your inequality step-by-step.
5(|x+9|)<10
Step 1: Divide both sides by 5.
5(|x+9|)/5 < 10/5
|x+9|<2
Step 2: Solve Absolute Value.
|x+9|<2
We know x+9<2 and x+9>−2
x+9<2(Condition 1)
x+9−9<2−9(Subtract 9 from both sides)
x<−7
x+9>−2(Condition 2)
x+9−9>−2−9(Subtract 9 from both sides)
x>−11
Answer:
x<−7 and x>−11
\(\huge\textsf{Hey there!}\)
\(\huge\textbf{Equation:}\)
\(\mathbf{5|x + 9| < 10}\)
\(\huge\textbf{Solving:}\)
\(\mathbf{5|x + 9| < 10}\)
\(\huge\textbf{DIVIDE 5 to BOTH SIDES:}\)
\(\mathbf{\dfrac{5|x \ + \ 9|}{5} = \dfrac{10}{5}}\)
\(\huge\textbf{Simplify it!}\)
\(\mathbf{|x + 9| < 2}\)
\(\huge\textbf{Solve the absolute value:}\)
\(\mathbf{|x + 9| < 2}\)
\(\huge\textbf{Possibly we know that:}\)
\(\mathbf{x + 9 < 2 \ and\ we\ know\ that \ x+9 > -2}\)
\(\huge\textbf{Solving for:}\)
\(\mathbf{x + 9 < 2}\)
\(\huge\textbf{SUBTRACT 9 to BOTH SIDES:}\)
\(\mathbf{x + 9 - 9 < 2 -9}\)
\(\huge\textbf{Simplify it!}\)
\(\mathbf{x < -7}\)
\(\huge\textbf{Solving for:}\)
\(\mathbf{x + 9 > -2}\)
\(\huge\textbf{SUBTRACT 9 to BOTH SIDES:}\)
\(\mathbf{x + 9 - 9 > -2 - 9}\)
\(\huge\textbf{Simplify it!}\)
\(\mathbf{x > -11}\)
\(\huge\textbf{Answer(s):}\)
\(\huge\boxed{\mathsf{x < -7\ or\ x > -11}}\huge\checkmark\)
\(\huge\text{Good luck on your assignment \& enjoy your day!}\)
~\(\frak{Amphitrite1040:)}\)
help please please i beg

Answers
Answer:
6²
Ste㏒㏒㏑p-by-step explanation:
Answer:
\(x=-1\)
Step-by-step explanation:
\(3^{x}+3^{x+2}=\frac{10}{3} \\=> 3^{x} + 3^{x}*3^{2}= \frac{10}{3} \\\\=> 3^{x} (1+9)=\frac{10}{3} \\=> 3^{x}=\frac{10}{3}:10\\=> 3^{x}=\frac{1}{3} \\ \\=> x=-1\)
A manufacturer needs coil springs that can stand a load of at least 20.0 pounds. Among two suppliers, Supplier A can supply coil springs that, on the average, can stand a load of 24.5 pounds with a standard deviation of 2.1 pounds, and Supplier B can supply coil springs that, on the average, can stand a load of 23.3 pounds with a standard deviation of 1.6 pounds. If we can assume that the distributions of these loads can be approximated with normal distributions, determine which of the two suppliers can provide the manufacturer with the smaller percentage of unsatisfactory coil springs
Answers
The supplier with the smaller probability will provide the manufacturer with a smaller percentage of unsatisfactory coil springs.
To determine which of the two suppliers can provide the manufacturer with the smaller percentage of unsatisfactory coil springs, we need to calculate the probabilities of the coil springs not meeting the load requirement of 20.0 pounds for each supplier.
For Supplier A:
Mean load capacity (μA) = 24.5 pounds
Standard deviation (σA) = 2.1 pounds
To calculate the probability of a coil spring from Supplier A not meeting the load requirement:
P(A < 20.0) = P(Z < (20.0 - μA) / σA)
For Supplier B:
Mean load capacity (μB) = 23.3 pounds
Standard deviation (σB) = 1.6 pounds
To calculate the probability of a coil spring from Supplier B not meeting the load requirement:
P(B < 20.0) = P(Z < (20.0 - μB) / σB)
Using the standard normal distribution, we can look up the corresponding probabilities for the calculated Z-values. A smaller probability indicates a smaller percentage of unsatisfactory coil springs.
Calculating the Z-values:
Z_A = (20.0 - 24.5) / 2.1
Z_B = (20.0 - 23.3) / 1.6
Using the Z-table or a calculator, we can find the corresponding probabilities for Z_A and Z_B.
To learn more about probability visit;
https://brainly.com/question/31828911
#SPJ11
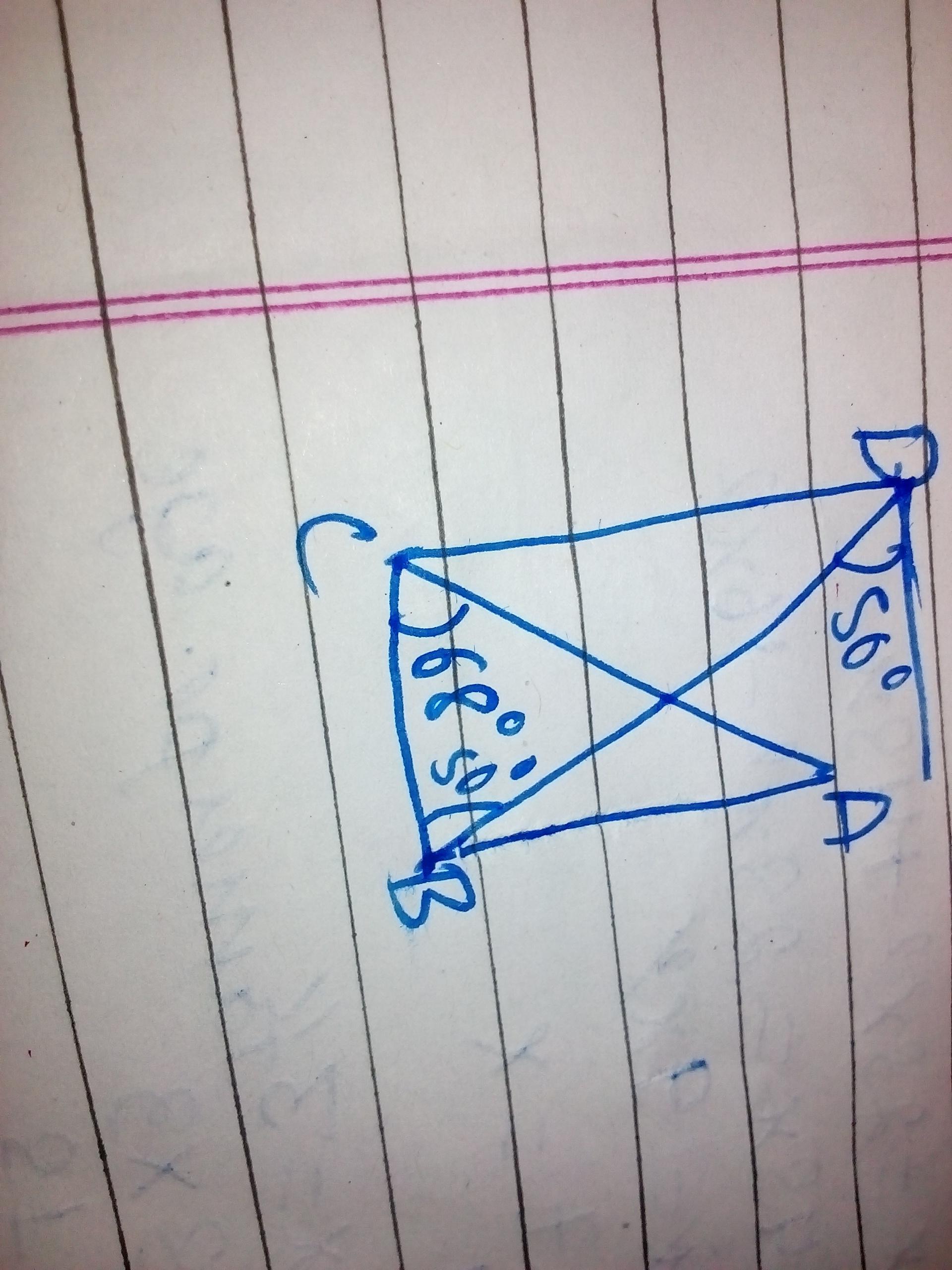
An observer stationed 20 m away from a tall
of elevation of the top of the building is 68° and the angle of depression of
its foot is 50 degrees. Calculate the height of the building.
Answers
Answer:
Step-by-step explanation:I don't say you must have to mark my ans as brainliest but my friend if it has really helped you plz don't forget to thank me...

Jason tossed a fair coin 3 times. what is the probability of getting a head and two tails in any order?
Answers
Step-by-step explanation:
fair coin is two sided Head H and Tail T
thrown 3 times
number of sample space n(s)=2³=>8
sample space (s)={HHH,HHT,HTH,HTT,THH,TTH,THT,TTT}
no of a head and two tails= 3/8
Write the equation in logarithmic form: 2^-3=0.125
Show your steps
Answers
The equation 2⁻³ = 0.125 in logarithmic form is -3log(2) = log(0.125)
What is an equation?An equation is an expression that shows the relationship between two or more numbers and variables.
A variable can either be dependent or independent. A dependent variable depend on other variable while an independent variable does not depend on other variables.
Given the equation:
2⁻³ = 0.125
Taking the logarithm of both sides:
log(2⁻³) = log(0.125)
-3log(2) = log(0.125)
The equation 2⁻³ = 0.125 in logarithmic form is -3log(2) = log(0.125)
Find out more on equation at: https://brainly.com/question/13763238
#SPJ1
Evaluate 9/m + 4 when m = 3
Answers
Answer:
Step-by-step explanation:
9/3 = 3
3+4 = 7
The answer is 7!
Answer:
7
Step-by-step explanation:
First, plug in m.
9/3+4
Because this equation has multiple operations, we need to use the order of operations, aka PEMDAS. It stands for Parentheses, Exponents, Multiplication/Division, and Addition/Subtraction. First in the order of operations that we have in this equation is multiplication/division. So, we need to divide 9/3 before adding.
9/3=3
The equation is now simplified to 3+4, which is 7.
Hope this helps! Have a great day :D