Answers
Answer:
What would you like me to help you with? ╰_╯
Related Questions
A parent rewards a child with 50 cents for each correctly solved mathematics problem and fines the child 30 cents for each incorrectly solved problem. If the child nets $22.00 after 100 problems. How many problems were solved correctly?
Answers
Answer:
65
Step-by-step explanation:
Let c = number of correct answer.
Let i = number of incorrect answers.
There are 100 questions, so
c + i = 100
The correct answers are worth 0.5c.
The incorrect answers are worth -0.3i (a negative value because 30 cents is taken away for each incorrect answer)
The total worth of the answers is 0.5c - 0.3i. The total worth of the answers is $22.00, so the second equation is
0.5c - 0.3i = 22
We have a system of two equations in 2 unknowns:
c + i = 100
0.5c - 0.3i = 22
We'll use the substitution method to solve the system of equations.
Solve the firt equation for c:
c = 100 - i
Now substitute 100 - i for c in the second equation.
0.5(100 - i) - 0.3i = 22
50 - 0.5i - 0.3i = 22
-0.8i = -28
i = 35
c = 100 - i
c = 100 - 35
c = 65
Answer: 65 problems were solved correctly.
a. (5) The demand function for a good X is Qx= m-3Px+2Py, where m is income, Px is the price of X, Py is the price of a related good Y and Qx is the demand for X. Income and prices are all positive. X
Answers
The demand function for good X is Qx = m - 3Px + 2Py, where Qx is the quantity demanded of X, m is income, Px is the price of X, and Py is the price of a related good Y. The equation shows that the demand for X is inversely related to its price and directly related to the price of Y. Income, price of X, and price of Y collectively affect the overall demand for X.
The demand function for good X is given by Qx = m - 3Px + 2Py, where Qx represents the quantity demanded of good X, m is the income, Px is the price of good X, and Py is the price of a related good Y. In this equation, the income and prices are assumed to be positive.
To determine the demand for good X, we can analyze the equation. The coefficient -3 in front of Px indicates that the demand for good X is inversely related to its price. As the price of X increases, the quantity demanded of X decreases, assuming other factors remain constant. On the other hand, the coefficient 2 in front of Py indicates that the demand for good X is directly related to the price of the related good Y. If the price of Y increases, the quantity demanded of X also increases, assuming other factors remain constant.
Furthermore, the term (m - 3Px + 2Py) represents the overall effect of income, price of X, and price of Y on the quantity demanded of X. If income (m) increases, the quantity demanded of X increases. If the price of X (Px) increases, the quantity demanded of X decreases. If the price of Y (Py) increases, the quantity demanded of X increases.
To know more about the factors affecting the demand for good X, refer here:
https://brainly.com/question/32566433#
#SPJ11
find the discriminant of 2x square + x - 6 is equal to zero?
Answers
Answer:
When the discriminant value is zero, we get one real solution; When the discriminant value is negative, we get a pair of complex solutions; Standard Form. The standard discriminant form for the quadratic equation ax 2 + bx + c = 0 is. Discriminant, D = b 2 – 4ac. Where. a is the coefficient of x 2. b is the coefficient of x. c is a constant term.
Estimated Reading Time: 50 secs
Step-by-step explanation:
in the graph of the simple linear regression equation, the parameter ß 1 is the _____ of the true regression line.
Answers
In the graph of the simple linear regression equation, the parameter ß1 is the slope of the true regression line.
The simple linear regression equation represents a linear relationship between a dependent variable and an independent variable. It can be written as y = ß0 + ß1x, where ß0 is the intercept and ß1 is the slope of the regression line.
The slope (ß1) determines the rate of change in the dependent variable (y) for each unit change in the independent variable (x). It represents the steepness or inclination of the regression line. The sign of ß1 indicates whether the line has a positive or negative slope, indicating the direction of the relationship between the variables.
Thus, in the context of the simple linear regression equation, the parameter ß1 is the slope of the true regression line.
learn more about regression equation here
https://brainly.com/question/32162660
#SPJ11
Please help will give brainist

Answers
Answer:
80 & 105
Step-by-step explanation:
Help please!!! Which graph represents a system of equations with NO SOLUTION. Explain how you know.

Answers
Answer:graph B
Step-by-step explanation:because if a line is parallel they have no solutions with a certain slope
If two lines intersect then they must have at least a solution
Pls help ASAP
Simplify \(\sqrt{.64x^2}\) if \(x\leq 0\)
The answer is not (4/5)x
Answers
Please help me solve this math problem... I will mark you brainliest

Answers
hope you understand and it's right. :)
All the best!

A data-entry clerk spends $86 per week for food. This is 20% of his weekly income. What is the weekly income? Show work please :
Answers
Answer:
$430
Step-by-step explanation:
Let the weekly income be x
Money spent on food= $86
% of weekly income spent on food = 20
20% of weekly income in terms = 20/100 * x = x/5
This, income is equal to $86 as given
thus,
x/5 = 86
x = 86*5 = 430
Thus, weekly income is $430.
what percentage of 7 1/2cm is 2 1/2cm?
Answers
Answer:
To find the percentage of 2 1/2 cm in 7 1/2 cm, we need to divide 2 1/2 by 7 1/2 and then multiply the result by 100 to get the percentage.
First, let's convert the mixed fractions into improper fractions:
7 1/2 = (7 x 2 + 1)/2 = 15/2
2 1/2 = (2 x 2 + 1)/2 = 5/2
Now, we can divide 5/2 by 15/2:
(5/2) ÷ (15/2) = 5/2 x 2/15 = 1/3
So, 2 1/2 cm is 1/3 of 7 1/2 cm.
To convert this fraction to a percentage, we need to multiply by 100:
1/3 x 100 = 33.33%
Therefore, 2 1/2 cm is 33.33% of 7 1/2 cm.
PLEASE HELP ASAP! WRITE A SHORT PARAGRAPH PLEASE!
How would you use the 60° angle to find sine and cosine of 120°, 240°, and 300°?
Answers
Answer:
120° will be found on in the second quadrant of the unit circle. Specifically, the reference angle will be 60°. This is because the angle measurement starts from the positive x axis, and rotates 120° counter-clockwise. The angle formed at this point between the line and the x-axis (negative direction) is 60°.
The special right triangle formed will have a hypotenuse of 1, and a reference angle of 60°.
We know that the adjacent line, the line opposite the 30° angle, will be 1/2 the hypotenuse, or -1/2. Negative because it goes in the negative x direction.
The opposite end, or vertical side, will be √3/2.
sin(120) = opposite/hypotenuse = √3/2 ÷ 1 = √3/2
cos (120) = adjacent/hypotenuse = (-1/2) / 1 = -1/2
tan (120) = opposite / adjacent = (√3/2) / (-1/2) = -√3
Step-by-step explanation:
Hope this helps...
show that if the polynomial p(z)=anz^n an-1z^n-1 .. a0 is written in factored form as p(z)=an(z-z1)^d1 (z-z2)^d2 …(z-zr)^dr, then
Answers
Yes, the polynomial p(z)=anz^n an-1z^n-1 .. a0 is written in factored form as p(z)=an(z-z1)^d1 (z-z2)^d2 …(z-zr)^dr.
How to prove the relation?To show that if the polynomial p(z) is written in factored form as\(p(z) = an(z-z1)^d1 (z-z2)^d2 ...(z-zr)^dr\), then the coefficients satisfy the following relations:
The degree of the polynomial p(z) is n, which means that the sum of the exponents of the factors in the factored form is equal to n:
\(d1 + d2 + ... + dr = n\)
The coefficient of the highest degree term in p(z) is an, which means that:
\(an = ad1d2*...*dr\)
To prove these relations, we start by expanding the factored form of p(z) using the distributive property:
\(p(z) = an(z-z1)^d1 (z-z2)^d2 ...(z-zr)^dr\)
\(= an*(z^d1 - z1^d1)(z^d2 - z2^d2)...*(z^dr - zr^dr)\)
The degree of p(z) is the highest power of z in this expression, obtained by adding up the highest exponents of z in each term.
Since each factor \((z - zi)\)has a maximum exponent of di, the highest power of z in\(p(z) is d1 + d2 + ... + dr.\)
\(n = d1 + d2 + ... + dr\)
The coefficient of the highest degree term in the expanded form of p(z) is obtained by multiplying the highest degree terms in each factor.
we compare it with the coefficient of the highest degree term in the original polynomial p(z).
\(an*(z1^d1)(z2^d2)...*(zr^dr)\)
On the other hand, the coefficient of the highest degree term in p(z) is the coefficient of \(z^n,\) which is an. Therefore, we have:
\(an = an*(z1^d1)(z2^d2)...*(zr^dr)\)
Dividing both sides by the product of the z-terms, we get:
\(1 = (z1^d1)(z2^d2)...*(zr^dr)\)
Multiplying both sides by an, we get:
\(an = ad1d2*...*dr\)
This proves that the coefficients of the polynomial p(z) satisfy the relations stated above.
Learn more about polynomial
brainly.com/question/11536910
#SPJ11
A circular tablecloth is 6 feet in diameter. How many feet of ribbon would be required
to edge the circumference of this tablecloth? Use 3.14 as the value of pi. Round to
the nearest whole number.
Answers
Answer:
You'll need 18.85 feet of ribbon.
Step-by-step explanation:
The circumference is \(\pi d\).
\(\pi * 6 = 18.85\)
Increase £854 by 89%
Give your answer rounded to 2 DP
Answers
Answer:
\({ \tt{ = (100 + 89)\%}} \\ = { \tt{(189\% \times £854)}} \\ = £1614.06\)
2n + 5 = 35 what is the value of n?
Answers
Answer:
\(15\)Step-by-step explanation:
2n+5=35
2n=35-5
2n=30
n=15
Answered by
\(*GraceRosalia*\)
Answer:
n = 15
Step-by-step explanation:
To find the value of n we need to
2n + 5 = 35
Transpose
2n = 35 - 5
2n = 30
n = 30 ÷ 2
n = 15
HELPPPPPPP ASAPPPPPPPP PLSSSS

Answers
Answer:
the first one is. hope helps
In the standard normal distribution, where X ~ N(0, 1), what proportion of the area is greater than 1
Answers
In the standard normal distribution, approximately 15.87% of the area is greater than 1.
In the standard normal distribution, where X follows a normal distribution with a mean of 0 and a standard deviation of 1 (X ~ N(0, 1)), we can determine the proportion of the area that is greater than a specific value using the z-score.
To find the proportion of the area greater than 1, we need to calculate the cumulative probability to the right of 1.
This involves finding the z-score corresponding to 1 and then using a standard normal distribution table or a calculator to determine the proportion.
The z-score represents the number of standard deviations a particular value is from the mean.
In this case, to find the z-score for 1, we subtract the mean (0) from 1 and divide by the standard deviation (1), giving us a z-score of 1.
Using a standard normal distribution table or a calculator, we can find the proportion of the area to the right of 1 by looking up the z-score of 1.
The corresponding value in the table or calculator represents the proportion of the area to the left of that z-score.
Since we want the proportion to the right of 1, we subtract the value obtained from 1.
In this case, the proportion of the area greater than 1 in the standard normal distribution is approximately 0.1587 or 15.87%.
For similar question on standard normal distribution.
https://brainly.com/question/4079902
#SPJ11
The most popular lengths of paddle boards can be written by the function V(x) equals the absolute value of |x - 10|where x is the length of the paddleboard and v represents a variation of 4 feet. Complete the statement. The vertex of the function is ? Which represents ?
Answers
The vertex of the absolute function for the paddle boards is at x = 10.
Explain about the absolute function?A function that wraps an algebraic expression in absolute value symbols is known as an absolute value function.
Remember that a number's distance from 0 upon that number line represents its absolute value.f(x)=| x | denotes the parent function of absolute values.The function g(x)= f(x - h) can be used to translate this same absolute value function f(x)=| x | horizontally.The graph of f(x) is moved h units towards the right to obtain g(x) when h>0.So,
lengths of paddle boards is defined by :
V(x) = |x - 10|.
x is the length of the paddleboardv is variation of 4 feet.Thus, the vertex of the absolute function for the paddle boards is at x = 10.
Know more about the absolute function
https://brainly.com/question/24734454
#SPJ9
Answer:
Step-by-step explanation:
v(x)=||x-10||
3. the provider orders that a patient be given 1000 ml of iv normal saline to run over 10 hours. the drop factor of the selected tubing is 15. what is the correct rate of infusion in drops per minute?
Answers
Therefore, the correct rate of infusion in drops per minute is 25. In order to calculate the correct rate of infusion in drops per minute for the given scenario, we need to consider the following factors: the volume of IV normal saline, the time over which it should be administered, and the drop factor of the tubing.
The provider orders 1000 mL of IV normal salines to be given to the patient over 10 hours. We can first convert the time into minutes since the required answer is in drops per minute:
10 hours × 60 minutes/hour = 600 minutes
Now, we can calculate the rate of infusion in milliliters per minute:
1000 mL ÷ 600 minutes ≈ 1.67 mL/minute
Next, we need to consider the drop factor of the selected tubing, which is 15 drops/mL. To find the correct rate of infusion in drops per minute, we multiply the rate in milliliters per minute by the drop factor:
1.67 mL/minute × 15 drops/mL ≈ 25 drops/minute
Therefore, the correct rate of infusion for the patient's IV normal saline is approximately 25 drops per minute.
Learn more about infusion here:
https://brainly.com/question/28790508
#SPJ11
I NEED HELPP 7TH GRADE IXL MATHH

Answers
Answer:
-1 all the way to 5
Step-by-step explanation:
k/2 is always greater than -2
A lineshaft runs at 360 rpm. A 18 inches pulley on the same shaft is belt connected to a 12 inches pulley on the counter shaft. From a 15 inches pulley on the counter shaft, motion is transmitted to the machine. Compute and check the required diameter of the pulley on the machine to give a spindle speed of 660 rpm. Select the correct response: 16 inches 10.5 inches 8.5 inches None of them
Answers
To solve the given problem, you must first identify the ratios of the pulleys. The ratio of a pulley is the relationship between its own diameter and that of another pulley.The formula for finding the ratio is.
D1/D2 = N1/N2Where:
D1 = diameter of pulley 1D2
= diameter of pulley 2N1
= speed of pulley 1N2
= speed of pulley 2A lineshaft runs at 360 rpm. An 18 inches pulley on the same shaft is belt connected to a 12 inches pulley on the counter shaft. Therefore, the ratio of the pulleys on the line shaft to those on the counter shaft are:18/12 = 3/2This means that for every three revolutions of the 18 inches pulley, the 12 inches pulley will rotate twice or vice versa.
The motion is transmitted to the machine via a 15 inches pulley on the counter shaft. From the given problem, the spindle speed on the machine should be 660 rpm. To find the diameter of the pulley on the machine, use the formula:D1/D2 = N1/N218/12
= N1/660660*18/12
= N1N1
= 990 rpmTherefore, the ratio of the pulley on the counter shaft to that on the machine is:15/D2
= 990/660D2
= 10.0 inchesThe required diameter of the pulley on the machine is 10 inches. Therefore, the correct response is 10.5 inches. Answer: 10.5 inches.
To know more about formula visit:
https://brainly.com/question/20748250
#SPJ11
I need the answer for Y please.

Answers
Answer:
follow me and answer my question for 50 points
Step-by-step explanation:
Suppose you toss three unbalanced coins where each coin independently has a 1/3 chance of landing on a head. What is the distribution of X, if X is a random variable denoting the number of heads
Answers
The distribution of X, the number of heads obtained by tossing three unbalanced coins, has probabilities of 8/27 for X=0, 4/27 for X=1, 2/27 for X=2, and 1/27 for X=3.
The possible outcomes of a single coin toss are either a head or a tail, with probabilities of 1/3 and 2/3 respectively. Since we are tossing three coins, there are \(2^3 = 8\) possible outcomes, which we can list in a sample space:
{HHH, HHT, HTH, THH, HTT, THT, TTH, TTT}
where H represents a head and T represents a tail.
To find the probability of each outcome, we can simply multiply the probabilities of each individual coin toss. For example, the probability of getting HHT is \($\frac{1}{3}\cdot\frac{1}{3}\cdot\frac{2}{3}=\frac{2}{27}$\), since the first two coins must land on a head and the third coin must land on a tail.
We can then calculate the probability of each value of X, the number of heads, by adding up the probabilities of the outcomes that correspond to that value of X.
X = 0: P(X=0) = P(TTT) = \($\left(\frac{2}{3}\right)^3 = \frac{8}{27}$\)
X = 1: P(X=1) = P(HTT, THT, TTH) = \($3\cdot\frac{1}{3}\cdot\frac{2}{3}\cdot\frac{2}{3}=\frac{4}{27}$\)
X = 2: P(X=2) = P(HHT, HTH, THH) = \($3\cdot\frac{1}{3}\cdot\frac{1}{3}\cdot\frac{2}{3}=\frac{2}{27}$\)
X = 3: P(X=3) = P(HHH) = \($\left(\frac{1}{3}\right)^3=\frac{1}{27}$\)
Therefore, the distribution of X is:
X 0 1 2 3
P(X) 8/27 4/27 2/27 1/27
To learn more about probabilities
https://brainly.com/question/30034780
#SPJ4
Jacob picks a 5-digit even number.
The first digit is a prime number.
The third digit is odd.
The four digit is 8
How many different 5-digit number could he pick
Answers
Answer:
Total no ways can be taken
Step-by-step explanation:
Please help ~~~~~7th Grade
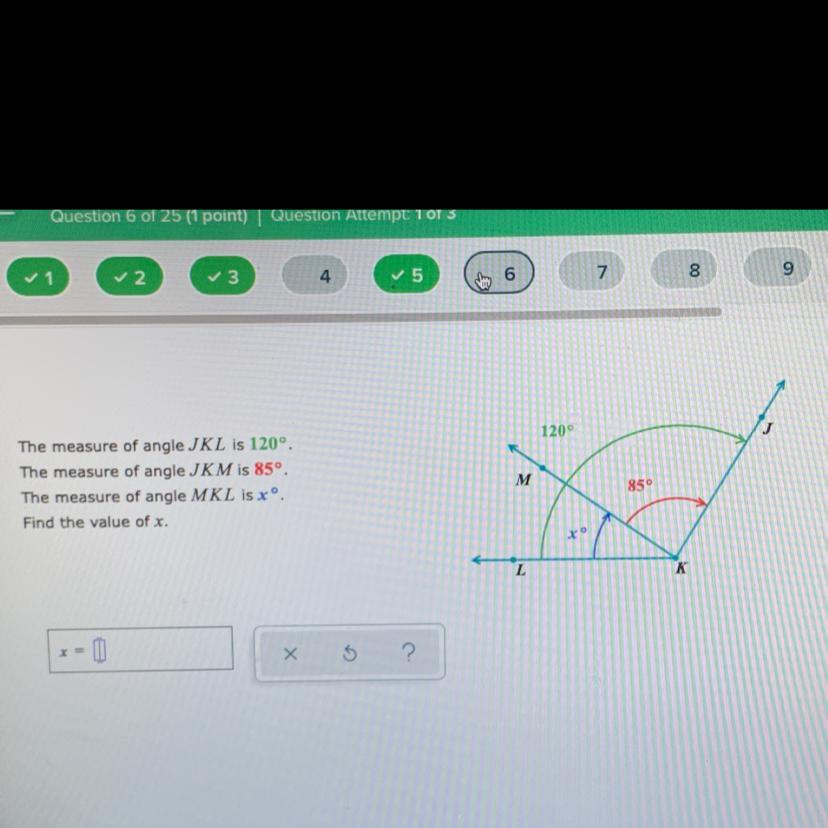
Answers
Answer: x = 35
Step-by-step explanation:
120 is the whole angle and 85 is part of that angle. Use the angle addition postulate:
85+ x = 120. Subtract 85 from both sides. X = 35.
New-Home Prices If the average price of a new one-family home is $246300 with a standard deviation of $ 15000, find the minimum and maximum prices of the houses that a contractor will build to satisfy the middle 70% of the market. Assume that the variable is normally distributed. Use a graphing calculator and round the answers to the nearest dollar.Minimum price: $=Maximum price: $=
Answers
Answer:
Minimum Price: $230,700
Maximum Price: $261,900
Explanation:
To find the minimum and maximum prices, we need to find the z-score of the middle 70%
70% = 0.7
1 - 0.7 = 0.3
0.3/2 = 0.15
Then the inverse normal of 0.15 is -1.04
\(z=\pm1.04\)Therefore,
\(\frac{x-246300}{15000}=\pm1.04\)\(\begin{gathered} x-246300=\pm15600 \\ x=246300\pm15600 \end{gathered}\)\(\begin{gathered} x=246300+15600 \\ =261900 \\ \text{MAXIMUM} \end{gathered}\)and
\(\begin{gathered} x=246300-15600 \\ =230700 \\ \text{MINIMUM} \end{gathered}\)HELP QUICK ...........

Answers
Answer:
By translating the graph of f(x) two units to the left
Answer:
down 2 units I think
Step-by-step explanation:
Help me please! Would be greatly appreciated! :)

Answers
Answer:
MR=20
Step-by-step explanation:
These appear to be isosceles triangles, so their bases are equal on both sides. For the little triangle in the center, one side is 6, so the other will also be 6. Now the larger one has MN=4, so QR=4 as well.
6+6+4+4=20.
i need help on this, i don't understand any of these


Answers
The baking soda that each mixing bowl will need is A. 1/9 cups.
How to calculate the value?A fraction simply means the part of a whole number. It's represented as a/b where a = numerator and b = denominator.
In this case, it can be seen that in the visual diagram, the value of B occurs in 3 places out of the 27 alphabets written.
This will be illustrated as:
= Number of B / Total alphabets
= 3 / 27
= 1/9.
Therefore, there'll be 1/9 cups.
Learn more about fractions on:
brainly.com/question/17220365
#SPJ1
I just need some help with the math for this 3-part problem.
Data for this question. A 180-cm3 soil sample was collected three days after a drenching rain when
the soil was assumed to be at field capacity (Not Saturated!). The wet weight of the sample was 274.1 g
and after drying overnight in an oven the sample weighed 214.7 g.
1. What was the gravimetric moisture content of this soil at field capacity?
1a. What is the dry bulk density of this sample?
1b. What is the porosity of this sample as a percent of the total volume?
Answers
1) The gravimetric moisture content of the soil at field capacity is approx 27.62%.
2) The dry bulk density of the sample is approximately 1.193 g/cm^3.
3) The porosity of the sample, as a percentage of the total volume, is approximately 54.96%.
1) The gravimetric moisture content of the soil at field capacity can be calculated using the following formula:
Gravimetric Moisture Content = ((Wet Weight - Dry Weight) / Dry Weight) * 100
Given:
Wet Weight = 274.1 g
Dry Weight = 214.7 g
Using the formula, we can substitute the values:
Gravimetric Moisture Content = ((274.1 - 214.7) / 214.7) * 100
Gravimetric Moisture Content = (59.4 / 214.7) * 100
Gravimetric Moisture Content ≈ 27.62%
The gravimetric moisture content of the soil at field capacity is approximately 27.62%.
The gravimetric moisture content represents the amount of water present in a given mass of soil. By subtracting the dry weight of the soil from the wet weight and dividing by the dry weight, we can determine the proportion of water in the sample. Multiplying by 100 gives the moisture content as a percentage.
1a. The dry bulk density of the sample can be calculated using the following formula:
Dry Bulk Density = Dry Weight / Sample Volume
Given:
Dry Weight = 214.7 g
Sample Volume = 180 cm^3
Substituting the values into the formula:
Dry Bulk Density = 214.7 g / 180 cm^3
Dry Bulk Density ≈ 1.193 g/cm^3
The dry bulk density of the sample is approximately 1.193 g/cm^3.
Dry bulk density refers to the mass of dry soil per unit volume. To calculate it, we divide the dry weight of the soil by the sample volume.
1b. The porosity of the sample can be calculated using the following formula:
Porosity = (1 - (Dry Bulk Density / Particle Density)) * 100
The particle density for soil is usually around 2.65 g/cm^3.
Given:
Dry Bulk Density = 1.193 g/cm^3
Particle Density = 2.65 g/cm^3
Substituting the values into the formula:
Porosity = (1 - (1.193 / 2.65)) * 100
Porosity ≈ 54.96%
The porosity of the sample, as a percentage of the total volume, is approximately 54.96%.
Porosity represents the void space within a soil sample. It is calculated by subtracting the ratio of the dry bulk density to the particle density from 1 and multiplying by 100 to get the percentage. In this case, the particle density of 2.65 g/cm^3 is a typical value for soil.
To know more about gravimetric moisture content, visit;
https://brainly.com/question/33657666
#SPJ11
