Rhombus wxyz is graphed on a coordinate plane. what is the area of the rhombus? 24 square units 28 square units 32 square units 48 square units
Answers
The area of the rhombus WXYZ is 48 unit².
From the diagram:
Diagonal WY = 8 units, Diagonal XZ = 6 units, hence:
Area = 8 * 6 = 48 unit².
A rhombus is a special case of a parallelogram. In a diamond, the opposite sides are parallel and the opposite sides are equal in angle. In addition, all sides of the rhombus are the same length and the diagonal bisects at right angles. Diamonds are also known as diamonds.
The diagonals of the rhombus intersect at right angles to form a non-uniform triangle. The opposite angles are equal. However, if all angles of the diamond are 90 degrees, the diamond is said to be square.
Learn more about rhombus here: https://brainly.com/question/20627264
#SPJ4

Related Questions
choose the abbreviation of the postulate or theorem that supports the conclusion that the triangles are congruent

Answers
ANSWER
LA
EXPLANATION
We are given two triangles ABC and DEF.
We are given that We are given that
We are alos given that BC = EF.
From the conditions given, we have that one set of corresponding angles are equal.
We are also given that one set of leg lengths are equal.
We can therefore conculde that the condition of congruence for these two triangles is Leg - Angle.
That is LA.
The following data shows the points scored by a basketball team during the first 13 games of the season.
{85, 94, 101, 118, 107, 110, 114, 96, 117, 105, 121, 88, 125}
Part A: Determine the best graphical representation to display the data. Explain why the type of graph you chose is an appropriate display for the data. (6 points)
Part B: Explain, in words, how to create the graphical display you chose in Part A. Be sure to include a title, axis label(s), scale for axis if needed, and a clear process of how to graph the data. (6 points)
Answers
Part A:
Best graphical representation to display data will be line graph.
Given,
Scores of basketball team during the first 13 games of the season
{85, 94, 101, 118, 107, 110, 114, 96, 117, 105, 121, 88, 125}.
Now.
The data of scores shows that the data is neither increasing constantly nor decreasing constantly. The data also indicates that the scores of the team is varying. So for this type of data when the scores are not constant and vary continuously the best way to represent will be through line graph.
Part B:
We can create line graph with a very simple technique.
Firstly,
On x - axis take the number of season the team has played. In our case the number is 13 so take 13 distinct points on the x - axis.
Secondly,
On y -axis take the scores of the team in each of the 13 seasons corresponding to their values at x - axis.
Then,
Plot the points on the graph for all 13 seasons .
Last step,
Join all the points in the graph with the help of ruler. This will form the required line graph of the question.
Know more about line graph,
brainly.com/question/31050175
#SPJ1
A collection of nickels and dimes is worth $2.85. If there
are 34 coins in the collection and each nickel is replaced
with a quarter, the value of the collection becomes:
O $13.50
O none of the above
O $5.05
O $8.50
Answers
In the collection, there are 11 nickels and 23 dimes. If each nickel is replaced with a quarter, the value of the collection becomes $5.05. So, C is correct. By writing them in the system of equations, we get the required value.
Conversion of the given units into dollars:The given units are nickels, dimes, and quarters. Their conversion into dollars is as follows:
1 nickel = $0.05
1 dime = $0.10
1 quarter = $0.25
Calculation:Given a collection of nickels and dimes is worth $2.85.
And there are 34 coins in the collection.
So, consider the number of nickel coins = n and the number of dime coins = d.
Then, the equation for the given collection is
n + d = 34...(1)
0.05n + 0.10d = 2.85...(2)
On simplifying (2), we get
5n + 10d = 2.85 × 100
⇒ 5n + 10d = 285
From (1), n = 34 - d, we get
5(34 - d) + 10d = 285
⇒ 170 - 5d + 10d = 285
⇒ 5d = 285 - 170 = 115
∴ d = 115/5 = 23
Thus, the number of dime coins is 23.
So, from (1), we get n + 23 = 34 ⇒ n = 34 - 23 = 11
∴ n = 11
Thus, the number of nickel coins is 11.
If each nickel coin in the collection is replaced with a quarter, then the value of the collection is
q + d = 34 and 0.25q + 0.10d = Y
Here q = 11; d = 23
So, we get
0.25(11) + 0.10(23) = Y
⇒ 2.75 + 2.3 = Y
⇒ 5.05 = Y
∴ Y = $5.05
The value of the new collection becomes $5.05.
Learn more about equations at the following link:
https://brainly.com/question/22688504
#SPJ1
the company sea esta has ten members on its board of directors. in how many different ways can it elect a president, vice-president, secretary and treasurer?
Answers
There are 10 members on the board, so there are 10 ways to elect a president, 9 ways to elect a vice president, 8 ways to elect a secretary, and 7 ways to elect a treasurer.
This is because each position can be filled by any of the members, except for the position that the member is already filling. For example, the president can be elected from any of the 10 members, but the vice president must be elected from the remaining 9 members.
The company sea esta has ten members on its board of directors, so it has a lot of different options for how to elect a president, vice-president, secretary, and treasurer. One way to elect a president would be to have the board members vote on who they want to be president.
Another way to elect a president would be to have the members of the company vote on who they want to be president. There are many different ways to elect officers, and it really depends on the company and what they want to do.
Learn more about election at : https://brainly.com/question/11185151
#SPJ4
what is a quadratic equation?
thanks :)
Answers
Answer:
It's basically an equation with multiple numbers (called "polynomial") and a single variable (usually "x").
Usually, there are three numbers in the equation, and it looks like this:
ax²+bx+c=0.
"a", "b", and "c" are numbers.
You are trying to solve for the variable, "x".
So it reads like this:
"First number times x squared, plus (or minus) a second number times x, plus (or minus) a third number."
Example:
6x² + 11x – 35 = 0
Definitions: A quadratic equation is a second-order polynomial equation in a single variable x ax2+bx+c=0. with a ≠ 0 . Because it is a second-order polynomial equation, the fundamental theorem of algebra guarantees that it has at least one solution. The solution may be real or complex.
THANKX✨ : D
~{ HAVE A BANGTAN DAY }~ANWSER both questions pls for brainly

Answers
Answer:
40x-24
3b(6c-3a+c)
Step-by-step explanation:
For the first one use distributive property and multiply each term by 8.
For the second one find a common factor which is 3b and divide all terms by that number.
Answer:
1). \(40x-24\)
2). D.
Step-by-step explanation:
PLEASE HELP WILL MARK BRAINLIEST!! DUE SOON

Answers
Answer:
i cant reall read the screen sorry
Step-by-step explanation:
Can someone please help me with this . will give brainliest :)
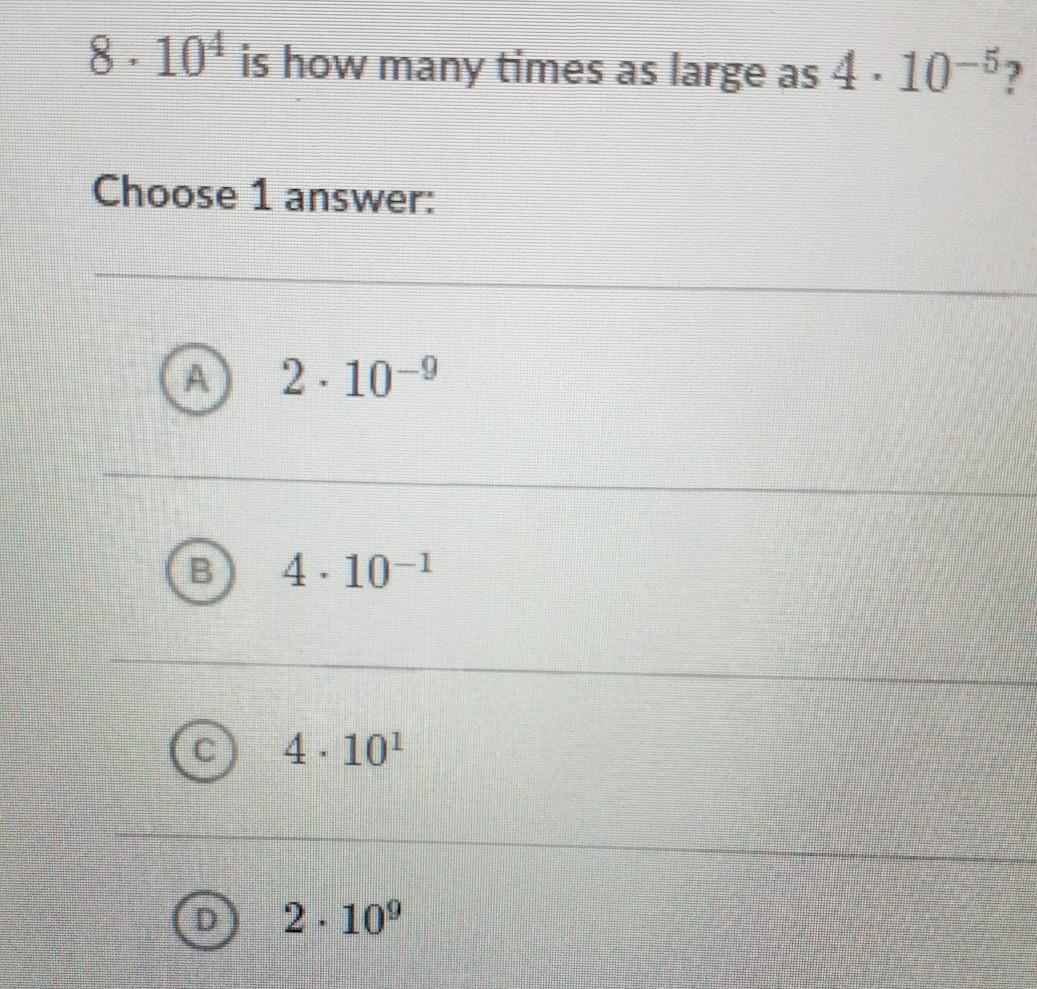
Answers
Answer:
Your answer is going to be letter D because:
.00004 x 2,000,000,000 = 80,000
D
can i get brainly?
Dr. Math's four-digit house number $ABCD$ contains no zeroes and can be split into two different two-digit primes ``$AB$'' and ``$CD$'' where the digits $A$, $B$, $C$ and $D$ are not necessarily distinct. If each of the two-digit primes is less than 40, how many such house numbers are possible
Answers
The total number possible house numbers is 2 × 28 = 56
From the question, we have the following information available is:
Dr. Math's four-digit house number ABCD contains no zeroes and can be split into two different two-digit primes AB and CD where the digits A, B, C and D are not necessarily distinct.
And, each of the two digit primes number is less than 40
We have to find the number of possible houses.
Now, According to the question:
We have to take out the primes number in between 1 to 40.
So, The prime numbers in between 1 to 40 are:
The two digit primes < 40 are
11, 13, 17, 19, 23, 29, 31, 37
Any two of these eight can be selected = C(8,2) = 28
And each of these can be arranged in two ways
Hence, the total number possible house numbers is 2 × 28 = 56
Learn more about Prime number at:
https://brainly.com/question/9315685
#SPJ4
I need help right now this due today(you have been in this situation).

Answers
Answer:
7.62
Step-by-step explanation:
We can draw another point at (-3, -4) named C to get a right-angled triangle.
AC = 2 (by counting)
BC = 7 (by counting)
Using Pythagoras Theorem,
\(AC^{2} +BC^{2} =AB^{2}\)
\(2^2+7^2=AB^2\)
\(4+49=AB^2\)
\(AB^2=58\)
\(AB=\sqrt{58} = 7.62\) (nearest hundredth)
Which of the following is the correct sequence of project phases? O A. Concept - Planning - Definition O B. Definition - Planning - Performance O C. Postcompletion - Performance - Planning OD. Performance - Concept - Planning
Answers
The correct sequence of project phases is Definition - Planning - Performance. The correct answer is B.
This is the typical order of project phases in a traditional project management approach. It starts with the definition phase, where the project's goals, the scope, and the requirements are established.
Then comes the planning phase, where the project plan is developed, including the allocation of resources, scheduling, and budgeting.
Finally, the performance phase begins, during which the project activities are executed, monitored, and controlled to ensure the successful project completion.
Therefore the sequence of project phase is Definition - Planning - Performance The correct answer is B.
Learn more about project phase at https://brainly.com/question/8963262
#SPJ11
SOMEONE PLS HELP ME!!!!!

Answers
Answer:
The answer is B
Answer: B because 48 divided by 6 is 8
What is the measure of <C? Round your answer to the nearest tenth of a degree.
A 73.4
B 74.1
C 106.6
D 100.7

Answers
Given:
In triangle \(ABC, AB=9,BC=4,AC=7\).
To find:
The measure of angle C.
Solution:
We have, \(AB=9,BC=4,AC=7\). It means \(c=9,a=4,b=7\).
According to the law of cosines:
\(cos C=\dfrac{a^2+b^2-c^2}{2ab}\)
\(cos C=\dfrac{4^2+7^2-9^2}{2(4)(7)}\)
\(cos C=\dfrac{16+49-81}{56}\)
\(cos C=\dfrac{-16}{56}\)
Taking cos inverse on both sides, we get
\(C=cos^{-1} \dfrac{-16}{56}\)
\(C=106.60155\)
\(C\approx 106.6\)
Therefore, the correct option is C.
A parking facility has five parking levels and a parking lot. If each level has 75
parking spaces and the lot has 120 spaces, how many cars will the facility hold
when it is completely full.(Please Show Your Work)
Answers
then add 120 from the lot to get 495
495
Step-by-step explanation:
(5 levels x 75 spaces each) + 120 spaces from lot
(5x75) + 120
495
4.
What is the trigonometric ratio for cos 0?
O opposite leg/adjacent leg
O hypotenuse/adjacent leg
O opposite leg/hypotenuse
O adjacent leg/hypotenuse
Answers
The trigonometric ratio for cos 0 is:
adjacent leg / hypotenuse
Why it is?
The definition of cosine of an angle in a right triangle is the ratio of the adjacent leg to the hypotenuse.
In the case of cos 0, the angle is 0 degrees, which means the adjacent leg of the right triangle is the same as the hypotenuse, since the adjacent side is opposite to an angle of 90 degrees and the hypotenuse is the longest side of the right triangle. Therefore, the ratio of the adjacent leg to the hypotenuse is 1/1 or simply 1.
So, the trigonometric ratio for cos 0 is:
adjacent leg / hypotenuse = 1/1 = 1
To know more about Trigonometry related questions, visit:
https://brainly.com/question/26719838
#SPJ1
a study conducted at a college shows that 90% of the school's graduates find a job in their chosen field with a year after graduation. find the probability that among 8 randomly selected graduates, at least one does not find a job in their chosen field within a year of graduating.
Answers
According to the question ,The probability that among 8 randomly selected graduates, at least one does not find a job in their chosen field within a year of graduating is 0.570
We are Given in the question that:
P = 0.1
q = 1-p
= 0.9
n = 8
Using binomial distribution P(n) = ⁿCₓ.pˣ.qⁿ⁻ˣ
We are Required to prove that F(x ≥ 1) = P(1) + ... + P(8)
= 8C₁(.1)¹(.9)²+...+8C₈(.1)⁸(.9)°
= 0.5695 ≈ 0.570
Hence the probability is 0.570
Learn more about Probability here:
brainly.com/question/25870256
#SPJ4
Point C is AB and Ab=30 m. Point c is 1.5 times farther from point A than point C is from point B. find Ac and CB.
Answers
Answer:
12, 18
Step-by-step explanation:
We can say that AC=1.5BC. Thus, since AC and CB add to 30, AC = 12 and BC= 18.
Help plz for this math question!!

Answers
Answer:
\(3x - 3 \leqslant 2 \times (x + 1) + x \geqslant 0 = \)
Step-by-step explanation:
\(3x - 3 \leqslant (2 \times x) - (2 \times 1) + x \geqslant 0\)
Answer the following: (10 points) a. Find the area to the right of z= -1 for the standard normal distribution. b. First year college graduates are known to have normally distributed annual salaries wi
Answers
The area to the right of z = -1 for the standard normal distribution is approximately 0.8413.
a. To find the area to the right of z = -1 for the standard normal distribution, we need to calculate the cumulative probability using the standard normal distribution table or a statistical calculator.
From the standard normal distribution table, the area to the left of z = -1 is 0.1587. Since we want the area to the right of z = -1, we subtract the left area from 1:
Area to the right of z = -1 = 1 - 0.1587 = 0.8413
Therefore, the area to the right of z = -1 for the standard normal distribution is approximately 0.8413.
b. To answer this question, we would need additional information about the mean and standard deviation of the annual salaries for first-year college graduates. Without this information, we cannot calculate specific probabilities or make any statistical inferences.
If we are provided with the mean (μ) and standard deviation (σ) of the annual salaries for first-year college graduates, we could use the properties of the normal distribution to calculate probabilities or make statistical conclusions. Please provide the necessary information, and I would be happy to assist you further.
Learn more about area here
https://brainly.com/question/25292087
#SPJ11
1) Find the most general antiderivative of the function. (Check your answer by differentiation. Use C for the constant of the antiderivative.)r(?) = sec(?) tan(?) ? 3e?2) What constant acceleration is required to increase the speed of a car from 30 mi/h to 57 mi/h in 3 seconds? (Round your answer to two decimal places.)Please solve both questions for rate
Answers
The antiderivative of the function is \([sec\theta]-3[e^{\theta}]+c\\\) and the constant acceleration is required to increase the speed of a car from 30 mi/h to 57 mi/h in 3 seconds is 13.20 ft/s².
Functions' antiderivative is often referred to as their integral. The original function is derived by differentiating the antiderivative of a function. The term "anti" derivatives refers to the process of integration, which is the opposite of differentiation.
Indefinite integrals are the standard name for antiderivatives. Nevertheless, antiderivatives can also be connected to definite integrals by applying the Basic Theorem of Calculus.
1) r(θ) = secθtanθ - 3\(e^{\theta}\)
Anti derivative,
\(\int {r(\theta)} \, d\theta=\int {sec\theta tan\theta \ d\theta } - 3\inte^{\theta}\)
\(= [sec\theta]-3[e^{\theta}]+c\\\)
2) 1hr = 3600 sec
1 minute = 5280 feet
The required formula to calculate acceleration
\(a=\frac{v_2-v_1}{t}\)
v1 = 30mi/h = 44 ft/sec
v2 = 57 mi/h = 83.6 ft/sec
time t = 3 sec
\(a=\frac{v_2-v_1}{t}\)
= \(\frac{83.6-44}{3}\)
= 39.6/3
= 13.20 ft/s².
Therefore, acceleration is required to increase the speed is 13.20 ft/s².
Learn more about Antiderivative:
https://brainly.com/question/20565614
#SPJ4
There are 312 students in the senior class. Four of the students will be chosen to be the President, Vice-President, Secretary, and Treasurer of the class. How many different ways can this happen?
Answers
Given:
Number of students = 312
Number to be chosen = 4
To find the number of ways this can happen, let's use combination meyhod.
Thus, we have:
\(\frac{312!}{4!(312\text{ - 4)!}}\)Solving further, we have:
\(=387278970\)Therefore there are 387,278,970 ways this can happen
ANSWER:
387,278,970 ways
what is s for the following function

Answers
11=3s+2
-2. -2
9=3s
9/3
=3
Answer:
S = 3
S = 5
S = 7
S = 9
Step-by-step explanation:
t = 3s + 2
when t = 11
11 = 3s + 2
11 – 2 = 3s
9 = 3s
s = 3
t = 3s + 2
when t = 17
17 = 3s + 2
17 – 2 = 3s
15 = 3s
s = 5
t = 3s + 2
when t = 23
23 = 3s + 2
23 – 2 = 3s
21 = 3s
s = 7
t = 3s + 2
when t = 29
29 = 3s + 2
29 – 2 = 3s
27 = 3s
s = 9
I HOPE ALL THIS HELPS
RATE AS BRAINLIEST PLS
Given a rectangular prism with width and height = 4x and length = 10x-6. The expression for the surface area of the rectangular prism shown in the picture is 2[(4x)(4x)] 4[(10x - 6)(4x)]. Simplify this expression.
Answers
3
is the simplify expression
Step-by-step explanation:
Given that the series the summation from k equals 1 to infinity of the quotient of k times the cosine of the quantity k times pi and the quantity k cubed plus 2 converges, suppose that the 3rd partial sum of the series is used to estimate the sum of the series. Which of the following values gives the best bound on the remainder (error) for this approximation?
a. 1/2
b. -3/29
c. 2/33
d. 1/5
Answers
The best bound on the remainder (error) for this approximation is c. 2/33
The given series converges, and we want to estimate the error when using the 3rd partial sum. Since the series is alternating (cosine of kπ is 1 for even k and -1 for odd k), we can use the Alternating Series Remainder Theorem. According to this theorem, the error is bounded by the absolute value of the next term after the last term used in the partial sum.
In this case, we use the 3rd partial sum, so the error is bounded by the absolute value of the 4th term:
|a₄| = |(4 * cos(4π)) / (4³ + 2)| = |(4 * 1) / (64 + 2)| = 4 / 66 = 2 / 33
Thus, the best bound on the remainder (error) for this approximation is c. 2/33
Learn more about converges here,
https://brainly.com/question/31433507
#SPJ11
Missy is constructing a fence that consists of parallel sides line AB and line EF. Complete the proof to explain how she can show that m∠AKL = 116° by filling in the missing justifications
Answers
The figure with explanation is given below .
When two rays meet each other is at a common point is called angle.
Given:
- A Fence with parallel sides AB and EF there is a point K on line AB point L on line EF
Angle AKL
We need to prove , m\(\angle AKL = 116^0\)
Proof:
\(m\angle AKL +m\angle KLE = 116^0\)
1. To create triangle AKL to draw a line KL.
2. Since AB is parallel to EF, we know that m∠AKL and m∠KLE are corresponding angles and are congruent.
3. Let x be the measure of angle KLE.
4. Since triangle AKL is a triangle, we know that the sum of its angles is \(180 ^0\) Therefore, m∠AKL + x + 64° = 180° (since m∠EKL = 64°, as it is a corresponding angle to m∠AKL).
5. Simplifying the equation in step 4, we get m\(\angle AKL +116^0\)
6. Since m\angle\(\angle KLE\) and m\(\angle AKL\) are congruent (as shown in step 2), we can substitute m∠KLE with x in the equation from step 5 to get m∠AKL + m∠KLE = 116°.
7. Combining like terms in the equation from step 6, we get m∠AKL = 116°.
Learn more about Triangle here :
https://brainly.in/question/54231692
#SPJ12

find the lateral area of this cone

Answers
Answer:
lateral area = 180π in²
Step-by-step explanation:
The lateral area is the surface of the cone only.
perimeter of base = 12 x 2 = 24π in
Surface Area = 1/2 perimeter x slant height
SA = 1/2 x 24π x 15 = 180π in²
What's More
Activity 1: Missing You!
Fill in the blank of the given equation. Write your answers on your answer sheet.
1. a² + 12a + 11 = (a + )(a + 11)
2. b² + 6b + 8 = (b + 2)(b+)
3. c² - 7c + 6 = (c-___) (c-1)
4. 2x² – 3x - 9 = (___x+3)(x-3)
5. 3x² + 5x - 2 = (___x - 1)(x+2)
Answers
1. 1
2. 4
3. 6
4. 2
5. 3
If Sale price = Rs 450 Discount amount = 50 Find the Discount % *
Answers
Answer:
The discount percentage is 11.1%
Step-by-step explanation:
Here, we want to find the percentage of discount
We simply place the discount amount over the sales price multiplied by 100
we have this as;
50/450 * 100%
= 1/9 * 100%
= 100%/9
= 11.1%
Kenji collected 1 3/4 pounds of newspaper for the recycling drive. Reagan collected 9 1/2 times as much newspaper as Kenji. How many pounds of the newspaper did Reagan collect?
Answers
Answer:
Reagan collected 16 5/8 pounds of newspaper
Step-by-step explanation:
Kenji = 1 3/4 lbs
Reagan = 9 1/2 times 1 3/4 lbs
To solve, find a common denominator:
1 3/4 and 9 1/21 6/8 and 9 4/8Now turn them into improper fractions:
1 6/8 = 14/89 4/8 = 76/8Multiply them together:
14/8 x 76/8 = 1064/641064/64 = 133/8133/8 = 16 5/8Just multiply
\(\\ \tt\hookrightarrow 9\dfrac{1}{2}\times 1\dfrac{3}{4}\)
\(\\ \tt\hookrightarrow \dfrac{19}{2}times \dfrac{7}{4}\)
\(\\ \tt\hookrightarrow \dfrac{19(7)}{2(4)}\)
\(\\ \tt\hookrightarrow 133/8\)
During an electronic store's Sale Days, the regular price of CD Players is discounted $10. There is a state sales tax of 5%, and the $10 discount is applied before the sales tax is calculated.
A. Write an expression that shows the regular price r of a CD Player minus the $10 discount.
B. Write a rule for the function p(r) that expresses the final price p of a CD Player with the discount applied and sales tax included
C. How much would you pay during Sale Days for a CD Player regularly priced at $29.50?
Answers
Answer:
A) r - 10
B) p(r) = 1.05(r - 10)
C) $20.48 (nearest cent)
Step-by-step explanation:
Part AIf r is the regular price of a CD player, then an expression for the regular price minus the $10 discount is:
r - 10Part BIf a state tax of 5% is applied to a sale, then the cost of the item will be 105% of its regular price. 105% expressed as a decimal is 1.05.
Therefore, we need to multiply the expression from part A by 1.05 to find the final price of the CD player:
p(r) = 1.05(r - 10)Part CTo calculate how much would you pay during Sale Days for a CD Player regularly priced at $29.50, substitute r = 29.5 into the function from part B:
⇒ p(r) = 1.05(29.50 - 10)
⇒ p(r) = 1.05(19.50)
⇒ p(r) = 20.475
Therefore, you would pay $20.48 (rounded to the nearest cent) for a CD Player during Sale Days.