Select three ratios that are equivalent to 11:1.
A(1:11
B(22:2
C(55:5
D(9:99
E(110:10
Answers
Answer:
22:2, 55:5, 110:10
Step-by-step explanation:
Related Questions
4. The diagram shows points A, B and C lying on the line 2y = x +4. The point A lies on the
y-axis and AB = BC. The line from D(10, -3) to B is perpendicular to AC. Calculate the
coordinates of B and C.
A
B
с
D(10,-3)
X

Answers
Considering the linear functions, the coordinates of B and C are given as follows:
B (6,5).C (12,8).What is a linear function?The slope-intercept representation of a linear function is given below:
y = mx + b
The coefficients of the function and their meaning are listed as follows:
m is the slope of the function, representing the rate of change of the linear function.b is the y-intercept of the function, giving the value of y when x = 0.The equation of line AC is given as follows:
2y = x + 4
y = 0.5x + 2.
When two lines are perpendicular, the multiplication of their slopes is of -1, hence the slope of line BD is:
0.5m = -1
m = -1/0.5
m = -2.
Then:
y = -2x + b
Considering point D, when x = 10, y = -3, hence the intercept is obtained as follows:
-3 = -20 + b
b = 17.
Point B is the intersection of these two lines, hence:
0.5x + 2 = -2x + 17
2.5x = 15
x = 15/2.5
x = 6
y = 0.5 x 6 + 2 = 5.
Point B is the midpoint of AC, that is, it's coordinates are the mean of the coordinates of A and C, hence the x-coordinate of C is obtained as follows:
6 = (0 + x)/2
x = 12.
The y-coordinate is obtained as follows:
5 = (2 + y)/2
2 + y = 10
y = 8.
More can be learned about linear functions at https://brainly.com/question/24808124
#SPJ1
Anders owns a hat shop.During a scale,he sells all his hats for 20% off.Is this a markup or a markdown
Answers
Please help ill give u brainliest!!!

Answers
.........................................
Answer:
221mile per day
Step-by-step explanation:
Mean=total distance her car traveled/days
11477-10150=1327
1327/6=221.167≈221mi
A factory made 3 jars of creamy peanut butter and 97 jars of chunky peanut butter. What percentage of the jars of peanut butter were creamy?
Answers
Answer:
3%
Step-by-step explanation:
They made 97+3 total jars, 3 out of 100 is 3 percent.
what is the product of -2 1/4 and -4 1/2
Answers
Answer:
i think 6 5/8
Step-by-step explanation:
So distribute
-2*-4+-2*1/2=7 1/2
1/4*-4+1/4*1/2=-7/8
7 4/8 - 7/8 is 6 5/8
what is the area of the rectangle below?

Answers
Answer:
8
Step-by-step explanation:
Mary used one big bag of flour. She baked four loaves of bread. Then, she used the remaining flour to make 48 muffins. How much flour was in the bag when Mary began? Please answer fast
Answers
4x + 5 = 2x + 9, find the value of x
Answers
Answer:
x =2
Step-by-step explanation:
4x - 2x = 9-5
2x = 4
x = 4
1. A right triangle LMN is given where: side MN = 8 side NL (the hypotenuse) =
10 What is the length of side LM?*
Answers
Step-by-step explanation:
\( \underline{ \underline{ \text{Given}}} : \)
Length of MN ( Base ) = 8 Length of NL ( Hypotenuse ) = 10\( \underline{ \underline{ \text{To \: find}}} : \)
Length of LM ( Perpendicular )\( \underline{ \underline{ \text{Using \: pythagoras \: theorem}}} : \)
\( \boxed{ \sf{ {Hypotenuse}^{2} = {Perpendicular}^{2} + {Base}^{2} }}\)
⤑ \( \sf{ Perpendicular = \sqrt{ {(Hypotenuse)}^{2} - {(Base)}^{2} } }\)
⤑ \( \sf{ \sqrt{ {(10)}^{2} - {(8)}^{2} } }\)
⤑ \( \sf{ \sqrt{100 - 64}} \)
⤑ \( \sf{ \sqrt{36}} \)
⤑ \( \boxed{ \sf{6\: units}}\)
\( \pink{ \boxed{ \boxed{ \tt{Our \: final \: answer : \boxed{ \underline { \tt{6 \: units}}}}}}}\)
Hope I helped ! ツ
Have a wonderful day / night ! ♡
▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁

Please help and show how you found the answer step by step.
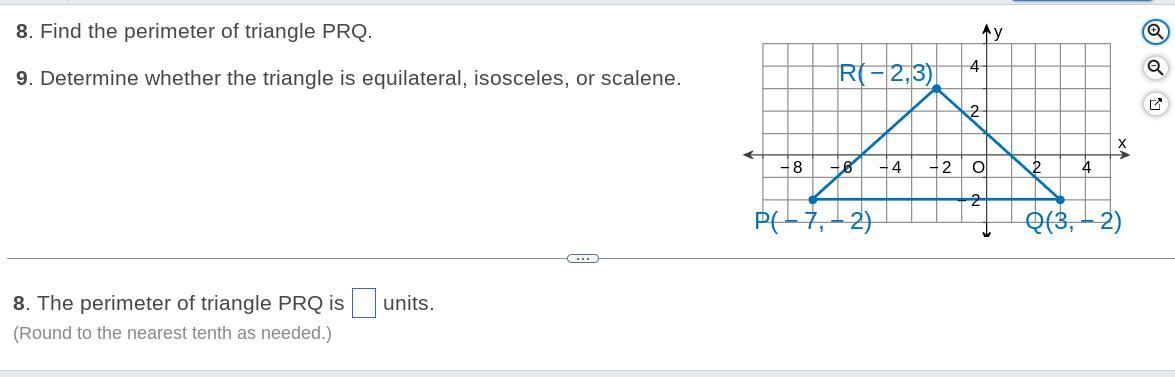
Answers
According to the information, the perimeter of the triangle is ≈ 31.18
How to calculate the perimeter of the triangle?To find the distance between two points, we can use the distance formula:
distance = sqrt((x2 - x1)^2 + (y2 - y1)^2)
Let's label the coordinates as follows:
Point 1: (-2, 3)
Point 2: (3, -2)
Now we can substitute these values into the distance formula:
distance = sqrt((3 - (-2))^2 + (-2 - 3)^2)
distance = sqrt((5)^2 + (-5)^2)
distance = sqrt(25 + 25)
distance = sqrt(50)
distance ≈ 7.07
Therefore, the distance from (-2, 3) to (3, -2) is approximately 7.07 units.
To find the perimeter of the triangle, we need to find the length of all three sides of the triangle and then add them up.
Using the distance formula, we can find the length of the sides:
Side 1: (-2,3) to (3,-2)
d = √[(3 - (-2))^2 + (-2 - 3)^2]
= √[5^2 + (-5)^2]
= √50
= 5√2
Side 2: (3,-2) to (-7,-2)
d = √[(-7 - 3)^2 + (-2 - (-2))^2]
= √[(-10)^2 + 0^2]
= 10
Side 3: (-7,-2) to (-2,3)
d = √[(-2 - (-7))^2 + (3 - (-2))^2]
= √[5^2 + 5^2]
= 5√2
Therefore, the perimeter of the triangle is:
5√2 + 10 + 5√2 = 15√2 + 10 ≈ 31.18 (rounded to two decimal places)
An two of the three sides are equal, so it is an isosceles triangle.
Lean more about isosceles triangle in: https://brainly.com/question/2456591
#SPJ1
please help me with i’ll give you brainlist

Answers
The box in the middle of the plot spans from the first quartile (Q1) to the third quartile (Q3), with a line inside representing the median.
What is whisker plot?A whisker plot, also known as a box and whisker plot, is a graphical representation of a set of numerical data through their quartiles. The plot consists of a box with whiskers extending from the top and bottom, showing the spread and distribution of the data. The five-number summary, which includes the minimum value, first quartile (Q1), median, third quartile (Q3), and maximum value, is used to create the whisker plot.
Here,
To create a box and whisker plot, we need to find the five-number summary of the data set, which includes:
Minimum value: the smallest value in the data set.
First quartile (Q1): the median of the lower half of the data set.
Median: the middle value in the data set.
Third quartile (Q3): the median of the upper half of the data set.
Maximum value: the largest value in the data set.
First, we need to put the data in order:
10, 12, 14, 15, 16, 18, 22, 24, 25, 28
The minimum value is 10 and the maximum value is 28.
The median is the middle value, which is 18.
To find the first quartile, we need to find the median of the lower half of the data set, which is:
10, 12, 14, 15, 16
The median of this lower half is 14.
To find the third quartile, we need to find the median of the upper half of the data set, which is:
22, 24, 25, 28
The median of this upper half is 24.
So, the five-number summary for this data set is:
Minimum value = 10
First quartile (Q1) = 14
Median = 18
Third quartile (Q3) = 24
Maximum value = 28
Now we can use this information to create the box and whisker plot:
| |
----+----+----+----+----
10 14 18 24 28
The box in the middle of the plot spans from the first quartile (Q1) to the third quartile (Q3), with a line inside representing the median. The whiskers extend from the box to the minimum and maximum values in the data set.
To know more about whisker plot,
https://brainly.com/question/2742784
#SPJ1
In the number 16.73, which number is in the ones place?
Answers
Answer:
6
hope this helps
have a good day :)
Step-by-step explanation:
100 Points! Algebra question. Photo attached. Please show as much work as possible. Thank you!

Answers
Answer: \((f \cdot g)(x) = x^4-11x^2+30.\)
Step-by-step explanation:
We want to find the value of \(f(g(x)).\) To do this, we plug in \(g(x)\) into the expression for \(f(x).\) This gives us:
\(f(g(x)) = (x^2-9)^2+7(x^2-9)+12 = x^4-18x^2+81+7x^2-63+12 = \boxed{x^4-11x^2+30}.\)
7. Use the Unit Circle to find the exact value of the trig function,
sin(180°)
Answers
PLZ HELP I WILL GIVE BRAINLIEST:
Find the average rate of change for the function f(x)= 3^x + 25
Using the intervals of x=2 to x=6.
PLZ SHOW WORK !
Answers
The average rate of change for the function f(x)= 3^x + 25 using the intervals of x=2 to x=6 is 6.75
The function is given as:
f(x) = 3^x + 25
The interval is given as:
x = 2 to 6
Start by calculating f(2) and f(6)
f(2) = 3^2 + 25 = 34
f(6) = 6^2 + 25 = 61
The average rate of change is then calculated as:
\(m = \frac{f(6) - f(2)}{6 -2}\)
This gives
\(m = \frac{61 - 34}{6 -2}\)
\(m = \frac{27}{4}\)
m = 6.75
Hence, the average rate of change is 6.75
Read more about average rate of change at:
https://brainly.com/question/8728504
can someone help me with this question?

Answers
Anna volunteers on the weekend at the Central Library. As a school project, she decides to record how many people visit the library, and where they go. On Saturday, 382 people went to The Youth Wing, 461 people went to Social Issues, and 355 went to Fiction and Literature. On Sunday, the library had 800 total visitors. Based on what Anna had recorded on Saturday, about how many people should be expected to go to The Youth Wing? Round your answer to the nearest whole number.
Answers
Based on the data recorded by Anna on Saturday, we can estimate the number of people expected to visit The Youth Wing on Sunday.
Let's calculate the proportion of visitors to The Youth Wing compared to the total number of visitors on Saturday:
\(\displaystyle \text{Proportion} = \frac{\text{Visitors to The Youth Wing on Saturday}}{\text{Total visitors on Saturday}} = \frac{382}{382 + 461 + 355}\)
Next, we'll apply this proportion to the total number of visitors on Sunday to estimate the number of people expected to go to The Youth Wing:
\(\displaystyle \text{Expected visitors to The Youth Wing on Sunday} = \text{Proportion} \times \text{Total visitors on Sunday}\)
Now, let's substitute the values into the equation and calculate the estimated number of visitors to The Youth Wing on Sunday:
\(\displaystyle \text{Proportion} = \frac{382}{382 + 461 + 355}\)
\(\displaystyle \text{Expected visitors to The Youth Wing on Sunday} = \text{Proportion} \times 800\)
Calculating the proportion:
\(\displaystyle \text{Proportion} = \frac{382}{382 + 461 + 355} = \frac{382}{1198}\)
Calculating the estimated number of visitors to The Youth Wing on Sunday:
\(\displaystyle \text{Expected visitors to The Youth Wing on Sunday} = \frac{382}{1198} \times 800\)
Simplifying the equation:
\(\displaystyle \text{Expected visitors to The Youth Wing on Sunday} \approx \frac{382 \times 800}{1198}\)
Now, let's calculate the approximate number of visitors to The Youth Wing on Sunday:
\(\displaystyle \text{Expected visitors to The Youth Wing on Sunday} \approx 254\)
Therefore, based on the data recorded on Saturday, we can estimate that around 254 people should be expected to go to The Youth Wing on Sunday.
\(\huge{\mathfrak{\colorbox{black}{\textcolor{lime}{I\:hope\:this\:helps\:!\:\:}}}}\)
♥️ \(\large{\underline{\textcolor{red}{\mathcal{SUMIT\:\:ROY\:\:(:\:\:}}}}\)
what is the domain of g

Answers
Answer:
We conclude that the set of numbers x satisfying -7 ≤ x ≤ 4 is an interval that contains -7, 4, and all numbers in between.
Thus, the domain of g is: -7 ≤ x ≤ 4
Step-by-step explanation:
We know that the domain of a function is the set of inputs or argument values for which the function is defined.
From the given graph, it is cleared that the function g starts from the x-value x = -7 and ends at x = 4.
It means the function is defined for the set of input values from x = -7 to x = 5 for which the function is defined.
Therefore, we conclude that the set of numbers x satisfying -7 ≤ x ≤ 4 is an interval that contains -7, 4, and all numbers in between.
Thus, the domain of g is: -7 ≤ x ≤ 4
Find an algebraic expression equivalent the given expression to sin(cos-1(x))
Answers
Answer: √(1 - x^2)
Step-by-step explanation: The inverse cosine function, cos^-1(x), gives the angle whose cosine is x. So, cos^-1(x) = θ where cos(θ) = x.Using the identity sin(cos^-1(x)) = √(1 - x^2), we can simplify the expression sin(cos^-1(x)) as:sin(cos^-1(x)) = √(1 - x^2)So, the algebraic expression equivalent to sin(cos^-1(x)) is √(1 - x^2).
this is simply a quick addition to the superb reply above by "MastG"
\(\textit{Pythagorean Identities} \\\\ \sin^2(\theta)+\cos^2(\theta)=1\implies \sin^2(\theta)=1-\cos^2(\theta)\implies \sin(\theta)=\boxed{\sqrt{1-\cos^2(\theta)}} \\\\[-0.35em] \rule{34em}{0.25pt}\)
\(sin( ~~ \underset{\theta }{cos^{-1}(x)} ~~ )\implies sin(\theta ) \\\\[-0.35em] ~\dotfill\\\\ cos^{-1}(x)=\theta \implies cos(\theta )=x\implies cos^2(\theta )=x^2 \\\\[-0.35em] ~\dotfill\\\\ \stackrel{\textit{so then we can say that}}{sin( ~~ cos^{-1}(x) ~~ )\implies sin(\theta )}\implies \sqrt{1-\cos^2(\theta)}\implies \boxed{\sqrt{1-x^2}}\)
Elena walked 12 miles. Then she walked 0.25 that distance. How far did she walk all together? Select all that apply. A: 12+0.25∙12 B: 12(1+0.25) C: 12∙1.25 D: 12∙0.25 E: 12+0.25
Answers
Answer:
Step-by-step explanation:
If Elena walk 12 miles, and then 0.25 that distance
0.25=1/4
12*1/4=3 miles is 1/4 of the distance
she walked in total 12+3=15 miles
choice A =12+12*1/4
Elena walked a total 12(1+0.25) distance.
What is a distance?The distance is the total movement of an object without any regard to direction.
First Elena walked 12 miles.
Then walked 0.25 or (1/4)th of 12 miles
i.e. 12 * (1/4) = 3
The total distance Elena walked is
12+3 = 15 miles or
12 + (1/4)*12 = 12(1+0.25)
Hence, 12(1+0.25) is the total distance walked by Elena.
To learn more about distance, use the link given below:
https://brainly.com/question/15172156
#SPJ2
what is
r+15=36
\(r + 15 = 36\)
Answers
Answer:
Step-by-step explanation:
R=21
It’s due late tonight can some pls help me pass

Answers
The x row goes up every time by two and the y decreases by 4.
6-4=2 ect...
MARK THE BEST PLZ!!
Above are two different models of the same rectangular hallway. If the length of the model on the top is 6 cm, what is the length of the model on the bottom?
Answers
Answer: 15cm
Step-by-step explanation: If the length of the model on the top is 6 cm, then the length of the model on the bottom must be 15 cm
Points x and y and points C,D,E and f are shown what is true about points
Answers
The true statement is "the line that can be drawn through points D and E is contained in plane Y".
option B is the correct answer
What are the true statements about the point x and y?The points x and y and points C,D,E and f are shown in the figure, the true statement is determined as follows;
We know that, if two points lie in a plane, then the line drawn through the points will contained in the same plane.
The points C lies outside the plane Y and the point D lies on the plane Y, so the line drawn through the points C and D will not contained in plane Y.
So, option (A) is NOT correct.
The points D and E, both lie in the plane Y, so the line drawn through the points D and E will also contained in plane Y.
So, option (B) is CORRECT.
Since the plane X contain infinitely many points, so F is not the only point that can lie in plane X.
So, option (C) is NOT correct.
Similarly, there are infinitely many points in the plane Y, so the points D and E are not the only points that can lie in plane Y.
So, option (D) is NOT correct.
Thus, the correct option is;
The line that can be drawn through points D and E is contained in plane Y.
Learn more about points on a plane here: https://brainly.com/question/31404411
#SPJ1
The complete question is below:
Points x and y and points C,D,E and f are shown what is true about points
(A) The line that can be drawn through points C and D is contained in plane Y.
(B) The line that can be drawn through points D and E is contained in plane Y.
(C) The only point that can lie in plane X is point F.
(D) The only points that can lie in plane Y are points D and E

Solve for x in simplest form.
4=1/6(5x—12)
Answers
Answer:
x = 2.4
Step-by-step explanation:
4 = 1/6 (5x - 12)
4 = 5/6x -2
5/6x - 2 = 4
5/6x = 2
x = 2.4
Determine the number of solutions to a system of equation:
Please help

Answers
Answer:
Step-by-step explanation:
These equations are all written in slope-intercept form, so the question is relatively easy to answer. These rules apply.
if slopes are different: 1 solutionif slopes are the same and y-intercepts are different, 0 solutionsif slopes are the same and y-intercepts are the same, infinitely many1. y=-6x-2; y=-6x-2 --- infinitely many
2. y=0.5x+5; y=0.5x+1 --- zero
3. y=0.25x-2; y=5x-4 --- one
4. y=2x+3; y=4x-1 --- one
5. y=2x+1.5; y=2x+1.5 --- infinitely many
6. y=-x-3; y=-x+3 --- zero
_____
Slope-intercept form is ...
y = mx +b
m is the slope
b is the y-intercept
Answer:
Step-by-step explanation: 1. y=-6x-2; y=-6x-2 --- infinitely many
2. y=0.5x+5; y=0.5x+1 --- zero
3. y=0.25x-2; y=5x-4 --- one
4. y=2x+3; y=4x-1 --- one
5. y=2x+1.5; y=2x+1.5 --- infinitely many
6. y=-x-3; y=-x+3 --- zero
11. In the square PQRS, the diagonals intersect
at O. M is a point on PS such that PM = PO.
Show that
Answers

Has anyone done the inverse functions mastery text on edmentum it’s super hard stuck on this question and the pictures wont help
Answers
Colleen is cutting pieces of string to tie around party bags for her sister’s
birthday party. The length of each piece of string needs to be 8.75 inches.
If she has 100 party bags, what is the length of string Colleen needs to tie
all the bags?
Answers
Answer:
875 inches
Step-by-step explanation:
almost 73 feet!
A union charges monthly dues of $6.00 plus $0.15 for each hour worked during the month. A union member's dues for March were $30.60. How many hours did the union member work during the month of March?
Answers
Answer:
4.5
Step-by-step explanation: