Answers
Answer:
d. -8x+102
Step-by-step explanation:
I also checked my answer, BTW. You can too, but i KNOW that it's correct. :)
Related Questions
Add using the number line.
7+(-10)
Drag and drop the word SUM to the correct value on the number line.
-18 -16 -14 -12 -10 -8 -6 -4 -2 0 2 4 6 8 10 12 14 16 18
SUM
Answers
7+(-1) = -3
Steps of adding 7+(-10) using number line are as following:
1) Draw a number line by ranging from -10 to +10.
2) Point out 7 on the number line.
3) Because -10 is added to 7 on the number line, please move 10 numbers left to 7 on the number line. We always go left in addition of a negative number on the number line.
4) The number we reach -3 on left side would be our final sum.
5) From the number we can clearly see that moving 10 number on left to 7, we get -3.
Note: We are moving left because of negative sign in front of 10.
to know more about number line visit:
brainly.com/question/11135636
#SPJ9

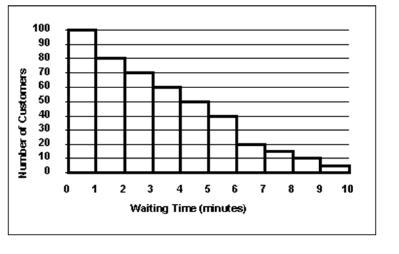
The staff of Mr. Wayne Wertz, VP of Operations at Portland Peoples Bank, prepared a frequency histogram of waiting time for walk-in customers. Approximately how many walk-in customers waited at least 6 minutes? (Note the position of the labels on the x-axis — the first column is the number of customers who waited 1 minute and the last column is the number of customers who waited 10 minutes)
Answers
The number of people who waited at least 6 minutes is given as follows:
50 people.
What is shown by the histogram?The height of each bin of the histogram represents the number of observations of the data-set in the desired interval.
The desired outcomes for this problem are given as follows:
Between 6 and 7 minutes: 20 people.Between 7 and 8 minutes: 15 people.Between 8 and 9 minutes: 10 people.Between 9 and 10 minutes: 5 people.Hence the number of people who waited at least 6 minutes is given as follows:
20 + 15 + 10 + 5 = 50 people.
More can be learned about histograms at brainly.com/question/25983327
#SPJ1
Find the coordinate of a point that partitions the segment AB, where A (0, 0) & B(6, 9) into a ratio of 2:1
Answers
let's call that point C, thus we get the splits of AC and CB
\(\textit{internal division of a line segment using ratios} \\\\\\ A(0,0)\qquad B(6,9)\qquad \qquad \stackrel{\textit{ratio from A to B}}{2:1} \\\\\\ \cfrac{A\underline{C}}{\underline{C} B} = \cfrac{2}{1}\implies \cfrac{A}{B} = \cfrac{2}{1}\implies 1A=2B\implies 1(0,0)=2(6,9)\)
\((\stackrel{x}{0}~~,~~ \stackrel{y}{0})=(\stackrel{x}{12}~~,~~ \stackrel{y}{18}) \implies C=\underset{\textit{sum of the ratios}}{\left( \cfrac{\stackrel{\textit{sum of x's}}{0 +12}}{2+1}~~,~~\cfrac{\stackrel{\textit{sum of y's}}{0 +18}}{2+1} \right)} \\\\\\ C=\left( \cfrac{ 12 }{ 3 }~~,~~\cfrac{ 18}{ 3 } \right)\implies C=(4~~,~~6)\)
To get from one term to the next in a sequence, we multiply by 2 and then
add 4.
The third term in the sequence is 48.
What is the first term in the sequence?
Answers
Answer: the first term in the sequence is 9.
Step-by-step explanation:
Let the primary term be x.
At that point the moment term is 2x + 4.
And the third term is 2(2x + 4) + 4 = 4x + 12.
Since the third term is given as 48, we will set up an condition and unravel for x:
4x + 12 = 48
4x = 36
x = 9
By some estimates __________ of employee learning occurs via on the job training.
a. 60-70 percent
b. 20-30 percent
c. 40-50 percent
d. 80-90 percent
Answers
By some estimates 80-90 percent of employee learning occurs via on the job training.
What does on the job training mean?
This is the term that is used to refer to the training that people would receive based on the fact that it is preparing them to have the competent skills that are useful for a particular job. It is one of the ways that employers help to get their employees ready for the task at hand.
It has been reported from available data that on the job training is a great component of employee learning.
Read more on on the job training here:
https://brainly.com/question/17057337
#SPJ1
Why does a logarithmic equation sometimes have an extraneous solution?
Answers
The main reason behind this is using properties of logarithm .
like
when solving
log_2(x+2)+log_2(x-3)=3There you use multiplication property and make addition to multiplication then you get extraneous solution because in plenty of cases they occur
like
(-2)²=(2)²But
-2≠2Same happens in case of logarithm
Answer:
yeah
Step-by-step explanation: l
If Y has a binomial distribution with parameters n and p, then p(hat)1 = Y/n is an unbiased estimator of p. Another estimator of p is p(hat)2 = (Y+1)/(n+2).
a. Derive the biase of p(hat)2.
b. Derive MSE(Pphat)1) and MSE(p(hat)2).
c. For what values of p is MSE(p(hat)1) < MSE(p(hat)2)?
Answers
a. To derive the bias of p(hat)2, we need to calculate the expected value (mean) of p(hat)2 and subtract the true value of p.
Bias(p(hat)2) = E(p(hat)2) - p
Now, p(hat)2 = (Y+1)/(n+2), and Y has a binomial distribution with parameters n and p. Therefore, the expected value of Y is E(Y) = np.
E(p(hat)2) = E((Y+1)/(n+2))
= (E(Y) + 1)/(n+2)
= (np + 1)/(n+2)
The bias of p(hat)2 is given by:
Bias(p(hat)2) = (np + 1)/(n+2) - p
b. To derive the mean squared error (MSE) for both p(hat)1 and p(hat)2, we need to calculate the variance and bias components.
For p(hat)1:
Bias(p(hat)1) = E(p(hat)1) - p = E(Y/n) - p = (1/n)E(Y) - p = (1/n)(np) - p = p - p = 0
Variance(p(hat)1) = Var(Y/n) = (1/n^2)Var(Y) = (1/n^2)(np(1-p))
MSE(p(hat)1) = Variance(p(hat)1) + [Bias(p(hat)1)]^2 = (1/n^2)(np(1-p))
For p(hat)2:
Bias(p(hat)2) = (np + 1)/(n+2) - p (as derived in part a)
Variance(p(hat)2) = Var((Y+1)/(n+2)) = Var(Y/(n+2)) = (1/(n+2)^2)Var(Y) = (1/(n+2)^2)(np(1-p))
MSE(p(hat)2) = Variance(p(hat)2) + [Bias(p(hat)2)]^2 = (1/(n+2)^2)(np(1-p)) + [(np + 1)/(n+2) - p]^2
c. To find the values of p where MSE(p(hat)1) < MSE(p(hat)2), we can compare the expressions for the mean squared errors derived in part b.
(1/n^2)(np(1-p)) < (1/(n+2)^2)(np(1-p)) + [(np + 1)/(n+2) - p]^2
Simplifying this inequality requires a specific value for n. Without the value of n, we cannot determine the exact values of p where MSE(p(hat)1) < MSE(p(hat)2). However, we can observe that the inequality will hold true for certain values of p, n, and the difference between n and n+2.
To learn more about binomial distribution: -brainly.com/question/29137961
#SPJ11
In the given scenario, we have two estimators for the parameter p of a binomial distribution: p(hat)1 = Y/n and p(hat)2 = (Y+1)/(n+2). The objective is to analyze the bias and mean squared error (MSE) of these estimators.
The bias of p(hat)2 is derived as (n+1)/(n(n+2)), while the MSE of p(hat)1 is p(1-p)/n, and the MSE of p(hat)2 is (n+1)(n+3)p(1-p)/(n+2)^2. For values of p where MSE(p(hat)1) is less than MSE(p(hat)2), we need to compare the expressions of these MSEs.
(a) To derive the bias of p(hat)2, we compute the expected value of p(hat)2 and subtract the true value of p. Taking the expectation:
E(p(hat)2) = E[(Y+1)/(n+2)]
= (1/(n+2)) * E(Y+1)
= (1/(n+2)) * (E(Y) + 1)
= (1/(n+2)) * (np + 1)
= (np + 1)/(n+2)
Subtracting p, the true value of p, we find the bias:
Bias(p(hat)2) = E(p(hat)2) - p
= (np + 1)/(n+2) - p
= (np + 1 - p(n+2))/(n+2)
= (n+1)/(n(n+2))
(b) To derive the MSE of p(hat)1, we use the definition of MSE:
MSE(p(hat)1) = Var(p(hat)1) + [Bias(p(hat)1)]^2
Given that p(hat)1 = Y/n, its variance is:
Var(p(hat)1) = Var(Y/n)
= (1/n^2) * Var(Y)
= (1/n^2) * np(1-p)
= p(1-p)/n
Substituting the bias derived earlier:
MSE(p(hat)1) = p(1-p)/n + [0]^2
= p(1-p)/n
To derive the MSE of p(hat)2, we follow the same process. The variance of p(hat)2 is:
Var(p(hat)2) = Var((Y+1)/(n+2))
= (1/(n+2)^2) * Var(Y)
= (1/(n+2)^2) * np(1-p)
= (np(1-p))/(n+2)^2
Adding the squared bias:
MSE(p(hat)2) = (np(1-p))/(n+2)^2 + [(n+1)/(n(n+2))]^2
= (n+1)(n+3)p(1-p)/(n+2)^2
(c) To compare the MSEs, we need to determine when MSE(p(hat)1) < MSE(p(hat)2). Comparing the expressions:
p(1-p)/n < (n+1)(n+3)p(1-p)/(n+2)^2
Simplifying:
(n+2)^2 < n(n+1)(n+3)
Expanding:
n^2 + 4n + 4 < n^3 + 4n^2 + 3n^2
To learn more about binomial distribution: -brainly.com/question/29137961
#SPJ11
What is the standard deviation of the number of customers who make a purchase during the first hour that the store is open
Answers
Answer:
busy people
Step-by-step explanation:
before shop ending
The deer population of a certain area is modeled by the formula: P=578e^0.045t where t is measured in years. In approximately how many years will the deer population reach 3000? 37.4 years 34.7 years 35.8 years 36.6 years
Answers
Answer:
36.6 years
Step-by-step explanation:
Here, we are to find the number of years it will take for the population of deers to reach 3,000
What to do here is simply substitute the value of 3,000 for P and use it to find t
3000 = 578e^0.045t
Divide through by 578
5.1903 = e^0.045t
Take the ln (natural logarithm of both sides)
Recall ln e = 1
ln 5.1903 = ln e^0.045t
ln 5.1903 = 0.045t
0.045t = 1.6468
t = 1.6468/0.045
t = 36.595 years which is 36.6 years
What's the value of x^2 - 3x + 1 when x= -2 and y = 4
Answers
(-2)^2 - 3(-2) + 1 = 4 + 6 + 1 = 11
However, there is no value of y in the expression, so it cannot be evaluated with y = 4.
=-2x-2=4
=4-(-6)+1
=4+6+1
=11
This is as per my understanding, I am Schooling Student
find and simplify the integral of 1 x from ac to bc, where 0 < a < b and c > 0.
Answers
To find and simplify the integral of 1/x from ac to bc, where 0 < a < b and c > 0, we can split the integral into two parts and then simplify the result.
∫[ac, bc] (1/x) dx = ∫[ac, bc] (1/x) dx
Using the properties of definite integrals, we can rewrite the integral as:
∫[ac, bc] (1/x) dx = ∫[ac, bc] (1/x) dx
Next, we can pull out the constant factors from the integral:
∫[ac, bc] (1/x) dx = ∫[ac, bc] (1/x) dx
Now, let's simplify the limits of integration:
When x = ac, we substitute it into the expression 1/x:
1/(ac) = 1/ac
When x = bc, we substitute it into the expression 1/x:
1/(bc) = 1/bc
Therefore, the integral becomes:
∫[ac, bc] (1/x) dx = ∫[ac, bc] (1/x) dx
Simplifying further:
∫[ac, bc] (1/x) dx = ln|x| evaluated from ac to bc
∫[ac, bc] (1/x) dx = ln|bc| - ln|ac|
Using the properties of logarithms, we can combine the two logarithms:
∫[ac, bc] (1/x) dx = ln(bc/ac)
Therefore, the integral of 1/x from ac to bc simplifies to ln(bc/ac).
To learn more about integration refer below:
https://brainly.com/question/24308099
#SPJ11
There were some people on a train.
18 people get off the train at the first stop and 21 people get on the train.
Now there are 65 people on the train.
How many people were on the train to begin with?
Answers
Answer:
There were 62 people on the train to begin with.
Step-by-step explanation:
Firstly,i I subtracted 21 with 18 so i got 3.
It means that the train got 3 more people from the start.
Then i subtracted 65 with 3.
And so i got 62.
Stacey worked on her garden for 4 3/4 hours.

Answers
Answer:
Number one and number 3 i think
Step-by-step explanation:
x + 4/3 = 55 + x -44
what is x
Answers
Answer: no solution
Step-by-step explanation:
There is no value for x that makes the equation true
Answer:
the answer is -0.8
We just started learning about this and I have no idea what this means..

Answers
HELP ASAP! Will name brainliest!

Answers
Answer:
The answer to your question is given in the attached photo.
Step-by-step explanation:
To determine the answer to the question,
First, we shall determine the value 3A.
This is obtained by multiplying matrix A by 3 as shown in the attached photo.
Next, we shall carry out the operation 3A – B as shown in the attached photo.
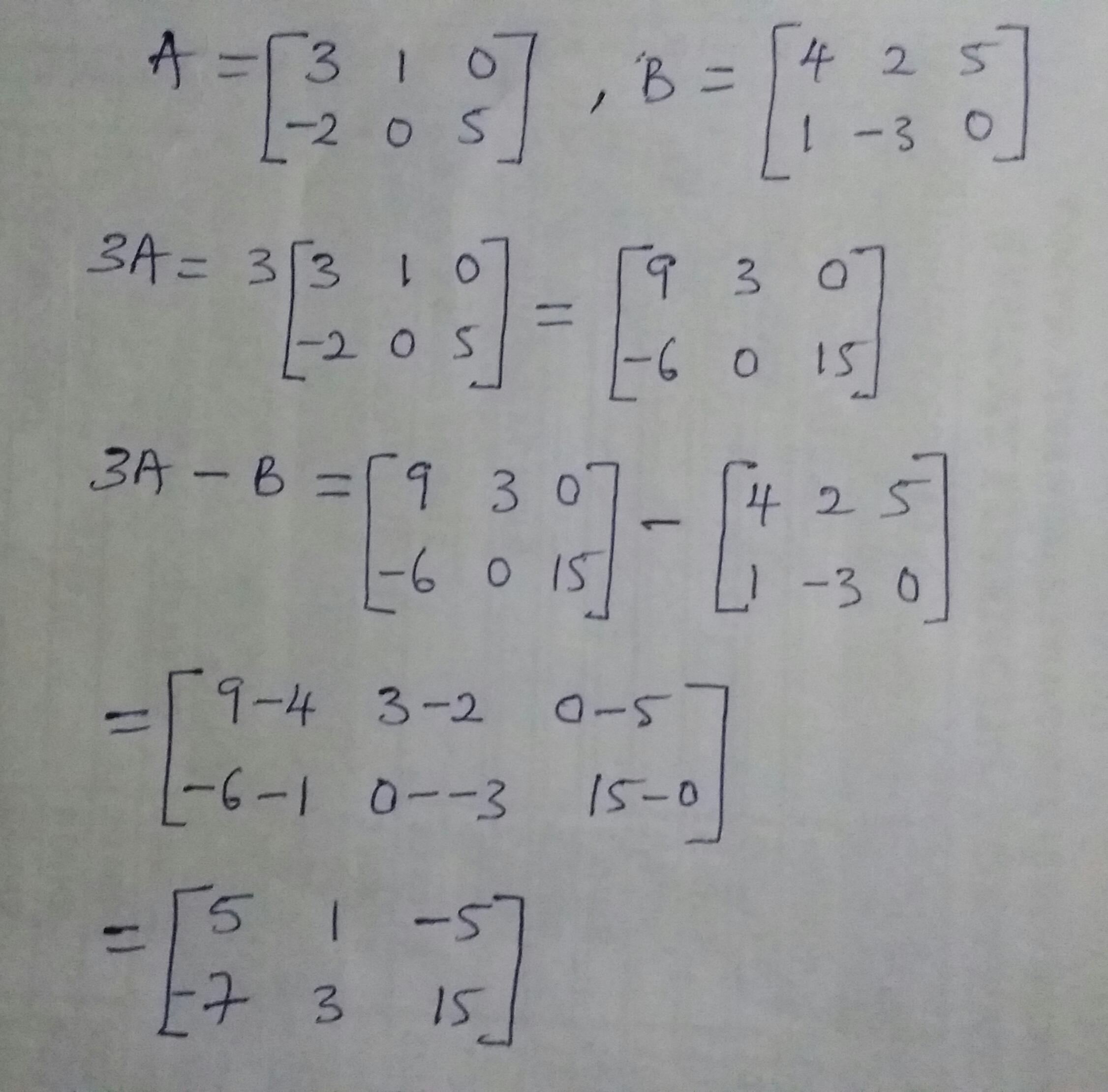
What should be added to 4c (– a + b + c) to obtain
3a (a + b + c) – 2b(a – b + c)?
Answers
Step-by-step explanation:
here hope it is helpful
the writing is not mine so yeah

Assume that women's weights are normally distributed with a mean given by μ=143 lb and a standard deviation given by σ =29 lb.
b) If 4 women are randomly selected, find the probability that they have a mean weight above 176
c) If 57 women are randomly selected, find the probability that they have a mean weight above 176
Answers
Answer:
b) P(mean weight > 176) = 1 - P(mean weight ≤ 176) = 1 - 0.9332 = 0.0668
c) P(mean weight > 176) = 1 - P(mean weight ≤ 176) = 1 - 0.9997 = 0.0003
What is the binary number for 21
Answers
Answer:
I believe this is 10101
I completely forgot how to solve this kind of problem, if anyone could solve it and give an explaination, that would be great

Answers
The measure of the angle Q is 50 degrees. Thus option A is correct option.
What is circle theorem?Circle theorems are properties that show relationships between angles within the geometry of a circle.
Given that the PQ is the tangent of the circle P. Thus it is in right angle and angle P is equal to the 90⁰.
We know that the sum of all the angles in a triangle is equal to the 180 degrees. therefore using the sum rule of the triangle, we get,
<PQR + <QPR + <PRQ = 180 degres
Put the value of the angle Q and angle P in the above equation we get,
Also,
<PQR + <QPR + <PRQ + arc 160 degrees =360⁰
Rewrite and solve the equation for angle R
90 +60+160 + <PQR = 360
This implies that <PQR = 360 - 310 = 50⁰
Hence, the measure of the angle Q is 50 degrees. Thus option A is correct option.
Study about circle theorem on https://brainly.com/question/19906313
#SPJ1
amy, beth, and jo listen to four different songs and discuss which ones they like. no song is liked by all three. furthermore, for each of the three pairs of the girls, there is at least one song liked by those two girls but disliked by the third. in how many different ways is this possible?(2012 amc 12b
Answers
If there is at-least one-song liked by those two girls but disliked by the third, then the "different-ways" this is possible is 132 ways.
There are ⁴C₃ ways to choose the "3-songs" which are liked by "3-pairs" of girls.
There are 3! ways to find the three songs are liked by which pair of girls.
In total, there are ⁴C₃×3! possibilities for the first "3-songs".
There are "3-cases" for fourth song, let that song be "D".
Case 1: Song "D" is disliked by all three girls means there is only one possibility.
Case 2: Song "D" is liked by exactly one girl means there are three possibility.
Case 3: Song "D" is liked by exactly two girls means there are three pairs of girls to choose from.
However, there is an overlap when "other-song" is liked by "same-pair" of girl is counted as "fourth-song" at some point, in which "case-D" will be counted as one of first "three-songs" liked by same girls.
Counting the overlaps, there are "three-ways" to choose pair with overlaps and 4×3=12 ways to choose what the other "two-pairs" like independently.
In total, there are 3×12=36 over-lapped possibilities,
Therefore, there are ⁴C₃ × 3! ×(3+1+3)-36 = 132 ways for the songs to be likely by the girls.
Learn more about Different Ways here
https://brainly.com/question/13773260
#SPJ4
what is the primed factored root for 12
Answers
Answer:
122 = 144
or
√12 = 3.464
3. What are the chances that he as he removes marbles from the bag, he doesn't get an orange marble until his third attempt?
Answers
Answer:
your question is not clear
Step-by-step explanation:
50 points! PLEASE HELP ME WITH THIS! Show how you got the answers!!! WIll mark as brainliest!!!DONT ANSWER IF YOU DONT KNOW IT


Answers
Answer:
A, C
Step-by-step explanation:
hypotenuse (longest) cannot be more than the adjacent (shortest) + the opposite (2nd shortest)
___________________________________________________________
Answer:
1 < BC < 3
Step-by-step explanation:
like I said, the hypotenuse cannot be more than the adjacent plus the opposite, so the longest BC can go is 3 because 4 goes over 12 (9+4 is over 12)
JoAnn paid $3.80 for each book at a book sale.
How much did she spend if she bought 9 books?
Answers
Answer:
$34.20
Step-by-step explanation:
total spent =3.80*9=$34.20
i need help with this please

Answers
Answer:
n= -1.2
Step-by-step explanation:
I think because if you use slope equation to find the slope which is 3/5 and then you use point slop equation to find out what the equation would be. y=3/5x and then you substitue y for 18 and solve/simplify the equation to get -1.2.
WORTH 25 POINTS!!! EASY POINTS! Plot C(-4, -5) ,D(-4,3) ,E( -3,2), and F(-8,2) in a coordinate plane. Are CD and EF congruent?

Answers
Answer: not congruent
Step-by-step explanation:
To know if CD and EF are congruent, you need to use the distance formula to find CD and EF. If the distance is the same, then they are congruent.
Distance formula: \(d=\sqrt{(x_{2}-x_1)^2+(y_2-y_1)^2}\)
CD
\(d=\sqrt{(-4-(-4))^2+(3-(-5))^2}\) [parenthesis]
\(d=\sqrt{(0)^2+(8)^2}\) [exponent]
\(d=\sqrt{0+64}\) [add]
\(d=\sqrt{64}\) [square root]
\(d=8\)
------------------------------------------------------------------------------------------------------------
EF
\(d=\sqrt{(-8-(-3))^2+(2-2)^2}\) [parenthesis]
\(d=\sqrt{(-5)^2+(0)^2}\) [exponent]
\(d=\sqrt{25+0}\) [add]
\(d=\sqrt{25}\) [square root]
\(d=5\)
------------------------------------------------------------------------------------------------------------
After finding the distance of CD and EF, we can say that they are not congruent. The line CD is 8 units long and line EF is 5 units long. Therefore, they are not congruent.
Which system of equations is represented by this matrix?
[2 -1 | -4]
[3 -2 | -6]
Answers
\( \sf{\blue{«} \: \pink{ \large{ \underline{A\orange{N} \red{S} \green{W} \purple{E} \pink{{R}}}}}}\)
The given system of equations is:
\(\displaystyle\sf \begin{align}2x - y &= -4\\3x - 2y &= -6\end{align}\)
To solve this system, we can use the method of elimination or substitution. Let's use the method of elimination:
First, we'll multiply the first equation by 2 to make the coefficients of \(\displaystyle\sf y\) in both equations equal:
\(\displaystyle\sf \begin{align}4x - 2y &= -8\\3x - 2y &= -6\end{align}\)
Now, we can subtract the second equation from the first equation to eliminate the variable \(\displaystyle\sf y\):
\(\displaystyle\sf (4x - 2y) - (3x - 2y) = -8 - (-6)\)
Simplifying the expression:
\(\displaystyle\sf x = -2\)
Now that we have found the value of \(\displaystyle\sf x\), we can substitute it back into either of the original equations to solve for \(\displaystyle\sf y\). Let's substitute it into the first equation:
\(\displaystyle\sf 2(-2) - y = -4\)
Simplifying the expression:
\(\displaystyle\sf -4 - y = -4\)
Solving for \(\displaystyle\sf y\):
\(\displaystyle\sf -y = 0\)
\(\displaystyle\sf y = 0\)
Hence, the solution to the system of equations is \(\displaystyle\sf x = -2\) and \(\displaystyle\sf y = 0\).
\(\huge{\mathfrak{\colorbox{black}{\textcolor{lime}{I\:hope\:this\:helps\:!\:\:}}}}\)
♥️ \(\large{\underline{\textcolor{red}{\mathcal{SUMIT\:\:ROY\:\:(:\:\:}}}}\)
You are at the grocery store choosing between bananas and walnuts. You know that the price of walnuts is $2 per pound and the price of bananas is $1 per pound, and your satisfaction from consuming is given by the following utility function: U=W .5
B .5
a. What is your marginal rate of substitution of walnuts for bananas? b. Using the Lagrangian approach, find your optimal consumption bundle. What is your total utility at this level? c. If the price of bananas doubles to $2 per pound, how much income must you have to maintain the same level of utility?
Answers
a. MRS = √(W/B)
b. Optimal bundle and total utility.
c. Adjusted income for constant utility.
a. The marginal rate of substitution (MRS) of walnuts for bananas is the rate at which you are willing to give up walnuts in exchange for one additional pound of bananas while keeping the utility constant. In this case, the MRS is equal to the ratio of the marginal utility of walnuts to the marginal utility of bananas. Since the utility function is U = √(W * B), the MRS can be calculated as MRS = √(W/B).
b. Using the Lagrangian approach, we can set up the following optimization problem: maximize U = √(W * B) subject to the constraint 2W + B = I, where W represents the pounds of walnuts, B represents the pounds of bananas, and I represents income. By solving the Lagrangian equation and the constraint, we can find the optimal consumption bundle and income level.
c. If the price of bananas doubles to $2 per pound, we need to determine the income required to maintain the same level of utility. With the new price, the constraint becomes 2W + 2B = I. By solving the Lagrangian equation again and substituting the new constraint, we can find the income level required to maintain the same level of utility.
Learn more about Function click here :brainly.com/question/572693
#SPJ11
Can someone find the surface area? I will award Brainlyest. Please equations as well

Answers
Radius would be 3.3125
