The 2 lines that make an X have a A above the middle and between the 2 lines on the right side says (4x-5) Degrees and on the left side between the lines says (x+16) degrees. Use the properties of vertical angles to find the value of x.
Responses
A 55
B 33
C 77
D 99
Answers
Answer:
C?
Step-by-step explanation:
If I understand your description correctly...
Vertical Angles are angles on opposite sides of the X formed by intersecting lines, and are Congruent (equal in measure).
So, 4x-5 = x+16
4x-x-5+5 = x-x+16+5
3x = 21
3x/3 = 21/3
x = 7
Related Questions
which mixed number is equivalent to the improper fraction of 41/10
Answers
Answer:
4 1/10 when u have a inproper fraction like that every 10 is one whole number
Between x = 0 and x = 1, which function has a greater average rate of change than y = 3x
?
Answers
Answer:
x=1
Step-by-step explanation:
y=3x so just add 1+3=4 then add veriable y=4x
Edouard Manet's Luncheon on the Grass was shown in the ___ after it was rejected for the annual salon.
A. World's Fair
B. Impressionist exhibition
C. salon d'automne
D. Osalon des refuses
Answers
Edouard Manet's Luncheon on the Grass was shown in the Salon des Refuses after it was rejected for the annual salon.
Option D is the correct answer.
We have,
Edouard Manet's painting "Luncheon on the Grass" was first rejected by the Paris Salon in 1863, as it was considered scandalous due to the nudity of the female figure in the painting.
In response to this rejection,
Emperor Napoleon III ordered an exhibition to be held for all the rejected paintings, which was known as the Salon des Refusés (Salon of the Refused).
Thus,
Edouard Manet's Luncheon on the Grass was shown in the Salon des Refuses after it was rejected for the annual salon.
Learn more about Edouard Manet's painting here:
https://brainly.com/question/26188885
#SPJ1
/ Solve . Round the answers to the nearest hundredth.
Answers
Answer:
226000
Step-by-step explanation:
225992 is closer to 226000 than 225900
The response to a question has three alternatives: A, B, and C. A sample of 120 responses provides 72 A, 36 B, and 12 C. Show the frequency and relative frequency distributions.
Class Frequency Relative
Frequency
A B C Total
Answers
The total frequency of the given data which is calculated to be is 120 1.
The frequency of an event or item in a set of data alludes to how frequently it happens.
Each response category's frequency is determined by how frequently it appears in the dataset. The percentage of all answers that fall into each category that represents the relative frequency of each response category.
(Class) (Frequency) (Relative Frequency)
(a) (60) 60/120 = (1/2)
(b) (12) 12/120 = (1/10)
(c) (48) 48/120 = (2/5)
Total 120 1
Therefore, the frequency and relative frequency distributions of the responses are:
(Class) (Frequency) (Relative Frequency)
a 60 1/2
b 12 1/10
c 48 2/5
Total 120 1
To know more about frequency:
https://brainly.com/question/5102661
#SPJ4
9 1/4 pints is equal to how many cups?
Answers
Answer:
18.5 cups
Step-by-step explanation:
There are 2 cups in 1 pint, so 9 1/4 pints is equal to 18.5 cups.
Hope this helps and if you have any more questions just ask in the comments
The data set of the diameters of the metal cylinders manufactured an automatic machine has sample size of n = 25, mean of x = 49.98 mm and std. of S = 0.14 mm. Expected diameter of metal cylinder is mu = 50.00 mm. Can we be 95% confident that machine calibrated properly? {Solution Tips: Yes; if 95% confidence interval includes expected diameter of metal cylinder.}
Answers
95% confidence that machine calibrated properly is (48.536, 51.424).
We have given that,
n = 25
mean of x = 49.98 mm
S = 0.14 mm
μ = 50.00 mm
and CI = 95%
from t-table we know that,
t at 95% and n - 1 = 25 - 1 = 24 is 2.064
Therefore 95% of CI means that
(x - 2.064 × S√n, x + 2.064 × S√n)
(49.98 - 2.064 × 0.14√25, 49.98 + 2.064 × 0.14√25)
(49.98 - 2.064 × 0.7, 49.98 + 2.064 × 0.7)
(49.98 - 1.444 , 49.98 + 1.444)
(48.536, 51.424)
Therefore 95% confidence that machine calibrated properly is
(48.536, 51.424).
The 95% confidence interval is a range of values that you can be 95% confident contains the true mean of the population.
To know more about confidence interval here
https://brainly.com/question/13073612
#SPJ4
g A car insurance company has determined that 8% of all drivers were involved in a car accident last year. If 15 drivers are randomly selected, what is the probability of getting 3 or more who were involved in a car accident last year
Answers
Answer:
\(P(x \geq 3)=1- [P(X=0)+P(X=1) +P(X=2)]\)
And we can find the individual probabilities like this:
\(P(X=0)=(15C0)(0.08)^0 (1-0.08)^{15-0}=0.286\)
\(P(X=1)=(15C1)(0.08)^1 (1-0.08)^{15-1}=0.373\)
\(P(X=2)=(15C2)(0.08)^1 (1-0.08)^{15-2}=0.227\)
\(P(x \geq 3)=1- [0.286+0.373+0.227]=0.114\)
Step-by-step explanation:
Let X the random variable of interest "number of cars involved in an accident", on this case we now that:
\(X \sim Binom(n=15, p=0.08)\)
The probability mass function for the Binomial distribution is given as:
\(P(X)=(nCx)(p)^x (1-p)^{n-x}\)
Where (nCx) means combinatory and it's given by this formula:
\(nCx=\frac{n!}{(n-x)! x!}\)
And we want to find this probability:
\(P(x \geq 3)=1- [P(X=0)+P(X=1) +P(X=2)]\)
And we can find the individual probabilities like this:
\(P(X=0)=(15C0)(0.08)^0 (1-0.08)^{15-0}=0.286\)
\(P(X=1)=(15C1)(0.08)^1 (1-0.08)^{15-1}=0.373\)
\(P(X=2)=(15C2)(0.08)^1 (1-0.08)^{15-2}=0.227\)
\(P(x \geq 3)=1- [0.286+0.373+0.227]=0.114\)
tiffany makes money by commission rates. She gets 21% of everything she sells. If tiffany sold $27000 worth of items this month, what is her salary for the month .
Answers
Answer:
\(Salary = \$5670\)
Step-by-step explanation:
Given
\(Items = \$27000\)
\(Rate = 21\%\)
Required
Determine her salary
Her salary is calculated as:
\(Salary = Rate * Items\)
This gives:
\(Salary = 21\% * \$27000\)
Convert % to decimal
\(Salary = 0.21 * \$27000\)
\(Salary = \$5670\)
Rebecca and Blake decide to roll a pair of fair 6-sided dice to determine who has to clean their dishes.
If the sum is 5 or 6, then Rebecca will clean the dishes.
If the sum is 8 or 9, then Blake will clean the dishes.
If the sum is anything else, they'll roll again.
Is this a fair way to decide who cleans the dishes? Why or why not?
Answers
Answer:
it's not fair
Step-by-step explanation:
... if Rebecca rolled the dice it's most likely going to give a 6 or a higher number if Blake rolled the same too would have happened thus it would be fair if the dice was 3 sided
Rebecca and Blake decide to roll a pair of fair 6-sided dice to determine who has to clean their dishes. If the sum is 5 or 6, then Rebecca will clean the dishes. If the sum is 8 or 9, then Blake will clean the dishes. If the sum is anything else, they'll roll again. This is a fair method of decision making by law of probability because both Rebecca and Blake are equally likely to wash the dishes.
What is probability?Probability is the branch of mathematics concerning numerical descriptions of how likely an event is to occur, or how likely it is that a proposition is true. The probability of an event is a number between 0 and 1, where, roughly speaking, 0 indicates impossibility of the event and 1 indicates certainty.
Total number of observations in sample space when a pair of dice is rolled = 6² = 36
Number of observations where sum is 5 or 6 = 9
((1,4)(4,1)(2,3)(3,2)(1,5)(5,1)(4,2)(2,4)(3,3))
Probability that Rebecca will clean the dishes = 9/36 = 0.25
Number of observations where sum is 8 or 9 = 9
((2,6)(6,2)(3,5)(5,3)(4,4)(3,6)(6,3)(4,5)(5,4))
Probability that Blake will clean the dishes = 9/36 = 0.25
Since, the probabilities of Rebecca and Blake cleaning the dishes are equal, the method of decision making is fair.
Learn more about probability here
https://brainly.com/question/11234923
#SPJ2
Find the equation of the line with slope m=−12 that contains the point (2,−4).
Answers
Answer:
y=-12x+20
Step-by-step explanation:
-4=-12(2) +b
-4=24+b
(add 24 both sides)
20=b
slope: -12
y intercept: (0,20)
Answer:
y + 4 = -12(x - 2)
Step-by-step explanation:
Use the point-slope formula for the equation of a straight line:
y - k = m(x - h)
Here m = -12, h = 2 and k = -4, and so we have:
y + 4 = -12(x - 2)
Determine if the given point satisfies either equations and is a solution of the system of inequalities
4y-23x _5x-11
The rest see it on the photo
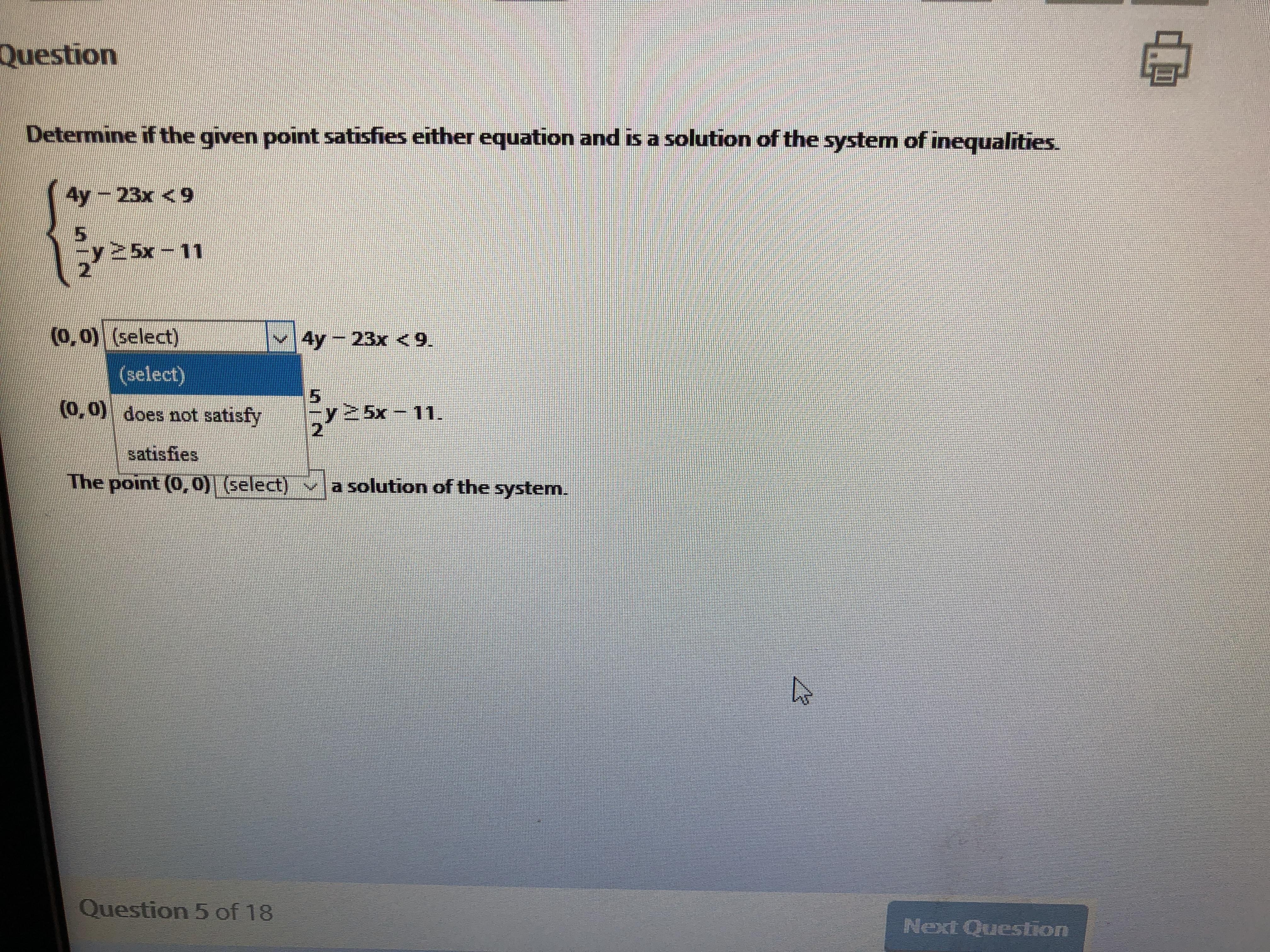
Answers
Answer:
(0,0) satisfies 4y - 23x < 9
(0,0) satisfies 5/2y > 5x -11
The point (0,0) is a solution of the system.
Step-by-step explanation:
The sum of two numbers is 12. The difference of the two numbers is 6. What are the two numbers?
Answers
Answer:
3 and 9
Step-by-step explanation:
x+y=12
y-x=6
y-x=6
+x. +x
y=6+x
x+(6+x)=12
6+2x=12
-6. -6
2x=6
/2. /2
x=3
3+y=12
-3. -3
y=9
Hopes this helps please mark brainliest
If cody makes 10$ an hour at his after job how many hours will it tske him to earn$150
Answers
Answer:
15 hours
Step-by-step explanation:
150/10=15
Answer: 15 hours
Step-by-step explanation:
I really need help with this. Thanks so much in advance!
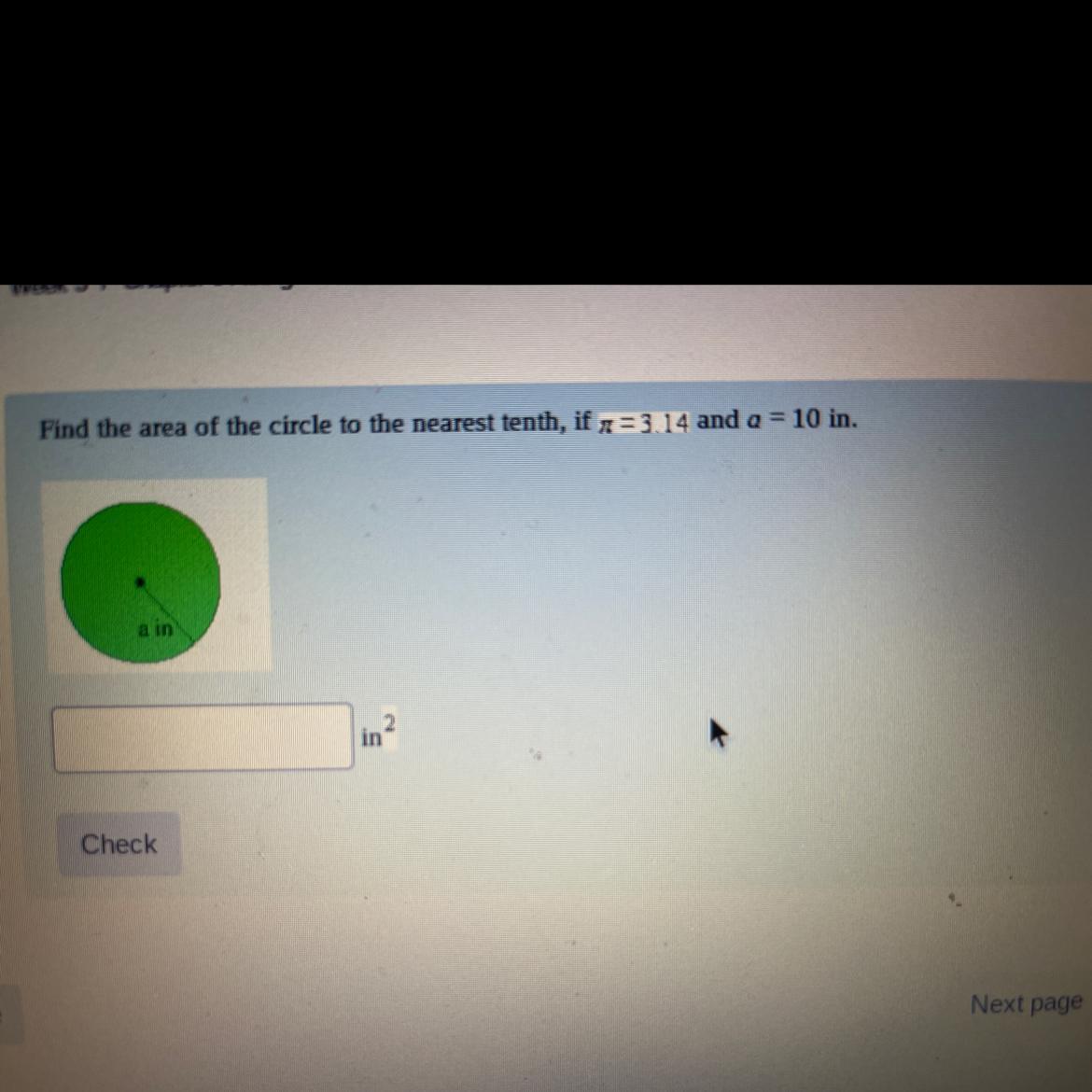
Answers
Given:
\(a=10\text{ in.}\)\(\begin{gathered} \text{Area of a circle=}\pi(a)^2 \\ =3.14(10)^2 \\ =3.14(100) \\ =314in^2 \end{gathered}\)Can someone please help me with this. Trying to receive extra credit. Show work, or no credit for work.

Answers
After 62.63 years Mr. Reihman's account outgrown Mr. Van Ausdall,
What is compound interest?The interest earned on savings that are computed using both the original principal and the interest accrued over time is known as compound interest. A = P(1 + r/n)^nt, where P is the principal balance, r is the interest rate, n is the number of times interest is compounded annually, and t is the number of years, which is the formula for compound interest.
Given, Van Ausdall and Reihman both make the decision to start retirement funds.
Mr. Reihman contributes $10,000 at a constant rate of 7%, and
Mr. Van Ausdall contributes $35,000 at a continual rate of 5%.
Let "x" be the time when Mr. Reihman and Mr. Van Ausdall will have the same amount of money
From the general formula of continuous growth of the amount:
Amount = Principal amount*e^(rt)
Where r =rate of interest
t = time
At time x Mr. Reihman will have = 10,000*e^(7%*x)
At time x Mr. van will have =35,000* e^(5%*x)
Since at x, they both will have the same amount
thus,
10,000*e^(7%*x) =3 5,000* e^(5%*x)
e^(7x/100 -5x/100) = 3.5
Taking natural logs on both the side
2x/100 = ln3.5
x = 50*ln 3.5
x = 62.63
Thus, after 62.63 years Mr. Reihman's account outgrown Mr. Van Ausdall,
Learn more about compound interest here:
https://brainly.com/question/29335425
#SPJ9
Complete question:
Reihman and Van Ausdall both decide to open retirement accounts. Mr. Van Ausdall puts in $35,000 with a continuous rate of 5% and Mr. Reihman puts in $10,000 with a continuous rate of 7%. After how much time will Mr. Reihman's account outgrow Mr. Van Ausdall? Solve algebraically and show all work.
Cuales don las dos terceras partes de tres medios
Answers
Answer:
una parte entenra
Step-by-step explanation:
\(\frac{3}{2}/\frac{2}{3}\\\\=\frac{3}{2}*\frac{3}{2}\\\\=1\)
if a linear space consists of polynomials with degrees less than 2, what is the dimension when the derivative is 0?
Answers
The dimension of V when the derivative is 0 is 1 since the l polynomial reduces to a 1-dimensional space.
The dimension of a linear space in which all polynomials with degrees less than 2 is 0 is 1. To estimate the dimension, we must take into account the basic elements of the space.
Let V be the collection of polynomials with a degree less than 2. Then the basic elements of V are 1, x
Let f(x)be a polynomial in V. Then f(x) can be written as f(x)= a0 + a1 x for some constants
We know that the derivative of f(x) is f'(x) =\(a_1$$\)
Therefore, when the derivative f'(x) is 0, it means that a1 = 0. In this case, the polynomial f(x) reduces to f(x) = a0, which is a constant.
So, the dimension of V when the derivative is 0 is 1, since the l polynomial reduces to a 1-dimensional space.
To learn more about, polynomials visit the link below:
brainly.com/question/11536910
#SPJ4
There are 3/4 kilograms of salt in the jar Ms.Hernandez used 1/3 of the salt when she was making lunch how much salt did she use
Answers
Answer:
sd
Step-by-step explanation:
Write an equation in point-slope form of the line that passes through the given points. (4, 0) and (−2, 1)
Answers
The Equation is y = -1/6x + 2/3 in point - slope form of line
Slope - Intercept Form:
A linear equation when graphed is a straight line that extends to infinity in both directions. One linear equation uses the slope, m, and the y - intercepts, b, in the form y = mx + b.
In the given question is that:
Write an equation in point-slope form of the line that passes through the given points. (4, 0) and (−2, 1)
Now, According to the question:
The slope - formula is:
\(m = \frac{y_{2}-y_{1} }{x_{2}-x_{1} }\)
Substitute the values :
\(x_{1} = 4\\\\x_{2}= -2 \\\\y_{1}= 0\\ \\y_{2} = 1\)
m = 1/ (-2 - 4)
m = -1/6
and, A line Equation is:
y = mx + b
Now, Substitute the values :
m = -1/6
x = 4
y = 0
0 = -1/6 x 4 + b
b = 2/3
Again, Substitute the values :
y = mx + b
y = -1/6x + 2/3
Hence, The Equation is y = -1/6x + 2/3 in point - slope form of line
Learn more about Slope - Intercept Form at:
https://brainly.com/question/9682526
#SPJ1
need help asap will mark brainliest!

Answers
Answer:
the answer is E
Step-by-step explanation:
A 95% confidence interval of 17.6 months to 49.2 months has been found for the mean duration of imprisonment, mu, of political prisoners of a certain country with chronic PTSD. a. Determine the margin of error, E. b. Explain the meaning of E in this context in terms of the accuracy of the estimate. c. Find the sample size required to have a margin of error of 11 months and a 99% confidence level. (Use sigmaequals45 months.) d. Find a 99% confidence interval for the mean duration of imprisonment, mu, if a sample of the size determined in part (c) has a mean of 36.5 months.
Answers
Answer:
a) \( E = \frac{49.2-17.6}{2}= 15.8\)
b) For this case we have 95% of confidence that the true mean would be between \(\pm 15.8\) units respect the true mean.
c) \(n=(\frac{2.58(45)}{11})^2 =111.39 \approx 112\)
So the answer for this case would be n=112
d) \(36.5-2.58\frac{45}{\sqrt{112}}=25.53\)
\(36.5+2.58\frac{45}{\sqrt{112}}=47.47\)
Step-by-step explanation:
Part a
For this case we know that the cinfidence interval for the true mean is given by:
\( \bar X \pm E\)
Where E represent the margin of error. For this case we have the confidence interval at 95% of confidence and we can estimate the margin of error like this:
\( E = \frac{49.2-17.6}{2}= 15.8\)
Part b
For this case we have 95% of confidence that the true mean would be between \(\pm 15.8\) units respect the true mean.
Part c
The margin of error is given by this formula:
\( ME=z_{\alpha/2}\frac{\sigma}{\sqrt{n}}\) (a)
And on this case we have that ME =11 and we are interested in order to find the value of n, if we solve n from equation (a) we got:
\(n=(\frac{z_{\alpha/2} \sigma}{ME})^2\) (b)
The critical value for 99% of confidence interval now can be founded using the normal distribution. The critical value would be \(z_{\alpha/2}=2.58\), replacing into formula (b) we got:
\(n=(\frac{2.58(45)}{11})^2 =111.39 \approx 112\)
So the answer for this case would be n=112
Part d
The confidence interval for the mean is given by the following formula:
\(\bar X \pm z_{\alpha/2}\frac{\sigma}{\sqrt{n}}\) (1)
Replcaing the info given we got:
\(36.5-2.58\frac{45}{\sqrt{112}}=25.53\)
\(36.5+2.58\frac{45}{\sqrt{112}}=47.47\)
y = 3/5x + 5
y = -3/5x + 5
y = 3/5x - 5
y = -3/5x - 5

Answers
Answer:
y= 3/5x+5
Step-by-step explanation:
The y intercept is 5 and the graph is going upwards so it is a postive slope. If you look at at the graph from the y intercept point to point B you can see it goes up by 3 and right by 5 so the slope is 3/5. This makes the equation y=3/5x+5
What are Yh coordinates of the image of Al for a dilation with center (0,0) and a scale factor 4.

Answers
Answer:
\(C\text{ : }(-8,20)\)Explanation:
Here, we want to get the coordinates of point L after dilation
Looking at the scale factor, we have it as 4
This means we have to multiply each of the coordinate values by 4
We have that as:
\(L^{\prime}^{\text{ }}\text{ = }(4\times(-2)\text{ , 4}\times5)\text{ = }(-8,20)\)find the values of x in the following, giving brief reasons for your answers.
please help me, thank you.

Answers
Answer:
For the first one x= 60 degrees
Second one x= 125 degrees
Step-by-step explanation:
a) To figure out this we can see that the total of angles in a 5- angled shape is 540 degrees.
So (2x X 4) + x = 540 degrees
= 9x= 540
= x = 60
b) To figure out this we can see that the total of angles in a 7- angled shape is 900 degrees.
So 60+2x+x+x+x+x+90= 900
= 150 +6x= 900
= 6x= 750
= x= 125
3/4 + 20 (2/5 x 4/7)
Answers
Answer:
i believe the answer is 5.3
The ratio of 36 apples to 18 children
Answers
It could be 1:1 or 2:1 if you dont give them more than 1 apple and save some
Step-by-step explanation:
Determine the turning points and distinguish between them when necessary y=x³ - 3x - 9x + 4
Answers
The turning points of the function y = x³ - 3x² - 9x + 4 are (3, -23) and (-1, 9).
To determine the turning points of the given function y = x³ - 3x² - 9x + 4, we need to find the critical points where the derivative of the function is equal to zero.
1. Find the derivative of the function:
y' = 3x² - 6x - 9
2. Set the derivative equal to zero and solve for x:
3x² - 6x - 9 = 0
3. Factorize the quadratic equation:
3(x² - 2x - 3) = 0
4. Solve the quadratic equation by factoring or using the quadratic formula:
(x - 3)(x + 1) = 0
This gives us two possible values for x: x = 3 and x = -1.
5. Substitute these critical points back into the original function to find the corresponding y-values:
For x = 3:
y = (3)³ - 3(3)² - 9(3) + 4
= 27 - 27 - 27 + 4
= -23
For x = -1:
y = (-1)³ - 3(-1)² - 9(-1) + 4
= -1 - 3 + 9 + 4
= 9
6. Therefore, the turning points are (3, -23) and (-1, 9).
Note: It appears that there was a typo in the original equation, where the term "-9x" should have been "-3x²". The above solution assumes the corrected equation.
For more such questions on points, click on:
https://brainly.com/question/26865
#SPJ8
Pls answer:
(-5)² - (-2)⁴
Answers
Answer:
24
Step-by-step explanation:
Answer:
-21
Step-by-step explanation:
-25+ 2²
evaluate -5²
evaluate -2²
subtract
The grade distribution for a course is listed in the following table. If a student is randomly selected fro mly selected from this class, what is the probability that they received an A, B, or C? A B C D F 40 40 33 20 14
Answers
Answer:
the probability that a randomly selected student received an A, B, or C is approximately 0.769, or about 76.9%.
Step-by-step explanation:
To find the probability that a randomly selected student received an A, B, or C, we need to add up the number of students who received each of these grades and divide by the total number of students in the class.
The total number of students in the class is:
total = A + B + C + D + E = 40 + 40 + 33 + 20 + 14 = 147
The number of students who received an A, B, or C is:
A + B + C = 40 + 40 + 33 = 113
Therefore, the probability that a randomly selected student received an A, B, or C is:
P(A or B or C) = (A + B + C) / total = 113 / 147 ≈ 0.769
So the probability that a randomly selected student received an A, B, or C is approximately 0.769, or about 76.9%.