The area of the bottom of a 250 mL beaker is found to be 38.5 cm2. What is this area in square millimeters
Answers
Answer:
3580mm²
Step-by-step explanation:
Given the area of 250mL beaker to be 38.5cm²
We need to convert the value to mm²
Using the conversion
1cm² = 100mm²
Using the equality postulate:
If 1cm² = 100mm²
35.8cm² = x mm²
Calculate x by cross multiplying
x mm² × 1 cm² = 35.8 cm² × 100mm²
x mm² = (35.8 cm² × 100mm²)/1cm²
x mm² = 3580mm²
Hence the area is square millimeters is 3580mm²
Related Questions
(2-I)(3+5i) multiple
Answers
Answer:
ii-7i
Step-by-step explanation:
These can be multiplied in a manner similar to expanding brackets:
(
2
+
i
)
(
3
−
5
i
)
=
6
+
3
i
−
10
i
−
5
i
2
Remember that
i
=
√
−
1
so
i
2
=
−
1
This will in turn allow us to gather the like terms like so:
6
+
5
+
3
i
−
10
i
=
11
−
7
i
WILL MARK BRAINIEST!!!!!!!!!!! PLEASE HELP ASAP
The figure below shows the location of 3 points around a lake. The length of the lake, BC, is also shown. A picture of a right triangle ABC with right angle at B is shown. The length of the side AC is labeled as 8 miles. The length BC of the triangle is labeled as 6 miles. This length BC is also the length of an irregular gray shaded shape. Which of the following options is closest to the distance (in miles) between points A and B?
A.) 2.24
B.) 2.65
C.) 3.74
D.) 5.29
Answers
Answer:
I think B but I'm not sure
Step-by-step explanation:
Answer:
B i think
Step-by-step explanation:
you're welcome <3
Sin and cos
Solve: 2 sin ϕ cos ϕ + 2 sin ϕ + cos ϕ + 1 = 0 for 0 < ϕ < 2π
Answers
Answer:
We can start by using the identity 2 sin ϕ cos ϕ = sin 2ϕ to simplify the left-hand side of the equation:
2 sin ϕ cos ϕ + 2 sin ϕ + cos ϕ + 1 = sin 2ϕ + 2 sin ϕ + cos ϕ + 1
Next, we can use another identity, sin^2 ϕ + cos^2 ϕ = 1, to eliminate the cosine term:
sin 2ϕ + 2 sin ϕ + cos ϕ + 1 = sin 2ϕ + 2 sin ϕ + √(1 - sin^2 ϕ) + 1
Now, we can substitute u = sin ϕ to obtain a quadratic equation in u:
sin 2ϕ + 2 sin ϕ + √(1 - sin^2 ϕ) + 1 = sin^2 ϕ + 2 sin ϕ + √(1 - sin^2 ϕ) + 1
sin 2ϕ = sin^2 ϕ
2 sin ϕ cos ϕ = sin^2 ϕ - cos^2 ϕ
2 u √(1 - u^2) = u^2 - (1 - u^2)
2 u √(1 - u^2) = 2 u^2 - 1
4 u^2 (1 - u^2) = (2 u^2 - 1)^2
4 u^4 - 4 u^2 + 1 = 0
This is a quadratic equation in u^2, which can be solved using the quadratic formula:
u^2 = [4 ± √(16 - 16)] / 8 = 1/2 or u^2 = 1
Since 0 < ϕ < 2π, we have 0 < u < 1, so u^2 = 1/2. Therefore, sin ϕ = u = ±√(1/2) and cos ϕ = ±√(1 - u^2) = ±√(1/2).
We can summarize the solutions as follows:
sin ϕ = √(1/2) or sin ϕ = -√(1/2)
cos ϕ = √(1/2) or cos ϕ = -√(1/2)
Therefore
I can’t figure out this other one, can I get some help?

Answers
place g(x) as the value x, into p(x)
to gain p(g(x)) = (1 / SqrRt(x) )² - 4
simplify = ( 1 / x ) - 4
find domain
x =/= 0 (undefined) & (1/4)
You model your investment account using the formula y = 20000(1.035)x where x represents the
number of years and y represents the account balance after x years. What is the growth rate of your
investment?
Answers
Answer:
Step-by-step explanation:
The growth rate of the investment is represented by the constant multiplier in the exponential term of the formula y = 20000(1.035)x. In this case, the constant multiplier is 1.035, which represents the annual growth rate of the investment account.
To calculate the growth rate as a percentage, we can subtract 1 from the constant multiplier, and then multiply the result by 100.
So, the growth rate of the investment is:
1.035 - 1 = 0.035
0.035 * 100 = 3.5%
Therefore, the growth rate of the investment is 3.5%.
The growth rate of the investment can be found by subtracting 1 from the base of the exponential function and converting the result to a percentage:
growth rate = (base - 1) x 100%
growth rate = (1.035 - 1) x 100%
growth rate = 0.035 x 100%
growth rate = 3.5%
Therefore, the growth rate of the investment is 3.5%.
what are the similarities and differences in linear and exponential in intercepts?
what are the similarities and differences in linear and exponential in domain and range?
what are the similarities and differences in linear and exponential in asymptotes?
what are the similarities and differences in linear and exponential in misc.?
Answers
Answer:
What is a linear function? A linear function is a function whose graph is a straight line. The rate of change of a linear function is constant. The function shown in the graph below, y = x + 2, is an example of a linear function.
Graph of linear function
Graph of linear function
A linear function has a constant rate of change. The rate of change is the slope of the linear function. In the linear function shown above, the rate of change is 1. For every increase of one in the independent variable, x, there is a corresponding increase of one in the dependent variable, y. This gives a slope of 1/1 = 1.
A linear function is typically given in the form y = mx + b, where m is equal to the slope, or constant rate of change.
Examples of linear functions include:
If a person drives at a constant speed, the relationship between the time spent driving (independent variable) and the distance traveled (dependent variable) will remain constant.
Assuming no change in price, the relationship between the number of pounds of bananas a person buys (independent variable) and the total cost of the bananas (dependent variable) will remain constant.
If a person earns an hourly wage at their job, the relationship between the time spent working (independent variable) and the amount earned (dependent variable) will remain constant.
Save Timeline Autoplay Speed Normal
Video Quiz Course
71K views
Exponential Functions
What is an exponential function? An exponential function is a function that involves exponents and whose graph is a smooth curve. The rate of change in an exponential function is not constant. The functions shown in the graph below, y = 0.5x and y = 2x, are examples of exponential functions.
Graphs of exponential functions
Graphs of exponential functions
An exponential function does not have a constant rate of change. The rate of change in an exponential function is the value of the independent variable, x. As the value of x increases or decreases, the rate of change increases or decreases as well. Rather than a constant change, as in the linear function, there is a percent change.
An exponential function is typically given in the form y = (1 + r)x, where r represents the percent change.
Examples of exponential functions include:
Step-by-step explanation:
The ratio of protein to fat in a health food bar is eight to three. which numerical value is equivalent to this ratio?
Answers
Answer:
8:3 or also 8/3
Step-by-step explanation:
If I understood it well it is a task to express a ratio.
8:3 or also 8/3
Answer:
8//3 and 8:3
Step-by-step explanation:
Consider this expression.
the value of the expression is ___?

Answers
Evaluating the expression in a = 2 and b = -4, we will get the value -3.
How to evaluate the expression?Here we have the following expression:
√(a³ - 7) - |b|
We want to evaluate it in the values:
a = 2
b = -4
That means that we need to replace these variables by these values, then we will get:
√(2³ + 7) - |-4|
Then we can solve the expression:
√(2³ + 7) - |-4|
= √(1) - 4
= 1 - 4
= -3
That is the value of the expression.
Learn more about evaluating expressions:
https://brainly.com/question/4541471
#SPJ1
Tom bought g gallons of ice cream for $3.55 per gallon. He also paid $12 for toppings. Which expressions represent the total amount Tom spent to prepare for his party?
Answers
Answer:
y=3.55x+12
Step-by-step explanation:
Convert from Standard form to Slope intercept form:
y/3 - 10/3 = x
Answers
*Hope this helped!!!
We will now perform cross-validation on a simulated data set. (a) Generate a simulated data set as follows: > set.seed (1) > x-rnorm (100) y-x-2x-2+rnorm (100) In this data set, what is n and what is p? Write out the model used to generate the data in equation form. (b) Create a scatterplot of X against Y. Comment on what you find. (c) Set a random seed, and then compute the LOOCV errors that result from fitting the following four models using least squares: Note you may find it helpful to use the data.frameO function to create a single data set containing both X and Y Are your results the same as what you got in (c)? Why? this whuat you expected? Explain your aniswer. (d) Repeat (c) using another random seed, and report your results. (e) Which of the models in (c) had the smallest LOOCV error? Is
Answers
a. n is 100 and p = 2 when we generate the simulated data set.
b. By generating a scatterplot we found a quadratic function. Y from -9 to 3 and x from -2 to 2.
c. Yes the result is the same as we got in question c.
d. Report of d is exactly the same because LOOCV will be the same since it evaluates n folds of a single observation.
e. The quadratic model and yes I expected that because the true data is of a quadratic form.
a. Generate a simulated data set.
set.seed(1)
Y <- rnorm(100)
X <- rnorm(100)
Y <- X - 2 × X² + rnorm(100)
n=100, p=2.
y=x−2x2+ϵ,ϵ∼N(0,1)
b. Create a scatterplot of X against Y . Comment on what you find.
ggplot(data.table(X=X, Y=Y), aes(x=X,y=Y)) + geom_point()
We can see a clear quadratic function. Y from -9 to 3 and x from -2 to 2.
c. Set a random seed, and then compute the LOOCV errors that result from fitting the following four models using least squares:
dt = data.table(X, Y)
# i
glm.fit1 <- glm(Y ~ X)
cv.glm(dt, glm.fit1)$delta
## [1] 5.890979 5.888812
# ii
glm.fit2 <- glm(Y ~ poly(X,2))
cv.glm(dt, glm.fit2)$delta
## [1] 1.086596 1.086326
# iii
glm.fit3 <- glm(Y ~ poly(X,3))
cv.glm(dt, glm.fit3)$delta
## [1] 1.102585 1.102227
# iv
glm.fit4 <- glm(Y ~ poly(X,4))
cv.glm(dt, glm.fit4)$delta
## [1] 1.114772 1.114334
d. Repeat (c) using another random seed, and report your results. Are your results the same as what you got in (c)? Why?
dt = data.table(X, Y)
set.seed(2)
# i
glm.fit1 <- glm(Y ~ X)
cv.glm(dt, glm.fit1)$delta
## [1] 5.890979 5.888812
# ii
glm.fit2 <- glm(Y ~ poly(X,2))
cv.glm(dt, glm.fit2)$delta
## [1] 1.086596 1.086326
# iii
glm.fit3 <- glm(Y ~ poly(X,3))
cv.glm(dt, glm.fit3)$delta
## [1] 1.102585 1.102227
# iv
glm.fit4 <- glm(Y ~ poly(X,4))
cv.glm(dt, glm.fit4)$delta
## [1] 1.114772 1.114334
Exact the same, because LOOCV will be the same since it evaluates n folds of a single observation.
e. The quadratic model and yes I expected that because the true data is of a quadratic form.
Learn more about cross-validation at
https://brainly.com/question/30508081
#SPJ4
answer the number 2 only

Answers
The missing variables on item 2 are given as follows:
\(o = 12\sqrt{3}\)i = 24.What are the trigonometric ratios?The three trigonometric ratios are the sine, the cosine and the tangent of an angle, and they are obtained according to the formulas presented as follows:
Sine = length of opposite side to the angle/length of hypotenuse of the triangle.Cosine = length of adjacent side to the angle/length of hypotenuse of the triangle.Tangent = length of opposite side to the angle/length of adjacent side to the angle = sine/cosine.For the angle of 60º, we have that:
o is the opposite side.12 is the adjacent side.Hence the length o is given as follows:
tan(60º) = o/12.
\(\sqrt{3} = \frac{o}{12}\)
\(o = 12\sqrt{3}\)
Applying the Pythagorean Theorem, the length i is given as follows:
i² = 12² + \((12\sqrt{3})^2\)
i² = 576
i² = 24²
i = 24.
A similar problem, also about trigonometric ratios, is given at brainly.com/question/24349828
#SPJ1
Answer:
o = 12√3
i = 24
Step-by-step explanation:
From observation of the given right triangle, we can see that two of its interior angles measure 60° and 90°. As the interior angles of a triangle sum to 180°, this means that the remaining interior angle must be 30°, since 30° + 60° + 90° = 180°. Therefore, the triangle is a special 30-60-90 triangle.
The side lengths in a 30-60-90 triangle have a special relationship, which can be represented by the ratio formula a : a√3 : 2a, where "a" represents a scaling factor that can be any positive real number.
Side a is opposite the 30° angle (shortest leg).Side a√3 is opposite the 60° angle (longest leg).Side 2a is the hypotenuse (longest side).In triangle #2, the shortest leg is 12 units.
As "a" is the shortest leg, the scale factor "a" is 12.
The side labelled "o" is the longest leg opposite the 60° angle. Therefore:
\(o = a\sqrt{3}=12\sqrt{3}\)
The side labelled "i" is the hypotenuse of the triangle. Therefore:
\(i= 2a = 2 \cdot 12=24\)
Therefore:
o = 12√3i = 247+(24/6)+(3²/3)+10 need help solveing it out
Answers
Answer: 24
Step-by-step explanation:
7+(24/6)+(3²/3)+10
To divide powers of the same base, subtract the denominator's exponent from the numerator's exponent. Subtract 1 from 2 to get 1.
7+24/6+3^1+10 Also when I say 3^1 I mean 3 and the exponent is 1
Divide 24 by 6 to get 4.
7+4+3^1+10
Add 7 and 4 to get 11.
11+3^1+10
Calculate 3 to the power of 1 and get 3.
11+3+10
Add 11 and 3 to get 14.
14+10
Add 14 and 10 to get 24.
A car is traveling at a speed of 60 miles per hour. How far will the car travel
in 3 hours? (multiply x)
Answers
The car will cover a distance of 180 miles in three hours.
We know that,
s = d/ t
or d = s* t
Where, s = speed
d = distance
t = time
According to the question,
speed of the car (s) = 60 miles/ hour
Time taken (t) = 3 hours
Distance travelled (d) = s * t
= 60miles/ hour * 3 hours
= 180 miles
Hence, the distance traveled by car in 3 hours is 180 miles.
Learn about Speed and Distance:
brainly.com/question/12759408
make e the subject
e-5=2f
Answers
Answer:
e-5=2f
take '-5' to the other side where '2f' is
e=2f+5
helppppppppppppppppppppppppp me

Answers
Answer:
600
Step-by-step explanation:
\(R(1)=400 \\ \\ R(2)=1000 \\ \\ R(2)-R(1)=600\)
Books: Josephine has seven chemistry books, three history books, and six statistics books. She wants to choose one book of each type to study. In how many
ways can she choose the three books?
The total number of ways Josephine can choose the three books is
Answers
Answer:
Step-by-step explanation:
we have totally 7+3+6=16 books.
let us choose one book from the all these books;
by using permutation formula we will get the answer
\(P(n,r)=\frac{(n)!}{(n-r)!} \\\)
\(P(7,1)=\frac{(7)!}{(7-1)!} = 7\\\\\ P(3,1)=\frac{(3)!}{(3-1)!} = 3\\\\ P(6,1)=\frac{(6)!}{(6-1)!} = 6\\\\\)
all these process are related to each other so we should multiply them
7*3*6=126
Maria has 8 more scarves than Lucy Lucy has 8 scarves. How many scarves does Maria have, write an equation?
Answers
The equation M = 8 + 8 represents the given information. Maria has 16 scarves.
What is the equation?The equation is defined as mathematical statements that have a minimum of two terms containing variables or numbers that are equal.
Let's let "M" represent the number of scarves that Maria has.
According to the problem, "Maria has 8 more scarves than Lucy." We know that Lucy has 8 scarves. So, Maria has 8 more than that.
We can represent this information in an equation:
M = 8 + 8
We add 8 to Lucy's 8 scarves to get the total number of scarves that Maria has, which is 16.
So, Maria has 16 scarves.
Learn more about the equation here:
brainly.com/question/13947055
#SPJ1
The required Maria has 16 scarves, and the expression models the situation is given as M = 8 + L.
What are equation models?The equation model is defined as the model of the given situation in the form of an equation using variables and constants.
Here,
Let's use the variable "M" to represent the number of scarves that Maria has. Since Maria has 8 more scarves than Lucy, and Lucy has 8 scarves, we can write an equation as,
M = 8 + L
where L is the number of scarves that Lucy has. Substituting L = 8 into this equation, we get:
M = 8 + 8
M = 16
Therefore, Maria has 16 scarves.
Learn more about models here:
https://brainly.com/question/22591166
#SPJ1
Simplify 3x^2x 4x^5 please help
Answers
Answer:
\(12x^7\)
Step-by-step explanation:
\(3x^2 \times 4x^5\)
\(3 \times x^2 \times 4 \times x^5\)
\(3 \times 4 \times x^{2+5}\)
\(12 \times x^7\)
If you wish to have $60,000 in 8 years, how much do you need to deposit in the bank today if the account pays 9% using present value and future value.
Answers
Answer:
Amount to deposit= $26799.0
Step-by-step explanation:
Amount A = $60,000
Years t = 8 years,
Rate R = 9% .
A= p(1+r/n)^(nt)
60000= p(1+0.09/9)^(9*9)
60000= p(1+0.01)^(81)
60000= p(1.01)^81
60000= p(2.23888)
60000/2.23888= p
26799.1138= p
P= $26799.0
Amount to deposit= $26799.0
To be able to have $60,000 after 8 years at a rate of 9% per annum, the amount to deposit today is $30,111.98
You can solve this using the Future Value formula which is:
Future value = Present value x ( 1 + rate)^ number of years
You have everything except the present value so solve for it:
60,000 = PV x ( 1 + 9%)⁸
60,000 = PV x 1.9925626416901921
PV = 60,000 / 1.9925626416901921
PV = $30,111.98
In conclusion, you should deposit $30,111.98 today.
Find out more about this at https://brainly.com/question/14715827.
find the balance if you deposit $2500 in an account earning 3% interest MONTHLY for 20 years.
Remember: Calculate inside the parenthesis first, then the exponent (240th power), and then multiply it by 2500.
Answers
Answer:
$4515
Step-by-step explanation:
Given data
Principal=$2500
Rate= 3%
Time= 20years
The compound interest formula is given as
A=P(1+r)^t
substitute
A=2500(1+0.03)^20
A=2500(1.03)^20
A=2500*1.806
A=$4515
Hence the final Amount is $4515
Someone out here know the answer?

Answers
Answer:
k = -5
Step-by-step explanation:
f(9) = 2/3*9 +k
f(9) = 6+k
k = -5
O GRAPHS AND FUNCTIONSEven and odd functions: Problem type 1

Answers
We have the following functions, given algebraically, and as a graph:
And we have to determine if the functions are even, odd, or neither.
To determine each case, we need to recall when a function is even, or odd as follows:
• A function is odd if we have that:
\(f(-x)=-f(x)\)And we can say that the function is symmetric with respect to the origin.
• A function is even if we have that:
\(f(x)=f(-x)\)And we can say that the function is symmetric with respect to the y-axis.
Then we can conclude from the graphs that (functions r and s):
Function rFor function r, the function r graphically is not symmetric with respect to the y-axis, and neither with respect to the origin. Therefore, the function is neither odd nor even function.
Function sThe function s is symmetric with respect to the origin, that is, the function looks in the same way right side up or upside down. Then the function s is an odd function.
Function g(x)
We can analyze this function algebraically as follows:
\(g(x)=5x^2\)Then to determine if it is even we have:
\(\begin{gathered} g(x)=g(-x) \\ \\ g(-x)=5(-x)^2=5(-1x)^2=5(-1)^2(x)^2=5(1)x^2=5x^2=g(x) \\ \\ \therefore g(-x)=g(x) \end{gathered}\)Therefore, this function is even.
We can also determine if the function is odd by using a similar procedure:
\(\begin{gathered} g(-x)=-g(x) \\ \text{ We already got that }g(-x)=g(x)\ne-g(x) \\ \end{gathered}\)Therefore, the function is NOT an odd function.
Function h(x)
To determine if the function is even, we have:
\(\begin{gathered} h(x)=h(-x) \\ \\ h(-x)=7(-x)^4-2(-x)^3=7(-1x)^4-2(-1x)^3 \\ \\ h(-x)=7(-1)^4x^4-2(-1)^3x^3=7(1)x^4-2(-1)x^3 \\ \\ h(-x)=7x^4+2x^3 \\ \\ \therefore h(x)\ne h(-x) \end{gathered}\)Then the function is NOT even.
Now, we have to determine if the function is odd:
\(\begin{gathered} h(-x)=-h(x) \\ \\ -h(x)=-(7x^4-2x^3)=2x^3-7x^4 \\ \\ \text{ From the previous result, we have that:} \\ \\ -h(x)\ne h(-x) \\ \end{gathered}\)Then the function is NOT odd.
Therefore, in summary, we can conclude that:
• Function r ---> Neither
,• Function s ---> Odd
,• Function g(x) ---> Even
,• Function h(x) ---> Neither

The product of 46 and a number added to the reciprocal of a number squared.

Answers
The expression for the given word phrase is
46M + M² + 2 + 1/M²
What is an expression?An expression is a way of writing a statement with more than two variables or numbers with operations such as addition, subtraction, multiplication, and division.
Example: 2 + 3x + 4y = 7 is an expression.
We have,
Number = M
Reciprocal of M = 1/M
Now,
46 x (M + 1/M)²
Step 1:
46M + M² + 2 + 1/M²
Thus,
The expression is 46M + M² + 2 + 1/M²
Learn more about expressions here:
https://brainly.com/question/3118662
#SPJ1
witch expression is equivalent

Answers
The equivalent expression of -14 - 6 is -14 - (+6)
What are equivalent expression?Equivalent expressions are expressions that have the same value when evaluated
How to determine the equivalent expression?The expression is given as:
-14 - 6
Put the terms of the expression in brackets
-14 - (6)
Rewrite 6 as +6
-14 - (+6)
Hence, the equivalent expression of -14 - 6 is -14 - (+6)
Read more about equivalent expression at
https://brainly.com/question/2972832
#SPJ1
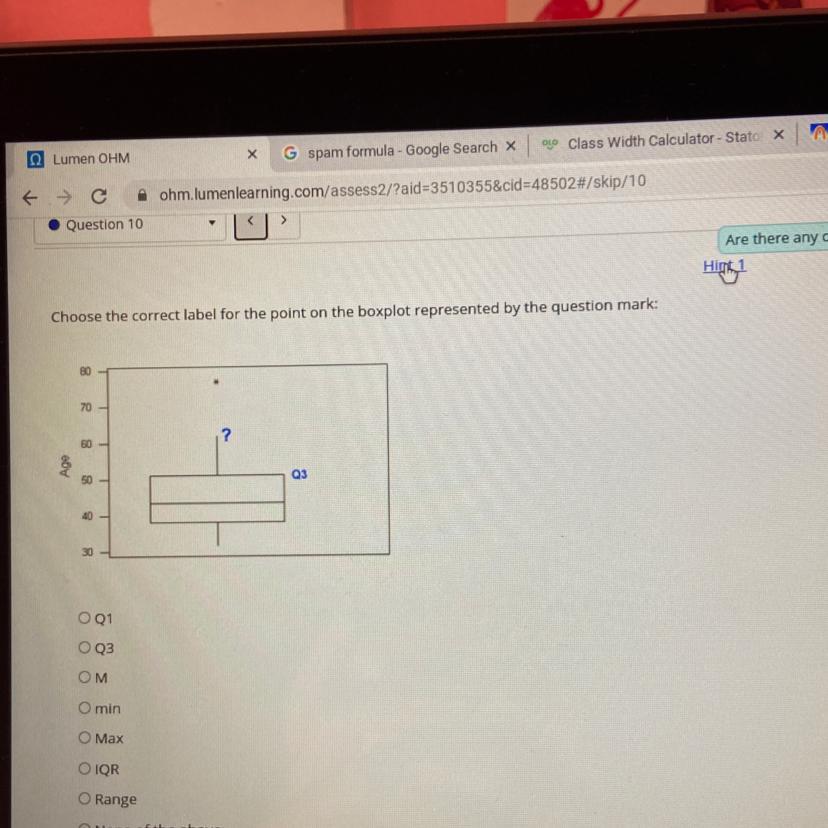
Hint 1
Choose the correct label for the point on the boxplot represented by the question mark
O 01
O Q3
OM
O min
Омах
QR
O Range
None of the above
Answers
Answer:
None of the above.
Step-by-step explanation:
The box plot given has an asterisk which represents an outlier in the data set plotted. This outlier is an extreme data set value which is obviously the maximum value in the data set.
The question mark can be regarded as the highest observation that doesn't take the outlier observation into consideration.
Therefore, none of the options given is the correct label for the point on the box plot represented by the question mark.
following box of Christmas ornaments that contains 14 silver ornaments, 12 red ornament, and 8 green ornaments. What is the probability of randomly choosing a red or green ornament from the box?
Answers
Answer: 0.5882
Step-by-step explanation:
1. Find the probability of finding each type of ornament by determining the total number of ornaments and dividing each type by the total
2. Add the probabilities of finding a green ornament and a red ornament together
components arriving at a distributor are checked for defects by two different inspectors (each component is checked by both inspectors). the first inspector detects 87% of all defectives that are present, and the second inspector does likewise. at least one inspector does not detect a defect on 26% of all defective components. what is the probability that the following occur?
Answers
The probability that a defective component will be detected only by the first inspector is 0.19
The probability that a defective component will be detected by exactly one of the two inspectors is 0.38
The probability that all three defective components in a batch escape detection by both inspectors is 0.
It is given that The first inspector detects 81% of all defectives that are present, and the second inspector does likewise.
Therefore P(A)=P(B)=81%=0.81
At least one inspector does not detect a defect on 38% of all defective components.
Therefore, bar P(A∩B)=0.38
As we know:
bar P(A∩B)=1-P(A∩B)=0.38
P(A∩B)=1-0.38=0.62
A defective component will be detected only by the first inspector.
P(A∩barB)=P(A)-P(A∩B)
=0.81-0.62
P(A∩barB)=0.19
The probability that a defective component will be detected only by the first inspector is 0.19
Part (B) A defective component will be detected by exactly one of the two inspectors.
This can be written as: P(A∩barB)+P(barA∩B)
As we know:
P(barA∩B)=P(B)-P(A∩B) and P(A∩bar B)=P(A)-P(A∩B)
Substitute the respective values we get:
P(A∩ barB)+P(bar A∩B)=P(A)+P(B)-2P(A∩B)
=0.81+0.81-2(0.62)
=1.62-1.24
P(A∩ barB)+P(bar A∩B)=0.38
The probability that a defective component will be detected by exactly one of the two inspectors is 0.38
Part (C) All three defective components in a batch escape detection by both inspectors
This can be written as: P(bar A∪ bar B)-P(bar A∩B)-P(A∩ barB)
As we know bar P(A∩B)=P(bar A∪ bar B)=0.38
From part (B): P(bar A∩B)+P(A∩bar B)=0.38
This can be written as:
P(bar A∪ bar B)-P(bar A∩B)-P(A∩bar B)=0.38-0.38=0
The probability that all three defective components in a batch escape detection by both inspectors is 0
To know more about the probability:
https://brainly.com/question/13485191
#SPJ4
Calculator
What is the volume of this figure?
Enter your answer in the box.
ft³
8 ft
25 ft
4 ft
20 ft
5 ft
Answers
The volume of the figure, by splitting it into two cuboids comes to be 3300 ft³.
What is the volume of a cuboid?The volume of a cuboid is the product of its three dimensions i.e. length, breadth, and height.
Let us split the given figure into two cuboids
The dimensions of one cuboid = 20 ft*25 ft *5ftThe dimension of the other cuboid = 4 ft*25ft * 8ftSo, the volume of the figure will be the sum of the volume of both the cuboids.
So, the volume of the cuboid with dimensions 20 ft x 25 ft x 5ft
= 20 x 25 x 5
=2500 ft³.
The volume of the cuboid with dimensions 4 ft x 25ft x 8ft
=4 x 25x 8
=800 ft³.
So, the volume of the figure = 2500 + 800 =3300 ft³.
Learn about cuboids here: https://brainly.com/question/26403859
#SPJ1

there's 240 candy bars 1/4 of candy bars are snickers 1/3 of the candy bars are twix 1/8 of the candy bars are hershey. how many candy bars are Mars? explain not with a lot of words but in numbers please.
Answers
Answer:
you have to add all the fractions of the candy1/4+1/3+1/8
=17/24
subtract from 1Step-by-step explanation:
1-17/24
=7/24
multiply with the total number of candy7/24×240
=70