The larger of two numbers is 3 fewer than 4 times the smaller number. If the
sum of the two numbers is 32, find the two numbers.
Answers
Answer:
25 and 7
Step-by-step explanation:
You want to find two numbers with a sum of 32 such that the larger is 3 less than 4 times the smaller.
SetupLet s represent the smaller number. Then the larger is (4s-3) and their sum is ...
s +(4s -3) = 32
SolutionCollect terms to get ...
5s -3 = 32
5s = 35 . . . . . . . add 3
s = 7 . . . . . . . divide by 5
4s-3 = 28-3 = 25 . . . . the larger number
The two numbers are 7 and 25.
Related Questions
Which table shows a proportional relationship between X and Y?

Answers
Your answer would be the bottom right image. In the bottom right image, x is divided by 5 consistently. No function can be determined in the other tables.
Suppose the prices of a certain model of new homes are normally distributed with a mean of 150,000. Use the 68-95-99.7 rule to find the percentage of buyers who paid between $149,000 and $151,000 if the standard deviation is $1000
Answers
The percentage of buyers is approximately 68.26% of buyers of new houses paid between \($149,000\) and \($151,000\) .
We are given that the prices of the new homes are normally distributed with a mean of \($150,000\) and a standard deviation of $1000.
Using the 68-95-99.7 rule, we know that: approximately 68% of the data falls within one standard deviation of the mean approximately 95% of the data falls within two standard deviations of the mean, approximately 99.7% of the data falls within three standard deviations of the mean.
In order to determine the proportion of customers who spent between $149,000 and , we must first determine the z-scores for these values:
z1 = (149,000 - 150,000) / 1000 = -1 z2 = (151,000 - 150,000) / 1000 = 1
Now, we can determine the proportion of data that falls between z1 and z2 using the z-table or a calculator. The region to the left of z1 is 0.1587, and the area to the left of z2 is 0.8413, according to the z-table. Thus, the region bounded by z1 and z2 is:
0.8413 - 0.1587 = 0.6826
We can get the percentage of consumers who spent between by multiplying this by 100% is \($149,000\) and \($151,000\):
0.6826 x 100% = 68.26%
Therefore, the standard deviation of customers who paid between is \($149,000\) and \($151,000\) for this model of new homes.
For such more questions on standard deviation
https://brainly.com/question/30557671
#SPJ11
Write the equation of the quadratic function in standard form given the roots are 6 and -2 and a point on the graph is (10,24)

Answers
EXPLANATION
Since we have that the roots are (6,0) and (-2,0) and a point on the graph, the canonical quadratic equation is as follows:
\(y=a(x-6)(x-(-2))\)Subtracting:
\(y=a(x-6)(x+2)\)Applying the distributive property:
\(y=a(x^2+2x-6x-12)\)Adding like terms:
\(y=a(x^2-4x-12)\)Now, in order to compute the value of a, we must plug the point (10,24):
\(24=a(10^2-4\cdot10-12)\)Multiplying numbers:
\(24=a(100-40-12)\)Adding numbers:
\(24=a(48)\)Dividing both sides by 48:
\(\frac{24}{48}=a\)Simplifying:
\(\frac{1}{2}=a\)Switching sides:
\(a=\frac{1}{2}\)Plugging in a into the equation:
\(y=\frac{1}{2}(x^2-4x-12)\)Applying the distributive property:
\(y=\frac{1}{2}x^2-2x-6\)In conclusion, the expression of the quadratic equation is as follows:
\(y=\frac{1}{2}x^2-2x-6\)tell me which situation has the correct equation and representation and inverse variation

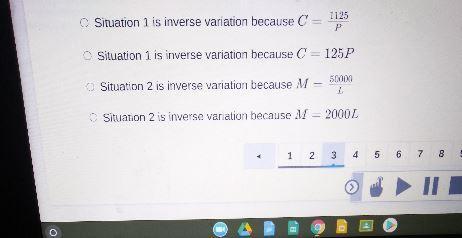
Answers
Let's begin by listing out the given information:
Direct variation refers to the relationship between two variables such that one variable is equivalent to the product of a constant & the other variable. Such that, an increase in one variable produces a commensurate increase in the second variable & a decrease in one variable produces a commensurate decrease in the other variable
Mathematically,
\(x\alpha y\Rightarrow x=k\cdot y\)Indirect variation refers to a relationship between two variables such that an increase in one variable produces a decrease in the second variable & a decrease in one variable produces a commensurate decrease in the other variable
Mathematically,
\(x\alpha\frac{1}{y}\Rightarrow x=\frac{k}{y}\)Looking at the picture, we will observe that:
Situation 1 is a direct variation (the more people sign up with the local league, the more money is generated)
Situation 2 is an indirect variation (the more money a student raises. the lesser the loan to take out)
\(\begin{gathered} 10,000M\alpha\frac{1}{5}L\Rightarrow10,000M=\frac{1}{5}L \\ L=10,000(5)=50,000M \\ \therefore M=\frac{50,000}{L} \end{gathered}\)Situation 2 is inverse variation because M = 50,000/L
2.5 Comparing Circles
Here are two circles. Their centers are A and F.
E
D
C
4 cm
A
B
H
1. What is the same about the two circles? What is different?
2. What is the length of segment AD? How do you know?
3. On the first circle, what segment is a diameter? How long is it?
8 cm
G

Answers
The same thing on both the circles is that they both have the same diameter, the length of AD is 4 cm and the segment which represents the diameter is EB.
What is a Circle?An irregular plane figure is a circle. Every spot on the circle is equally distant from the circle's fixed center. It has a radius of 1, making it a 2D shape. The Latin word "circulus," which means "little ring," is the source of the English word "circle."
As per the given information in the question,
1.
There are two circles given,
The radius of the first circle = 4 cm
Then, its diameter will be,
D = 2r = 2 × 4 = 8 cm
And, the diameter of the second circle is also 8 cm. It means that both are same on this aspect.
The second has three segments whereas the first only has two, hence the segments are distinct.
2.
The length of the segment AD will be equal to 4 cm because it is from the center of the circle to the circumference of the circle then it becomes equal to the radius, so the segment must be of 4 cm.
3.
On the first circle, the segment which represents the diameter is EB. Because as per the definition of diameter, a line connected with two points which passes through the center of circle is said to be the diameter.
To know more about Circle:
https://brainly.com/question/29142813
#SPJ1
Some students are making muffins for a fundraiser. They have already made 80 muffins
and they can make 30 muffins in an hour. How many additional hours would they spend
to make 380 muffins?
Answers
Answer:
10 hours
Step-by-step explanation:
380 - 80 = 300
30 : 1 = 300 : x
x = 300 / 30
x = 10
A man gave 90000.00 to his two daughters Jane and Lydia, 75.00 was given to Lydia to pay her load. After sharing the money Lydia has twice as
much as Jane. How much did each received?
Answers
Jane received $30025 and Lydia received $60000. Let's assume the amount of money that Jane received as x; then Lydia's share of the money will be twice the share of Jane.
We are to find out the share of each person. Here is the solution in steps:Suppose Jane's share was x dollars, and Lydia's share was y dollars.
Given that the total amount given to the two daughters was $90000. Also, given that Lydia paid off her $75, hence she got $75 less than Jane.
Therefore, y = 2x - 75; this is because we are given that Lydia got twice the share of Jane, and also, she got $75 less than Jane. Hence, x + y = $90000, this is because the total sum of money shared is $90000.
Substituting y = 2x - 75 into x + y = $90000 gives x + (2x - 75) = $90000.
Simplifying, we have :3x = $90000 + 75 = $90075.
Dividing both sides by 3, we get:x = $30025. Hence, Jane's share is $30025 Lydia's share = 2x - 75 = 2($30025) - $75 = $60075 - $75 = $60000.
Therefore, Jane received $30025 and Lydia received $60000.
For more question on amount
https://brainly.com/question/25720319
#SPJ8
What's the solution to the following linear system?
y = 4x + 1
y = 4x
Question 4 options:
(−2, 3)
(4, 0)
Infinitely many solutions
No solution
Answers
Answer:
No solution
Step-by-step explanation:
4x = 4x + 1
0 unequal to 1
Answer: D. No solution
please mark Brainliest :)
Solve the equation: 9-15x = 28 + 4x
Answers
Answer:
x = -1
Step-by-step explanation:
To solve this, we want to isolate x:
9 - 15x = 28 + 4x
Subtract 9 from both sides:
-15x = 19 + 4x
Subtract 4x from both sides:
-19x = 19
Divide both sides by -19:
x = -1
the shortest side of a right triangle measures 7m. The lengths of the other two sides are Consecutive integers. What is the length of the other two sides?
Answers
The lengths of the other two sides of the right triangle are 24m and 25m, respectively.
Let's assume the consecutive integers representing the lengths of the other two sides of the right triangle are x and x + 1, where x is the smaller integer. We are given that the shortest side measures 7m. Now, we can use the Pythagorean theorem to solve for the lengths of the other two sides.
According to the Pythagorean theorem, in a right triangle, the square of the length of the hypotenuse (the longest side) is equal to the sum of the squares of the lengths of the other two sides.
Using this theorem, we have the equation:
\(7^2 + x^2 = (x + 1)^2\)
Expanding and simplifying this equation, we get:
\(49 + x^2 = x^2 + 2x + 1\)
Now, we can cancel out \(x^2\) from both sides of the equation:
49 = 2x + 1
Next, we can isolate 2x:
2x = 49 - 1
2x = 48
Dividing both sides by 2, we find:
x = 24
Therefore, the smaller integer representing the length of one side is 24, and the consecutive integer representing the length of the other side is 24 + 1 = 25.
Hence, the lengths of the other two sides of the right triangle are 24m and 25m, respectively.
For more question on lengths visit:
https://brainly.com/question/28322552
#SPJ8
what is the expression, 2x^2+5y-8 when x=5 and y=-2?

Answers
Answer:
Step-by-step explanation:
Substitute x=5 and y=-2 in the expression:
2x^2+5y-8 = 2(5)^2 + 5(-2) - 8
= 2(25) - 10 - 8
= 50 - 18
= 32
Therefore, the value of the expression 2x^2+5y-8 when x=5 and y=-2 is 32.
Answer: C) 32
Step-by-step explanation:
The exercise is 2x² + 5y - 8
Here we substitute in the exercise x and y.
x = 5y = -2We follow the PEMDAS order of operations:
P - PatentsE - ExponentsM - MultiplicationD-DivisionA - AdditionS - SubtractionWe substitute and solve:
2(5)² + 5(-2) - 8
2(25) + 5(-2) - 8
50 + 5(-2) - 8
50 - 10 - 3
32 ===> Alternative C
In Texas, 30% of parolees from prison return to prison within 3 years.Suppose 15 prisoners are released from a Texas prison on parole. Assume that whether or not one prisoner returns to prison is independent of whether any of the others return to prison. Let the random variable X be the number of parolees out of 15 that return to prison within 3 years. What is the probability that more than 5 parolees out of 15 that return to prison within 3 years?
Answers
Answer:
The probability that more than 5 parolees out of 15 that return to prison within 3 years is 0.2784.
Step-by-step explanation:
The random variable X be the number of parolees that return to prison within 3 years.
The probability of occurrence of the random variable X is, p = 0.30.
A random sample of n = 15 prisoner are selected.
It is assumed that whether or not one prisoner returns to prison is independent of whether any of the others return to prison.
The random variable X follows a binomial distribution with parameters n = 15 and p = 0.30.
Compute the probability that more than 5 parolees out of 15 that return to prison within 3 years as follows:
\(P(X>5)=1-P(X\leq 5)\)
\(=1-\sum\limits^{5}_{0}{{15\choose x}(0.30^{x}(1-0.30)^{15-x}}\\\\=1-[0.00475+0.03052+0.09156+0.17004+0.21862+0.20613]\\\\=0.27838\\\\\approx 0.2784\)
Thus, the probability that more than 5 parolees out of 15 that return to prison within 3 years is 0.2784.
Estimate the area of a rectangle with length 5/8 foot and a width of 4/9 foot.
Answers
Hello there!
Area = Length * Width
Area = \(\frac{5}{8} * \frac{4}{9}\)
Area = \(\frac{5}{18}\)
Thanks!
~Garebear
Ok so I need help my little sister is asking me this but I dont remember how to do this stuff so could someone help ? ill give brainliest as soon as possible, thanks </3.

Answers
Answer:
the second one 253
Step-by-step explanation:
There are 135 people in a sport centre.
77 people use the gym.
62 people use the swimming pool.
65 people use the track.
27 people use the gym and the pool.
23 people use the pool and the track.
31 people use the gym and the track.
4 people use all three facilities.
How many people use at least two
facilities?
Answers
Answer:
he total number of people who use at least two facilities is 4 + 45 = 49
Step-by-step explanation:
To find the number of people who use at least two facilities, we can find the number of people who use all three facilities and add the number of people who use only two facilities.
The number of people who use all three facilities is 4, and the number of people who use only two facilities is 27 + 23 + 31 - 3 * 4 = 45.
Therefore, the total number of people who use at least two facilities is 4 + 45 = 49.
the unit rate for each option. 9. Option A: $15.40 for 3.5 lbs of gummy bears
Answers
The unit rate for Option A is $4.4 per pound of gummy bears.
The unit rate for each option is a mathematical expression that compares two quantities of different measures by dividing one quantity by the other.
The unit rate is used to determine how much an item costs per unit of measure.
To find the unit rate for each option, we can use the following formula: Unit rate = price ÷ weight Option A: $15.40 for 3.5 lbs of gummy bears Unit rate = 15.40 ÷ 3.5Unit rate = $4.4 per pound of gummy bears.
Therefore, the unit rate for Option A is $4.4 per pound of gummy bears.
For more such questions on gummy bears
https://brainly.com/question/25831000
#SPJ8
Here is the complete question given below:
The unit rate for each option. 9. Option A: $15.40 for 3.5 lbs of gummy bears. $7.65 for 1.8 Ibs of gummy bears.
How do the expressions 72 divided by 9 and Negative 72 divided by (negative 9) compare when they are evaluated?
They have different values and are different signs.
They have different values but are the same sign.
They have the same value but are different signs.
They have the same value and the same sign.
Answers
Answer:
D
Step-by-step explanation:
Hunter started with the following model to show the problem 1 . 45 ÷ 5 1 . 45 ÷ 5 . One 10 by 10 flat, 4 rods of 10 cubes , and 5 single cubes are shown. To complete the division model of 1 . 45 ÷ 5 1 . 45 ÷ 5 , how many hundredths would there be in each of the division groups? A. 1 B. 2 C. 5 D. 9
Answers
The model Hunter uses makes the division possible given that each
number of digits in a place value are represented by a cube.
The number of hundredth in each division group is D. 9Reasons:
The model Hunter started to show the problem is as follows;
Number of 10 by 10 flat = 1
Number of rods of 10 cubes = 4
Number of single cubes = 5
The division is 1.45 ÷ 5, which can be expressed as follows;
\(\displaystyle \frac{1.45}{5}\)
Given that the 5 cubes represent the .05 in 1.45, we have;
1 cube = 0.01
Dividing each of the set by 5 gives, for each of the division group;
10 by 10 flat ÷ 5 = 10 × 2 flat = 20 cubes = 20 × 0.01 = 0.2
4 rods × 10 cubes ÷ 5 = 40 cubes ÷ 5 = 8 cubes = 8 × 0.01 = 0.08
5 cubes ÷ 5 = 1 cube = 1 × 0.01 = 0.01
Therefore, each of the division group is 0.2 + 0.08 + 0.01 = 0.29
The tenths are the digits after the decimal point to the right.
The hundredths are the digits in the second position after the decimal point to the right.
The number of tenths in each group, (0.2) = 2
There are 2 tenths in each group
The number of hundredths in each group, (0.09) = 9
There are 9 hundredths in each group.
Therefore;
The correct response to the question on how many hundredths would be there in each of the division groups is D. 9
Learn more about division and place values here:
https://brainly.com/question/3060423
https://brainly.com/question/14021681
https://brainly.com/question/574471
Find the area of the figure.
Use a = 3.14
10 ft,
16 ft
8 ft

Answers
Answer: A = 132 . 93 cm 2
Step-by-step explanation:
A = lw
A = 8.7
A = 56cm2
A=2
A=(3.14)(7) 2
A 153 . 86 cm 2
76. 93 cm 2
A = 56cm 2 + 76.93 cm 2
A = 132 . 93 cm 2
Can anyone help me find the correct angle for ZWP?

Answers
Answer:
56 degrees
Step-by-step explanation:
Here, I think you just accidentally put the value for the angle ZWX, which would be ZWP+PWX(56+56).
You actually already wrote it on the paper, so nothing wrong with your math here, just a thinking error :)
To calculate simple interest, the amount of interest, I, can be found using this formula, where P is the principal, r is the interest rate, and t is time: I=Prt Which formula can be used to determine the interest rate when the other variables are known? a.) r=IPt b.)r=I/Pt c.)r=IP/t d.) r=Pt/I
Answers
Answer:
b
Step-by-step explanation:
I = Prt Solve the equation for r
I = Prt Divide both sides by Pt to get r on one side of the equation by itself
Pt Pt Cancel the P and t on right side as P/P and t/t = 1
This leaves: I = r or r = I
Pt Pt
The interest rate formula with other known variables is R=I/PT. Therefore, the correct answer is option B.
The given simple interest formula is I=PRT.
Where, P is the principal, r is the interest rate, and t is time.
Simple interest is a method to calculate the amount of interest charged on a sum at a given rate and for a given period of time.
To calculate the interest rate:
By rearranging the formula I=PRT, we get
R=I/PT
Therefore, the correct answer is option B.
To learn more about the simple interest visit:
https://brainly.com/question/25845758.
#SPJ4
1. a committee consists of 4 chemical engineers and 3 electrical engineers. this committee is to be formed from a group of 6 chemical engineers and 5 electrical engineers. find the number of ways in which this can be done if (a) any ee and any ce can be included. (b) one particular ce must be in the committee. (c) two particular ce cannot be in the same committee.
Answers
A) There are 330 possible ways to form the committee if any EE and any CE can be included.
B) There are 210 possible ways to form the committee if one particular CE must be in the committee.
C) The total number of ways to form the committee with two particular CE excluded is 205
In this case, we are given a scenario where a committee is to be formed from a group of chemical and electrical engineers. Let's dive into the details of the problem and explore how probability can be used to solve it.
(a) If any EE and any CE can be included, we need to find the number of ways to form a committee of 7 members from a group of 6 CE and 5 EE. In this case, the order in which the committee members are selected does not matter, so we can use the formula for combinations.
The total number of ways to select 7 members from a group of 11 engineers is given by:
C(11,7) = 11! / (7! * 4!) = 330
(b) If one particular CE must be in the committee, we can first select that CE and then form the rest of the committee from the remaining engineers. The probability of selecting that particular CE is 1/6, since there are 6 CE in total.
Once we have selected that particular CE, we need to select 6 more members from a group of 5 EE and 5 CE (excluding the one we have already selected). The total number of ways to do this is given by:
C(10,6) = 10! / (6! * 4!) = 210
(c) If two particular CE cannot be in the same committee, we can use the principle of inclusion-exclusion to find the total number of ways to form the committee.
First, we find the total number of ways to form the committee without any restrictions. This is given by:
C(11,7) = 330
Next, we find the number of ways to form the committee with both particular CE included. This is given by:
C(9,5) = 126
We subtract this from the total number of ways to form the committee to get the number of ways with at least one of the particular CE excluded:
330 - 126 = 204
However, we have counted the case where both particular CE are excluded twice, so we need to add this back in:
C(7,7) = 1
Therefore, the total number of ways to form the committee with two particular CE excluded is:
204 + 1 = 205
To know more about probability here.
https://brainly.com/question/11234923
#SPJ4
.........................

Answers
Step-by-step explanation:
→ (1.2*(10^57)) + 1.2*(10^56) → 1.32e+57→ 1.32 × 10^57 1.(drag) → 1.32 and 2. (drag) → 57» 1.32e+57
explain why the graph would look like the line y=x if you graph it

Answers
The graph of f(x) = x(x+5)(x+3) / (x+3)(x+5) bears resemblance to the line y=x since both plots share similar linear characteristics.
How to explain the informationBoth the numerator and denominator of f(x) = x(x+5)(x+3) / (x+3)(x+5) cancel out the factors (x+3) and (x+5), giving us a simplified expression for the function, f(x) = x.
A line with a slope of 1 passes through the origin in an upward direction. When we examine the graph of y=x, which is a straight line passing through the origin with a slope of 1, it becomes apparent that adding 1 to x also adds 1 to y.
Since the simplified expression is merely f(x) = x, we can deduce that the graph of this function follows a linear path passing through the origin with a slope of 1.
In conclusion, the graph of f(x) = x(x+5)(x+3) / (x+3)(x+5) bears resemblance to the line y=x since both plots share similar linear characteristics.
Learn more about factor on
https://brainly.com/question/25829061
#SPJ1
The count in a bacteria culture was 500 after 15 minutes and 1500 after 30 minutes. Assuming the
count grows exponentially,
What was the initial size of the culture?
Find the doubling period.
Find the population after 105 minutes.
When will the population reach 12000.
You may enter the exact value or round to 2 decimal places.
Answers
Hence, it will take about 43.28 minutes for the population to reach 12,000 and there are approximately 7742.85 people in the world after 105 minutes.
how can we describe logarithm ?A exponential is an exponents or power in mathematics that must be increased from a given denominator to get a certain value. In other words, just as division is the opposite of multiplication,
the logarithm is the greater operating of exponentiation. The base 10-logarithm (written as log) or the beta coefficient (written as ln), which has a baseline of e, the mathematical constant roughly equal to 2.71828, are the two most widely used logarithms.
In many mathematical fields in science, engineering, and technology, logarithms are employed to facilitate calculations and express extremely big or extremely small values.
given
a) The culture's initial size is:
P(0) = \(500/e^{15415*15}\)
≈ 98.90
b) We can easily enter t = 105 into the exponential growth model to determine the population after 105 minutes:
P(105)=98.9*\(e^{15415*105}\)
≈ 7742.85
Hence, there are approximately 7742.85 people in the world after 105 minutes.
d) To determine the population's peak, we set P(t) = 12000 and solve for t as follows:
1200=98.9*\(e^{0.15415t(0.15415t)}\)
By taking the natural logarithm and dividing both sides by 98.90, we arrive at:
ln(12000/98.90) = 0.15415t
To solve for t, we obtain:
t ≈ 43.28
Hence, it will take about 43.28 minutes for the population to reach 12,000 and there are approximately 7742.85 people in the world after 105 minutes.
To know more about logarithm visit:
brainly.com/question/30085872
#SPJ9
Please help me! 5:34 est answer as soon as you can!!

Answers
B because it is the only one were the 'y-coordinate' is not the same for 2 different 'x-coordinates'
hope this helps!!
what is the place value of 8 in fullowing number? 348000
Answers
Answer: 8000
Step-by-step explanation:
The 8 in the number 348,000 is in the thousands place.
So it represents the value of 8,000 .
Where is the function increasing?

Answers
9514 1404 393
Answer:
A) 1 < x < ∞
Step-by-step explanation:
"Increasing" means the graph goes up to the right. This graph is increasing for values of x greater than 1. (x=1 is a minimum. The graph is decreasing toward the minimum on the left of that value, and increasing from the minimum on the right of that value.)
1 < x < ∞
Answer:
A if your doing prep
Step-by-step explanation:
math
Cliff takes out a $5,000 personal loan with 7
fixed annual interest compounded monthly to pay for his wedding. He repays the loan in 2 year.s
How much total interest does Cliff pay on his loan?
Answers
Cliff pays a total interest of approximately $679.90 on his $5,000 loan.
To calculate the total interest paid on the loan, we need to use the formula for compound interest:
\(A = P(1 + r/n)^{(nt)}\)
Where:
A is the final amount (loan amount + interest)
P is the principal (loan amount)
r is the annual interest rate (in decimal form)
n is the number of times interest is compounded per year
t is the number of years
Given that Cliff takes out a $5,000 loan with a fixed annual interest rate of 7% compounded monthly, we can substitute the values into the formula:
P = $5,000
r = 7% = 0.07
n = 12 (monthly compounding)
t = 2 years
\(A = 5000(1 + 0.07/12)^{(12 \times 2)\)
Calculating this expression:
A ≈ 5000\((1.00583)^{(24)\)
A ≈ 5000(1.13598)
A ≈ 5679.90
The final amount (A) is the loan amount plus the total interest paid. Therefore, to find the total interest paid, we subtract the principal (P) from the final amount (A):
Total Interest = A - P
Total Interest = 5679.90 - 5000
Total Interest ≈ $679.90
For similar question on total interest.
https://brainly.com/question/29415701
#SPJ8
100 POINTS PLUS BRAINLIEST!!!!
screenshot with problem attached below
answers must be serious
non-serious answers / incorrect answers will be deleted
(ATTACHEMENT BELOW)

Answers
Answer:
see step by
Step-by-step explanation:
a) the polynomial must be fifth degree, so it must have a \(x^5\) term, also need to have 2 additional terms (Lets add any, lets say \(x^2+8\) (notice this is totally random, just need to be under 5th degree)
So a polynomial can be
\(x^5+x^2+8\)
notice is in standard form since degrees drops from left to right.
Also notice there's an infinite amount of possible answers
b) p-q is the same as -q+p
For example, lets say
\(p=x+1\)
\(q=3x^2-5\)
\(p-q=x+1-(3x^2-5)=x+1-3x^2+5=-3x^2+x+6\)
also
\(-q+p=-(3x^2-5)+x+1=-3x^2+5+x+1=-3x^2+x+6\)
notice is the same expression.