The manger of a company planned to distribute a $50 bouns to each employee from the company fund, but the fund contained $5 less than what was needed. Instead, the manger gave each employee a $45 bouns and kept the remaing $95 in the company fund. How much money was in the company fund before any bonuses were paid?
Answers
The total company fund before any bonuses were paid is $995 and there were 20 staffs.
What is an equation?An equation is an expression that shows the relationship between two numbers and variables.
An independent variable is a variable that does not depend on any other variable for its value whereas a dependent variable is a variable that depend on any other variable for its value.
Let y represent the total company fund and x represent the number of staffs.
From the first statement:
y - 50x = -5 (1)
Also:
y - 45x = 95 (2)
From equation 1 and 2:
x = 20, y = 995
The total company fund before any bonuses were paid is $995 and there were 20 staffs.
Find out more on equation at: https://brainly.com/question/2972832
#SPJ1
Related Questions
Cate's Bake Shop has a clearance on day-old baked goods. Loaves of bread *
that usually sell for $2.35 are on sale for $1.39. What is the markdown?
O $0.98
O $0.96
O $0.88
O $0.89
Answers
take 2.35 and subtract 1.39 from it
A distribution has the five-number summary shown below. What is the
interquartile range (IQ) of this distribution?
Answers
Answer:
tiookvgvc. jbjvth kivtcth jjvf h. bkbgv
Answer:
The IQR of the given distribution is
Step-by-step explanation:
The given distribution has the five-number
28, 34, 43, 59, 62
Divide these numbers in two equal parts.
(28, 34), 43,( 59, 62)
Now divide each parenthesis in two equal parts.
(28), (34), 43,( 59), (62)
It means first quartile is the average of 28 and 34. Third quartile is the average of 59 and 62.
The interquartile range (IQR) of this distribution is
Therefore the IQR of the given distribution is 29.5.
Round 0.93 to the nearest tenth.
Answers
Answer:
the answer would be 0.9
Step-by-step explanation:
when rounding anything under 5 remains the same and anything over 5 rounds up to the next number. in this case three is less than five so the 9 would remain the same
Is 17/23 a irrational number
Answers
Answer:
NO.
Step-by-step explanation:
An irrational number cannot be written as a fraction.
make x the subject of the formula in R = ax-p/q+bx
Answers
R = (ax - p)/(q + bx)
(ax - p) = R(q + bx)
ax - p = Rq + Rbx
ax - Rbx = Rq + p
x(a - Rb) = Rq + p
x = (Rq + p)/(a - Rb)
0.36% as a fraction in simplest form
Answers
Answer: 9/2500
Step-by-step explanation:
We start with turning it into a fraction:
0.36/100
Then, we turn every number into a whole number by multiplying by whatever the last decimal place is called (hundreth= x100)
36/10000
Lastly, we simplify as much as we can by finding the least common denominator. In this case, the smallest whole number both 36 and 10000 can be divided by is 4:
(36/4)/(10000/4)
= 9/25000
Simplify the expressions:
−8y³ x 5y8
Answers
I hope this helps.
Hi! Your answer is 11. When multiplying terms with exponents, the exponents add up.
Step-by-step explanation:
You want to multiply the terms with the exponents. You should get 3 and 8, add those up and you will get 11.
Hope this helps! Have a good day :)
4) A snowboarder descends down a mountain at a rate of 15 feet per second. How far will she travel after 9 seconds?
Answers
Which of the points is on a line with positive slope?
A
(-6, 1) and (-6, 9)
B
(2, 6) and (11, -3)
C
5, 0) and (8, 4)
D
(3, 4) and (-2, 12)
Answers
it’s the only one when graphed that the line (from left to right) goes up,
help please!! m=5 and n=2. 2m-5n!
Answers
Step-by-step explanation:
so, what's the problem ?
it's all there, we only need to take the calculator (or simply our heads) and do the calculation :
2×5 - 5×2 = 10 - 10 = 0
you see ? that was all that was needed.
put the given values for the variables into the places of these variables, and then calculate !
that is what variables are for : placeholders for actual values.
CAN SOMEONE HELP WITH THIS QUESTION?✨

Answers
The percentage error in the density of metal will be 3.4%.
What is the percentage?The percentage is defined as representing any number with respect to 100. It is denoted by the sign %. The percentage stands for "out of 100." Imagine any measurement or object being divided into 100 equal bits.
Given that the density of the metal in the handbook is 4.018 g/mL and the measured value of the density is 4.16 g/mL.
The percentage error will be calculated as:-
Percentage = [ ( 4.16 - 4.018 ) / 4.16 ] x 100
Percentage = 0.034x 100
Percentage = 3.4%
Hence, the percentage error will be 3.4% for the densities.
To know more about the percentage follow
https://brainly.com/question/1600371
#SPJ1
. Three professors examined awareness of four widely disseminated retirement rules among employees at University of Utah. These rules provide simple answers to questions about retirement planning. At the time of the investigation, there were approximately 10,000 benefited employees, and 3,095 participated in the study. Demographic data collected on these 3,095 employees included gender, age (years), education level (years completed), marital status, household income ($), and employment category.(30pts)(a) Describe the population of interest.(b) Describe the sample that was collected.(c) Indicate whether each of the demographic variables mentioned is categorical or numerical.
Answers
Answer:
a) The population of interest = 10,000 benefited employees. It includes all benefited employees.
b) The sample collected = 3,095. These are the benefited employees who participated in the study.
c) Indication of demographic variables as categorical or numerical:
Demographic data: Categorical or Numerical
Gender = Categorical
Age (years) = Numerical
Education level = Numerical
(years completed)
Marital status = Categorical
Household income ($) = Numerical
Employment Category = Categorical
Step-by-step explanation:
a) Data and Calculations:
Population of interest = 10,000 benefited employees
Sample = 3,095 participants
Demographic data:
Gender
Age (years)
Education level (years completed)
Marital status
Household income ($)
Employment Category
b) A variable is numerical value (e.g. length, test scores, etc.). It is a continuous variable. A categorical variable assumes a limited or fixed number of possible values, or it is categorized in groups.
Solve.
b + 2/9 = 1 3/5
Answers
B=107/45
Decimal form
B=2.36
Mixed number form
B=2:17/45
- 3X + 6Y = 0 and x+7y=9
Answers
Y = 1
-3(2) + 6(1) = 0
(2) + 7(1) = 9
In the diagram, the measures of 23 and 27 are 45°. The measure of 25 is
135°. Are lines cand dparallel?
F
5
8
OA. Yes, because 23 and 27 are congruent.
OB. No, because 27 and 25 are not congruent.
C. Yes, because 25 and 27 are supplementary.
D. No, because 23 and 25 are not supplementary.
Answers
The correct answer is D. No because 23 and 25 are not supplementary.
In the given diagram, it is stated that the measures of angles 23 and 27 are 45°, and the measure of angle 25 is 135°. To determine if lines C and D are parallel, we need to analyze the angles formed by these lines.
If the alternate interior angles or corresponding angles are congruent, then the lines are parallel. However, in this case, we don't have enough information about the angles formed by lines C and D to make that determination.
The fact that angle 23 and angle 27 are congruent (both measuring 45°) doesn't provide any information about the relationship between lines C and D. Similarly, the measure of angle 25 being 135° doesn't give us any insight into the parallelism of lines C and D. Therefore, we cannot conclude that lines C and D are parallel based on the given information, and the correct answer is D.
For more such answers on supplementary angle
https://brainly.com/question/12919120
#SPJ8
geometry need help asap

Answers
The value of angle LAF in the intersecting chords is determined as 104⁰.
What is the value of angle LAF?The value of angle LAF is calculated by applying intersecting chord theorem, which states that the angle at tangent is half of the arc angle of the two intersecting chords.
Also this theory states that arc angles of intersecting secants at the center of the circle is equal to the angle formed at the center of the circle by the two intersecting chords.
m∠LAF = ¹/₂ (arc LF + arc YS)
From the diagram, we have arc LF = 160⁰ and YS = 48⁰
m∠LAF = ¹/₂ (160 + 48)
m∠LAF = 104⁰
Thus, the value of angle LAF is calculated by applying intersecting chord theorem.
Learn more about chord angles here: brainly.com/question/23732231
#SPJ1
Use a system of equations to solve the following problem.The local theater has three types of seats for Broadway plays: main floor, balcony, and mezzanine.Main floor tickets are $52, balcony tickets are $37, and mezzanine tickets are $31. One particularnight, sales totaled $50,512. There were 86 more main floor tickets sold than balcony and mezzaninetickets combined. The number of balcony tickets sold is 139 more than 2 times the number ofmezzanine tickets sold. How many of each type of ticket were sold?

Answers
Main floor ticket is $52
Balcony ticket is $37
Mezzanine ticket is $31
Let x represent main floor tickets
Let y represent balcony tickets
Let z represent main mezzanine tickets
On a particular day, total sales totaled $50512, i.e
\(\begin{gathered} 52\times x+37\times y+31\times z=50512 \\ 52x+37y+31z=50512\ldots(1) \end{gathered}\)There were 86 more main floor tickets sold than balcony and mezzanine tickets combined, i.e
\(\begin{gathered} y+z+86=x \\ x-y-z=86\ldots(2) \end{gathered}\)The number of balcony tickets sold is 139 more than 2 times the number of mezzanine tickets sold, i.e
\(\begin{gathered} 2z+139=y \\ y-2z=139\ldots(3) \end{gathered}\)The equatons are
\(\begin{gathered} 52x+37y+31z=50512\ldots(1) \\ x-y-z=86\ldots(2) \\ y-2z=139\ldots(3) \end{gathered}\)Solving to find the values of x, y and z
From equation (3), make y the subject
\(\begin{gathered} y-2z=139 \\ y=139+2z\ldots(4) \end{gathered}\)Substitute for y into equation (2)
\(\begin{gathered} x-y-z=86 \\ x-(139+2z)-z=86 \\ x-139-2z-z=86 \\ x-139-3z=86 \\ x-3z=225 \end{gathered}\)Make x the subject
\(\begin{gathered} x-3z=225 \\ x=225+3z\ldots(5) \end{gathered}\)Substitute for x and y into equation (1)
\(\begin{gathered} 52x+37y+31z=50512 \\ 52(225+3z)+37(139+2z)+31z=50512_{} \\ 11700+156z+5143+74z+31z=50512 \\ \text{Collect like terms} \\ 156z+74z+31z=50512-11700-5143 \\ 261z=33669 \\ \text{Divide both sides by 261} \\ \frac{261z}{261}=\frac{33669}{261} \\ z=129 \end{gathered}\)Substitute for z into equation (5) to find x
\(\begin{gathered} x=225+3z \\ x=225+3(129) \\ x=225+387 \\ x=612 \end{gathered}\)Substitute for z into equation (4) to find y
\(\begin{gathered} y=139+2z \\ y=139_{}+2(129) \\ y=139+258 \\ y=397 \end{gathered}\)Hence,
The number of main floor tickets (x) sold is 612
The number of balcony tickets (y) sold is 397
The number of mezzanine tickets (z) sold is 129
What is the y-intercept of the graph of 4x-10y =20???
Answers
10y = 4x - 20
y = 4/10x - 2
Solution: y intercept is -2
Find the rate of change demonstrated in the graph below.

Answers
The rate of change of a graph, is the slope of the graph.
The rate of change of the graph is 4.5
The points on the graph are:
\(\mathbf{(x,y) = (2,9) (4,18)}\)
So, the slope (m) is calculated as:
\(\mathbf{m = \frac{y_2 - y_1}{x_2 - x_1}}\)
This gives
\(\mathbf{m = \frac{18 - 9}{4-2}}\)
\(\mathbf{m = \frac{9}{2}}\)
\(\mathbf{m = 4.5}\)
Hence, the rate of change of the graph is 4.5
Read more about rates at:
https://brainly.com/question/12786410
The Acme Company manufactures widgets. The distribution of widget weights is bell-shaped. The widget weights have a mean of 48 ounces and a standard deviation of 3 ounces. Use the 68-95-99.7 Rule and a sketch of the normal distribution in order to answer these questions. a) 95% of the widget weights lie between and b) What percentage of the widget weights lie between 39 and 54 ounces? % c) What percentage of the widget weights lie above 45 ?
Answers
After calculating we found that: a) 95% of widget weights lie between 42 and 54 ounces.b) 97.35% of widget weights lie between 39 and 54 ounces and c) 0.3% of widget weights lie above 45 ounces.
a) Since the distribution is bell-shaped and the mean is 48 ounces with a standard deviation of 3 ounces, we can use the 68-95-99.7 Rule to determine that 68% of the widget weights lie within one standard deviation of the mean, 95% lie within two standard deviations of the mean, and 99.7% lie within three standard deviations of the mean. Thus, we know that 95% of the widget weights lie between:
48 - 2(3) = 42 ounces and 48 + 2(3) = 54 ounces.
b) To find the percentage of widget weights that lie between 39 and 54 ounces, we need to determine how many standard deviations away from the mean these values are.
39 ounces is 9 ounces below the mean, so it is (9/3) = 3 standard deviations below the mean.
54 ounces is 6 ounces above the mean, so it is (6/3) = 2 standard deviations above the mean.
Using the 68-95-99.7 Rule, we know that 99.7% of the widget weights lie within three standard deviations of the mean. Since 39 ounces is three standard deviations below the mean, we can conclude that 0.15% (or 0.003 x 100) of the widget weights lie below 39 ounces.
Likewise, since 54 ounces is two standard deviations above the mean, we know that 2.5% of the widget weights lie above 54 ounces. Thus, the percentage of widget weights that lie between 39 and 54 ounces is:
100% - 0.15% - 2.5% = 97.35%
c) We need to find the percentage of widget weights that lie above 45 ounces. Since 45 ounces is three standard deviations below the mean, we know that 99.7% of the widget weights lie above 45 ounces. Therefore, the percentage of widget weights that lie above 45 ounces is:
100% - 99.7% = 0.3%.
To know more about standard deviation, visit: https://brainly.com/question/23907081
#SPJ1
The product of (3 - 4i) and ____ is 25.
Answers
Answer:
( 3 + 4i )
Step-by-step explanation:
For this problem, we need to know that i^2 = -1. With this, let's solve the multiplication problem.
So to get rid of the i, we know it needs to be squared. The question is, for the sun of the products do we have a -4i or a +4i? So we will test both.
( 3 - 4i )^2 ?= 25
( 3 - 4i )( 3 - 4i ) ?= 25
9 - 12i - 12i - 16 ?= 25
We can stop here since we know that 24i will never equal 25.
So let's try the -4i
( 3 + 4i )( 3 - 4i ) ?= 25
9 + 12i - 12i + 16 ?= 25
9 + 16 ?= 25
25 == 25
Thus, our term for this to be true is ( 3 + 4i ).
Cheers.
Can someone please help me out? Thank you! I will give brainly
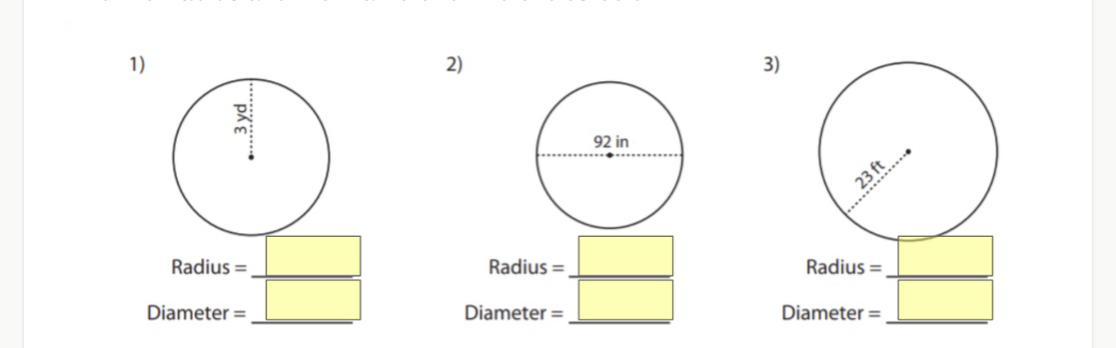
Answers
1) In first circle radius is 3yard and diameter is 6 yard
2) In second circle radius is 46 inch and diameter is 92 inch
3)In third circle radius is 23 ft and diameter is 46ft
What's the radius solution?
The radius formula is easily obtained by halving the circle's diameter. The line segment created when we join a point on a circle's circumference to its exact center is referred to as the ring's radius. The radius of a circle is the separation between its center and circumference.
What is diameter, for instance?
If you look at the cycle wheel, the spikes that run through the center from one end to the other are an illustration of diameter. This is comparable to the diameter of a circle, which is the line segment that extends from one end of the circle to the other end while passing through the center of the circle.
Learn more about diameter and radius
https://brainly.com/question/390660
#SPJ1
John wishes to choose a combination of two types of cereals for breakfast - Cereal A and Cereal B. A small box (one serving) of Cereal A costs $0.50 and contains 10 units of vitamins, 5 units of minerals, and 15 calories. A small box (one serving) of Cereal B costs $0.40 and contains 5 units of vitamins, 10 units of minerals, and 15 calories. John wants to buy enough boxes to have at least 500 units of vitamins, 600 units of minerals, and 1200 calories. How many boxes of each cereal should he buy to minimize his cost?
Answers
Let's assume that John buys x boxes of Cereal A and y boxes of Cereal B. Then, we can write the following system of inequalities based on the nutrient and calorie requirements:
10x + 5y ≥ 500 (minimum 500 units of vitamins)
5x + 10y ≥ 600 (minimum 600 units of minerals)
15x + 15y ≥ 1200 (minimum 1200 calories)
We want to minimize the cost, which is given by:
0.5x + 0.4y
This is a linear programming problem, which we can solve using a graphical method. First, we can rewrite the inequalities as equations:
10x + 5y = 500
5x + 10y = 600
15x + 15y = 1200
Then, we can plot these lines on a graph and shade the feasible region (i.e., the region that satisfies all three inequalities). The feasible region is the area below the lines and to the right of the y-axis.
Next, we can calculate the value of the cost function at each corner point of the feasible region:
Corner point A: (20, 40) -> Cost = 20
Corner point B: (40, 25) -> Cost = 25
Corner point C: (60, 0) -> Cost = 30
Therefore, the minimum cost is $20, which occurs when John buys 20 boxes of Cereal A and 40 boxes of Cereal B.
Please help me solve it

Answers
Step-by-step explanation:
D
NO LINKS!!
43. For the following functions, state whether they are growth or decay, then determine each function's growth/decay rate. (NOT MULTIPLE CHOICE)
a. f(x) = 0.3(1.6)^x
b. g(x) = 0.8^0.5x - 7
c. h(x) = 3(-5)^x
d. f(x) = e^(-0.45x) + 4
Answers
Answer:
a) Exponential growth function: 60%
b) Exponential decay function: 20%
c) Neither
d) Exponential decay function: 45%
Step-by-step explanation:
\(\textsf{Exponential Growth}: \quad y=a(1+r)^x\)
\(\textsf{Exponential Decay}: \quad y=a(1-r)^x\)
where:
a = initial value (the amount before measuring growth or decay)r = growth/decay rate (in decimal form)Part aGiven function:
\(f(x) = 0.3(1.6)^x\)
The function is an exponential growth function and the growth rate is:
\(1+r=1.6 \implies r= 0.6 = 60\%\)
Part bGiven function:
\(g(x) = 0.8^{0.5x} - 7\)
The function is an exponential decay function and the decay rate is:
\(1-r=0.8 \implies r=0.2=20\%\)
Part cGiven function:
\(h(x) = 3(-5)^x\)
This is neither an exponential growth or decay function as exponential functions cannot have negative bases.
Part dNatural base exponential function
\(y=ae^{rx}\)
If a > 0 and r > 0, the function is an exponential growth function.
If a > 0 and r < 0, the function is an exponential decay function.
Given function:
\(f(x) = e^{-0.45x} + 4\)
As a = 1 > 0 and r = -0.45 < 0, the function is exponential decay function and the decay rate is:
\(r=-0.45=45\%\)
find the least common multiple (LCM) of 6 and 12.

Answers
Given the numbers 6 and 12, we are asked to find their common multiple. The steps can be seen below;
The Least Common Multiple (LCM) is also referred to as the Lowest Common Multiple (LCM) and Least Common Divisor (LCD). For two integers a and b, denoted LCM(a,b), the LCM is the smallest positive integer that is evenly divisible by both a and b
We will use the listing multiple method to find the answer:
\(\begin{gathered} Multiples\text{ of 6 = 6,12,18,24} \\ Multiples\text{ of 12 = 12, 24, 48} \\ \therefore\text{Multiple of 6 and 12 = 12} \end{gathered}\)Answer: Option B
3. Solve: x + 9 = -5 x + 9 = 45 Check: ✓ Right Hand Side
Answers
Answer:
\(x + 9 = - 5x + 9 = 45 \\ x + 5x = 9 - 9 = 45 \\ 6x = 0 = 45 \\ 6x = 45 \\ x = 7.5\)
this the best I can do
Step-by-step explanation:
Good luck
As a cube what is -1/64 ________ to the power of ^3
Answers
Answer:
(-1/64)= (-1/4)^3
Explanation:
\(\sqrt[3]{-\frac{1}{64} }=-\frac{1}{4}\)
\((-\frac{1}{4})^3=-\frac{1}{64}\)
Andrea did her her homework in 2/3 the amount of time it took Dina to finish hers. Dina took 2 4/5 hours to finish her homework. Which expression shows how long Andrea took to finish her homework?
Answers
Answer:
28/15
Step-by-step explanation:
:)
What is it ????????????????????????

Answers
Answer:
1/8 or 8/64
Step-by-step explanation:
its 1/8 you just times them both by 8 it ez