The members of an equestrian team score from 10 to 25 points per show. Their average score is 19. A new member with an
outlyng score of 4 joins the team.
Which statement describes how the new member's score will affect center and spread of the equestrian team?
The new member's score will decrease the center but increase the spread of the equestrian team.
The new member's score will decrease the center and spread of the equestrian team.
O The new member's score will increase the center and spread of the equestrian team.
O The new member's score will increase the center but decrease the spread of the equestrian team.
Answers
Related Questions
Find the quotient of z₁ by z2. Express your answer in
trigonometric
form.
² - 3 (0 (4) + (*))
Z₁ cos
+/sin
Z₂
²2 = 7 (cos(377)+
COS
8
O A. 7 (cos (577) + i sin (5/77))
8
B.
21(cos(577)+isin (577))
8
OC. 21 cos
21(cos(-7)+ i sin(-77))
O D. 7 (cos(-7) + + sin(-7))
i
+/sin
37T
8

Answers
The quotient of z₁ by z₂ in trigonometric form is:
7/21 * (cos(584°) + i sin(584°))
To find the quotient of z₁ by z₂ in trigonometric form, we'll express both complex numbers in trigonometric form and then divide them.
Let's represent z₁ in trigonometric form as z₁ = r₁(cosθ₁ + isinθ₁), where r₁ is the magnitude of z₁ and θ₁ is the argument of z₁.
We have:
z₁ = 7(cos(577°) + i sin(577°))
Now, let's represent z₂ in trigonometric form as z₂ = r₂(cosθ₂ + isinθ₂), where r₂ is the magnitude of z₂ and θ₂ is the argument of z₂.
From the given information, we have:
z₂ = 21(cos(-7°) + i sin(-77°))
To find the quotient, we divide z₁ by z₂:
z₁ / z₂ = (r₁/r₂) * [cos(θ₁ - θ₂) + i sin(θ₁ - θ₂)]
Substituting the given values, we have:
z₁ / z₂ = (7/21) * [cos(577° - (-7°)) + i sin(577° - (-7°))]
= (7/21) * [cos(584°) + i sin(584°)]
The quotient of z₁ by z₂ in trigonometric form is:
7/21 * (cos(584°) + i sin(584°))
Option C, 21(cos(-7°) + i sin(-77°)), is not the correct answer as it does not represent the quotient of z₁ by z₂.
For more questions on trigonometric form
https://brainly.com/question/31744474
#SPJ8
se va a desarrollar un torneo de ajedrez entre 10 grandes maestros en un sistema round robin ( todos contra todos) .Calcula la cantidad de juegos esperados durante el torneo
Answers
We can expect a total of 45 games during the tournament.
What is round robin scheduling?
Round robin scheduling is a way of sharing CPU time among processes in a fair and cyclic manner.
In a round-robin tournament, each player must play against every other player exactly once. So, if we have n players, then each player will play (n-1) games.
In this case, we have 10 grandmasters, so each one will play 9 games. However, we have to be careful not to count each game twice (i.e., once for each player). So, the total number of games in the tournament is:
10 players × 9 games each ÷ 2 = 45 games
Therefore, we can expect a total of 45 games during the tournament.
Learn more about round robin system on:
https://brainly.com/question/30023475
#SPJ1
The correct question is:
A chess tournament will be developed between 10 grandmasters in a round robin system. Calculate the number of games expected during the tournament.
Simplify the complex question: n/4 / 3/8

Answers
Answer:
\( \frac{2n}{3} \)
hope, I'm right
Answer:
\( \frac{2n}{3} \)
Step-by-step explanation:
\( \frac{ \frac{n}{4} }{ \frac{3}{8} } = \frac{n}{4} \div \frac{3}{8} \)
\( \frac{n}{4} \times \frac{8}{3} = \frac{n \times 8}{4 \times 3} \)
\( \frac{n \times 2}{3} = \frac{2n}{3} \) ✅
The prices of all college textbooks follow a bell-shaped distribution with a mean of $113 and a standard deviation of $12. Using the empirical rule, find the interval that contains the prices of 99.7% of college textbooks.
Answers
Step-by-step explanation:
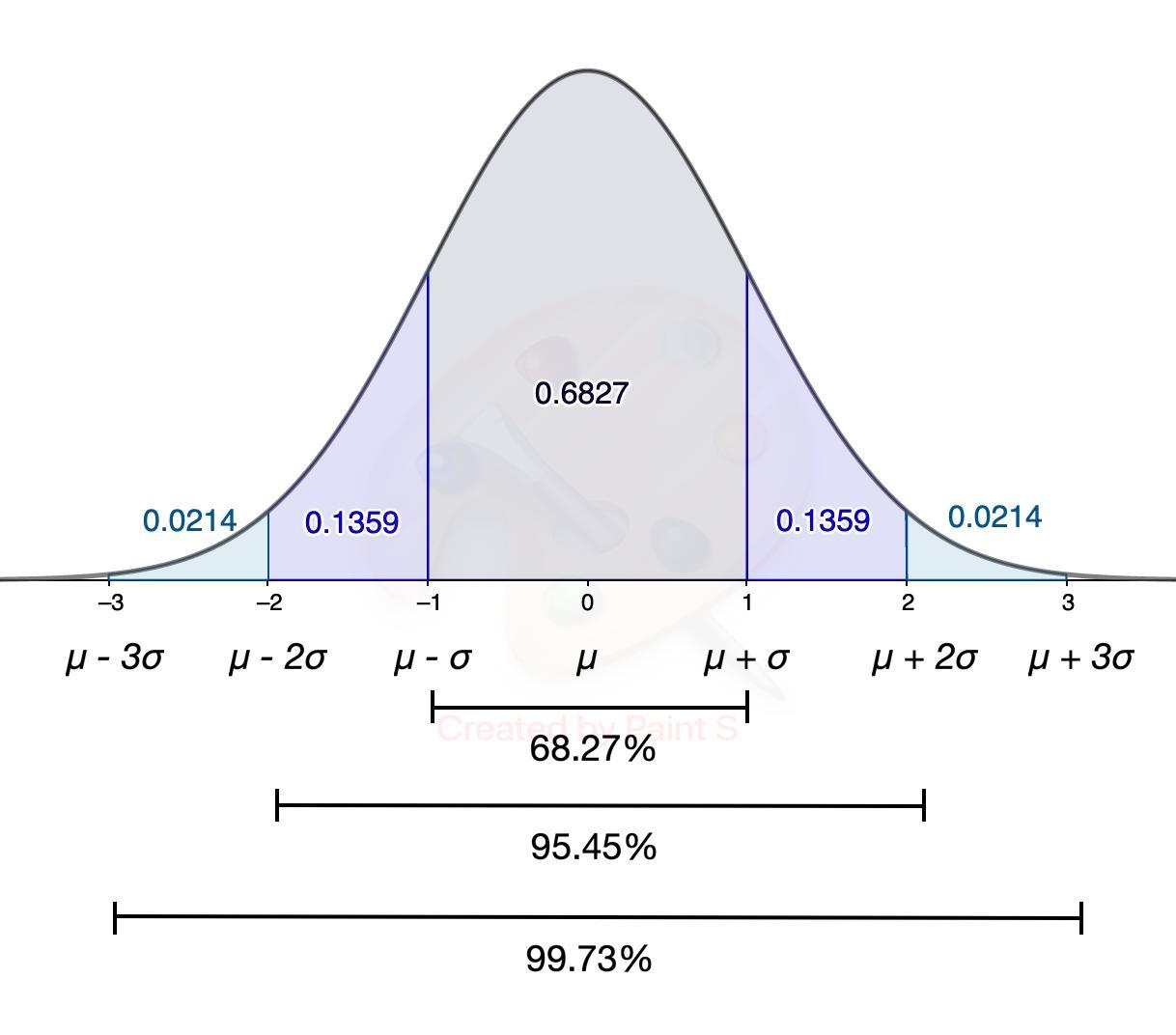
In statistics, the empirical rule states that for a normally distributed random variable,
68.27% of the data lies within one standard deviation of the mean.95.45% of the data lies within two standard deviations of the mean.99.73% of the data lies within three standard deviations of the mean.In mathematical notation, as shown in the figure below (for a standard normal distribution), the empirical rule is described as
\(\Phi(\mu \ - \ \sigma \ \leq X \ \leq \mu \ + \ \sigma) \ = \ 0.6827 \qquad (4 \ \text{s.f.}) \\ \\ \\ \Phi(\mu \ - \ 2\sigma \ \leq X \ \leq \mu \ + \ 2\sigma) \ = \ 0.9545 \qquad (4 \ \text{s.f.}) \\ \\ \\ \Phi}(\mu \ - \ 3\sigma \ \leq X \ \leq \mu \ + \ 3\sigma) \ = \ 0.9973 \qquad (4 \ \text{s.f.})\)
where the symbol \(\Phi\) (the uppercase greek alphabet phi) is the cumulative density function of the normal distribution, \(\mu\) is the mean and \(\sigma\) is the standard deviation of the normal distribution defined as \(N(\mu, \ \sigma)\).
According to the empirical rule stated above, the interval that contains the prices of 99.7% of college textbooks for a normal distribution \(N(113, \ 12)\),
\(\Phi(113 \ - \ 3 \ \times \ 12 \ \leq \ X \ \leq \ 113 \ + \ 3 \ \times \ 12) \ = \ 0.9973 \\ \\ \\ \-\hspace{1.75cm} \Phi(113 \ - \ 36 \ \leq \ X \ \leq \ 113 \ + \ 36) \ = \ 0.9973 \\ \\ \\ \-\hspace{3.95cm} \Phi(77 \ \leq \ X \ \leq \ 149) \ = \ 0.9973\)
Therefore, the price of 99.7% of college textbooks falls inclusively between $77 and $149.
I need a word problem for 4 divided into 1/2 will give bwainliest
Answers
I’ll answer your question, i just wanna know what the equation is
pls help im confused im not smart

Answers
Explain how to estimate the sum of the distances ran in the three months to the nearest kilometer. A 2-column table with 3 rows titled Total Distance Andrea Ran (kilometers). Column 1 has entries March, April, May. Column 2 has entries 48.7, 31.2, 35.66.
Answers
Answer:
round to the nearest kilometer
Step-by-step explanation:
i did this answer and got it right
Answer:
Sample response: If the number in the tenths place is 5 or greater, then round the ones place up. Otherwise, the ones place stays the same.
Step-by-step explanation:
Did it on Edge 2021
find the equation of a line perpendicular to 2x + 2y= -10 that passes through the point (6,2)
Answers
Answer: The equation of a line perpendicular to 2x + 2y = - 10 that passes through the point (6, 2) is y = x - 4
i need help with this, please and thanks. I'll appreciate your help.

Answers
Answer:
the answer is -15 17/34, -15 ,8/20, 15 9/30
Step-by-step explanation:
pllz may i have brainliest
Answer:
-15 17/34, -15, 8/20, & 15 9/30
Step-by-step explanation:
1st: -15 17/34
2nd: -15
3rd: 8/20
4th: 15 9/30
I hope this helps you with your assignment and gl on your assignment! :D
A tank in the shape of a hemisphere has a diameter of 24 feet. If the liquid that fills the tank has a density of 92.5 pounds per cubic foot, what is the total weight of the liquid in the tank, to the nearest full pound?
Answers
The total weight of the liquid in the tank is approximately 12,628 pounds.
To calculate the weight of the liquid, we need to determine the volume of the hemisphere and then multiply it by the density of the liquid. The formula for the volume of a hemisphere is V = (2/3)πr³, where r is the radius of the hemisphere.
In this case, the diameter of the tank is given as 24 feet, so the radius is half of that, which is 12 feet. Plugging this value into the formula, we get V = (2/3)π(12)³ ≈ 904.78 cubic feet.
Finally, we multiply the volume by the density of the liquid: 904.78 cubic feet * 92.5 pounds per cubic foot ≈ 12,628 pounds. Therefore, the total weight of the liquid in the tank is approximately 12,628 pounds.
In summary, to calculate the weight of the liquid in the tank, we first determine the volume of the hemisphere using the formula V = (2/3)πr³. Then, we multiply the volume by the density of the liquid.
By substituting the given diameter of 24 feet and using the appropriate conversions, we find that the total weight of the liquid is approximately 12,628 pounds.
for such more questions on weight
https://brainly.com/question/29892643
#SPJ8
Question 37?Find the indicated function and state its domain in interval notation?

Answers
Given the functions:
\(\begin{gathered} f(x)=-\sqrt[]{x-3} \\ g(x)=3x \end{gathered}\)You need to multiply them, in order to find:
\((f\cdot g)(x)\)Then, you get:
\(\begin{gathered} (f\cdot g)(x)=(-\sqrt[]{x-3})(3x) \\ (f\cdot g)(x)=-3x\sqrt[]{x-3} \end{gathered}\)In order to find the Domain, you need to remember that the Domain of a Radical Function are those input values (x-values) for which the Radicand is positive. Then, in this case, you need to set up that:
\(x-3\ge0\)Now you have to solve for "x":
\(x\ge3\)Therefore:
\(Domain\colon\lbrack3,\infty)\)Hence, the answer is:
\(\begin{gathered} (f\cdot g)(x)=-3x\sqrt[]{x-3} \\ \\ Domain\colon\lbrack3,\infty) \end{gathered}\)Can I please get help plsss

Answers
Answer:
1000 ft/min
Step-by-step explanation:
Rate of change in ft per min is 1000 ft per 1 minute from reading the graph
Enter the equation of a circle that is congruent to circle C and is centered at point P

Answers
The equation of a circle that is congruent to circle C and is centered at point P is (x - 5)² + (y - 1)² = 5².
What is the equation of a circle?In Geometry, the standard form of the equation of a circle is modeled by this mathematical equation;
(x - h)² + (y - k)² = r²
Where:
h and k represent the coordinates at the center of a circle.r represent the radius of a circle.Based on the information provided, we have the following parameters for the equation of this circle:
Center (h, k) of P = (1, 5)Radius (r) of P = 5 units.By substituting the given parameters, we have:
(x - h)² + (y - k)² = r²
(x - 5)² + (y - 1)² = 5²
Read more on equation of a circle here: brainly.com/question/15626679
#SPJ1
Bigco Corporation is one of the nation’s leading distributors of food and related products to restaurants, universities, hotels, and other customers. A simplified version of its recent income statement contained the following items (in millions).
Cost of sales $ 11,571
Income taxes 249
Interest expense 23
Net earnings 1,442
Sales 16,400
Earnings before income taxes 1,691
Selling, general, and administration expense 3,543
Other revenues 428
Total expenses (excluding income taxes) 15,137
Total revenues 16,828
Prepare an income statement for the year ended June 30, current year. (Hint: First order the items as they would appear on the income statement and then confirm the values of the subtotals and totals.)
Answers
Step-by-step explanation:
I hope this answer is helpful ):

The width of a rectangle, in centimeters, is 17 less than 4 times its length, in centimeters. The area
of the rectangle is 15 square centimeters. What is the width, in centimeters, of the rectangle?
Answers
Answer:
Step-by-step explanation:
W = 4L-17
LW = 15
L(4L-17) = 15
4L^2-17L-15= 0
L = [17 ±√(17^2-4(4)(-15))]/[2(4)] = 5,-3/4
-3/4 is an extraneous solution
L = 5 cm
W = 4L-17 = 3 cm
A water bottle has 20 ounces in it. After you drink x ounces of water, there are 8 ounces left. Which equation represents this situation?.
Answers
Answer:
Step-by-step explanation
The bottle had 20 ounces that before and 8 ounces after
8+x=20
20-x=8
20-8=x
20-8=12
Simplify the following expression.

Answers
Answer:
\(\displaystyle \frac{cd^6}{a^4b^2}\)
Step-by-step explanation:
\(\displaystyle \frac{a^{-4}b^{-2}cd^6}{e^{-7}}\\\\=a^{-4}b^{-2}cd^6e^7\\\\=\frac{cd^6}{a^4b^2}\)
Notice that the variables with negative exponent that were originally in the denominator went to the numerator (like with \(e^{-7}\)) and became positive, and vice versa with those originally in the numerator went in the denominator (like with \(a^{-4}b^{-2}\)) and also became positive.
Step-by-step explanation:
a‐⁴b‐²cd⁶
_______
e‐⁷
cd⁶e⁷
_____
a⁴b²
Which problem solving strategy would be best to solve this problem?
Brandon went to the store and spent $2.50 on pencils. He spent half of what he had left on a notebook and paper. When he got home he had $5.00 left. How much money did Brandon have before going to the store?
Answers
Answer: The best problem solving strategy to solve this problem with is a formula. Additionally Brandon had $12.50 before going to the store.
Step-by-step explanation: Formula and Solving it
He Spent $2.50 on pencils.
Amount left after buying a pencil = x - 2.50
Next, he spent half of the amount left in his pocket.
He spent (x - 2.50)/2 on notebook and paper.
So the total amount he spent = 2.50 + (x -2.50)/2
Amount left when he got home =$ 5.00
That means, x - Total amount spent = 5
=> x - (2.50 + (x -2.50)/2 ) = 5
=> x - 2.50 -x/2 + 2.50/2 = 5
=> x/2 - 2.50/2 = 5
=> x/2 = 5 + 2.50/2
=> x/2 = 6.25
=>x = $12.5
Saved
250 mg
sing value in
50 mg
10 ml
X
Choice
Answers
What is the question and then i can help!
If a = 5 units , b = 8 C = 4 Units, and d = 3 units what is the volume of the composite figure?
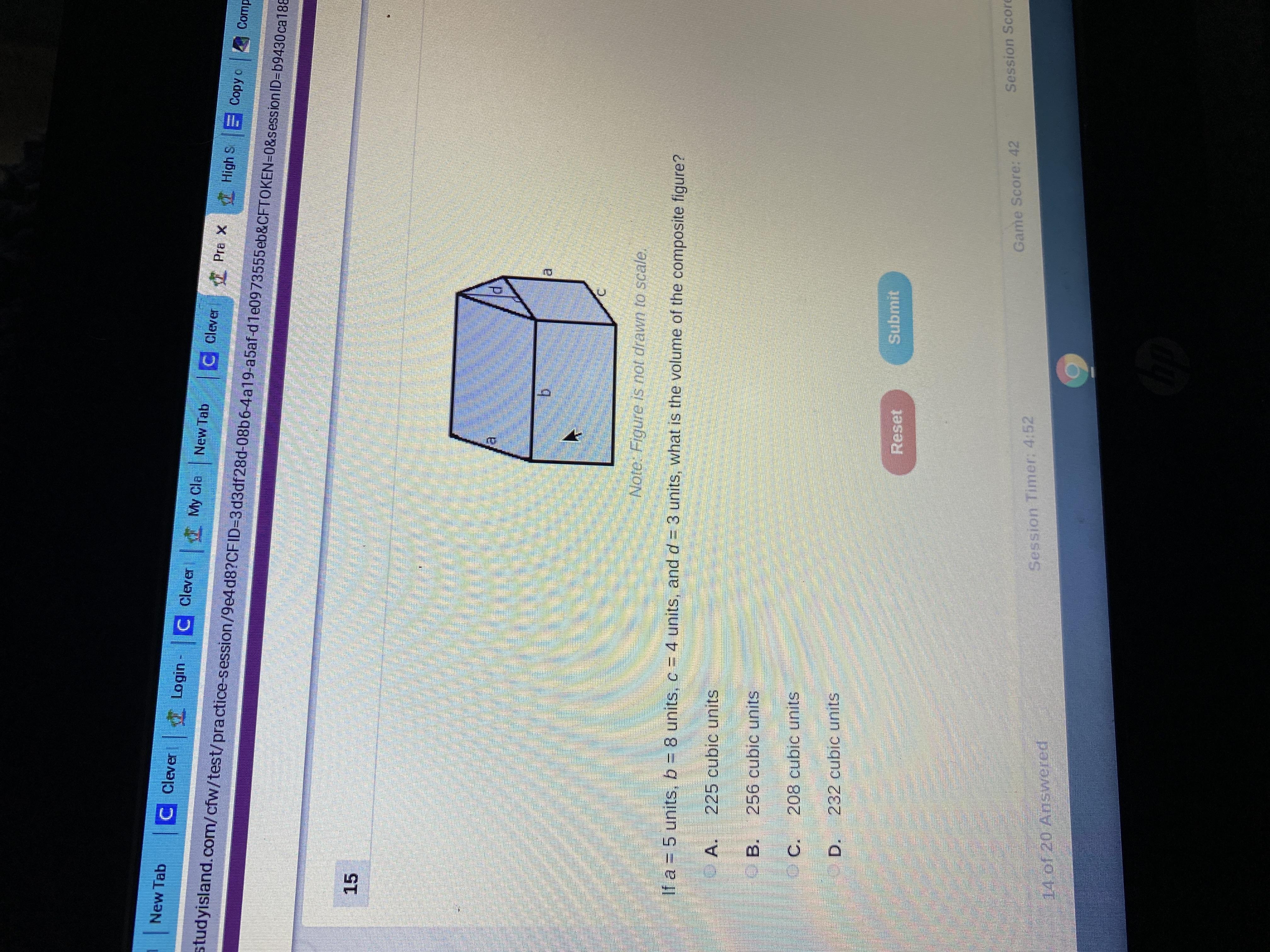
Answers
Answer:
\(V = 208\,u^{3}\) (Option C)
Step-by-step explanation:
The volume of the composite figure is:
\(V = \frac{1}{2}\cdot (3\,u)\cdot (4\,u)\cdot (8\,u) + (5\,u)\cdot (4\,u)\cdot (8\,u)\)
\(V = 208\,u^{3}\) (Option C)
Please help fast!!!!!!!

Answers
Answer: 156.38 m
Step-by-step explanation:
Using the arc length formula, the answer is \(2\pi(8^2) \cdot \frac{140}{360} \approx 156.38\)
Solve the inequality 3x + 4 < 22

Answers
Answer:
3х<18
х<6
хє(-infinity ;6);
Answer:
x<6
Step-by-step explanation:
3x+4<22
First, subtract 4 from both sides of the inequality.
3x-4<22-4
This cancels out the 4, so the 3x is isolated
3x/3<18/3
Finally, simplify to get the answer.
x<6
please mark as brainliest!!
You can use the fact that is irrational to answer the questions below. You can also use other facts proven within this exercise. (a) Prove that is irrational. (b) Prove that is irrational. (c) Is it true that the sum of two positive irrational numbers is also irrational
Answers
Answer:
See Explanation for proofs
Step-by-step explanation:
Given
See attachment for complete question
Solving (a):
\(\frac{\sqrt{2}}{2}\) is irrational
\(\sqrt{2} =1.41421356237.....\)
So, we have:
\(\frac{\sqrt{2}}{2} = \frac{1.41421356237.....}{2}\)
\(\frac{\sqrt{2}}{2} = 0.70710678118....\)
\(0.70710678118....\) can not be represented as a fraction of whole numbers.
Hence:
\(\frac{\sqrt{2}}{2}\) is irrational
Solving (b):
\(\sqrt{2} -2\) is irrational
\(\sqrt{2} =1.41421356237.....\)
So, we have:
\(\sqrt{2} -2 = 1.41421356237..... - 2\)
\(\sqrt{2} -2 = -0.58578643763.....\)
\(-0.58578643763.....\) can not be represented as a fraction of whole numbers.
Hence:
\(\sqrt{2} -2\) is irrational
Solving (c):
The sum of two positive irrational number is always irrational
Proof Below
If a is irrational and b is also irrational,
then
\(a + b = irrational\)
Take for instance:
\(a = \sqrt{2\)
\(b = \sqrt{8\)
\(a + b = \sqrt{2} + \sqrt{8\)
\(a + b = \sqrt{2} + \sqrt{4*2\)
\(a + b = \sqrt{2} + \sqrt{4}*\sqrt{2\)
\(a + b = \sqrt{2} + 2\sqrt{2\)
\(a + b = 3\sqrt{2\)
\(3\sqrt{2\) is irrational and this is true for the sum of every positive irrational numbers

Find the values of w and x that makeup NOPQ a parallelogram. A. W = 1/2 X = 1/2 B. W = 3 X = 2 C. W = 2 X = 2 D. W = 3 X = 1/2 Please select the best answer from the choices Provided

Answers
Solution
Step 1:
Properties of Parallelograms Explained
1. Opposite sides are parallel. ...
2. Opposite sides are congruent. ...
3. Opposite angles are congruent. ...
4. Same-Side interior angles (consecutive angles) are supplementary. ...
5. Each diagonal of a parallelogram separates it into two congruent triangles. ...
6. The diagonals of a parallelogram bisect each other.
Step 2:
The diagonals of a parallelogram bisect each other.
\(\begin{gathered} The\text{ }diagonals\text{ }of\text{ }a\text{ }parallelogram\text{ }bisect\text{ }each\text{ }other. \\ w\text{ + 7 = 5w - 5} \\ and \\ \frac{3}{2x}\text{ = 3} \end{gathered}\)Step 3
\(\begin{gathered} Solve\text{ for w:} \\ w\text{ + 7 = 5w - 5} \\ Add\text{ similar terms} \\ 7\text{ + 5 = 5w - w} \\ 12\text{ = 4w} \\ w\text{ = }\frac{12}{4} \\ w\text{ = 3} \end{gathered}\)Step 4
\(\begin{gathered} Solve\text{ for x:} \\ \frac{3}{2x}\text{ = 3} \\ 3\times2x\text{ = 3} \\ \\ 6x\text{ = 3} \\ \\ x\text{ = }\frac{3}{6} \\ \\ x\text{ = }\frac{1}{2} \end{gathered}\)Final answer
\(\text{w = 3 . x = }\frac{1}{2}\)Last season, two running backs on the Steelers
football team rushed for a combined total of
1550 yards. One running back ran for 450 yards
more than the other. How many yards did they
each run?
Answers
Answer:
Step-by-step explanation:
Last season two running backs on the Steelers football team rushed a combined total of 1550 yards. One rushed 4 times as many yards as the other. Let x and y represent the number of yards each individual player rushed. this is the equation that was used
x + y = 1550
y = 4x
What is the volume 4ft by 4ft by 8ft
Answers
Step-by-step explanation:
Volume is = 4ft × 4ft × 8ft
= 128 cubic feet
let a and be positive integers satisfying (ab+1)/(a+b) < 3/2. The maximum possible value of (a^3b^3+1)/(a^3+b^3) is p/q. where p and q are relatively prime positive integers. Find p+q.
Answers
p/q = (2x^3+1)/2x^3, which implies that p = 2x^3+1 and q = 2x^3. p + q = 2x^3+1 + 2x^3 = 3x^3.
Let a and b be positive integers satisfying (ab+1)/(a+b) < 3/2. The maximum possible value of (a^3b^3+1)/(a^3+b^3) is given by the equation p/q, where p and q are relatively prime positive integers. We can expand the numerator and denominator as follows: a^3b^3+1 = a^3b^3 + a^3 + b^3 and a^3+b^3 = a^3 + a^2b + ab^2 + b^3. Therefore, the expression is equal to (a^2b^2+a^2+ab+1)/(a^2b+ab^2+1). Since (ab+1)/(a+b) < 3/2, we have (ab+1)/(a+b) < 5/3 and (a^2b^2+a^2+ab+1)/(a^2b+ab^2+1) < 5/3.To find the sum of p and q, we can use the fact that the maximum value occurs when a and b are equal. Thus, setting a = b = x, we have (x^3x^3+1)/(x^3+x^3) = (x^6+1)/2x^3 = (2x^3+1)/2x^3. Thus, p/q = (2x^3+1)/2x^3, which implies that p = 2x^3+1 and q = 2x^3. Therefore, p + q = 2x^3+1 + 2x^3 = 3x^3.
Learn more about positive integers here:
https://brainly.com/question/13733829
#SPJ4
Is 9pi rational or irrational
Answers
Answer:
irrational
Step-by-step explanation:
every number that times with pi are irrational, only zero gives a rational number
Find the slope of the hill.
please help
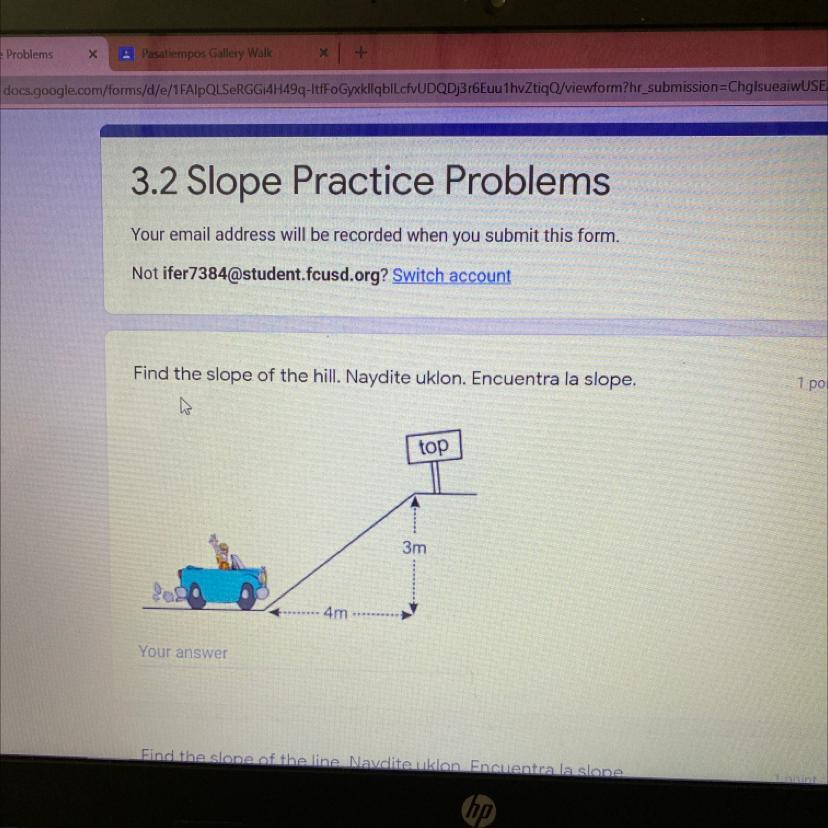
Answers
Answer: slope = 3/4
Step-by-step explanation: 3 up and 4 to the right so the fraction(slope) is 3/4
Find the area of the rectangle shown
7 1/2 ft
5 1/4ft

Answers
Answer:
39 3/8 ft ^2
Step-by-step explanation:
To find the area of a rectangle, multiply the length times the width.
A = l*w
= 7 1/2 * 5 1/4
Change the mixed numbers to improper fractions.
A = 15/2 * 21/4
=315/8
Change back to a mixed number.
8 goes into 315 39 times with 3 left over.
= 39 3/8
Answer:
Area = 39.375 ft²
(you can round to 39.4)
Step-by-step explanation:
Find the area of the rectangle shown
7 1/2 ft
5 1/4ft
7 1/2 = 7.5 ft
5 1/4 = 5.25 ft
Area = L x W
Area = 7.5 x 5.25
Area = 39.375