There are TWO answers needed for this question John rode 2 kilometers on his bike. His sister Sally rode 300 meters on her bike. Who rode the farthest and how much farther did they ride (answer in km)?
Answers
John rode 1.7 kilometers farther than Sally. In conclusion, John rode the farthest, 1.7 kilometers farther than Sally
To answer this question, we first need to convert Sally's distance from meters to kilometers as we have to give the answer in km.
1 kilometer = 1000 meters
Therefore, Sally's distance in kilometers would be:
300 meters ÷ 1000 meters/kilometer
= 0.3 kilometers
Now, to find out who rode the farthest, we need to compare their distances.
John rode 2 kilometers while Sally rode 0.3 kilometers.
Therefore, John rode farther than Sally. The difference in their distances would be:
2 kilometers - 0.3 kilometers= 1.7 kilometers
Therefore, John rode 1.7 kilometers farther than Sally. In conclusion, John rode the farthest, 1.7 kilometers farther than Sally
To know more about kilometers visit:
https://brainly.com/question/21334478
#SPJ11
Related Questions
please help answer ill give brainlest and all 5 stars

Answers
10x=80
Solution
X=8
Answer:
Let the speed of the boat in still water be x mph. As, the speed of stream is 5 mph, upstream speed will be (x−5) and downstream speed will be (x+5). and time taken by boat for travelling 13 miles downstream is 13/(x+5). The speed of boat in still water be 8 mph
Step-by-step explanation:
PLEASE HELP ME (again) PLEASE AND THANK YOU!!

Answers
Explanation:
Slope formula: slope(m) = y2 - y1 / x2 - x1
(-6=x1, 7=y1) (0=x2, 3=y2)
Rectangles J and K are similar. If the area of rectangle J is 440, what is the area of rectangle K? I need this today
Answers
Answer:
\(Area = 27.5\)
Step-by-step explanation:
Given
\(J_{Area} = 440\)
\(J_{Width} = 22\)
\(K_{Width} = 5.5\)
See attachment
Required
Determine the area of K
First, we need to calculate the length of the rectangle J
\(J_{Length} * J_{Width} = J_{Area}\)
This gives:
\(J_{Length} * 22 = 440\)
Divide both sides by 22
\(\frac{J_{Length} * 22}{22} = \frac{440}{22}\)
\(J_{Length} = 20\)
So, the length of the rectangle J is 20.
Since both shapes are similar, then:
\(J_{Length} : J_{Width} = K_{Length} : `K_{Width}\)
Substitute the known values:
\(20 : 22 = K_{Length} : `5.5\)
Express as fraction:
\(\frac{20 }{ 22 }= \frac{K_{Length} }{ `5.5}\)
Make Length, the subject of formula
\(K_{Length} = \frac{5.5 * 20}{22}\)
\(K_{Length} = \frac{110}{22}\)
\(K_{Length} = 5\)
The area of K is:
\(Area = K_{Length} * K_{Width\)
\(Area = 5.5 * 5\)
\(Area = 27.5\)

i’ll mark you brainliest

Answers
Answer:
thanks and it is 25
there are 4 prime numbers in the spinner
A 100% = 4
? = what about
100×1/4
= 25%
What is (1/243) ^-x/3 = 4
Answers
Using the log, the answer to (1/243) ^-x/3 = 4 is \(-x=\log _{\frac{1}{243}}(12)\).
What is a logarithm?The power to which a number must be increased in order to obtain another number is known as a logarithm.
For instance, the logarithm of 100 in base ten is 2, since ten multiplied by two equals 100: log 100 = 2, since 102 = 100.
So, solve using a log as follows: (1/243) ^-x/3 = 4
\(\begin{aligned}& \frac{\left(\frac{1}{243}\right)^{-x}}{3}=4 \\& 3 \cdot \frac{\left(\frac{1}{243}\right)^{-x}}{3}=3 \cdot 4\end{aligned}\)
\(\begin{aligned}& 3 \cdot \frac{\left(\frac{1}{243}\right)^{-x}}{3}=3 \cdot 4 \\& \left(\frac{1}{243}\right)^{-x}=3 \cdot 4\end{aligned}\)
\(\begin{aligned}& \left(\frac{1}{243}\right)^{-x}=3 \cdot 4 \\& \left(\frac{1}{243}\right)^{-x}=12\end{aligned}\)
\(\begin{aligned}& \left(\frac{1}{243}\right)^{-x}=12 \\& -x=\log _{\frac{1}{243}}(12) \\& \frac{\left(\frac{1}{243}\right)^{-x}}{3}=4 \\& -x=\log _{\frac{1}{243}}(12)\end{aligned}\)
Therefore, using the log, the answer to (1/243) ^-x/3 = 4 is \(-x=\log _{\frac{1}{243}}(12)\).
Know more about logarithms here:
https://brainly.com/question/25710806
#SPJ1
2x^3y + 18xy - 10x^2y - 90y
Part A: rewrite the expression so that the GCF is factored completely
Part B: rewrite the expression completely factored. Show the steps of your work
___________________________
Part A: the area of a square is (9x^2 + 24x + 16) square units. Determine the length of each side of the square by factoring the area expression completely. Show your work.
Part B: the area of a rectangle is (16x^2 - 25y^2) square units. Determine the dimensions of the rectangle by factoring the area expression completely. Show your work.
___________________________
f(x) = 2x^2 - 5x + 3
Part A: what are the x-intercepts of the graph of f(x)? Show your work
Part B: is the vertex of the graph of f(x) going to be a maximum or minimum? What are the coordinates of the vertex? Justify your answer and show your work.
Part C: what are the steps you would use to graph f(x)? Justify that you can use the answer in part A and part B to draw the graph.
Answers
The expression where the greatest common factor (GCF) is factored completely is \(2x^3y + 18xy - 10x^2y - 90y = 2y(x^3 + 9x - 5x^2 - 45)\)
The expression completely factored in is
\(2y(x^3 + 9x - 5x^2 - 45) = 2y[x(x^2 - 5) + 9(x^2 - 5)]\\= 2y(x - 5)(x^2 + 9)\)
Please refer below for the remaining answers.
We have,
Part A:
To rewrite the expression 2x³y + 18xy - 10x²y - 90y so that the greatest common factor (GCF) is factored completely, we can factor out the common terms.
GCF: 2y
\(2x^3y + 18xy - 10x^2y - 90y = 2y(x^3 + 9x - 5x^2 - 45)\)
Part B:
To completely factor the expression, we can further factor the quadratic term.
\(2y(x^3 + 9x - 5x^2 - 45) = 2y[x(x^2 - 5) + 9(x^2 - 5)]\\= 2y(x - 5)(x^2 + 9)\)
Now,
Part A:
To determine the length of each side of the square given the area expression (9x² + 24x + 16), we need to factor it completely.
The area expression (9x² + 24x + 16) can be factored as (3x + 4)(3x + 4) or (3x + 4)².
Therefore, the length of each side of the square is 3x + 4.
Part B:
To determine the dimensions of the rectangle given the area expression (16x² - 25y²), we need to factor it completely.
The area expression (16x² - 25y²) is a difference of squares and can be factored as (4x - 5y)(4x + 5y).
Therefore, the dimensions of the rectangle are (4x - 5y) and (4x + 5y).
Now,
f(x) = 2x² - 5x + 3
Part A:
To find the x-intercepts of the graph of f(x), we set f(x) equal to zero and solve for x.
2x² - 5x + 3 = 0
The quadratic equation can be factored as (2x - 1)(x - 3) = 0.
Setting each factor equal to zero:
2x - 1 = 0 --> x = 1/2
x - 3 = 0 --> x = 3
Therefore, the x-intercepts of the graph of f(x) are x = 1/2 and x = 3.
Part B:
To determine if the vertex of the graph of f(x) is maximum or minimum, we can examine the coefficient of the x^2 term.
The coefficient of the x² term in f(x) is positive (2x²), indicating that the parabola opens upward and the vertex is a minimum.
To find the coordinates of the vertex, we can use the formula x = -b / (2a), where a and b are the coefficients of the quadratic equation.
For f(x),
a = 2 and b = -5.
x = -(-5) / (2 x 2) = 5/4
To find the corresponding y-coordinate, we substitute this x-value back into the equation f(x):
f(5/4) = 25/8 - 25/4 + 3 = 25/8 - 50/8 + 24/8 = -1/8
Therefore, the vertex of the graph of f(x) is at the coordinates (5/4, -1/8), and it is a minimum point.
Part C:
To graph f(x), we can start by plotting the x-intercepts, which we found to be x = 1/2 and x = 3.
These points represent where the graph intersects the x-axis.
Next,
We can plot the vertex at (5/4, -1/8), which represents the minimum point of the graph.
Since the coefficient of the x² term is positive, the parabola opens upward.
We can use the vertex and the symmetry of the parabola to draw the rest of the graph.
The parabola will be symmetric with respect to the line x = 5/4.
We can also plot additional points by substituting other x-values into the equation f(x) = 2x² - 5x + 3.
By connecting the plotted points, we can draw the graph of f(x).
The steps to graph f(x) involve plotting the x-intercepts, the vertex, and additional points, and then connecting them to form the parabolic curve.
The answer in part A (x-intercepts) and part B (vertex) are crucial in determining these key points on the graph.
Thus,
The expression where the greatest common factor (GCF) is factored completely is \(2x^3y + 18xy - 10x^2y - 90y = 2y(x^3 + 9x - 5x^2 - 45)\)
The expression completely factored in is
\(2y(x^3 + 9x - 5x^2 - 45) = 2y[x(x^2 - 5) + 9(x^2 - 5)]\\= 2y(x - 5)(x^2 + 9)\)
Learn more about expressions here:
https://brainly.com/question/3118662
#SPJ1
Baker Hazel is making several cakes for a big party. She uses 1 cup of butter
for each cake she makes. She has a big tub that has six cups of butter in it. She
needs to save 1 cups of butter to make frosting later. How many cakes can
she make using the butter she has left?
Answers
Answer:
5
Step-by-step explanation:
1 cup for 1 cake
cups of butter=6-1=5
5 butter left therfore. 5 cakes
Step-by-step explanation:
ans : 3
total cup of butter = 6 cups
1 cup of butter for each cake + 1 cup of butter to make frosting
so , she can make 3 of them
twice a number is 27 more than half the number. what is the number
Answers
Answer:
18
Step-by-step explanation:
trust me
On a coordinate plane, a v-shaped line crosses the x-axis at (negative 2, 0), the y-axis at (0, negative 2), and the x-axis at (2, 0). What is the domain of the function on the graph? all real numbers all real numbers greater than or equal to –2 all real numbers greater than or equal to –5 all real numbers greater than or equal to 0
Answers
Answer:
Domain of the given function is all real numbers i.e. R.
Step-by-step explanation:
It is given that the given graph is a V shaped graph.
It is crossing x - axis at \((-2,0)\)
then y-axis at \((0,-2)\) and
then again x - axis at \((2,0)\).
If we try to plot the graph, the graph will be like it comes down from the negative x axis side and then intersect x axis at \((-2,0)\), then intersects y axis at \((0,-2)\) and then rises towards the right side (i.e. positive x axis) intersecting the positive x axis at \((2,0)\).
Please find attached graph for the same.
This graph denotes the following function:
\(y=f(x)=|x|-2\)
Domain of a function \(y =f(x)\) is the values of \(x\) that can be provided to the function.
Here function is a modulus function and from graph we can easily infer that it can have any Real Number, R as its input.
Modulus function has R as its domain.
So, the answer is:
Domain of given function is all Real Numbers, R

Answer:
A on edg. all real numbers
Step-by-step explanation:
i need just the answer please!

Answers
Answer:
Step-by-step explanation:
According to Triangle Property: Angle A + Anlge B + Angle C = 180°
Solution:
1. ∠A = 119° ( ∵ ∠A > 90°. It is an Obtuse Angle )
2. ∠B = 38° ( ∵ ∠B < 90°. It is an Acute Angle )
3. ∠C = 23° ( ∵ ∠C < 90° . It is an Acute Angle
∴ The triangle is an Obtuse Triangle as it consists of an Obtuse
Angle.
The price of a Nintendo switch was reduced from $200 to $50. By what percentages was the price of the Nintendo switch reduced?
Answers
Answer:
1\4 of the price
Step-by-step explanation:
200 -50 =150-50=100-50=50-50=0 you can do it 5 times so its reduced by 1\4
Answer:
25% off
Step-by-step explanation:
simplify the expression (a^3 over 2)^3

Answers
Step-by-step explanation:
(a3/2)3
(a3)3/(2)3
a9/8
Hope it is helpful for you
morgan is walking her dog on an 8-meter-long leash. she is currently 500 meters from her house, so the maximum and minimum distances that the dog may be from the house can be found using the equation |x – 500|
Answers
The maximum distance the dog can be from the house is 8 meters and the minimum distance the dog can be from the house is also 8 meters on the basis of the equation |x - 500|.
The equation |x - 500| can be used to find the maximum and minimum distances that Morgan's dog may be from her house while walking on an 8-meter-long leash.
The equation |x - 500| represents the absolute value of the difference between the dog's distance from the house (x) and 500 meters. The absolute value ensures that the result is always positive.
To find the maximum distance, we need to consider the scenario where the dog is as far away from the house as possible. In this case, the dog would be at the end of the 8-meter-long leash, which means x would equal 500 + 8 = 508 meters. Plugging this value into the equation gives us |508 - 500| = 8 meters which is the maximum distance.
To find the minimum distance, we need to consider the scenario where the dog is as close to the house as possible. In this case, the dog would be at the other end of the leash, which means x would equal 500 - 8 = 492 meters. Plugging this value into the equation gives us |492 - 500| = 8 meters which is maximum distance.
In summary, the maximum and minimum distances that the dog may be from the house while walking on an 8-meter-long leash are both 8 meters.
Learn more about the modulus equation here:
https://brainly.com/question/27158448
#SPJ11
given circle o what is the values of x and y

Answers
Three-quarters of a pound of candy cost 66 cents. Find the cost of one pound
of candy.
Answers
Answer:
$0.88 per pound
Step-by-step explanation:
you want to divide the 3/4 pounds by 3, and when you do that, you also have to divide the 66 cents by 3 as well, then multiply the 1/4 you get from dividing 3/4 by 3, and multiply it by 4 to get 1 pound, but when you do that, you have to do the same thing with the 22 cents you get from dividing 66 cents by 3, which will give you 88 cents per pound
I need help with this maths question!

Answers
14 people gave the modal response
How many people gave the modal response?In a survey, modal response is the most frequently occurring response to a question. It can be used to identify the most important issues or concerns of a population.
You will notice the key says one circle represents 4 people. So half circle represents 2 people. Thus, we can say:
Swedish = 4 + 4 + 2 = 10
German = 4 + 4 = 8
French = 4 + 4 + 4 + 2 = 14
Since French has the highest number of people (i.e. 14 people). Therefore, 14 people gave the modal response.
Learn more about modal response on:
https://brainly.com/question/14532771
#SPJ1
can someone help me with this

Answers
Answer:
29
Step-by-step explanation:
Ian has $6,000.00 to invest for 2 years. The table shows information about two investments Ian can make.
Ian makes no additional deposits or withdrawals. Which investment earns the greater amount of interest over a period of 2 years?

Answers
Investment X earns the greater amount of interest over a period of 2 years.
What is simple interest?Simple interest is a method of calculating interest on an amount for n period of time with a rate of interest of r. It is calculated with the help of the formula,
SI = PRT
where SI is the simple interest, P is the principal amount, R is the rate of interest, and T is the time period.
Let's consider that Ian invests in X, then:
Principle amount, P = $6,000
Time, T = 2 Years
Rate of Interest, R = 4.5% at simple Interest = 0.045
The interest earned is:
Interest = PRT = $6,000 × 0.045 × 2 = $540
Now, consider that Ian invests in Y, then:
Principle amount, P = $6,000
Time, n = 2 Years
Rate of Interest, R = 4% at Compound Interest = 0.04
The interest earned is:
Interest = P(1+R)ⁿ - P
= $6,000(1+0.04)² - $6,000
= $489.6
Since $540>$489.6, therefore, Investment X earns the greater amount of interest over a period of 2 years.
Learn more about Simple Interest here:
https://brainly.com/question/2793278
#SPJ1
Please answer either or all of them and please show me how you solve it

Answers
Answer:
Step-by-step explanation:
using pythagoras theorem
a^2 + b^2 = c^2
4^2 + y^2 = 21^2
16 + y^2 = 441
y^2 = 441 - 16
y^2 = 425
\(y = \sqrt{425}\)
\(y = 5\sqrt{17}\)
y = 13.2
a^2 + b^2 = c^2
4^2 + x^2 = 7^2
16 + x^2 = 49
x^2 = 40 - 16
x^2 = 33
x = \(\sqrt{33}\)
x = 5.7 m
What size is 20cm in inches?
Answers
Answer:
7.87402 C:
Step-by-step explanation:
Very easy question - please answer fast

Answers
Answer:
d or c
Step-by-step explanation:
Answer:
2/5
Step-by-step explanation:
Since the formula for grid's are y by x Y=2 X=5 put them as a fraction there you have 2/5 as I think it is
Based on the data, which of the following statements must be true?


Answers
Answer:
hope this help
Step-by-step explanation:
here is the answer

A train travels at a constant speed during a portion of its cross county route the table shows the distance the train traveled over different intervals of time at this speed.
what is the Speed of the train in Miles per hour?
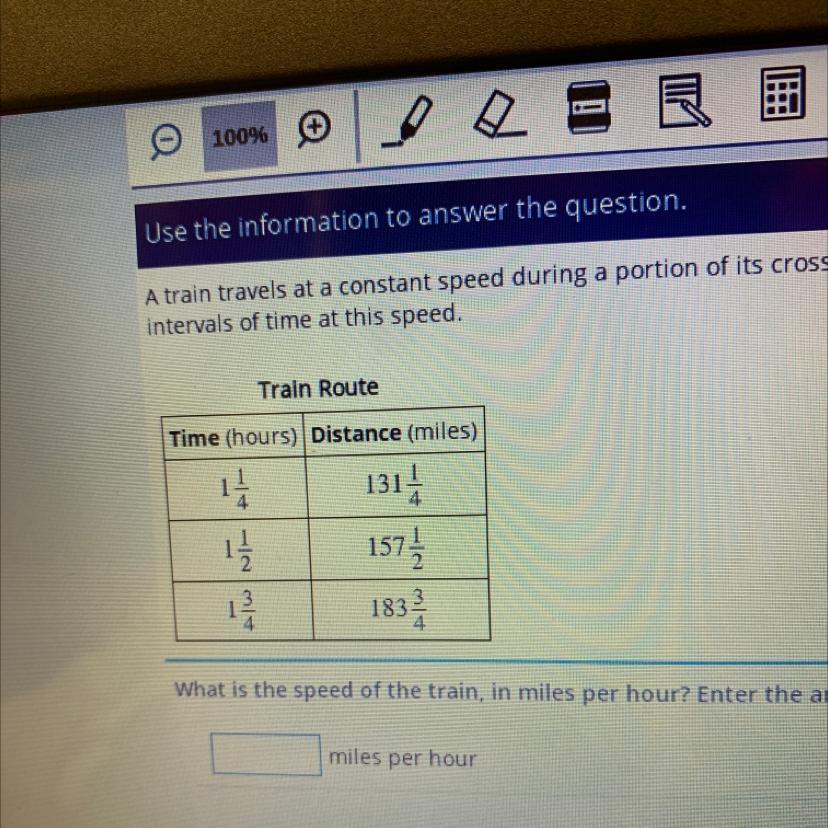
Answers
The Speed of the train in Miles per hour will be 105 miles per hour.
What is a linear equation?A connection between a number of variables results in a linear model when a graph is displayed. The variable will have a degree of one.
The slope of the line is given as,
m = (y₂ - y₁) / (x₂ - x₁)
A train travels at a constant speed during a portion of its cross-country route the table shows the distance the train traveled over different intervals of time at this speed.
The slope represents the speed of the train. Then we have
m = (157.5 - 131.25) / (1.50 - 1.25)
m = 26.25 / 0.25
m = 105 miles per hour
The Speed of the train in Miles per hour will be 105 miles per hour.
More about the linear equation link is given below.
https://brainly.com/question/11897796
#SPJ1
a test to see whether two variables, measured at the same point in time, are correlated is known as a)cross-sectional correlations b)longtitudal design c)descriptive statistics d)divergence
Answers
Answer:
a)cross-sectional correlation
Step-by-step explanation:
a test to see whether two variables, measured at the same point in time, are correlated is known as a cross-sectional correlation test
A longitudinal study is a research design that involves repeated observations of the same variables over long periods of time
and so on
Find m∠1. Then classify the triangle by its angles

Answers
9514 1404 393
Answer:
∠1 = 60°equilateral triangleStep-by-step explanation:
The sum of angles in a triangle is 180°, so we have ...
60° +60° +∠1 = 180°
∠1 = 180° -120° = 60°
The measure of angle 1 is 60°.
All of the angles are the same, so this is an equiangular triangle, better known as an equilateral triangle.
5k + 2 = 6
What is this
Answers
I really need help I don’t understand how to do this

Answers
Answer:
Step-by-step explanation:
Remark
The way this is worded, it sounds like it is a horizontal distance. However by the marking it looks like they want the hypotenuse. Life can be very confusing sometimes.
The angle of the triangle that you need to know is the one on the right. The line of sight line is parallel to the base of the triangle. So the angle on the right is 8 degrees by the Z theorem.
Solution
Sin(8) = 1817 / x Multiply both sides by x
x*sin(8) = 1817 Divide by sin(8)
x = 1817/sin(8)
sin(8) = 0.1392
x = 1817/0.1392
x = 13055.7
Note
Watch how you do this. I would put it into your calculator as
1817
÷
sin(8)
=
If you use the rounded answer I gave for sin(8), you might get it wrong. This is a big distance and you should just use the exact number you get for sin(8).
A study on students drinking habits wants to determine the true average number of alcoholic drinks all UF "greek" students have in a one week! period. We know from preliminary studies that the standard deviation is around 6.3. How many students should be sampled to be within 0.5 drink! of population mean with 95% probability? 609 *305 304 610
Answers
Number of students should be sampled to be within 0.5 drink of population mean with 95% probability is 617 students.
To determine the sample size required to estimate the population mean with a given level of precision, we can use the formula for the margin of error
Margin of error = Z × (standard deviation / sqrt(sample size))
where Z is the critical value of the standard normal distribution corresponding to the desired level of confidence. For a 95% confidence level, Z is 1.96.
We want the margin of error to be no more than 0.5 drinks, so we can set up the equation
0.5 = 1.96 × (6.3 / sqrt(sample size))
Solving for the sample size, we get
sqrt(sample size) = 1.96 × 6.3 / 0.5
sqrt(sample size) = 24.82
sample size = (24.82)^2
sample size = 617
Learn more about margin of error here
brainly.com/question/10501147
#SPJ4
Express the ratio below in its simplest form.
4:2:2
Answers
Answer:
2: 1 : 1
Step-by-step explanation:
4:2:2
Divide all sides by 2
4/2:2/2:2/2
2: 1 : 1
Simon bought a new laptop for $800 during the cyber monday sale. He paid $200 up front and will pay $60 a month until the balance is paid off. Fin the number of months ut will take simon to pay off his laptop?
Answers
Answer:
7 monthsStep-by-step explanation:
Step one:
given data
the cost of the laptop= $800
initial payment= $200
monthly payment = $60
let the number of months be x
the expression for the situation is the function for the equation of line
y=mx+c
Step two:
the expression for the situation is
800=200+60x
solve for x
800-200=60x
400=60x
divide both sides by 60
x=400/60
x=6.7
Approximately 7 months