Two cheeseburgers and one small order of fries contain a total of 1400 calories. Three cheeseburgers and two small orders of fries contain a total of 2260 calories. Find the caloric content of each item.
Answers
Let the calories of a cheeseburger be C, and the calories of a small order of fries be F. Using this notation: Two cheeseburgers and one small order of fries contain a total of 1400 calories. Calories in 2 cheeseburgers + Calories in 1 small order of fries = 14002C + F = 1400. Three cheeseburgers and two small orders of fries contain a total of 2260 calories. Calories in 3 cheeseburgers + Calories in 2 small orders of fries = 22603C + 2F = 2260. We can solve for C and F by solving these two equations for C and F using the method of elimination.
Let's double the first equation and subtract the second equation: 4C + 2F = 2800 -(3C + 2F = 2260). 1C = 540 C = 540. Calories in a cheeseburger = C = 540. Substituting this value of C into either of the two equations and solving for F gives us:2C + F = 14002(540) + F = 1400. F = 320. Calories in a small order of fries = F = 320. Therefore, two cheeseburgers contain 2C = 2(540) = 1080 calories, and one small order of fries contains F = 320 calories. Three cheeseburgers contain 3C = 3(540) = 1620 calories, and two small orders of fries contain 2F = 2(320) = 640 calories.
Answer: Calories in a cheeseburger = C = 540Calories in a small order of fries = F = 320. Calories in two cheeseburgers = 2C = 2(540) = 1080. Calories in three cheeseburgers = 3C = 3(540) = 1620. Calories in one small order of fries = F = 320Calories in two small orders of fries = 2F = 2(320) = 640.
To know more about elimination method, click here:
https://brainly.com/question/13877817
#SPJ11
Related Questions
solve for T. pls help this is literally the last one i need to finish
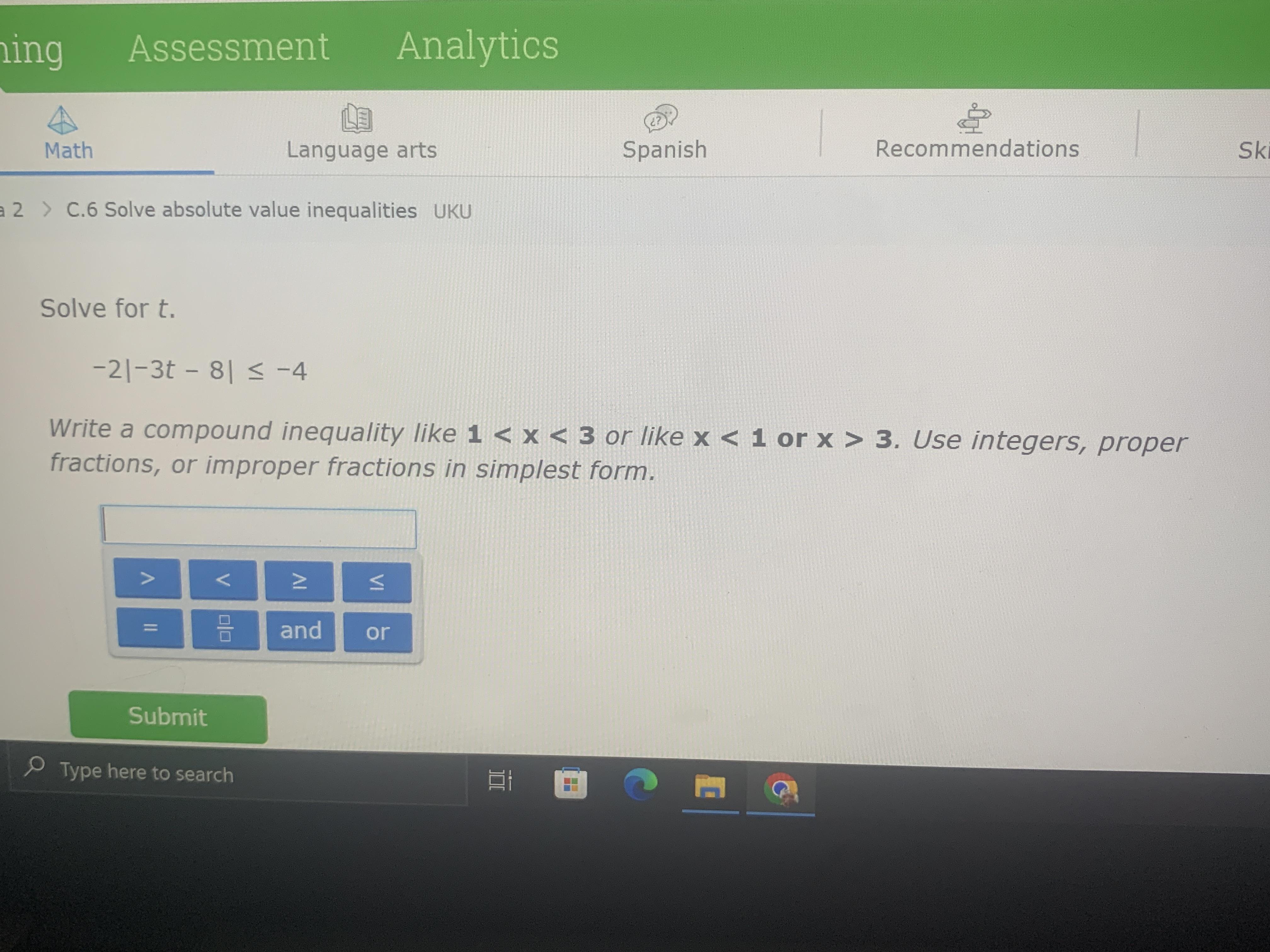
Answers
The solution of the given linear inequality will be -10/3 <= t <= -2.
What are linear inequalities?
Inequality represents the mathematical expression in which both sides are not equal. If the relationship makes the non-equal comparison between two expressions or two numbers, then it is known as inequality in Maths. In this case, the equal sign “=” in the expression is replaced by any of the inequality symbols such as greater than symbol (>), less than symbol (<), greater than or equal to symbol (≥), less than or equal to symbol (≤) or not equal to symbol (≠). The different types of inequalities in Maths are polynomial inequality, rational inequality, absolute value inequality.
The symbols ‘<‘ and ‘>’ express the strict inequalities and the symbols ‘≤’ and ‘≥’ denote slack inequalities. A linear inequality seems exactly like a linear equation but there is a change in the symbol that relates two expressions.
e.g. y>x+2
Now,
Given inequality is -2 I-3t-8I <=-4
So there are two inequalities 1. -2+3t+8<=-4 2. -2-3t-8<=-4
1. 3t+6<=-4 2.-3t-10<=-4
1. t>=-10/3 2. t<=-2
Hence,
The solution of the given linear inequality will be -10/3 <= t <= -2.
To know more about linear inequalities visit the link
https://brainly.com/question/11897796?referrer=searchResults
#SPJ1
- m/3 = -4
find the answer for m with work provided
Answers
Answer:
m = 12
Step-by-step explanation:
\(-\frac{m}{3} =-4\\\)
→ Multiply both sides by 3
\({-m} =-12\\\)
→ Multiply both sides by -1
m = 12
According to the rational root theorem, which statement about f(x)=66x^4-2x^3+11x^2+35 is true?

Answers
Answer:
Option (1)
Step-by-step explanation:
If a polynomial is given in the form of f(x) = ax³ + bx² + c
By rational root theorem rational roots of the given polynomial will be,
x = \(\frac{\pm \text{Factors of c}}{\pm \text{Factors of a}}\)
Given polynomial in the question is,
f(x) = 66x⁴- 2x³ + 11x²+ 35
Possible rational roots will be,
x = \(\frac{\pm \text{factor of 35}}{\pm \text{Facors of 66}}\)
Therefore, Option (1) will be the answer.
WHAT AMOUNT SHOULD YOU INVEST IF YOU WANT TO RECEIVE PAYMNT OF 3000 AT THE END OF EACH YEAR FOR 10 YEARS WITH THE RECEIPT OF THE FIRST PAYMENT 3 YEARS FROM NOW IF THE MONEY IS COMPOUNDED 5% ANNUALLY?
Answers
You should invest $22,627.84 if you want to receive payments of $3,000 at the end of each year for 10 years, with the first payment 3 years from now, if the money is compounded at 5% annually.
To solve this problem, we can use the present value formula for an ordinary annuity: PV = PMT * [1 - (1 + r)^-n] / r
Where:
PV = present value
PMT = periodic payment
r = interest rate
n = number of payments
In this case, we have:
PV = unknown
PMT = $3,000
r = 5%
n = 10 years (first payment in 3 years, so there are 10 payments after that)
Plugging these values into the formula,
we get:
PV = $3,000 * [1 - (1 + 0.05)^-10] / 0.05
PV = $22,627.84
Therefore, you should invest $22,627.84 if you want to receive payments of $3,000 at the end of each year for 10 years, with the first payment 3 years from now, if the money is compounded at 5% annually.
To know more about rate click here
brainly.com/question/14018365
#SPJ11
What is the least common multiple (LCM) of 8 and 12?
A. 24
B. 20
C. 4
D. 2

Answers
Step-by-step explanation:
A. 24
.....
hope it helps !
Answer:
The answer is probably A
The total time, measured in units of 100 hours, that a teenager runs her hair dryer over a period of one year is a continuous random variable X that has the density function
f(x)= x, 0
f(x) = 2 – x, 1 ≤ x < 2
f(x) = 0, elsewhere
evaluate the mean of the random variable , where Y is equal to the number of kilowatt hours expended annually
Answers
The expected value of PX is 2.Therefore(Y) = E(PX) / 100= 2 / 100= 0.02The mean of the random variable Y, where Y is equal to the number of kilowatt hours expended annually is 0.02. Therefore, the correct option is (D) 0.02.
Why The expected value of PX is 2?
The given problem states that the total time, measured in units of 100 hours, that a teenager runs her hair dryer over a period of one year is a continuous random variable X that has the density function f(x)=x, 0 < x < 1, f(x) = 2 – x, 1 ≤ x < 2 and f(x) = 0, elsewhere. We need to evaluate the mean of the random variable where Y is equal to the number of kilowatt hours expended annually.
The total time, measured in units of 100 hours, that a teenager runs her hair dryer over a period of one year is given as X. Let us calculate the expected value of the random variable Y which is equal to the number of kilowatt hours expended annually.The number of kilowatt hours expended annually Y is calculated as follows:Y = Power consumed × Hours of use per yearLet Power consumed = P and Hours of use per year = X × 100.The number of kilowatt hours expended annually Y is equal to the product of the power consumed and hours of use per year.
Therefore, we haveY = PX × 100 = (P/100) XThe expected value of Y is given byE(Y) = E(PX) / 100where E(PX) is the expected value of PX.So, we have to calculate E(PX).The expected value of PX is given bye(PX) = ∫ xf(x) dx (0 to 1) + ∫ xf(x) dx (1 to 2) (as given)Now, substituting the given function in the above formula we getE(PX) = ∫ x² dx (0 to 1) + ∫ 2x - x² dx (1 to 2)E(PX) = [x³/3] (0 to 1) + [(x² - x³/3)] (1 to 2)E(PX) = 2/3 + 4/3 = 2
The expected value of PX is 2.Therefore,E(Y) = E(PX) / 100= 2 / 100= 0.02The mean of the random variable Y, where Y is equal to the number of kilowatt hours expended annually is 0.02. Therefore, the correct option is (D) 0.02.
Learn more about PX.
brainly.com/question/30976942
#SPJ11
Help me put the inequality on the number line!

Answers
Answer:
3 with an arrow pointing to the left
Step-by-step explanation:

Please help! What mistake did I make?

Answers
Answer:
Step-by-step explanation:
to make a negative, in addition, you can’t add a negative with a positive or the number would go from -3 + 2 = -1.
for discrete logarithm problem (dlp), given the finite cyclic group integer numbers subscript p superscript asterisk times then we should solve the equation alpha to the power of x identical to beta space m o d space p. which parameters are given in the equation and which parameters we have to solve in this equation? given primitive element α and another element x, find β. given elements x and β, find primitive elements α. given primitive element α and another element β, find x. given primitive element α find elements x and β.
Answers
The parameters given in the equation α^x ≡ β (mod p) are α and β, and the parameter we need to solve for is x.
In the discrete logarithm problem (DLP), we are given a finite cyclic group of integer numbers, denoted by p*. We need to solve the equation α^x ≡ β (mod p).
Let's break down the parameters in this equation:
1. α: This is the given primitive element in the group. It is raised to the power of x.
2. x: This is the unknown element we need to find. It is the exponent to which α is raised.
3. β: This is the given element in the equation. We need to find x such that α^x ≡ β (mod p).
So, in this equation, we are given α and β, and we need to solve for x.
Now, let's consider the other scenarios:
1. Given elements x and β, find primitive element α:
In this case, we are given x and β, and we need to find the primitive element α. Unfortunately, this is not possible without additional information. We cannot determine the primitive element α solely based on the values of x and β.
2. Given primitive element α and another element β, find x:
In this case, we are given α and β, and we need to find the value of x. Solving the discrete logarithm problem involves finding the value of x that satisfies the equation α^x ≡ β (mod p). This can be a challenging problem, and there are various algorithms available to solve it, such as the Pollard's rho algorithm, the index calculus algorithm, or the baby-step giant-step algorithm.
3. Given primitive element α, find elements x and β:
In this case, we are given α and we need to find the values of x and β. Similar to the previous scenario, we cannot determine the values of x and β without additional information. We need either x or β to be given in order to solve the equation α^x ≡ β (mod p).
In summary, the parameters given in the equation α^x ≡ β (mod p) are α and β, and the parameter we need to solve for is x. The other scenarios mentioned have specific requirements in order to determine the missing parameters.
To know more about discrete logarithm problem refer here:
https://brainly.com/question/33235170
#SPJ11
Evaluate the line integral, whereCis the given curve.∫ xyds,C:x=t^2 ,y=2t,0≤t≤2C
Answers
According to the given information the line integral is \(=\frac{8}{15}(1+125.\sqrt{10})\).
What distinguishes a line integral from a definite integral?Line integrals include several integrals across a curve, but definite integrals only entail a single integral over a specified interval. This is the primary distinction between the two types of integrals.
\(\int_C\hairsp xyds,C:x=t^2,y=2t,0\le t\le3\\x^\prime=2t\\y^\prime=2\\ds=\sqrt{\left(x^\prime\right)^2+\left(y^\prime\right)^2}\\\sqrt{{4\left(t\right)}^2+4}\\\int C\ xy\ ds\ =\int_0^3\hairspt^2\cdot2t\cdot2\cdot\sqrt{1+t^2}\mathrm{\ }dt\\t=\ \ tan\ \theta\\dt=(1+{tan}^2\theta)d\theta=({Sec}^2\theta)d\theta\ \\{=\int}_0^3\hairsp4t^3\sqrt{1+t^2}\mathrm{\ }dt\\=\frac{8}{15}(1+125.\sqrt{10})\)
To know more about line integral visit:
https://brainly.com/question/15052855
#SPJ4
All the outcomes contained in one or the other of two random events, or possibly in both, make up:
Question options:
the intersection of two events
the union of two events
the probability space of an experiment
the events of an experiment
Answers
The outcomes contained in one or the other of two random events, or possibly in both, make up the union of two events.
In probability theory, the union of two events refers to the set of outcomes that are present in either one or both of the events. It represents the combination of all possible outcomes from the individual events.
For example, let's consider two events A and B. The union of these events, denoted as A ∪ B, includes all the outcomes that belong to event A, event B, or both. It represents the combined set of outcomes from the two events.
Mathematically, the union of two events is defined as:
A ∪ B = {x | x ∈ A or x ∈ B}
So, when we talk about the outcomes contained in one or the other of two random events, or possibly in both, we are referring to the union of those events. The union captures all the possible outcomes that can occur in either event or in both events simultaneously.
To learn more about probability click here
brainly.com/question/31828911
#SPJ11
Jeff drove 5,784.5 miles in 12 days . To the nearest mile , what was the rate of miles Jeff drive each day
Answers
Answer:
500mph
Step-by-step explanation:
5784.4 miles --> estimates to 6000 miles
6000/12=500
500mph
Derivations (20 marks): For each of the questions in this section provide a derivation. Other methods will receive no credit i. ∃x(Fx & Gx) ⊢ ∃xFx & ∃xGx (5 marks)
ii. ¬ 3x(Px v Qx) ⊢ Vx ¬ Px (5 marks) iii. ¬ Vx(Fx → Gx) v 3xFx (10 marks; Hint: To derive this theorem use RA.)
Answers
¬ Vx(Fx → Gx) v 3xFx ⊢ Fx → Gx [1-5, Modus Ponens]
i. ∃x(Fx & Gx) ⊢ ∃xFx & ∃xGx (5 marks)
Proof:
1. ∃x(Fx & Gx) [Premise]
2. Fx & Gx [∃-Elimination, 1]
3. ∃xFx [∃-Introduction, 2]
4. ∃xGx [∃-Introduction, 2]
5. ∃xFx & ∃xGx [Conjunction Introduction, 3 and 4]
6. ∃x(Fx & Gx) ⊢ ∃xFx & ∃xGx [1-5, Modus Ponens]
ii. ¬ 3x(Px v Qx) ⊢ Vx ¬ Px (5 marks)
Proof:
1. ¬ 3x(Px v Qx) [Premise]
2. ¬ Px v ¬ Qx [DeMorgan’s Law, 1]
3. Vx ¬ Px [∀-Introduction, 2]
4. ¬ 3x(Px v Qx) ⊢ Vx ¬ Px [1-3, Modus Ponens]
iii. ¬ Vx(Fx → Gx) v 3xFx (10 marks; Hint: To derive this theorem use RA.)
Proof:
1. ¬ Vx(Fx → Gx) v 3xFx [Premise]
2. (¬ Vx(Fx → Gx) v 3xFx) → (¬ Vx(Fx → Gx) v Fx) [Implication Introduction]
3. ¬ Vx(Fx → Gx) v Fx [Resolution, 1, 2]
4. (¬ Vx(Fx → Gx) v Fx) → (Fx → Gx) [Implication Introduction]
5. Fx → Gx [Resolution, 3, 4]
6. ¬ Vx(Fx → Gx) v 3xFx ⊢ Fx → Gx [1-5, Modus Ponens]
Learn more about Derivations
brainly.com/question/30365299
#SPJ11
I need the answerrrrrrrr plsssssssss

Answers
Answer:
Hope this helps
Step-by-step explanation:
Range: 6
Number of students with 6 siblings: 1
Number of students with no siblings: 3
Number of students with 3 or more siblings: 11
Number of observations: 20
Shape of the data: Left skewed
A farmer intends to share 32 goats amongst his sons john, peter and thomas in the ratio 1 : 3 : 4 respectively what fraction of the goats will peter get?
Answers
The number of goats Peter will have is 12.
What are ratios and proportions?An ordered pair of numbers a and b, represented as a / b, is a ratio if b is not equal to 0. A proportion is an equation that sets two ratios at the same value.
Given that a farmer intends to share 32 goats amongst his sons john, peter, and Thomas in the ratio 1 : 3: 4 respectively.
The number of the fractions will be calculated by assuming the common ratio as x. Then write an expression as below:-
x + 3x + 4x = 32
8x = 32
x = 32 / 8
x = 4
Number of goats for Peter = 3x
Number of goats for Peter = 3 x 4 = 12
Therefore, the number of goats Peter will have is 12.
To know more about ratios and proportions follow
https://brainly.com/question/12024093
#SPJ1
Graph points (-4,-1) (4, 3). Write the equation of the line that passes through the points in slope-intercept form.
Hurry please! I need it by the next 15 minutes ♡︎☺︎︎.
Answers
I upload a picture on solving it. Hope this helps!
Solve each equation or formula for the variable indicated .
11. u=vw+z, for v 12. x=b-cd, for c
13. fg-9h=10j, for g 14. 10m-p= -n, for m
Answers
The solution of each of the equations for the variable indicated in each case are as follows;
11. v = (u - z)/w.12. c = (b - x)/d.13. g = (10j + 9h)/f.14. m = p - n.Solution of equations for variables.It follows from the task content that the equations given are to be solved for the variables indicated.
The equations can therefore be solved as follows;
11. u = vw + z
Isolate vw so that we have;
vw = u -z; Divide both sides by w;
v = (u - z)/w.
12. x = b - cd
Isolate cd so that we have;
cd = b -x; Divide both sides by d;
c = (b - x)/d.
13. fg - 9h = 10j
Isolate fg so that we have;
fg = 10j + 9h; Divide both sides by f;
g = (10j + 9h)/f.
14. m - p = -n
Isolate m; so that we have;
m = p - n.
Read more on change of subject of formula;
https://brainly.com/question/28640952
#SPJ1
can somebody help me understand imaginary numbers???how do I simplify \( {i}^{13} \)how do I solve \( \sqrt{ - 49} \)
Answers
------------------------------------------------------------------------------------------------
\(\begin{gathered} \sqrt[]{-49} \\ \text{Use this property:} \\ \sqrt[]{a\cdot b}=\sqrt[]{a}\cdot\sqrt[]{b} \\ So\colon \\ \sqrt[]{-49}=\sqrt[]{49\cdot(-1)}=\sqrt[]{49}\cdot\sqrt[]{-1} \\ \text{Where:} \\ \sqrt[]{49}=7 \\ \sqrt[]{-1}=i \\ so\colon \\ \sqrt[]{49}\cdot\sqrt[]{-1}=7i \end{gathered}\)what is the density of the rock???!!!!!!!!!!

Answers
Answer:
he actual densities of pure, dry, geologic materials vary from 880 kg/m3 for ice (and almost 0 kg/m3 for air) to over 8000 kg/m3 for some rare minerals. Rocks are generally between 1600 kg/m3 (sediments) and 3500 kg/m3 (gabbro).
Step-by-step explanation:
10.
Find the measure of angle x. Hint. The sum of the measures of the angles in a triangle is 180 degrees.
A. 6°
B. 90°
C. 180°
D. 86°

Answers
Answer:
D
Step-by-step explanation:
Básicamente sumas esos 2 y se lo restas a 180.
Answer:
D. 86
Step-by-step explanation:
since the angles of a triangle add up to equal 180, all you have to do is add the angles you know and then find the one you don't by subtracting it from 180.
this is how it looks mathematically:
180=26+68+x
180=94+x
180-94=x
x=86
the confidence interval for the mean amount of money spent per household on internet service
each month is $47.45 to $72.45. what is the estimated mean?
internet each
Answers
The estimated mean amount of money spent per household on internet service each month is $59.95 with confidence interval.
To calculate the estimated mean amount of money spent per household on internet service each month, we first need to find the lower and upper bounds of the confidence interval. The lower bound is $47.45 and the upper bound is $72.45. We then take the average of these two numbers to get the estimated mean. The average of $47.45 and $72.45 is
($47.45 + $72.45) / 2
= $59.95.
Therefore, the estimated mean amount of money spent per household on internet service each month is $59.95.
Learn more about confidence interval here
https://brainly.com/question/24131141
#SPJ4
COMPLETELY simplify the following. (Show Work) (Worth a lot of points)

Answers
Answer:
\(\frac{27y^6}{8x^{12}}\)
Step-by-step explanation:
1) Use Product Rule: \(x^ax^b=x^{a+b}\).
\((\frac{3x^{-5+2}{y^3}}{2z^0yx}) ^3\)
2) Use Negative Power Rule: \(x^{-a}=\frac{1}{x^a}\).
\((\frac{3\times\frac{1}{x^3} y^3}{2x^0yx} )^3\)
3) Use Rule of Zero: \(x^0=1\).
\((\frac{\frac{3y^3}{x^3} }{2\times1\times yx} )^3\)
4) use Product Rule: \(x^ax^b=x^{a+b}\).
\((\frac{3y^3}{2x^{3+1}y} )^3\)
5) Use Quotient Rule: \(\frac{x^a}{x^b} =x^{a-b}\).
\((\frac{3y^{3-1}x^{-4}}{2} )^3\)
6) Use Negative Power Rule: \(x^{-a}=\frac{1}{x^a}\).
\((\frac{3y^2\times\frac{1}{x^4} }{2} )^3\)
7) Use Division Distributive Property: \((\frac{x}{y} )^a=\frac{x^a}{y^a}\).
\(\frac{(3y^2)^3}{2x^4}\)
8) Use Multiplication Distributive Property: \((xy)^a=x^ay^a\).
\(\frac{(3^3(y^2)^3}{(2x^4)^3}\)
9) Use Power Rule: \((x^a)^b=x^{ab}\).
\(\frac{27y^6}{(2x^4)^3}\)
10) Use Multiplication Distributive Property: \((xy)^a=x^ay^a\).
\(\frac{26y^6}{(2^3)(x^4)^3}\)
11) Use Power Rule: \((x^a)^b=x^{ab}\).
\(\frac{27y^6}{8x^12}\)
#SPJ1
Answer:
\(\displaystyle \frac{27y^{6}}{8x^{12}}\)
Step-by-step explanation:
\(\displaystyle \biggr(\frac{3x^{-5}y^3x^2}{2z^0yx}\biggr)^3\\\\=\biggr(\frac{3x^{-5}y^2x}{2}\biggr)^3\\\\=\frac{(3x^{-5}y^2x)^3}{2^3}\\\\=\frac{3^3x^{-5*3}y^{2*3}x^3}{8}\\\\=\frac{27x^{-15}y^{6}x^3}{8}\\\\=\frac{27y^{6}x^3}{8x^{15}}\\\\=\frac{27y^{6}}{8x^{12}}\)
Notes:
1) Make sure when raising a variable with an exponent to an exponent that the exponents get multiplied
2) Variables with negative exponents in the numerator become positive and go in the denominator (like with \(x^{-15}\))
3) When raising a fraction to an exponent, it applies to BOTH the numerator and denominator
Hope this helped!
Data obtained from a number of women clothing stores show that there is a (linear) relationship between sales (y, in dollars) and advertising budget (x, in dollars). The regression equation was found to be
y = 5000+ 7.25x
where y is the predicted sales value (in dollars). If the advertising budgets of two women clothing stores differ by $30,000, what will be the predicted difference in their sales?
Select one:
a. $150,000,000
b. $222,500
c. $5,000
d. $7250
e. $217,500
Answers
Therefore, the predicted difference in sales between two women's clothing stores differing by $30,000 is $217,500, which is option E.
Given a regression equation is y = 5000 + 7.25x, where y is the predicted sales value (in dollars) and x is advertising budget (in dollars).To find the predicted difference in sales of two stores which differ by $30,000 in advertising budget. Here, the slope of the line is 7.25. This means that for every dollar increase in advertising budget, sales will increase by $7.25. Therefore, a $30,000 difference in advertising budget will lead to a difference in sales of:7.25 × 30,000 = 217,500Therefore, the predicted difference in sales between two women's clothing stores differing by $30,000 is $217,500, which is option E.
To know more about regression,
https://brainly.com/question/28178214
#SPJ11
The number of units produced does not affect net operating income when using _____ costing.
Answers
The number of units produced does not affect net operating income when using absorption costing.
All production expenses, including direct materials, direct labour, and both variable and fixed overhead costs, are included in the cost of each unit produced in absorption costing. This means that as more units are manufactured, the total manufacturing cost is distributed over a greater number of units, resulting in a reduced cost per unit.
Variable costing, on the other hand, includes only variable production expenses, such as direct materials, direct labor, and variable overhead costs, in the cost of each unit produced. Fixed manufacturing overhead costs are considered period expenses and are not included in the product cost.
For such more question on Units:
https://brainly.com/question/26959824
#SPJ4
Determine the possible combinations of real and imaginary zeros, then identify all zeros
Answers
The value of f(x) = x³ - x² -5x - 3 will be (x-3)³ +8(x-3)² + 16(-3).
How to calculate the valueA polynomial is defined as an expression which is composed of variables, constants and exponents, that are combined using mathematical operations such as addition, subtraction, multiplication and division
f(x) = x³ - x² -5x - 3
= (x - 3)³ +9x² - 27x +27- x² -5x -3
= (x-3)³ +8x² - 32x + 24
= (x-3)² +8(x-3)² + 48x -72-32x +24
= (8-3)² + 8(8-3)² + 16x-48
= (x-3)³ + 8(x-3)² + 16(x-3)+48-48 =
= (x-3)³ +8(x-3)² + 16(-3).
Learn more about polynomial on:
https://brainly.com/question/4142886
#SPJ1
Una escuela debe transportar 200 estudiantes a un evento. Hay disponibles
tanto autobuses grandes como pequeños. Un autobús grande tiene
capacidad para 50 personas y alquilarlo para el evento cuesta $800. Un
autobús pequeño tiene capacidad para 40 personas y alquilarlo para el
evento cuesta $600. Hay 8 conductores disponibles el día del evento.
* Encuentra la combinación de autobuses que puedan transportar a los 200 estudiantes al menor costo posible utilizando no más de 8 conductores.
• Escribe la función objetivo y cuantifique las restricciones como desigualdades.
• Verifica que el problema se puede resolver utilizando la programación lineal.
• Grafica el sistema de desigualdades lineales. Identifique la región viable y los vértices.
• Sustituye los vértices en la función objetivo para determinar las soluciones que brindan la
solución mínima o máxima.
• Interpreta la solución en términos de otras variables de decisión
Answers
The combination of buses that can transport the 200 students at the lowest possible cost using no more than 8 drivers is 3 large buses and 2 small buses, at a total cost of $3,600.
Let's start by using just large buses. We would need 4 buses to transport all 200 students, at a cost of $3,200 (4 buses x $800 per bus). However, this would require 4 drivers, leaving only 4 drivers available for any additional buses.
Next, let's try using just small buses. We would need 5 buses to transport all 200 students, at a cost of $3,000 (5 buses x $600 per bus). This would also require 5 drivers, leaving only 3 drivers available for any additional buses.
Now, let's try a combination of large and small buses. Let's start with 3 large buses and 1 small bus. This would transport 190 students (3 buses x 50 seats + 1 bus x 40 seats), leaving 10 students who would need to be transported on another small bus. The total cost for this combination would be $3,000 (3 large buses x $800 per bus + 1 small bus x $600 per bus).
We still have 7 drivers remaining, so let's add another small bus to transport the remaining 10 students. This would bring the total cost to $3,600 (3 large buses x $800 per bus + 2 small buses x $600 per bus).
Learn more about the combination
https://brainly.com/question/19692242
#SPJ4
Translate Question: A school must transport 200 students to an event. Both large and small buses are available. A large bus holds 50 people and costs $800 to rent for the event. A small bus holds 40 people and costs $600 to rent for the event. There are 8 drivers available on the day of the event. *
Find the combination of buses that can transport the 200 students at the lowest possible cost using no more than 8 drivers. •
Please help me with this math problem!! :)

Answers
The sample standard deviation of differences sd is equal to the difference of the sample standard deviations s1 - s2.
Answers
No, the sample standard deviation of differences (sd) is not equal to the difference of the sample standard deviations (s1 - s2).
The sample standard deviation of differences is a measure of the dispersion or spread of the differences between two sets of data. It is calculated by taking the square root of the average of the squared differences between each paired observation. On the other hand, s1 and s2 represent the individual sample standard deviations of two separate data sets. Simply subtracting these values (s1 - s2) does not give you the correct measure of the dispersion of the differences between the two sets of data.
The sample standard deviation of differences (sd) cannot be obtained by merely subtracting the sample standard deviations of the individual data sets (s1 - s2). Instead, the proper method for calculating the sample standard deviation of differences should be used.
To know more standard deviation, click here
https://brainly.com/question/23907081
#SPJ11
Write each product using an exponent:
20 x 20
Answers
I apologize if I’m wrong, or misunderstood the question.
Answer:
400 or the exponent is 20²
Step-by-step explanation:
20² or 20×20=400
If a circle has the diameter of 37in, what is the area?
Answers
Answer:
A = pi (r)^2, so first you have to find the radius. The radius = 18.5 since its diameter/2. So if you plug it in, its A = pi (18.5)^2 and your answer would be A = 1075.21. Hope I helped :))
Step-by-step explanation: