Two rational numbers are each less than 1. Is their product less than 1?
Answers
Depends...
If you mean "any rational number less than 1," meaning you could use negative numbers like -3 and -4, then the answer is "no."
example: (-3)(-4) = 12.
As a general statement, the answer would be no.
If the context was that you were working only with positive numbers, then "less than 1" would imply "between 0 and 1" and the answer would be "yes".
Related Questions
plz answer only if you know ur correct. the best answer gets brainliest

Answers
Answer:
b and e are correct and f maybe
Step-by-step explanation:
theta with hat on top subscript 1 and theta with hat on top subscript 2 are unbiased estimators of the same parameter theta. what condition must be imposed on the constants k subscript 1 and k subscript 2 so that the quantity 10 k subscript 1 theta with hat on top subscript 1 plus 10 k subscript 2 theta with hat on top subscript 2 is also an unbiased estimator of theta?
Answers
The scaled values sum up to 1/10.
How to find unbiased estimator?For a linear combination of unbiased estimators to be unbiased, the coefficients must add up to 1. That is:k subscript 1 + k subscript 2 = 1
E[10 k subscript 1 theta with hat on top subscript 1 + 10 k subscript 2 theta with hat on top subscript 2] = 10 k subscript 1 E[theta with hat on top subscript 1] + 10 k subscript 2 E[theta with hat on top subscript 2]
Since both estimators are unbiased, we have:E[theta with hat on top subscript 1] = theta
E[theta with hat on top subscript 2] = theta
Substituting these values into the above equation, :E[10 k subscript 1 theta with hat on top subscript 1 + 10 k subscript 2 theta with hat on top subscript 2] = 10 k subscript 1 theta + 10 k subscript 2 theta = theta (10 k subscript 1 + 10 k subscript 2) = theta
k subscript 1 + k subscript 2 = 1
10 k subscript 1 + 10 k subscript 2 = 10
Dividing both sides by 10:k subscript 1 + k subscript 2 = 1
k subscript 1/10 + k subscript 2/10 = 1/10
Therefore, their sum is equal to 1, and their scaled values sum up to 1/10.
Learn more about unbiased estimator
brainly.com/question/30982574
#SPJ11
A point Q is 24 km away and at a bearing of 072 degrees from P. From Q a man walks at a bearing of 320 degrees, to a point R, located directly north of P. Calculate the distance of PR and QR.
Answers
Answer:
RQ=35.51 km
PR=34.62 km
Step-by-step explanation:
Bearing of Q from P = 72 degrees
The complementary angle of 72 degrees is 18 degrees.Using alternate angles, we get the first angle at Q to be 18 degrees.Bearing of R from Q=320 degrees
320=270+50
Therefore, the second angle of Q is 50 degrees.
\(\angle P=72^\circ\\\angle Q=68^\circ\\\angle R=180^\circ-(72^\circ+68^\circ)=40^\circ\)
Using Law of Sines
\(\dfrac{r}{\sin R} =\dfrac{p}{\sin P} \\\dfrac{24}{\sin 40} =\dfrac{p}{\sin 72} \\p=\sin 72 \times \dfrac{24}{\sin 40}\\\\p=RQ=35.51$ km\)
Using Law of Sines
\(\dfrac{q}{\sin Q} =\dfrac{r}{\sin R} \\\dfrac{q}{\sin 68} =\dfrac{24}{\sin 40} \\q=\dfrac{24}{\sin 40}\times \sin 68\\\\q=PR=34.62$ km\)

if the point p falls on the unit circle and has an x coordinate of 5/13 find the y coordinate of point p
Answers
To find the y-coordinate of point P on the unit circle, given that its x-coordinate is 5/13, we can utilize the Pythagorean identity for points on the unit circle.
The Pythagorean identity states that for any point (x, y) on the unit circle, the following equation holds true:
x^2 + y^2 = 1
Since we are given the x-coordinate as 5/13, we can substitute this value into the equation and solve for y:
(5/13)^2 + y^2 = 1
25/169 + y^2 = 1
To isolate y^2, we subtract 25/169 from both sides:
y^2 = 1 - 25/169
y^2 = 169/169 - 25/169
y^2 = 144/169
Taking the square root of both sides, we find:
y = ±sqrt(144/169)
Since we are dealing with points on the unit circle, the y-coordinate represents the sine value. Therefore, the y-coordinate of point P is:
y = ±12/13
So, the y-coordinate of point P can be either 12/13 or -12/13.
\(\huge{\mathfrak{\colorbox{black}{\textcolor{lime}{I\:hope\:this\:helps\:!\:\:}}}}\)
♥️ \(\large{\underline{\textcolor{red}{\mathcal{SUMIT\:\:ROY\:\:(:\:\:}}}}\)
Suppose that the rational preference relation is continuous and monotone. Then there is a continuous utility function that represents the rational preference relation. Prove it with the help of a diagram for 2 commodity framework.
Answers
Yes, there is a continuous utility function that represents a rational preference relation when it is both continuous and monotone.
In a two-commodity framework, let's assume the two commodities are represented by X and Y. We can construct a diagram with the axes representing the quantities of X and Y. The indifference curves, which represent the bundles of X and Y that yield the same level of utility, can be plotted on this diagram.
Since the preference relation is continuous and monotone, we can draw the indifference curves as smooth, upward-sloping curves without any gaps or jumps. The upward slope reflects the monotonicity, indicating that more of either X or Y is preferred to less.
To represent this preference relation with a continuous utility function, we can assign utility levels to different bundles of X and Y. For example, we can assign a utility level of 1 to a specific bundle (X₁, Y₁), and higher utility levels to bundles that are preferred to (X₁, Y₁).
By assigning utility levels in a continuous manner across the diagram, we can construct a continuous utility function that represents the rational preference relation. This function will map each bundle of X and Y to a corresponding level of utility.
In conclusion, for a rational preference relation that is continuous and monotone in a two-commodity framework, there exists a continuous utility function that represents this preference relation. The utility function can be constructed by assigning utility levels to different bundles of commodities, and the resulting indifference curves on a diagram will be smooth, upward-sloping curves. This relationship between the utility function and the preference relation allows us to mathematically model and analyze the choices and preferences of individuals in economics.
To know more about continuous utility function, visit;
https://brainly.com/question/28593010
#SPJ11
evaluate 14c 3d\dfrac14c 3d41c 3dstart fraction, 1, divided by, 4, end fraction, c, plus, 3, d when c
Answers
According to the given statement :
(1/4)c + 3d= 45/2 when c = 6 & d = 7
In math, what is a fraction?An element of a whole is a fraction. The number is represented mathematically as a quotient, where the numerator and denominator are split. Both are integers in a simple fraction. A fraction appears in the numerator as well as the denominator of a complex fraction.
How is a fraction made?Fractions can be used to represent decimals. Place your decimal number above its place value to convert it to a fraction. For instance, since the number six occupies the tenths place in the fraction 0.6, we must multiply it by 10 to get the comparable fraction, 6/10.
According to the given data:Evaluate (1/4)c + 3d
when c = 6 & d = 7
x = (1/4)c + 3d
putting c = 6
& d = 7
=> x = (1/4) * 6 + 3*7
=> x = 3/2 + 21
=> x = (3 + 42)/2
=> x = 45/2
=> x = 22.5
=> (1/4)c + 3d= 22.5 when c = 6 & d = 7
To know more about Fraction visit:
https://brainly.com/question/10354322
#SPJ4
An artcie in the San Jose Mercury News stated that students in the California state university system take 4.5 years, on average, to finish their undergraduate degrees. Suppose you believe that the mean time is longer. You conduct a survey of 49 students and obtain a sample mean of 5.1 with a sample standard devlation of 1.2. Do the data support your claim at the 19 level?
Answers
The data supports your claim that the mean time to finish undergraduate degrees is longer than the reported average at the 19 levels of significance. To determine if the data supports your claim, a hypothesis test can be conducted.
Using a significance level of 0.05 (19 levels), the null hypothesis (H0) assumes that the mean time is 4.5 years, while the alternative hypothesis (Ha) assumes that the meantime is greater than 4.5 years.
A one-sample t-test can be employed to compare the sample mean to the population mean. With a sample size of 49, a sample mean of 5.1, and a sample standard deviation of 1.2, the test statistic (t-value) is calculated as 3.497.
By comparing the calculated t-value to the critical t-value of 1.96 at a significance level of 0.05, it can be determined if the data supports your claim.
Since the calculated t-value (3.497) exceeds the critical t-value (1.96), the null hypothesis can be rejected. This indicates that the data supports your claim that the mean time to finish undergraduate degrees is longer than the reported average at the 19 levels of significance.
It should be noted that this conclusion is specific to the given sample and assumes that the sample is representative of the entire population. Further analysis and replication of the study may be necessary for a more comprehensive understanding.
Learn more about hypothesis tests at
brainly.com/question/17099835
#SPJ4
Mr. Cooper was training for a bicycle race. He rode 21.5 miles away from his house, rode back toward the house for 6.2 miles, and then rode away from his house for 3.8 miles. How far is he from his house? *
Answers
Answer: 19. 1 miles
Step-by-step explanation:
21.5 - 6.2 = 15.3
15.4 + 3.8 = 19.1
Answer:
Mr. Cooper rode 21.5 miles away from his house, then he rode back toward his house 6.2 miles. Then he rode away from his house for another 3.8 miles.
21.5 miles - 6.2 miles = 15.3 miles
15.3 miles + 3.8 miles = 19.1 miles
Mr. Cooper is 19.1 miles away from his house.
Step-by-step explanation:
q29:a survey was conducted with 900 ohio university students. one of the conclusions fromthis survey was that 55% of the students believed the academics at ohio university were 'verystrong'. based on these sample results, are you convinced that a majority (i.e. over 50%) of allstudents believe the academics are 'very strong'?
Answers
Based on the information provided, we can conduct a hypothesis test to determine if a majority of all students at Ohio University believe the academics are 'very strong'.
Let's set up the null and alternative hypotheses:
Null hypothesis (H₀): The proportion of all students who believe the academics are 'very strong' is equal to 50% or less.
Alternative hypothesis (H₁): The proportion of all students who believe the academics are 'very strong' is greater than 50%.
To test these hypotheses, we can use a one-sample proportion test. We will compare the sample proportion (55%) to the hypothesized proportion (50%) and assess if the difference is statistically significant.
Using appropriate statistical methods, such as calculating the test statistic and obtaining the p-value, we can evaluate the evidence against the null hypothesis. If the p-value is less than the chosen significance level (e.g., 0.05), we would reject the null hypothesis and conclude that a majority of all students at Ohio University believe the academics are 'very strong'. Otherwise, if the p-value is greater than the significance level, we would fail to reject the null hypothesis and conclude that there is not enough evidence to support the claim of a majority belief.
Please note that without the actual test results or the p-value, we cannot make a definitive conclusion in this particular case.
Learn more about majority here
https://brainly.com/question/28293697
#SPJ11
Represent the following sentence as an algebraic expression, where "a number" is the letter x. \text{a number raised to the third power.} a number raised to the third power.
Answers
Answer: #x^3
Step-by-step explanation:
"A number " is represented by the letter xA number (x) raised (^) the third power will be,x^3
note: An algebraic expression doesn't have an equal sign (=)
Know more about algebraic expressions here:
https://brainly.com/question/6566332
https://brainly.com/question/12470265
when the spring is stretched and the distance from point a to point b is 5.3 feet, what is the value of θ to the nearest tenth of a degree?
a. 60.0
b. 35.2
c. 45.1
d. 55.5
Answers
When the spring is stretched and the distance from point a to point b is 5.3 feet, the value of θ is 53.13 degrees
The distance between point a to point b = 5.3 feet
The length of the top side = 3 feet
Therefore, it will form a right triangle
Here we have to use trigonometric function
Here adjacent side and the hypotenuse of the triangle is given
The trigonometric function that suitable for the given conditions is
cos θ = Adjacent side / Hypotenuse
Substitute the values in the equation
cos θ = 3 / 5
θ = cos^-1(3 / 5)
θ = cos^-1(0.6)
θ = 53.13 degrees
Therefore, the value of θ is 53.13 degrees
Learn more about trigonometric function here
brainly.com/question/25618616
#SPJ4
For how many (not necessarily positive) integer values of n is the value 4000x(0.4)^n an integer?
I solved this, nevermind!
Answers
The number of integer values of n for which the value 4000 × (0.4)ⁿ is an integer is; 9
How to find the integer values in algebra?We are given the expression;
4000 × (0.4)ⁿ
This can also be expressed as;
4000 × (²/₅)ⁿ which can further be expressed in exponent form as;
(2⁵ × 5³) × (²/₅)ⁿ = 2⁽⁵ ⁺ ⁿ⁾ × 5⁽³ ⁻ ⁿ⁾
Since this expression is an integer, we need:
1) 5 + n ≥ 0 for which n ≥ -5
2) 3 - n ≥ 0 for which n ≤ 3
Taking the intersection gives;
-5 ≤ n ≤ 3
Thus;
Number of Integer Values = 3 - (-5) + 1 = 9
Read more about Integer Values at; https://brainly.com/question/17695139
#SPJ1
A bicycle has tires with a diameter of 26 inches. Find the radius and circumference of each tire. Round your answer to the nearest hundredth, if necessary. radius: in. circumference: in.
Answers
For a bicycle with tires having a diameter of 26 inches, the radius and circumference of each tire can be calculated.
The radius of a circle is equal to half of its diameter. Therefore, to find the radius of each tire, we divide the diameter by 2.
Given that the diameter of the bicycle tires is 26 inches, the radius can be calculated as 26/2 = 13 inches.
The circumference of circle is the distance around its perimeter and can be calculated using the formula C = 2πr, where r is the radius.
Substituting the value of the radius (13 inches) into the formula, we get C = 2π(13) = 26π inches.
To round the answers to the nearest hundredth, we can use an approximation of π as 3.14.
Therefore, the radius of each tire is approximately 13 inches, and the circumference of each tire is approximately 26π inches or approximately 81.64 inches (rounded to the nearest hundredth).
Learn more about circumference of circle here:
https://brainly.com/question/17130827
#SPJ11
ly| ≤3
Are the lines on graph at 3 and -3 also part of the answer?

Answers
Answer:
Yes, the lines on the graph at 3 and -3 a part of the solution,
Step-by-step explanation:
The inequality \(|y| \leq 3\) contains all the values of \(y\) 3 units from the origin including the values 3 and -3.
Thus, the lines on the graph y =-3 and y = 3 are the part of the solution.
Learn more about Graphs and inequality here,
brainly.com/question/16608196
brainly.com/question/17448505
1.) a.) Given the data representing test scores in a chemistry class, construct a grouped frequency distribution of
the data using classes 30-40, 40-50, and so on using the grid provided.
72
55
35
53
85
67
48
86
39
73
57
99
45
48
40
80
Classes
70
56
59
75
85
97
65
62
66
88
94
74
Frequency
92
74
98
81
100 87
76
89
84
73
85
80
Answers
Given statement solution is :- To construct a grouped frequency distribution for the given test scores data, we will use the provided classes: 30-40, 40-50, and so on. Here's the grouped frequency distribution:
Classes Frequency
30-40 2
40-50 3
50-60 4
60-70 8
70-80 9
80-90 7
90-100 7
To construct a grouped frequency distribution for the given test scores data, we will use the provided classes: 30-40, 40-50, and so on. Here's the grouped frequency distribution:
Classes Frequency
30-40 2
40-50 3
50-60 4
60-70 8
70-80 9
80-90 7
90-100 7
To create this distribution, we count the number of scores that fall within each class range. For example, the class 30-40 has 2 scores falling within that range (35 and 39), and the class 40-50 has 3 scores (45, 48, and 48).
Note: It seems there is an inconsistency in the data provided. The class range 70-80 has 9 scores, but the frequencies given for the other classes do not match the actual number of scores falling within those ranges. Therefore, I have used the actual counts from the data to construct the grouped frequency distribution.
For such more questions on Grouped Frequency Distribution
https://brainly.com/question/29115601
#SPJ8
Only Question #5!
If JKLM is a rectangle, find
Measure NML

Answers
Answer;
5. 22°
6. 74°
Step-by-step explanation:
5. Since rectangle JKLM has two equal diagonals that intersect each other, therefore JN = MN.
If JN = MN therefore:
m<NMJ = m<NJM
Thus:
3x + 38 = 7x - 2
Solve for x. Collect like terms
3x - 7x = -38 - 2
-4x = - 40
Divide both sides by -4
x = 10
✔️Find m<NMJ
m<NMJ = 7x - 2
Plug in the value of x
m<NMJ = 7(10) - 2 = 70 - 2
m<NMJ = 68°
✔️Find m<NML;
m<NML = 90 - 68° (Complimentary angles)
m<NML = 22°
6. Diagonal of a rhombus bisects teach vertex angle, this means that <U is divided into two equal parts. Therefore:
10x - 23 = 3x + 19
Solve for x. Collect like terms
10x - 3x = 23 + 19
7x = 42
Divide both sides by 7
x = 6
✔️Find m<RST:
m<SUT = m<RST (opposite angles of a rhombus are congruent)
Thus,
m<SUT = (10x - 23) + (3x + 19)
Plug in the value of x
m<SUT = (10(6) - 23) + (3(6) + 19)
= (60 - 23) + (18 + 19)
= 37 + 37
m<SUT = 74°
Also, m<RST = 74°
Joyce has two pendants. One is in the shape of a triangular prism with a base of 10 square centimeters and a height of 1.5 centimeters. The second is in the shape of a triangular pyramid with the same base and height dimensions. How much gold was used to make both pendants?
Answers
The total volume of gold used for both pendants is 15 + 5 = 20 cubic centimeters.
How to find the total volume usedTo find the amount of gold used to make the pendants, we first need to calculate the volume of each pendant.
The volume of the triangular prism is given by:
V = Bh
Where B is the base area (10 square centimeters) and h is the height (1.5 centimeters).
So, the volume of the triangular prism is:
V = 10 x 1.5 = 15 cubic centimeters
The volume of the triangular pyramid is given by:
V = (Bh) / 3
Where B is the base area (10 square centimeters) and h is the height (1.5 centimeters).
So, the volume of the triangular pyramid is:
V = (10 x 1.5) / 3 = 5 cubic centimeters
The total volume of gold used for both pendants is 15 + 5 = 20 cubic centimeters.
Read more about volume here:
https://brainly.com/question/1972490
#SPJ1
I don't understand the question.

Answers
Answer:
Distribution means to spread the product throughout the marketplace such that alarmed number of people can buy it.
Represent each expression as a multiple of a sum of whole numbers with no common factor. 9 × (2 + 4 + 3) 4 × (4 + 7 + 12) 12 × (3 + 8 + 1) 14 × (9 + 2 + 1) 27 × (2 + 3 + 1) 22 × (4 + 3 + 1) (36 + 96 + 12) arrowRight (126 + 28 + 14) arrowRight (16 + 28 + 48) arrowRight (18 + 36 + 27) arrowRight (54 + 81 + 27) arrowRight (88 + 66 + 22) arrowRight
Answers
Answer:
(18 + 36 + 27) = 9 x (2+4+3)
(88 + 66 + 22) = 22 x (4+3+1)
(36 + 96 + 12) = 12 x (3+8+1)
(126 + 28 + 14) = 14 x (9+2+1)
(54 + 81 + 27) = 27 x (2+3+1)
(16 + 28 + 48) = 4 x (4+7+12)
Step-by-step explanation:
The equations in the solution represent each expression as a multiple of a sum of whole numbers with no common factor.
What is the algebraic expression?Algebraic expressions are mathematical statements with a minimum of two terms containing variables or numbers.
As per the given question, the required solution would be as:
(18 + 36 + 27) = 9 × (2+4+3)
This equation represents each expression as a multiple of a sum of whole numbers with no common factor.
Similarly further equations like as :
(88 + 66 + 22) = 22 × (4+3+1)
(36 + 96 + 12) = 12 × (3+8+1)
(126 + 28 + 14) = 14 × (9+2+1)
(54 + 81 + 27) = 27 × (2+3+1)
(16 + 28 + 48) = 4 × (4+7+12)
Thus, the above equations represent each expression as a multiple of a sum of whole numbers with no common factor.
Learn more about the algebraic expression here :
brainly.com/question/21751419
#SPJ5
Please help!!! Angles!

Answers
Answer:
m∠JKM = 63°
m∠MKL = 27°
Step-by-step explanation:
Since ∠JKL is a right angle. This means that by summing up both m∠JKM and m∠MKL will result in the same as ∠JKL figure. Thus, m∠JKM + m∠MKL = m∠JKL which is 90° by a right angle definition.
\(\displaystyle{\left(12x+3\right)+\left(6x-3\right) = 90}\)
Solve the equation for x:
\(\displaystyle{12x+3+6x-3 = 90}\\\\\displaystyle{18x=90}\\\\\displaystyle{x=5}\)
We know that x = 5. Next, we are going to substitute x = 5 in m∠JKM and m∠MKL. Thus,
m∠JKM = 12(5) + 3 = 60 - 3 = 63°
m∠MKL = 6(5) - 3 = 30 - 3 = 27°
Find the ordered pair solution for the
system of equations.
f(x) = x² + 4
f(x) = 4x
([? ], 1)
Enter the smallest x first.
Answers
To find the ordered pair solution for this system of equations, we need to find the value of x that satisfies both equations and then find the corresponding value of f(x) using either of the equations. the ordered pair solution is: (2, 1)
What is the system of equations?Setting the two functions equal to each other, we have:
\(x^2 + 4 = 4x\)
Rearranging, we get:
\(x^2 - 4x + 4 = 0\)
This quadratic equation can be factored as:
\((x - 2)^2 = 0\)
Therefore, the only solution is \(x = 2.\)
Substituting x = 2 into either of the original equations, we have:
\(f(2) = 2^2 + 4 = 8\)
Therefore, the ordered pair solution is: \((2, 1)\)
Learn more about equations here:
https://brainly.com/question/24065247
#SPJ9
Brandy's candies paid $23 million in dividends during 1998, while also making net common stock repurchases of $27 million. what was the cash flow to stockholders for 1998?
Answers
The cash flow to stockholders for 1998 is -$4 million.
To calculate the cash flow to stockholders for 1998, follow these steps:
Identify the dividends paid: Brandy's Candies paid $23 million in dividends during 1998.
Determine the net common stock repurchases: Brandy's Candies made net common stock repurchases of $27 million during 1998.
Subtract the net common stock repurchases from the dividends paid: $23 million - $27 million = -$4 million.
The resulting cash flow to stockholders for 1998 is -$4 million, indicating a net outflow of cash from stockholders.
You can learn more about cash flow at
https://brainly.com/question/10922478
#SPJ11
Mark and his best friend found some
money in an envelope. They split the
money evenly, each getting $6. How
much money did they find?
Please explain how you got your answer.
Answers
Answer:
$12
Step-by-step explanation:
They each get $6
6 + 6 = 12
Hope this helps!
What is the product of 2x3 +9 and x3 +7?
Answers
The product of the expression is 2x⁶ + 23x³ + 63
How to determine the productFirst, we should note that algebraic expressions are described as expressions that are composed of coefficients, terms, constants, variables and factors.
These algebraic expressions are also made up of mathematical operations, such as;
BracketAdditionMultiplicationDivisionParenthesesSubtractionFrom the information given, we have that;
2x3 +9 and x3 +7?
Then,
(2x³ + 9)(x³ + 7)
expand the bracket
2x⁶ + 14x³ + 9x³ + 63
add like terms
2x⁶ + 23x³ + 63
Learn about algebraic expressions at: https://brainly.com/question/4344214
#SPJ1
A fair spinner has 12 equal sections: 5 red, 4 blue and 3 green.
It is spun twice.
What is the probability of getting the same colour twice?
(give your answer as a fraction)
Answers
1/5 I think I believe
At Simba Travel Agency, the price of a climbing trip to Mount Kilimanjaro includes an initial fee plus a constant fee per meter.
The variable f models the fee (in dollars) for climbing d meters.
f=110+0.12d
What is the initial fee?
Answers
110 is the initial fee.
intial fee is the y-intercept.
Which expression is equal to

Answers
explanation: below
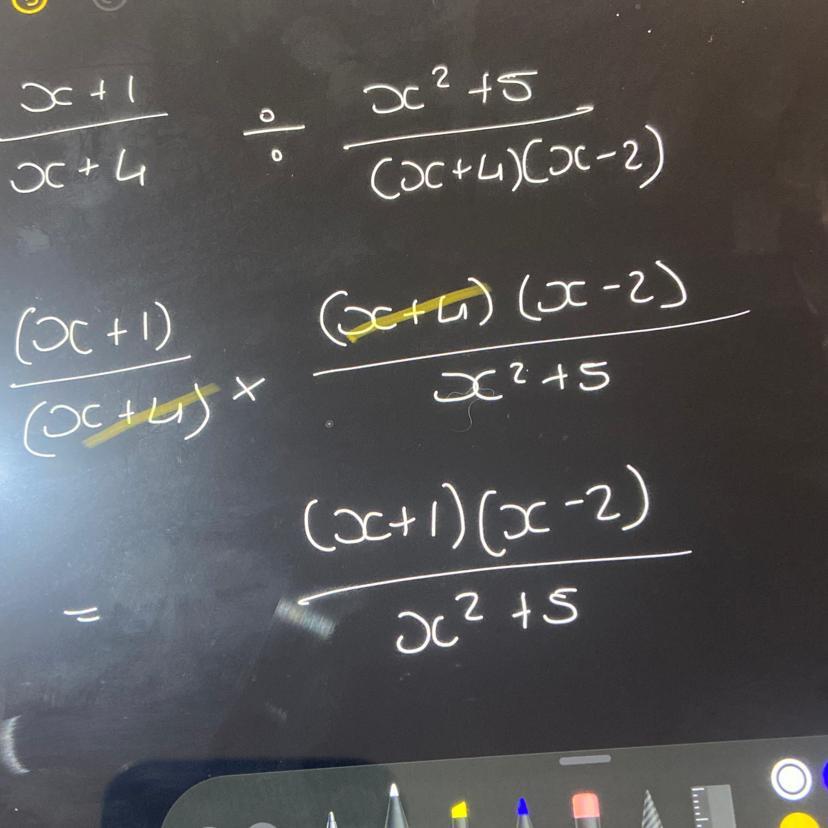
The equation represents which of the following curves? A hyperbola with new origin (x, y) = (2, -1). An ellipse with new origin (x, y) = (2,−1). A hyperbola with new origin (x, y) = (2, 1). An ellipse with new origin (x, y) = (-2,1). None of these options are correct. 3x² 12x 4y² 8y4=0
Answers
The equation \(\(3x^2 + 12x + 4y^2 + 8y^4 = 0\)\) does not represent any of the given options: a hyperbola with a new origin (2, -1), an ellipse with a new origin (2,-1), a hyperbola with a new origin (2, 1), or an ellipse with a new origin (-2,1).
The given equation can be rewritten as\(\(3(x^2 + 4x) + 4(y^2 + 2y^4) = 0\).\) By completing the square, we can rewrite it as\(\(3(x^2 + 4x + 4) + 4(y^2 + 2y^4 + 1) = 12 + 4\).\)Simplifying further, we get\(\(3(x + 2)^2 + 4(y^2 + 2y^4 + 1) = 16\).\)
This equation represents an ellipse with its center at the point (-2, 0) in the x-direction and (0, 0) in the y-direction. The term \(x + 2^2\)\)indicates a horizontal shift of the ellipse to the left by 2 units. The term\(\(y^2 + 2y^4 + 1\)\)represents the vertical stretching and compression of the ellipse. Since the coefficient of \(\(y^2\)\)is positive, the ellipse is elongated vertically.
Therefore, the equation represents an ellipse with a new origin at (-2, 0).
Learn more about ellipse here
https://brainly.com/question/20393030
#SPJ11
Nicole invests a sum of money in a savings account with a fixed annual interest rate of 4.61% compounded annually. After 6 years, the balance reaches $5,485.85. What was the amount of the initial investment? (Round your answer to the nearest whole dollar)
Answers
The amount of the the initial investment (principal) is $4,188( nearest dollar)
What is compound interest?Compound interest is calculated on the principal amount and the accumulated interest of previous periods.
Compound interest is given as A = P(1+r/100)^t
where A is the amount over a period of time
P is the Principal amount before the interest and t is the time and r is the interest rate
Therefore 5485.85= P( 1+4.61/100)⁶
5485.85= P(1+0.0461)⁶
5485.85= P (1.0461)⁶
5485.85= 1.31P
divide both sides 1.31
P = 5485.85/1.31
P= $4188( nearest dollars)
therefore the principal amount is $4188
learn more about compound interest from
https://brainly.com/question/24274034
#SPJ1
Could someone give me the answer to this question

Answers
Answer:
Circumference = 62.8
Step-by-step explanation:
The equation for discovering a circle's circumference is simple:
-------------------------------------------------------
C = 2πr
C is the circumference (how long is the circle around)
R is the radius (half of the width of the circle)
--------------------------------------------------------
So, instead of giving us the radius, they gave us the diameter. (the full width of the circle) So, we divide that by 2 to get the radius.
20/2=10
Now, time to fill in the equation.
C = 2π10
C = 20π
C = 62.8
*They told us to use 3.14 for pi.