what is 6/10 as hundredths in fraction form and in decimal form
Answers
Answer:
Hundredths: \(\frac{6}{10}\)
6 × 10 = 60 & 10 × 10 = 100
(multiply both the numerator and denominator by 10)
Decimal: 0.6
6 ÷ 10 =
good luck, hope this helps :)
Related Questions
The first two steps in determining the solution set of the system of equations, y = x2 – 6x + 12 and y = 2x – 4, algebraically are shown in the table.
Which represents the solution(s) of this system of equations?
(4, 4)
(–4, –12)
(4, 4) and (–4, 12)
(–4, 4) and (4, 12)

Answers
Answer:
(4,4)
Step-by-step explanation:
The solution set of the system of equations can be found by setting the two equations equal to each other and solving for x.
x^2 - 6x + 12 = 2x - 4
x^2 - 8x + 16 = 0
(x - 4)^2 = 0
x = 4
Since both equations in the system are equal to y, we can substitute x = 4 into either equation to find the corresponding value of y.
y = 2x - 4 = 2(4) - 4 = 4
Therefore, the solution of this system of equations is (4, 4).
Therefore, the correct answer is (4, 4).
how much is a penny that doubles everyday for 30 days?
Answers
If you doubled a penny every day, you would have $5,368,709.12
You can see that the decision is rather straightforward: having a single penny that doubles every day for a month are preferable to receiving $1 million upfront. This is due to compound interest's strength.
The interest earned on savings that are computed using both the original principal and the interest accrued over time is known as compound interest.
It is thought that Italy in the 17th century is where the concept of "interest on interest" or compound interest first appeared. It will accelerate the growth of a total more quickly than simple interest, which is solely calculated on the principal sum.
Money multiplies more quickly thanks to compounding, and the more compounding periods there are, the higher the compound interest will be.
If I double this particular penny every day, I would have 30 extra pennies, plus my original one. I have $0.31 literal dollars
On day 30, if you doubled a penny every day, you would have $5,368,709.12.
Know more about compound interest at:
https://brainly.com/question/28020457
#SPJ4
A gardener planted a newly sprouted oak tree that was just 3.5 inches tall. The sapling grew 12 inches each year.
Write an equation that shows how the sapling's height in inches, y, depends on the number of years since it was planted, x.
Answers
Answer:
y = 12x + 3.5--------------------------------------
Initial height is the y-intercept of the line.
Yearly growth rate is the slope.
So we have:
m = 12, b = 3.5.The equation of the line with these constants is:
y = mx + by = 12x + 3.5Abram competed in a 100-meter swimming race. He swam
the first 50 m in 29.65 seconds. He swam the second 50 m
in 30.24 seconds. How long did it take Abram to complete
the race?
Answers
Answer:
Step-by-step explanation:
59.89
Which describes the algebraic expression, 4x + 6?
Answers
Answer:
10x=4 +x +6
Step-by-step explanation:
Find the measure of each numbered angle and name the theorems that you used to justify your work. ∠2 and ∠3 are complementary. ∠1≅∠4 and m∠2=28°
Answers
Since ∠2 and ∠3 are complementary, we know that their sum is equal to 90 degrees. Thus, we can say that m∠3 = 90 - m∠2 = 90 - 28 = 62 degrees.
Also, since ∠1 ≅ ∠4, they have the same measure. Therefore, we can say that m∠1 = m∠4.
Now, we can use the fact that the sum of the measures of the angles in a triangle is 180 degrees. In triangle ABC, we have:
m∠1 + m∠2 + m∠3 = 180
Substituting the values we know, we get:
m∠1 + 28 + 62 = 180
Simplifying the equation, we get:
m∠1 = 90
Therefore, each of ∠1 and ∠4 has a measure of 90 degrees.
To justify our work, we used the following theorems:
Complementary angles theorem: If two angles are complementary, their sum is equal to 90 degrees.
Triangle angle-sum theorem: The sum of the measures of the angles in a triangle is equal to 180 degrees.
To learn more about equation click here: brainly.com/question/29538993
#SPJ11
How do you write 156 in scientific notation?
Answers
Answer:
Why is 156 written as 1.56 x 10^2 in scientific notation?
Step-by-step explanation:
find the angles marked with the letters.

Answers
Answer:
bigger b is 55°, the other b is 35°, c is 29°
Step-by-step explanation:
angles opposite each other on a point are the same so the bigger b is 55
55+90=145
180-145=35 so b is 35
alternate angles are the same so 35+116=151
180-151=29 so c=29
Identify the area of sector AMC in terms of pi and rounded to the nearest hundredth.HELP PLS

Answers
Answer:
Step-by-step explanation:
The formula for the area of a sector is
\(A=\frac{\theta}{360}*\pi r^2\) where θ is the measure of the central angle and r is the radius.
Our central angle is a right angle and the radius is 4, so filling in the formula looks like this:
\(A=\frac{90}{360}*\pi (4)^2\) and
\(A=\frac{1}{4}*\pi (16)\)
16 divides by 4 evenly, so
A = 4π.in²
If we multiply 4 by the value of π and then round, that number, to the nearest hundredth, is
A = 12.57 in²
An alloy contains 13. 5 gms of copper and 4. 5 gms of zinc. Find the ratio by mass of copper to zinc in the alloy
Answers
The ratio by mass of copper to zinc in the alloy is 3:1.
To find the ratio by mass of copper to zinc in the alloy, we need to first calculate the total mass of the alloy. We can do this by adding the mass of copper and zinc:
Total mass of alloy = 13.5 g + 4.5 g = 18 g
Now we can find the ratio of copper to zinc by dividing the mass of copper by the mass of zinc:
Ratio of copper to zinc = 13.5 g / 4.5 g = 3:1
Therefore, the ratio by mass of copper to zinc in the alloy is 3:1.
Learn more about mass here
https://brainly.com/question/86444
#SPJ11
Help me pls
What is the students error?

Answers
Answer:
first one;)
Step-by-step explanation:
what percentage of the cube’s volume is underwater while in equilibrium?
Answers
The percentage of the cube's volume that is underwater while in equilibrium is equal to the ratio of the density of the cube to the density of water, multiplied by 100.
To determine the percentage of the cube's volume that is underwater while in equilibrium, we can use the following formula:
percentage underwater = (V_submerged / V_cube) * 100
where V_submerged is the volume of the cube that is submerged in water and V_cube is the total volume of the cube.
Since the cube is in equilibrium, the buoyant force acting on the cube is equal to the weight of the cube. The buoyant force is equal to the weight of the water displaced by the submerged portion of the cube. Therefore, we can write:
density of water * V_submerged * g = density of cube * V_cube * g
where g is the gravitational acceleration. Solving for V_submerged, we get:
V_submerged = (density of cube / density of water) * V_cube
Substituting this expression for V_submerged into the formula for the percentage underwater, we get:
percentage underwater = [(density of cube / density of water) × V_cube / V_cube] × 100
= (density of cube / density of water) × 100
Your complete Question is here:
The cube is placed in a container of water. It is observed to float, i.e., it reaches equilibrium when part of the cube is below the surface of the water. What percentage of the cube’s volume is underwater while in equilibrium?
Click the below link, to learn more about buoyancy force:
https://brainly.com/question/17117181
#SPJ11
three interior angles of a quadrilateral have measures of 120°, 100°, and 75°. what's the measure of the fourth interior angle? question 8 options: a) 65° b) 360° c) 70° d) 100°
Answers
The measure of the fourth interior angle of the quadrilateral is 65°. Hence, the correct answer is (a) 65°.
To calculate the measure of the fourth interior angle of a quadrilateral when the measures of three interior angles are known, we can use the fact that the sum of the interior angles of a quadrilateral is always equal to 360 degrees.
Let's denote the measure of the fourth interior angle as x.
Provided that the measures of the three known interior angles are 120°, 100°, and 75°, we can write the equation:
120° + 100° + 75° + x = 360°
Combining like terms, we have:
295° + x = 360°
To solve for x, we subtract 295° from both sides of the equation:
x = 360° - 295°
Calculating this, we obtain:
x = 65°
Hence, the answer is (a) 65°.
To know more about quadrilateral refer here:
https://brainly.com/question/29934440#
#SPJ11
help :(( pleaseeeeeee

Answers
Answer:
69.29 deg
Step-by-step explanation:
Recall that tan(x) = opposite/adjacent. This is helpful because we know the opposite and adjacent leg from the angle x! This gives us tan(x) = 43/18, so we can solve for x by taking tan^-1 of both sides, giving x = tan^-1(43/18), or 67.29 degrees.
please help me best answer gets an brainlist !!!
What values for y would complete the table provided below that would model the given equation:
y=20x+50
Question 2 options:
0, 20, 40, 60
50, 70, 90, 110
50, 100, 150, 200
20, 40, 60, 80

Answers
The answer is the range of (50, 70, 90, 110).
Based on experience, 60% of the women who request a pregnancy test at a certain clinic are actually pregnant.
In a random sample of 12 women
a) what is the probability that at least 10 are pregnant?
b) what is the probability that exactly 6 are pregnant?
c) what is the probability that at most 2 are pregnant?
d) what are the mean and Standard Diviation of this distrubution?
Answers
For a) the total probability of at least 10 are pregnant is 0.4509, or 45.09%. For b) the probability that exactly 6 women are pregnant are 0.2128, or 21.28%. For c) same as option b). For d) Mean is (μ) = \(n * p\) , Standard Deviation (σ) = \(sqrt(n * p * q)\).
To solve these probability questions, we can use the binomial probability formula. In the given scenario, we have:
- Probability of success (p): 60% or 0.6 (a woman requesting a pregnancy test is actually pregnant).
- Probability of failure (q): 40% or 0.4 (a woman requesting a pregnancy test is not pregnant).
- Number of trials (n): 12 ( women in the sample).
a) To find the probability that at least 10 women are pregnant, we need to calculate the probability of 10, 11, and 12 women being pregnant and sum them up.
\(\[P(X \geq 10) = P(X = 10) + P(X = 11) + P(X = 12)\]\)
Where X follows a binomial distribution with parameters n and p.
Using the binomial probability formula, the probability for each scenario is:
\(\[P(X = k) = \binom{n}{k} \cdot p^k \cdot q^{(n-k)}\]\)
Using this formula, we can calculate:
\(\[P(X = 10) = \binom{12}{10} \cdot (0.6)^{10} \cdot (0.4)^2\]\)
\(\[P(X = 11) = \binom{12}{11} \cdot (0.6)^{11} \cdot (0.4)^1\]\)
\(\[P(X = 12) = \binom{12}{12} \cdot (0.6)^{12} \cdot (0.4)^0\]\)
To find the total probability of at least 10 women being pregnant, we need to calculate the probabilities for each possible number of pregnant women (10, 11, and 12) and add them up.
Let's calculate each individual probability:
For 10 pregnant women:
\(\[P(X = 10) = \binom{12}{10} \cdot (0.6)^{10} \cdot (0.4)^2\]\)
For 11 pregnant women:
\(\[P(X = 11) = \binom{12}{11} \cdot (0.6)^{11} \cdot (0.4)^1\]\)
For 12 pregnant women:
\(\[P(X = 12) = \binom{12}{12} \cdot (0.6)^{12} \cdot (0.4)^0\]\)
Now, we can add up these probabilities to find the total probability of at least 10 women being pregnant:
\(\[P(\text{{at least 10 women pregnant}})\) = \(P(X = 10) + P(X = 11) + P(X = 12)\]\)
Calculating each of these probabilities:
\(\[P(X = 10) = \binom{12}{10} \cdot (0.6)^{10} \cdot (0.4)^2 = 0.248832\]\)
\(\[P(X = 11) = \binom{12}{11} \cdot (0.6)^{11} \cdot (0.4)^1 = 0.1327104\]\)
\(\[P(X = 12) = \binom{12}{12} \cdot (0.6)^{12} \cdot (0.4)^0 = 0.06931408\]\)
Adding up these probabilities:
\(\[P(\text{{at least 10 women pregnant}})\) = \(0.248832 + 0.1327104 + 0.06931408 = 0.45085648\]\)
Therefore, the total probability of at least 10 women being pregnant is approximately 0.4509, or 45.09%.
b) To find the probability that exactly 6 women are pregnant, we can use the binomial probability formula:
\(\[P(X = 6) = \binom{12}{6} \cdot (0.6)^6 \cdot (0.4)^{12-6}\]\)
To find the probability that exactly 6 women are pregnant, we can use the binomial probability formula:
\(\[P(X = 6) = \binom{12}{6} \cdot (0.6)^6 \cdot (0.4)^{12-6}\]\)
Let's calculate this probability:
\(\[\binom{12}{6}\]\) represents the number of ways to choose 6 women out of 12. It can be calculated as:
\(\[\binom{12}{6} = \frac{12!}{6! \cdot (12-6)!} = \frac{12!}{6! \cdot 6!} = 924\]\)
Now, we can substitute this value along with the given probabilities:
\(\[P(X = 6) = 924 \cdot (0.6)^6 \cdot (0.4)^{12-6}\]\)
Evaluating this expression:
\(\[P(X = 6) = 924 \cdot (0.6)^6 \cdot (0.4)^6\]\)
Calculating the values:
\(\[P(X = 6) = 924 \cdot (0.6)^6 \cdot (0.4)^6 = 0.21284004\]\)
Therefore, the probability that exactly 6 women are pregnant is approximately 0.2128, or 21.28%.
c) To find the probability that at most 2 women are pregnant, we need to calculate the probabilities for 0, 1, and 2 women being pregnant and sum them up:
\(\[P(X \leq 2) = P(X = 0) + P(X = 1) + P(X = 2)\]\)
To find the probability that exactly 6 women are pregnant, we can use the binomial probability formula:
\(\[P(X = 6) = \binom{12}{6} \cdot (0.6)^6 \cdot (0.4)^{12-6}\]\)
Let's calculate this probability:
\(\[\binom{12}{6}\]\) represents the number of ways to choose 6 women out of 12. It can be calculated as:
\(\[\binom{12}{6} = \frac{12!}{6! \cdot (12-6)!} = \frac{12!}{6! \cdot 6!} = 924\]\)
Now, we can substitute this value along with the given probabilities:
\(\[P(X = 6) = 924 \cdot (0.6)^6 \cdot (0.4)^{12-6}\]\)
Evaluating this expression:
\(\[P(X = 6) = 924 \cdot (0.6)^6 \cdot (0.4)^6\]\)
Calculating the values:
\(\[P(X = 6) = 924 \cdot (0.6)^6 \cdot (0.4)^6 = 0.21284004\]\)
Therefore, the probability that exactly 6 women are pregnant is approximately 0.2128, or 21.28%.
d) The mean and standard deviation of a binomial distribution are given by the formulas:
Mean (μ) = \(n * p\)
Standard Deviation (σ) = \(sqrt(n * p * q)\)
To know more about deviation visit-
brainly.com/question/14614117
#SPJ11
Find the measure of CD⎯⎯⎯⎯⎯⎯⎯⎯.
A. 8
B. 6
C. 5
D. 7

Answers
Answer:
Using the property of intersecting secant:-
\((DE)(CE)=(FE)(LE)\)
\((4)(x-1+14)=(5)(x-4+5)\)
\(4(x+3)=(5x+1)\)
\(4x+12=5x+5\)
\(4x+-5x=5-12\)
\(-x=-7\)
\(x=7\)
\(So, CD=x-1=7-1=6\)
\(so,~your ~answer ~is ~B) ~6\)
----------------------------hope it helps...have a great day!!By intersecting secants theorem, the measure of CD is 6 units.
What is intersecting secants theorem?
The theorem states that when two secants intersect at an exterior point, the product of the one whole secant segment and its external segment is equal to the product of the other whole secant segment and its external segment.
For the given situation,
The diagram shows the circle with the two secants intersecting at the exterior point.
By intersecting secants theorem,
\((CE)(DE)=(LE)(FE)\) -------- (1)
Here, CE = CD + DE
⇒ \(CE = (x-1)+4\)
DE = 4
LE = LF + FE
⇒ \(LE = (x-4)+5\)
FE = 5
On substituting the above values in 1,
⇒ \((x-1+4)(4)=(x-4+5)(5)\)
⇒ \((x+3)(4)=(x+1)(5)\)
⇒ \(4x+12=5x+5\)
⇒ \(5x-4x=12-5\)
⇒ \(x=7\)
Thus CD = \(x-1\)
⇒ \(7-1=6\)
Hence we can conclude that by intersecting secants theorem, the measure of CD is 6 units.
Learn more about intersecting secants theorem here
https://brainly.com/question/9853828
#SPJ2
Please help my pick A B C OR D

Answers
the ans is (A) . -2x^3 + 8x^2 - 3.
Answer:
ANSWER IS OPTION A OR -3X^3+8X^2-3
1. When publishing a script from the MATLAB Editor, the resulting document contains section headers derived from the MATLAB code. What is the source of these headers?
a. Section titles(lines starting with %% followed by a space and then a title)
b. An option set in the publishing configuration
c. Command lines that contain no executable code (lines starting with %)
d. Comment lines with the markup HEADER (lines starting with %HEADER)
e. The H1 line
2. Which of the following statements about the purpose of code in the MATLAB Editor is not true?
a. Identify and summarize related blocks of code in a large file.
b. Automatically pause code execution at the start of each section when running a script.
c. Interactively evaluate a single block of code independently.
3. GIven a non scalar matrix X, which commands return a matrix of the same size as x?
a. mean(x)
b. sin(x)
c. log(x)
d. sum(x)
e. std(x)
f. sqrt(x)
Answers
1. (A) Section titles (lines starting with %% followed by a space and then a title).
2. (B) Automatically pause code execution at the start of each section when running a script. (This is not a purpose of code in the MATLAB Editor.)
3. All these functions operate element-wise on each element of the matrix X and return a matrix of the same size.
The source of the section headers in the resulting document when publishing a script from the MATLAB Editor is:
a. Section titles (lines starting with %% followed by a space and then a title).
The statement that is not true about the purpose of code in the MATLAB Editor is:
b. Automatically pause code execution at the start of each section when running a script. (This is not a purpose of code in the MATLAB Editor.)
3. The commands that return a matrix of the same size as x, given a non-scalar matrix X, are:
a. mean(x)
b. sin(x)
c. log(x)
d. sum(x)
e. std(x)
f. sqrt(x)
All these functions operate element-wise on each element of the matrix X and return a matrix of the same size.
To know more about Section titles click here :
https://brainly.com/question/31723428
#SPJ4
Solve for the value of m. Answer: m = (4m-6) (6m+6)° Submit Answer

Answers
Answer:
m = 9
Step-by-step explanation:
The three angles shown all add up together to 180° . The angle in the middle is marked with a little square that means it is 90°. So we can write an equation:
4m-6+90+6m+6=180°
combine like terms.
10m + 90° = 180°
subtract 90
10m = 90
divide by 10
m = 9
Which linear inequality is represented by the graph?
O y > 2x + 2
O yz 2x + 1
Oy> 2x + 1
O y z 2x+2

Answers
By analyzing the graph, we will see that the inequality is:
y > 2x + 1
How to know the inequality represented by the graph?
From a first look, we can see two things.
The line is a dashed line, so the values on the line are not solutions.The shaded region is above that line.From that we can conclude that the inequality is something like:
y > line.
To find the equation of the line, we can look at the graph.
We can see that it intercepts the y-axis at y = 1, and for each increase of one unit in the x-axis increases 2 units on the y-axis, so the line is:
2x + 1
Then the inequality is:
y > 2x + 1.
If you want to learn more about inequalities, you can read:
https://brainly.com/question/11234618
Give one real-world example and one real-world non-example of the Symmetric, Transitive, and Substitution properties.
Answers
The real word examples of Symmetric, Transitive, and Substitution properties are {2 = 1 + 1 ⇒ 1 + 1 = 2} , {4 = 2 + 2 and 2 + 2 = 5 - 1 then 4 = 5 - 1} , { if 4 = 2 + 2 then 8×4 = 8(2+2) } respectively there is no non-example.
What is a number system?A decimal number is a very common number that we use frequently.
Any of the multiple sets of symbols and the guidelines for utilizing them to represent numbers are included in the Number System.
Symmetric property states that if a = b then b = c
For example 2 = 1 + 1 ⇒ 1 + 1 = 2
Transitive property states that if a = b , b = c then a = c
For example 4 = 2 + 2 and 2 + 2 = 5 - 1 then 4 = 5 - 1
Substitution property states that if a = b then we can put b instant of an in any equation.
For example 4 = 2 + 2 then 8×4 = 8(2+2)
Hence "The example of real word on Symmetric, Transitive, and Substitution properties are {2 = 1 + 1 ⇒ 1 + 1 = 2} , {4 = 2 + 2 and 2 + 2 = 5 - 1 then 4 = 5 - 1} , { if 4 = 2 + 2 then 8×4 = 8(2+2) } respectively".
For more about the number system,
https://brainly.com/question/22046046
#SPJ1
Which describes the correlation shown in the scatterplot

Answers
Hope this helps!!
Calculate the forecasted registrations for years 2 through 12 using exponential smoothing, with a smoothing constant ( α ) of 0 . starting forecast of 4.00 for year 1 (round your responses to one decimal place):
Answers
The forecasted registrations for years 2 through 12 using exponential
smoothing, with a smoothing constant (α) of 0 and a starting forecast of 4.00 for year 1 are:
Year | Forecasted registrations
------- | --------
2 | 4.00
3 | 4.00
4 | 4.00
5 | 4.00
6 | 4.00
7 | 4.00
8 | 4.00
9 | 4.00
10 | 4.00
11 | 4.00
12 | 4.00
Exponential smoothing is a forecasting method that uses past data to predict future values. The smoothing constant (α) determines how much weight is given to the most recent data. In this case, we are using a smoothing constant of 0, which means that all of the weight is given to the most recent data. This means that the forecasted registrations for all years will be the same as the starting forecast of 4.00.
Here is the formula for exponential smoothing:
```
Forecasted registrations = α * most recent data + (1 - α) * previous forecast
```
In this case, α = 0, so the formula becomes:
```
Forecasted registrations = most recent data
```
Since the most recent data is always 4.00, the forecasted registrations will always be 4.00.
to learn more about constant click here:
brainly.com/question/33831354
#SPJ11
Which is equal to 1/58^8 ? a.58^-8 b.-1/58^-8 c.58^8 d.1/(58)^-8
Answers
a) 58^-8 is the same as 1/58^8. we can also simplify the expression by applying the rule for negative exponents to the entire fraction.
How to evaluate this expression ?To evaluate this expression, we can use the rule that any number raised to a negative exponent is equal to the reciprocal of the same number raised to the same positive exponent. In other words, x^-n = 1/x^n.
Applying this rule to the expression 1/58^8, we get:
1/58^8 = 1/(58^8) = 58^-8
Therefore, the correct answer is 58^-8.
It is important to note that the exponentiation operator (^) has higher precedence than the division operator (/), so we need to use parentheses to indicate that the exponent should be applied to the entire denominator before the division is performed. In this case, we can also simplify the expression by applying the rule for negative exponents to the entire fraction:
1/58^8 = (1/58)^8 = 58^-8
This simplification shows that the correct answer is still 58^-8.
To learn more about evaluating expression refer :
https://brainly.com/question/28337857
#SPJ1
in terms of a dot product, give a definition of what it means for two vectors in r4 to be orthogonal.
Answers
Two vectors in ℝ⁴ are orthogonal if their dot product is zero.
Two vectors in ℝ⁴ are said to be orthogonal if their dot product is zero. The dot product of two vectors measures the similarity or alignment between them. When the dot product is zero, it signifies that the vectors are perpendicular to each other and do not share any common direction.
Geometrically, orthogonality between two vectors in ℝ⁴ means that they are linearly independent and span different directions in four-dimensional space. It implies that there is no projection of one vector onto the other, and they are completely perpendicular to each other.
The concept of orthogonality is fundamental in many areas of mathematics, physics, and engineering. In linear algebra, orthogonal vectors play a crucial role in defining orthogonal bases and orthogonal projections. They also have applications in vector calculus, where they are used to define gradients and normal vectors. In physics, orthogonal vectors are relevant in studying forces, velocities, and geometric transformations. Overall, understanding orthogonality is essential for analyzing vector relationships and geometric properties in multi-dimensional spaces.
To know more about dot product of vectors, visit:
https://brainly.com/question/33811285
#SPJ11
hector received three a's and one b in his college courses. what is his grade point average?assume each course is three credits. a
Answers
The grade point average received by hector is 3.75.
What is GPA?Your grade point average (GPA) is calculated by dividing the total number of credits you have earned in high school by the sum of all of your course grades. The majority of colleges and secondary schools use a 4.0 scale to report grades. A perfect score, or an A, is a 4.0.
The unit value for each course in which a student obtains one of the grades mentioned above is multiplied by the grade point total for that grade to determine the GPA. Then, divide the sum of these products by the sum of the units. The cumulative GPA is calculated by dividing the total grade points by the total number of units.
3 a and one is B received by Hector.
The A = 4.0, B = 3.0, C = 2.0, D = 1.0 is given by college
We have GPA= A+A+A+B/4
GPA=4+4+4+3/4
GPA= 15/4
GPA=3.75
To learn more about GPA refer here
https://brainly.com/question/1686355#
#SPJ4
Complete question
Hector Ramirez received three A's and one B in his college courses. What is his grade point average? Assume each course is three credits. A = 4.0, B = 3.0, C = 2.0, D = 1.0
The angle measurements in the diagram are represented by the following expressions.

Answers
The angle measurements of angle B as represented in the diagram shown is 150°
What is an equation?An equation is an expression that shows the relationship between two or more numbers and variables.
From the diagram:
∠A = ∠B (alternate interior angles)
Hence:
8x - 10 = 3x + 90
x = 20
∠B = 3(20) + 90 = 150°
The angle measurements of angle B as represented in the diagram shown is 150°
Find out more on equation at: https://brainly.com/question/2972832
#SPJ1
PLZ HELP ASPPP I WILL MARK YOU AS BRAINLIST
If PQ=29 and QR=51 find PR.
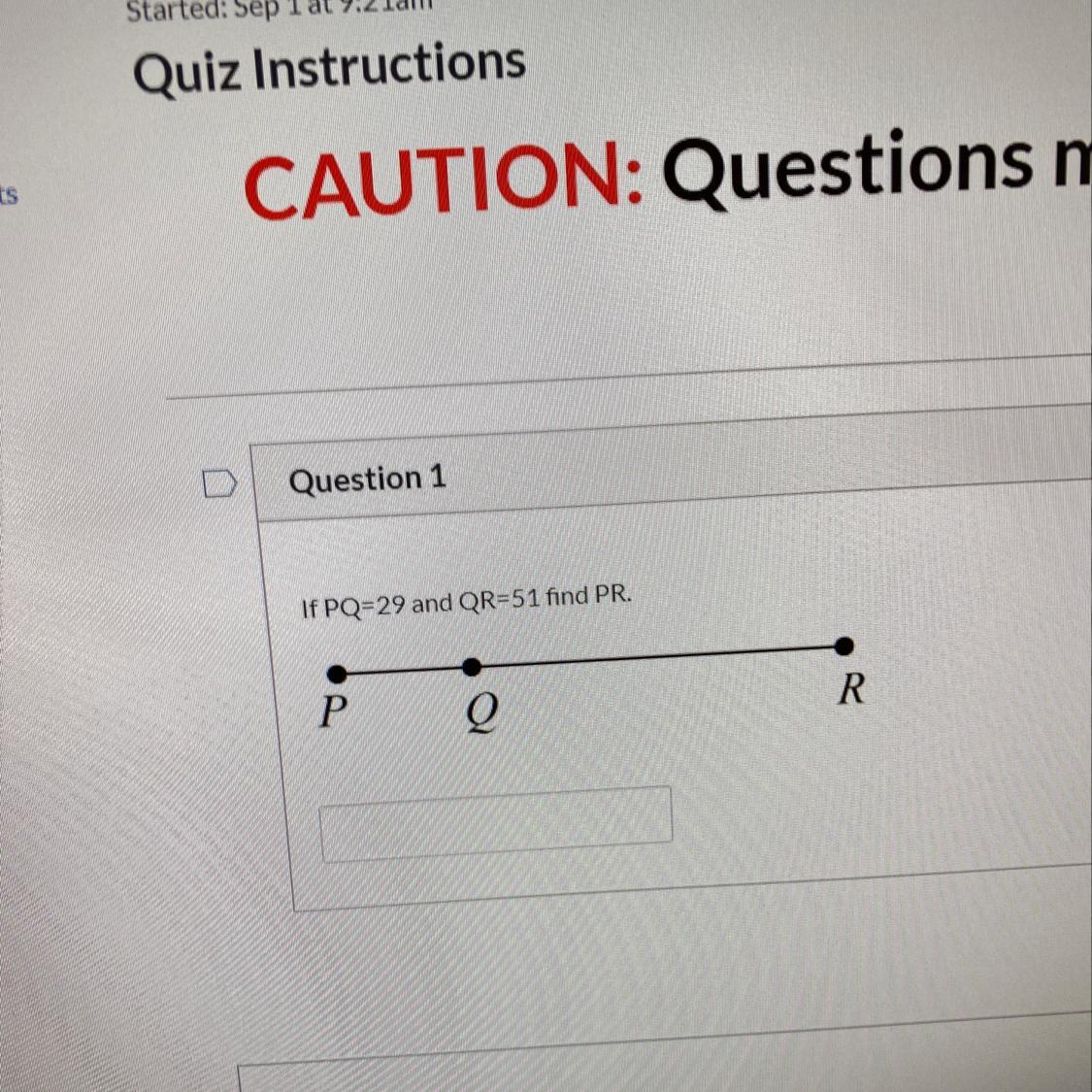
Answers
Roger is training for the upcoming track season and records the number of miles that he runs each day for 20 days: 2.5, 0.5, 3.5, 4, 1.5, 5, 2, 2.5, 0.5, 4, 4.5, 3, 1.5, 1, 0.5, 2.5, 3, 5, 2.5, 0.5, 4, 4.5, 2, 4 which dotplot displays the data correctly? a dotplot titled roger apostrophe s training. a number line labeled miles run goes from 0.5 to 5 in increments of 0.5. 0.5, 4; 1, 1; 1.5, 2; 2, 2; 2.5, 3; 3, 2; 3.5, 0; 4, 3; 4.5, 2; 5, 1. a dotplot titled roger apostrophe s training. a number line labeled miles run goes from 0 to 3.5. 0, 4; 2.5, 3; 4, 3; 1.5, 2; 2, 2; 3, 2; 4.3, 2; 1, 2; 5, 1; 3.5, 0. a dotplot titled roger apostrophe s training. a number line labeled miles run goes from 0 to 5. 0, 4; 1, 1; 1.5, 2; 2, 2; 2.5; 3, 3, 2; 4, 3; 4.5, 2; 5, 1.
Answers
By examining the dotplot, you can see the frequency and distribution of the miles run by Roger. For example, there are 4 instances where Roger ran 0.5 miles, 3 instances where he ran 4 miles, and so on.
The dotplot that displays the data correctly is the one titled "Roger's Training" with a number line labeled "Miles Run" that goes from 0.5 to 5 in increments of 0.5. The dotplot should have the following data points:
0.5, 4
1, 1
1.5, 2
2, 2
2.5, 3
3, 2
3.5, 0
4, 3
4.5, 2
5, 1
This dotplot accurately represents the number of miles Roger ran each day over a 20-day period. Each dot represents a data point from the given list of miles run. The number line indicates the range of miles run, starting from 0.5 and ending at 5, with increments of 0.5.
By examining the dotplot, you can see the frequency and distribution of the miles run by Roger. For example, there are 4 instances where Roger ran 0.5 miles, 3 instances where he ran 4 miles, and so on. This visual representation allows you to easily interpret the data and observe any patterns or trends in Roger's training.
To learn more about dotplot
https://brainly.com/question/15853311
#SPJ11