What type of association does the graph show between x and y? a Linear positive association Nonlinear positive associazion Linear negative association Nonlinear negative association

Answers
Answer:
Linear Negative Association is the answer I believe.
Related Questions
On a map, the beach is 4 inches from downtown Galveston. If the map has a scale of 1 inch = 3.5 miles, what is the actual Distance between downtown and the beach?
Answers
Simplify m7m−4.
please please helpppp
Answers
Answer:
\(m^8-4\)
Step-by-step explanation:
\(Rule: a^b\cdot \:a^c=a^{b+c}\\=m^7m=m^{7+1}\\=m^{7+1}-4\\=m^8-4\)
Solve for x: 2x² - 7x - 30 = 0
Answers
Answer:
x=-10
Step-by-step explanation:
2x²-7x=0+30
2x²-7x=30
4x-7x=30
-3x=30
x=-10
Answer:
Step-by-step explanation:
(2x + 5)(x - 6) = 0
2x + 5 = 0
x = -5/2
x - 6 = 0
x = 6
based on derivative how many times can function cross the x-asix
Answers
Based on the derivative, a function can cross the x-axis a certain number of times depending on the number of times the derivative changes sign.
A derivative is a mathematical tool that measures the rate of change of a function at a specific point. Specifically, it is the slope of the tangent line to the function at that point. The derivative can be used to determine where a function is increasing, decreasing, or reaching a maximum or minimum value.
When a function crosses the x-axis, it means that the y-value of the function is equal to zero. In order for a function to cross the x-axis, it must change sign from positive to negative or vice versa. This happens when the function passes through a maximum or minimum point.
If the derivative of the function is positive at a point, it means that the function is increasing at that point. If the derivative is negative, the function is decreasing. If the derivative is zero, it means that the function is neither increasing nor decreasing, and it could be at a maximum or minimum point.
Therefore, the number of times a function can cross the x-axis depends on the number of times the derivative changes sign. If the derivative changes sign twice, the function can cross the x-axis twice. However, if the derivative changes sign an odd number of times, the function can only cross the x-axis once.
Learn more about derivative here:
https://brainly.com/question/29144258
#SPJ11
What is the domain and range of this function

Answers
Domain (from X-axis):
(-∞, ∞)Range (from Y-axis):
(0, ∞)Simplify the following expressions by factoring using the greatest common factor:
1. 17mn – 5mnx + 15mx
2. 4rst +18rst – 34rst
3. 12ax + 20bx + 32cx
Answers
Answ
Step-by-step explanation:
−42−5i 2y−1(y−2)(y−2)
what are the missing numbers?
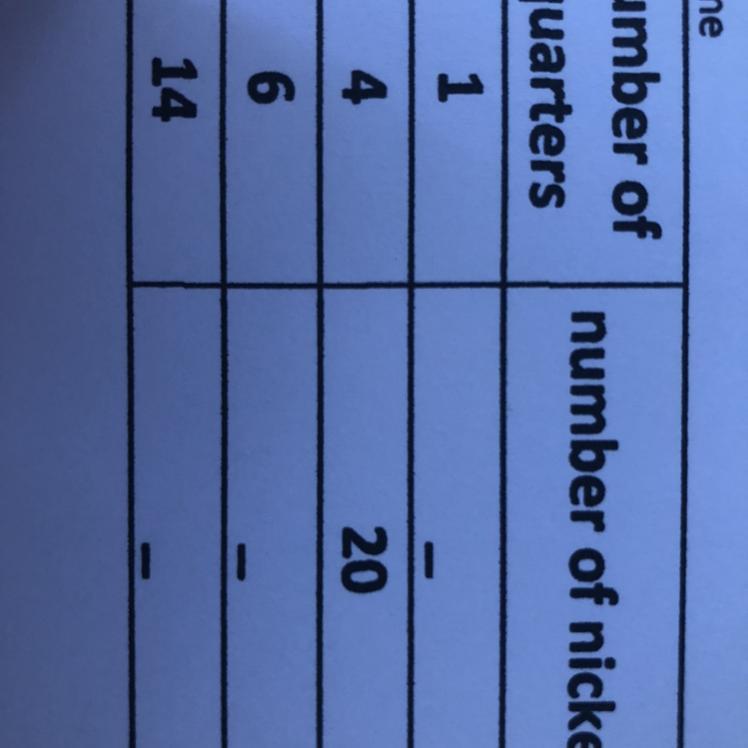
Answers
6Q = 30
14Q= 70
Answer:
5, 30, and 70
Step-by-step explanation:
Multiply by number of quarters by 5 to get the number of nickels.
which theorem or postulate proves that â–³abc and â–³def are similar?
Answers
Angle-Angle (AA) similarity postulate, if two angles of one triangle are congruent to two angles of another, then the triangles are similar.
Similar triangles are the triangles that have corresponding sides in proportion to each other and corresponding angles equal to each other. Similar triangles look the same but the sizes can be different.
As per the diagram,
Triangles RPQ & RST are similar since
∠P = ∠S & ∠Q = ∠T
Side - side - side (SSS) similarity theorem - If the lengths of the corresponding sides of two triangles are proportional, then the triangles are similar.
Triangles RPQ & RST are similar since
RP/RS = RQ/RT = ST/PQ
To know more about similar triangles
https://brainly.com/question/29793437
#SPJ4

Which of the following functions represents a linear function?

Answers
Answer:
The top and the bottom
Step-by-step explanation:
Answer:
#3, the one with the straight line --> linear bc its a constant slope
Find the unit tangent vector t(t) at the point with the given value of the parameter t. r(t) = 4 t i 3t2 j 3t k, t = 1
Answers
The unit tangent vector t(1) at t = 1 is:
t(1) = (4 i + 6 j + 3 k) /\(\sqrt{(61)}\)
To find the unit tangent vector,
we need to calculate the derivative of the position vector r(t) with respect to the parameter t, and then normalize the resulting vector.
Given the position vector r(t) = 4t i + 3t^2 j + 3t k,
we can find the derivative:
r'(t) = d(r(t))/dt
= 4 i + 6t j + 3 k
Now, we can evaluate r'(t) at t = 1:
r'(1) = 4 i + 6(1) j + 3 k
= 4 i + 6 j + 3 k
To obtain the unit tangent vector,
we need to normalize r'(1):
t(1) = r'(1) / ||r'(1)||
where ||r'(1)|| represents the magnitude or length of r'(1).
The magnitude of r'(1) is given by:
||r'(1)|| = \(\sqrt{((4^2) + (6^2) + (3^2))}\)
= \(\sqrt{61}\))
This is the normalized vector representing the direction of the tangent to the curve at the point where t = 1.
Learn more about Vectors here:
https://brainly.com/question/31900604
#SPJ11
Find g(x) if g(x) is the resulting function from moving f(x) = (x + 2) right 1 unit and up 6 units.
Answers
g(x) = (x+2-3) + 4 = (x-1)+4
Please help me this is my last question.

Answers
Answer:
\(y = \frac{5}{4}x\)
Step-by-step explanation:
1. The slope-intercept form of a linear equation is y = mx + b! Y is the y-coordinate, is the slope, x is the x-coordinate, and b is the y-intercept.
2. To find the slope, let's take any two points from the line, such as (0,0) and (400,500), and plug their values into this formula: \(\frac{y_2-y_1}{x_2-x_1}\)
\(\frac{500-0}{400-0}\) \(\frac{500}{400}\) \(\frac{5}{4}\)3. The slope is 5/4, so the equations looks like this so far: \(y=\frac{5}{4}x + 0\)
4. Because b is the y-intercept, b = 0 because the line intersects with the y-axis at (0,0).
5. Now that we have our slope (5/4) and y-intercept (0), we plug in those values to the slope-intercept form and get the equation of: \(y= \frac{5}{4}x\)
Rendell cycles 42 km at an average speed of 18 km/hr. Find the time taken, giving your
answer as a fraction of an hour in its simplest form.
Answers
Answer:
2.3333333333333333333333333333333 sec
HELP THIS IS DUE TODAY

Answers
Answer:
Can you add a closer photo?
Step-by-step explanation:
im sure i can answer if u do so
9 is subtracted by the sqaure of a number
Answers
Answer:
I believe it is x^2-9
Step-by-step explanation:
Since we don't have a specific "number" you use x. It says it's squared to put an exponent of 2. It also says that 9 is subtracted by it so put -9.
M is the midpoint of RT. RM = 5x + 9 and MT = 8x - 36. Find x, RM, MT, and RT. = .
Answers

The values of x, RM, RT, and MT are 15, 84, 168, and 84 respectively.
What is a System of equations?simultaneous equations, a system of equations Two or more equations in algebra must be solved jointly (i.e., the solution must satisfy all the equations in the system). The number of equations must match the number of unknowns for a system to have a singular solution.
There are four methods for solving systems of equations: graphing, substitution, elimination, and matrices.
Given a system of equations Where RM = 5x + 9 and MT = 8x - 36.
Since,
M is the midpoint of RT
Thus,
RM = MT
5x + 9 = 8x - 36
3x = 45
x = 15
So,
1) RM = 5x + 9
RM = 75 + 9 = 84
2) MT = 8x -36
MT = 120 - 36 = 84
3) RT = RM + MT
RT = 84 +84 = 168
Therefore, The values of x, RM, RT, and MT are 15, 84, 168, and 84 respectively.
Learn more about the System of equations here:
https://brainly.com/question/12628931
#SPJ5
Ojos del Salado is the highest mountain in Chile, with a peak at about 6900 meters above sea level. The Atacama Trench, just off the coast of Peru and Chile, is about 8100 meters below sea level (at its lowest point).
a. What is the difference in elevations between Mount Ojos del Salado and the Atacama Trench?
b. Is the elevation halfway between the peak of Mount Ojos del Salado and the Atacama Trench above sea level or below sea level?
Explain without calculating the exact value. c. What elevation is halfway between the peak of Mount Ojos del Salado and the Atacama Trench?

Answers
A) add the two elevations together:
6900 + 8100 = 15,000 meters.
B) Divide the total distance by 2:
15,000 / 2 = 7,500 meters.
Because the distance to the halfway point is larger than the elevation of Ojos del Salado, the elevation would be below seal level.
C Add the halfway point from part B to the lowest elevation:
-8100 + 7500 = -600 meters
Kate is a buyer for a men’s fashion retail store. She will order a new cloth overcoat from Paris for the fall fashion season. Based on her experience, she expects to sell at least 100 coats, and at most 400, but she feels that any number of sales in between is equally likely. Therefore, she estimates that her sales are uniformly distributed between 100 and 400. The total cost to the store is $100 per coat, and the retail price is set at $180. Any coats left over at the end of season would be sold at $60 each.
part 1: a) How many coats should Kate buy if she wants to maximize profits?
part 2: b) Assume Kate buys the number of coats suggested in part a), what is the probability that the coats sell out? What is the probability that they do not sell out?
Answers
Part 1: Kate should buy 100 coats to maximize profits.Part 2: The probability that the coats sell out is 0.25 (25%), and the probability that they do not sell out is 0.75 (75%).
To maximize profits, Kate should consider the scenario where she sells all the coats without any left over at the end of the season.
Since the sales are uniformly distributed between 100 and 400, buying 100 coats ensures that she meets the minimum expected sales of 100. Purchasing more than 100 coats would increase costs without a guarantee of higher sales, potentially leading to excess inventory and lower profits.
Given that the sales are uniformly distributed between 100 and 400 coats, Kate's purchase of 100 coats covers the minimum expected sales.
The probability of selling out can be calculated by finding the proportion of the range covered by the desired sales (100 out of 300). Therefore, the probability of selling out is 100/300 = 0.25 or 25%. The probability of not selling out is the complement, which is 1 - 0.25 = 0.75 or 75%.
learn more about probability here:
https://brainly.com/question/31828911
#SPJ11
A stock decreases $11 a day for 5 days. Which integer represents the total decrease?
Group of answer choices
55
-16
16
-55
Answers
Answer:-55
Step-by-step explanation:
-11 times 5= -55
a theater charges $8 for an adult ticket and $6 for a childerns ticket on a certin day a total of 225 tickets were sold for a total cost of $1,850 how many more childerns tickets were sold than sold than adult tickets
Answers
The number of adult tickets sold more than the children's tickets are 65.
It is given in the question that the charge of an adult ticket and a children's ticket is $8 and $6 respectively.
It is also given that, on a certain day the number of tickets sold are 255 at a total cost of $1,850.
We have to find the number by which children's ticket were sold more than adult tickets.
Let the number of children tickets sold on that particular day be x.
Let the number of adult tickets sold on that particular day be y.
Hence, according to the question,
x + y = 255 ...(1)
Also,
6*x + 8*y = 1850
Dividing 2 from both sides, we get
3x + 4y = 925 ...(2)
Multiplying (1) by 3, we get
3x + 3y = 765 ...(3)
(2) - (3)
(3x - 3x) + (4y - 3y) = 925 - 765
0 + y = 160
y = 160
Hence, the number of adult tickets sold are 160.
We know that,
x + y = 255
Hence,
x + 160 = 255
x = 255 - 160
x = 95
Hence, the number of children's tickets sold are 95.
The number of adult tickets sold more than the children's ticket are =
160 - 95 = 65 tickets.
thanks if you can help or just try it's appreciated.

Answers
Answer:
a l parallel n alt angle
b j parallel k corresponding angle
i have a deck of cards, and i deal all of the cards to players, with each player getting cards. if is at least and is at least , then how many possible values of are there?
Answers
Answer:
4
Step-by-step explanation:
We want xy=54=2*3^3 such that x is at least 2 and y is at least 5. Thus, the possible combinations (x,y) are (2,27), (3,18), (6,9), and (9,6). There are 4 such combinations.
Solve the simultaneous equation.

Answers
The value of a = -2 and b = 3.
What is a system of equations?
A finite set of equations for which common solutions are sought is referred to in mathematics as a set of simultaneous equations, often known as a system of equations or an equation system.
Here, we have
Given: 3a - 5b = -26, a + 2b = 6
We have to solve this equation.
3a - 5b = -26...(1)
a + 2b = 6...(2)
Now we multiply equation (1) by 2 and equation (2) by 5 and we get
6a - 10b = -52....(3)
5a + 10b = 30....(4)
After solving equations (3) and (4), we get
11a = -22
a = -2
Now we put the value of 'a' in equation(3) and we get
6(-2) - 10b = -52
-10b = -52 + 12
-10b = -30
b = 3
Hence, the value of a = -2 and b = 3.
To learn more about the system of equations from the given link
https://brainly.com/question/25976025
#SPJ1
will u simplify -164
Answers
Answer:
No, I will not simplify -164
Step-by-step explanation:
You can not Simplify -164
so the answer will be you can not simplify it
8- (-3) = please help me

Answers
Answer:
11
Step-by-step explanation:
you are subtracting a negative number, therefore you are adding the number
Answer:
The answer is 11.
Step-by-step explanation:
Two negatives equal a positive, so it would be 8+3=11.
help please ASAP please

Answers
Answer:
0
Step-by-step explanation:
So for the first number 5/6. You can round to 1. And for 2/3 it is also close to 1 so you can round to 1.
The answer is near 0.
Does this help?
I also need help on this one please help

Answers
Answer: blue
Step-by-step explanation:
or b
Find f[g(x)] and g[f(x)] for the given functions. 3 f(x) = -x³ +3, g(x) = 4x+7 (Simplify your answer. Do not factor.) (Simplify your answer. Do not factor.) f[g(x)] = g[f(x)] =
Answers
The value of f[g(x)] is - 64x³ - 336x² - 588x - 340 and the value of g[f(x)] is -4x³ + 19
The functions are as follows; f(x) = -x³ +3 and g(x) = 4x+7
The value of f[g(x)] is obtained by replacing every x in f(x) with the value of g(x) as given below
f[g(x)] = f(4x + 7) = - (4x + 7)³ + 3
When we expand (4x + 7)³, it gives us 64x³ + 336x² + 588x + 343
Then
f[g(x)] = - 64x³ - 336x² - 588x - 340
Similarly, g[f(x)] is obtained by replacing every x in g(x) with the value of f(x) as shown below;
g[f(x)] = g(-x³ + 3) = 4(-x³ + 3) + 7g
[f(x)] = -4x³ + 19
Therefore,
f[g(x)] = - 64x³ - 336x² - 588x - 340
g[f(x)] = -4x³ + 19
Learn more about the function at
https://brainly.com/question/32520165
#SPJ11
Use the diagram to the right.
If 17, what theorem can you use to show that ?

Answers
Answer:
its c
Step-by-step explanation:
dont ask why to much to type
Please help 50 points!

Answers
d=10
Answer:
Solution given:
\(\sqrt{25+3x}=d+2x\)
squaring both side
25+3x=(d+2x)²
when x=-3
25+3*-3=(d+2*-3)²
25-9=(d-6)²
16=(d-6)²
\(\sqrt{16}=d-6\)
4=d-6
d=4-6
d=10
Answer:
d = 6
Step-by-step explanation:
\( \sqrt{25 + 3( - 3)} = d + 2( - 3) \\ 4 = d - 6 \\ d = 10\)
hopefully this makes sense
:)