Which equation represents a function?
Responses
A y = ±2x + 3y = ±2x + 3
B x2
+ y2
= 16x 2 + y 2 = 16
C y = ±3x+8−−−−−√
y = ± 3 x + 8
D y2
= -5x - 8y 2 = -5x - 8
E x = 4y + 7
Answers
Option (A) (y = ±2x + 3) represents a function. In this equation, for every value of x, there is only one corresponding value of y, which satisfies the equation.
A function is a relation between two variables where each input is associated with exactly one output. To determine if an equation represents a function, we can use the vertical line test.
Out of the given options, only equation A (y = ±2x + 3) represents a function. In this equation, for every value of x, there is only one corresponding value of y, which satisfies the equation. When plotted on a coordinate plane, a vertical line will intersect the graph at most once for every value of x.
Equations B\((x^2 + y^2 = 16)\), C\((y = \pm3x + 8)\), D \((y^2 = -5x - 8)\), and E \((x = 4y + 7)\) do not represent functions. In these equations, a vertical line will intersect the graph more than once for some values of x, meaning that there are multiple outputs for a single input, which violates the definition of a function.
Learn more about vertical line test :
https://brainly.com/question/29263148
#SPJ4
Related Questions
Find two positive numbers so that twice their sum equals their product and one number is 10 times the other number. Enter the smaller number first.
Answers
Answer:
Step-by-step explanation:
We'll call the 2 numbers x and y. Starting with the last part of that first sentence "one number is 10 times the other number" can be written, in algebraic form:
y = 10x
Now on to the first statement about the numbers: "twice their sum" is 2(x + y) and "equals their product" is = xy. Putting that all together:
2(x + y) = xy and we know that y = 10x so
2(x + 10x) = x(10x) and
\(2(11x)=10x^2\) and
\(10x^2-22x=0\) and
x(10x - 22) = 0 so
x = 0 or 10x - 22 = 0 which makes
x equal to \(\frac{22}{10}=2.2\)
So x = 2.2 and y = 22.
in symbolizing truth-functional claims, the word "if" used alone introduces the consequent of a condition. "only if" represents the antecedent.
Answers
In symbolizing truth-functional claims, the word "if" is used to introduce the consequent of a condition, while the phrase "only if" represents the antecedent.
Symbolizing truth-functional claims involves representing statements or propositions using logical symbols. When using the word "if" in a truth-functional claim, it typically introduces the consequent of a conditional statement. A conditional statement is a type of proposition that states that if one thing (the antecedent) is true, then another thing (the consequent) is also true. For example, the statement "If it is raining, then the ground is wet" can be symbolized as "p → q," where p represents "it is raining" and q represents "the ground is wet."
On the other hand, the phrase "only if" is used to represent the antecedent in a truth-functional claim. In a conditional statement using "only if," it states that if the consequent is true, then the antecedent must also be true. For example, the statement "The ground is wet only if it is raining" can be symbolized as "q → p," where p represents "it is raining" and q represents "the ground is wet."
In summary, when symbolizing truth-functional claims, the word "if" introduces the consequent of a condition, while the phrase "only if" represents the antecedent. These terms help express the relationships between propositions in logical statements.
Learn more about symbols here: https://brainly.com/question/30763849
#SPJ11
There are 6 teams in the basketball
league. Each team has 8 players. How
many players are there?
Answers
Answer:
48
Step-by-step explanation:
8 players for every team: 6x8=48.
Answer:
48 players
Step-by-step explanation:
There are 6 teams
There are 8 players per team
You can multiply them: 6 x 8 = 48
1 team = 82 teams = 8 + 8 = 163 teams = 16 + 8 = 244 teams = 24 + 8 = 325 teams = 32 + 8 = 406 teams = 40 + 8 = 48There are 48 players
-Chetan K
What is the standard deviation around the regression line?.
Answers
Answer:
The standard deviation of the residuals calculates how much the data points spread around the regression line. The result is used to measure the error of the regression line's predictability.
Step-by-step explanation:
How do you find the standard deviation around the regression line?STDEV. S(errors) = (SQRT(1 minus R-squared)) x STDEV. S(Y). So, if you know the standard deviation of Y, and you know the correlation between Y and X, you can figure out what the standard deviation of the errors would be be if you regressed Y on X.
What does standard deviation tell you?A standard deviation (or σ) is a measure of how dispersed the data is in relation to the mean. Low standard deviation means data are clustered around the mean, and high standard deviation indicates data are more spread out.
how many seating arrangements are possible if fred (a boy) and carrie (a girl) must be seated next to each other?
Answers
(n-1) x (n-2)! ways of seating arrangements are possible if Fred (a boy) and Carrie (a girl) must be seated next to each other.
A permutation is the act of putting things or numbers in a certain order. Combinations are a method of picking things or numbers from a set of objects or collections without regard for their order.
Let's say there are n seats in total.
Then, there are (n-1) gaps between the seats that Fred and Carrie can be placed in.
If we place Fred and Carrie in one of these gaps, there will be (n-2)! possible arrangements for the rest of the seats.
Therefore, there will be (n-1) x (n-2)! total possible seating arrangements for Fred and Carrie if they must be seated next to each other.
For more questions on Permutations and Combinations
https://brainly.com/question/28065038
#SPJ4
Nick worked 16 hours last week. He earned $5 per hour at a local restaurant and $5.50 per hour at a grocery store. If he earned a total of $82, how many hours did he work at the grocery store?
Answers
Answer:
4 hours in the grocery store
Step-by-step explanation:
x for restaurant, y for grocery store
\(\left \{ {{5x+5.5y=82} \atop {x+y=16}} \right.\)
\(\left \{ {{5x+5.5y=82} \atop {x=16-y}} \right.\)
Substitute.
- 5y + 5.5y + 80 = 82
0.5y = 2
y = 4
x + 4 = 16
x = 12
negative five divided by five sevenths
Answers
Answer:
-7
Step-by-step explanation:
-5 ÷ 5/7
⇒ -5 × 7/5
⇒ -7
hope this helps !
pls mark as brainliest :))
\(\\ \sf\longmapsto -5\div \dfrac{5}{7}\)
\(\\ \sf\longmapsto -5\times \dfrac{7}{5}\)
\(\\ \sf\longmapsto -1(7)\)
\(\\ \sf\longmapsto -7\)
If P(A) = .46 and P(B) = .17 and P(A U B) = .63, then A and B are:
Select one:
a. mutually exclusive
b. collectively exhaustive
c. statistically independent
d. mutually exclusive and collectively exhaustive
e. none of the above/can’t be determined with info given
Answers
The answer is e. none of the above/can’t be determined with info given.
Based on the information given, we have:
P(A) = 0.46
P(B) = 0.17
P(A U B) = 0.63
Note that P(A U B) represents the probability of either event A or event B occurring, or both.
If events A and B are mutually exclusive, it means they cannot occur at the same time. In other words, if event A occurs, event B cannot occur, and vice versa. In this case, the probability of both events occurring would be zero.
On the other hand, if events A and B are collectively exhaustive, it means that together they account for all possible outcomes. In other words, either event A or event B (or both) must occur, and there are no other possibilities.
Using these definitions, we can see that events A and B are neither mutually exclusive nor collectively exhaustive. This is because the probability of both events occurring (i.e., the intersection of A and B) is not zero, which means they are not mutually exclusive. Additionally, the probability of either A or B occurring (i.e., the union of A and B) is not equal to one, which means they are not collectively exhaustive.
Know more about probability here:
https://brainly.com/question/31828911
#SPJ11
f(t)=e5t+4t+7ln(t2+3c)+te-1+5e6
where c is constant
Answers
The function f(t) is defined as e raised to the power 5t plus 4t plus the natural logarithm of the quantity t squared plus 3 times the constant c, raised to the power of 7, plus t times e raised to the power of -1 plus 5 times e raised to the power of 6.
Given a function:
f(t)=e5t+4t+7ln(t2+3c)+te-1+5e6
where c is a constant.
The solution to the question is shown below.
Step 1: We have given a function:
f(t)=e5t+4t+7ln(t2+3c)+te-1+5e6
We have to find the number of words we have to write to express this function in words.
Step 2: Solution
f(t) = et5+4t + ln(t²+3c)⁷ +te-1+5e⁶
Where,
et5+4t = exponential function
ln(t²+3c)⁷ = natural logarithmic function
te-1 = linear function
e⁶ = exponential function
Therefore, f(t) can be expressed in words as:
The function f(t) is defined as e raised to the power 5t plus 4t plus the natural logarithm of the quantity t squared plus 3 times the constant c, raised to the power of 7, plus t times e raised to the power of -1 plus 5 times e raised to the power of 6.
Step 3: Conclusion
Hence, the function f(t) can be expressed in words with:The function f(t) is defined as e raised to the power 5t plus 4t plus the natural logarithm of the quantity t squared plus 3 times the constant c, raised to the power of 7, plus t times e raised to the power of -1 plus 5 times e raised to the power of 6.
To know more about natural logarithm visit:
https://brainly.com/question/29154694
#SPJ11
PQ= RQ and PS= RS a=?

Answers
The measure of angle a is 15 degrees and this can be determined by using the properties of the isosceles triangle.
What are interior angles?In geometry, interior angles are formed in two ways. One is inside a polygon, and the other is when parallel lines cut by a transversal. Angles are categorized into different types based on their measurements.
Given:
The length of the segment PQ is equal to the length of the segment RQ.The length of the segment PS is equal to the length of the segment RS.The following steps can be used in order to determine the measure of angle a:
Step 1 - According to the given data, it can be concluded that triangle PQR and triangle PSR are isosceles triangles.
Step 2 - Apply the sum of interior angle property on triangle PQR.
\(\angle\text{Q}+\angle\text{P}+\angle\text{R}=180\)
\(\angle\text{Q}+2\angle\text{R}=180\)
\(2\angle\text{R}=180-60\)
\(\angle\text{R}=60^\circ\)
Step 3 - Now, apply the sum of interior angle property on triangle PSR.
\(\angle\text{P}+\angle\text{S}+\angle\text{R}=180\)
\(\angle\text{S}+2\angle\text{R}=180\)
\(2\angle\text{R}=180-90\)
\(\angle\text{R}=45^\circ\)
Step 4 - Now, the measure of angle a is calculated as:
\(\angle\text{a}=60-45\)
\(\angle\text{a}=15\)
The measure of angle a is 15 degrees.
For more information on interior angles, refer to the link given below:
https://brainly.com/question/28795639
PLEASE ILL MARK BRAINLIEST!!!! Point P on the number line shows Lara's score after the first round of a quiz:
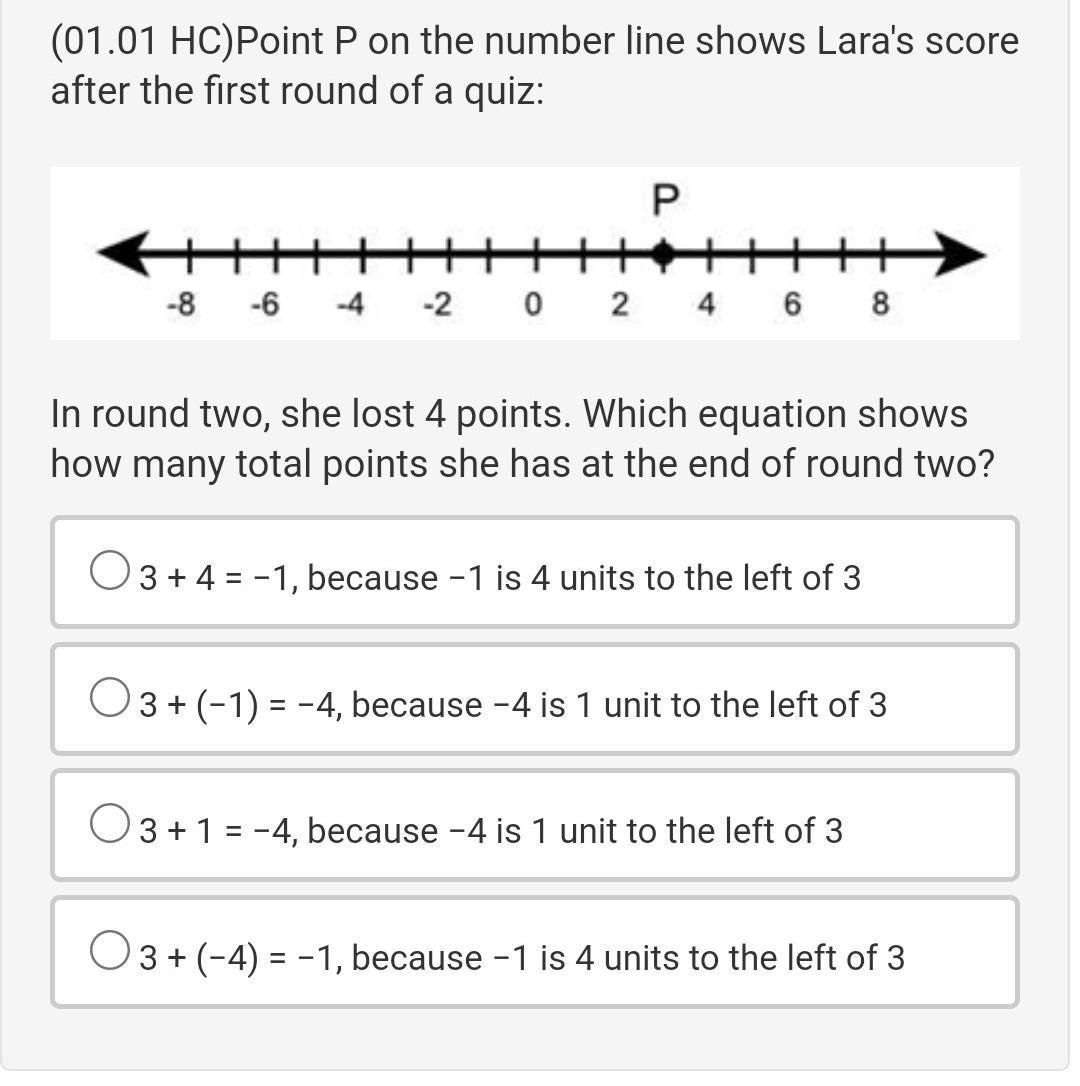
Answers
Sarah is walking diagonally across a park as modeled by the equation y = x − 1. Suppose Marcus starts walking at the point (0, 9) so his path is perpendicular to Sarah's. At what point will Sarah's path and Marcus's path intersect?
Answers
Answer:
\(A(10,9)\)
Step-by-step explanation:
Consider the attached figure.
The equation is \(y=x-1\)
At \(x=0;y=0-1=-1\)
At \(y=0;0=x-1,x=1\)
Plot points \((0,-1)\,,\,(1,0)\).
Marcus starts walking at the point B(0, 9) so that his path is perpendicular to Sarah's at point A.
From the graph, Sarah's path and Marcus's path intersect at point \(A(10,9)\)
i don’t understand how to do this

Answers
Answer:
There is only 1 solution where x is greater than 3 that is on the line and that is where the line Crosse where x is 4.
Step-by-step explanation:
p(x>3) = p(x= 5) - p(x= 3)
= (Area of ∆ from x= 0 to x= 5)- (Area of ∆ from x= 0 to x= 3)
=[ 1/2•(5-0)•0.4] -[1/2•(3-0)• 0.4)]
= 1/2•0.4(5-3)
p(x>3) = 0.4
Evaluate the integral using integration by parts with the given choices of u and dv. (Use C for the constant of integration.) ∫ x cos 4x dx; u =x,dv = cos 4x dx
Answers
The integration is (1/4)x sin 4x + (1/16)cos 4x + C.
To evaluate the integral ∫ x cos 4x dx using integration by parts, we need to use the formula ∫u dv = uv - ∫v du. We are given that u = x and dv = cos 4x dx. Now, we need to find v and du.
To find v, we need to integrate dv. So, v = ∫cos 4x dx = (1/4)sin 4x + C.
To find du, we need to differentiate u. So, du = dx.
Now, we can plug in the values of u, v, du, and dv into the formula and simplify:
∫ x cos 4x dx = x(1/4)sin 4x - ∫(1/4)sin 4x dx
= (1/4)x sin 4x - (1/4)∫sin 4x dx
= (1/4)x sin 4x - (1/4)(-1/4)cos 4x + C
= (1/4)x sin 4x + (1/16)cos 4x + C
So, the final answer is (1/4)x sin 4x + (1/16)cos 4x + C.
To know more about integration click on below link:
https://brainly.com/question/18125359#
#SPJ11
Renaldo has a
budget of $500 to buy gift boxes for a party.
Large boxes cost $65 and small boxes cost
$35. Write and graph an inequality that
represents the number of each type of gift
box that Renaldo can buy. If Renaldo buys
5 small gift boxes, how many large gift boxes
can he afford to buy?
Answers
Renal can buy a box that is $35
Step-by-step explanation:
The inequality is 35 × 5 + 65y ≤ 500. Then the number of large gift boxes will be 5.
What is inequality?Inequality is defined as an equation that does not contain an equal sign. Inequality is a term that describes a statement's relative size and can be used to compare these two claims.
Renaldo has a budget of $500 to buy gift boxes for a party. Large boxes cost $65 and small boxes cost $35.
Let 'x' be the number of small boxes and 'y' be the number of large boxes. Then the inequality is written as,
35x + 65y ≤ 500
If Renaldo buys 5 small gift boxes. Then the number of large gift boxes will be given as,
35 × 5 + 65y ≤ 500
175 + 65y ≤ 500
65y ≤ 325
y ≤ 5
The inequality is 35 × 5 + 65y ≤ 500. Then the number of large gift boxes will be 5.
More about the inequality link is given below.
https://brainly.com/question/19491153
#SPJ2

a survey asked people if they were aware that maintaining a healthy weight could reduce the risk of stroke. a 95% confidence interval was found using the survey results to be (0.54, 0.62). what is the correct interpretation of this interval?
Answers
Keeping a healthy weight will lower your risk of stroke, according to the 95% confidence interval of (0.54, 0.62), which suggests that if the survey were conducted repeatedly, 95% of the intervals produced would represent the real population percentage of individual, who are aware of this.
In other words, there is a 95% confidence interval between 0.54 and 0.62 for the genuine population fraction of those who are aware of this knowledge.
It should not be assumed from this range that 95% of respondents indicated that they were aware of this knowledge.
Instead, it offers a range of numbers that, with a given level of certainty, are likely to include thee genuine population percentage.
To know more about Percentage
https://brainly.com/question/24877689
#SPJ4
Let f(x) = cx3 and Sx = [0, 2] (X is continuous).a. What value of c will make f(x) a valid density?
Answers
The value of c that makes f(x) a valid density function over [0, 2] is c = 1/4.
The function given to us is f(x) = cx, and we are asked to find the value of c that makes this a valid density function over the interval [0, 2]. To be a valid density function, a function must satisfy two properties:
The function must be non-negative over the interval.
The integral of the function over the interval must be equal to 1.
Let's first check the first property. For f(x) to be non-negative over the interval [0, 2], c must be non-negative as well. This is because x³ is always non-negative, and multiplying it by a negative value of c would make f(x) negative for some values of x.
So, we can conclude that c ≥ 0.
Now let's check the second property. We need to find the value of c that makes the integral of f(x) over [0, 2] equal to 1:
∫₀² cx³ dx = 1
We can solve this integral using the power rule of integration:
(c/4) [x⁴]₀² = 1
(c/4) (2⁴ - 0⁴) = 1
16c/4 = 1
4c = 1
c = 1/4
To know more about function here.
https://brainly.com/question/28193995
#SPJ4
A toy is accidentally dropped by a kid from his roof. The final velocity of the toy before it reached the ground was 8m/s. Find the height of the building. please solve this from which I could understand easily
Answers
The height of the building is 3.27 m
Since the question described free-fall under gravity, we will be using the equation for fall under gravity.
Using v² = u² - 2gh where u = initial velocity of toy = 0 m/s (since it is falls from rest), v = final velocity of toy as it hits the ground = 8 m/s, g = acceleration due to gravity = 9.8 m/s² and h = distance the ball falls = height of building
So, making h subject of the formula, we have
h = -(v² - u²)/2g
Substituting the values of the variables into the equation, we have
h = -(v² - u²)/2g
h = -[(8 m/s)² - (0 m/s)²)/(2 × 9.8 m/s²)
h = -(64 m²/s² - 0 m²/s²)/(2 × 9.8 m/s²)
h = -64 m²/s²/19.6 m/s²
h = -3.265 m
h ≅ -3.27 m
The value is negative since we take the top of the building as 0 m and downward direction as negative.
So, the height of the building is 3.27 m
Learn more about free fall here:
https://brainly.com/question/23180622
For what value(s) of α, if any, will lim t→[infinity] y(t) exist for the following initial value problem? y^ first derivative−3y = e^−2t, y(0) = α
Answers
The limit of y(t) as t approaches infinity exists if and only if the coefficient α of the e^(3t) term is nonzero.
To find the value(s) of α for which the limit exists as t approaches infinity, we need to solve the differential equation and then analyze the behavior of the solution as t goes to infinity.
The differential equation is:
y'(t) - 3y(t) = e^(-2t)
To solve this differential equation, we first find the homogeneous solution by setting the right-hand side to zero:
y'(t) - 3y(t) = 0
The characteristic equation is:
r - 3 = 0
which has the solution r = 3. Therefore, the homogeneous solution is:
y_h(t) = c e^(3t)
where c is a constant determined by the initial condition:
y(0) = α
Substituting t = 0 and y(0) = α into the equation for the homogeneous solution, we get:
y_h(0) = c = α
So, the homogeneous solution is:
y_h(t) = α e^(3t)
To find a particular solution to the nonhomogeneous equation, we use the method of undetermined coefficients and assume that the particular solution has the form:
y_p(t) = A e^(-2t)
where A is a constant to be determined. Substituting this into the nonhomogeneous equation, we get:
-2A e^(-2t) - 3A e^(-2t) = e^(-2t)
Solving for A, we get:
A = -1/5
Therefore, the particular solution is:
y_p(t) = (-1/5) e^(-2t)
The general solution to the differential equation is:
y(t) = y_h(t) + y_p(t) = α e^(3t) - (1/5) e^(-2t)
As t approaches infinity, the term e^(-2t) goes to zero faster than the term e^(3t), so the behavior of the solution is dominated by the term α e^(3t). Therefore, the limit of y(t) as t approaches infinity exists if and only if the coefficient α of the e^(3t) term is nonzero. In other words, the limit exists for all α ≠ 0.
Learn more about differential equation here
brainly.com/question/14620493
#SPJ4
Jay makes a base salary of $2000 per month. Once he reaches $50000 in total sales, he earns an additional 5% commission (salary) on the amount of sales over $50000. Let s be the amount of his total sales for the month and j(s) be the amount of his salary for the month.
Answers
Answer:
\(j(s) = 0.05s -500\)
Step-by-step explanation:
Given
\(Base\ Salary = 2000\)
\(Commission = 5\%\) on Sales over 50000
Required
Determine the function j(s) for sales over 50000
Represent the total sales in a month with s.
Sales over 50000 in that month will be: s - 50000
So, the function j(s) is:
j(s) = Base Amount + Commission * Sales over 50000
\(j(s) = 2000 + 5\% * (s - 50000)\)
Convert % to decimal
\(j(s) = 2000 + 0.05 * (s - 50000)\)
Open bracket
\(j(s) = 2000 + 0.05 * s - 0.05 * 50000\)
\(j(s) = 2000 + 0.05s - 2500\)
Collect Like Terms
\(j(s) = 0.05s - 2500 + 2000\)
\(j(s) = 0.05s -500\)
ASAP HELpppppppppppppppppppppppppppppppppppppppppppppp

Answers
Answer:
5
This is essentially the third identical question that you posted with the same
type of solution ... if you look at the previous question you asked and the explanation.. you will see that the red line crosses a corner of a box at (4,20)
4 cups = 20 pralines thus the number you are looking for is 20/4 = 5
Step-by-step explanation:
lets try this "story" to explain...
assume that you can walk 3 miles in 1 hour
this means that at 0 hr you go 0 miles , 1 hr you go 3 miles etc
0,0
1,3
2,6
3,9
etc.
if you graph that you will have the red line in you graph, and the x values would be 0,1,2,3 with the y values being 0,3,6,9....
if you DIVIDE any of those points
3/1 ,6/2, 9,3 the result is always a "3" that is the speed that you are walking at
it is a CONSTANT (in this problem) ... note: if the speed is NOT constant then this basically becomes a calculus problem, ant an algebra one.
describe some of the units of measurement you see on this food label. what does each unit measure? what other information do you see?
Answers
The units of measurement you see on a food label vary depending on the nutrient being measured. Common units of measurement include grams (g), milligrams (mg), micrograms (mcg), kilocalories (kcal), and daily values (%DV).
Grams (g) are the most common unit of measurement for food labels. They are used to measure the weight of a food item. For example, a serving of cereal might be 1 cup (240 g).
Milligrams (mg) are used to measure smaller quantities of nutrients. For example, a serving of cereal might contain 10 mg of iron.
Micrograms (mcg) are even smaller units of measurement and are used to measure very trace amounts of nutrients. For example, a serving of cereal might contain 2 mcg of vitamin B12.
Kilocalories (kcal) are used to measure the energy content of a food item. For example, a serving of cereal might contain 150 kcal.
Daily values (%DV) are a way of comparing the nutrient content of a food item to the recommended daily intake of that nutrient. For example, a serving of cereal might contain 10% of the DV for iron.
In addition to the units of measurement listed above, you may also see other information on a food label, such as the serving size, servings per container, and the ingredients. The serving size is the amount of food that is considered to be a single serving. The servings per container is the number of servings in the entire package. The ingredients list all of the ingredients that are used to make the food item. daily values to assist consumers in making informed dietary choices.
Learn more about units of measurement here:
brainly.com/question/11943039
#SPJ11
-2(3y+3)=-4 find the value of 5y
Answers
Answer:
\(5y = - 2 - y\)
Step-by-step explanation:
\( - 2(3y + 3) = - 4\)
First, let's solve the brackets. Multiply 3y and 3 with -2 to get -6y -6.
\( - 6y - 6 = - 4\)
Now, send -6 to the other side.
\( - 6y = - 4 + 6\)
\( - 6y = 2\)
As we want to find out the value of 5y, we have to make -6y into 5y. To do that let's first change -6y into positive by multiplying the whole equation by -1.
\( - ( - 6y) = - (2)\)
\(6y = - 2\)
Next subtract y from each side to get 5y.
\(6y - y = - 2 - y\)
\(5y = - 2 - y\)
Viola! Here's the value of 5y.
Hope this helped!
The undergraduate grade point averages (UGPA) of students taking an admissions test in a recent year can be approximated by a normal distribution, as shown in the figure. (a) What is the minimum UGPA that would still place a student in the top 10% of UGPAs? (b) Between what two values does the middle 50% of the UGPAs lie?
Answers
a) The Corresponding minimum UGPA = 3.70
b) The range of middle 50% values will be: (3.28, 3.52).
What is z Score in Statistics?A measure of how many standard deviations below or above the population mean a raw score is called z score. It will be positive if the value lies above the mean and negative if it lies below the mean. It is also known as standard score. It indicates how many standard deviations an entity is, from the mean. In order to use a z-score, the mean μ and also the population standard deviation σ should be known. A z score helps to calculate the probability of a score occurring within a standard normal distribution. It also enables us to compare two scores that are from different samples.
a) z score corresponding to top 5% area = 1.645
Hence, Corresponding minimum UGPA = 3.40 + 1.645*0.18 = 3.70
b) z score corresponding to middle 50% area = -0.67, 0.67
Hence, The range of middle 50% values will be:
(3.40 - 0.67*0.18, 3.40 + 0.67*0.18)
(3.28, 3.52)
Learn more about z score at:
https://brainly.com/question/15016913
#SPJ4

Helppppppp!!!!!!!!??????

Answers
Answer:
5/10
Step-by-step explanation:
a school is purchasing graphing calculators for use in math classrooms. the graph shows a relationship between the number of graphing calculators purchased and the total cost
Answers
Answer:
there is no picture
Step-by-step explanation:
What is the answer?

Answers
how to change fraction in decimal example 108/30 change it in decimal
Answers
Answer:
You divide
Example:
- 5/10= 0,5
-108/30= 3,6
explanation: 108 divided by 30 is 3.6
What is the reason for Statement 3 of the two-column proof?
O Linear Pair Postulate
O Angle Addition Postulate
O Definition of complementary angles
Definition of angle
k
Given: mzJMK = 52"
m2KML = 38
Prove: ZJML is a right angle.
Statements
1. m/JMK = 52"
2 m/KML = 38"
3.
M
m/JMK+m/KML = m/JML
4.52 +38 = m/JML
5.90 = m/JML
6 /JML is a right angle
Reasons
Given
Given
Substitution
Property of Equality
Simplification
Definition of right
angle
Answers
The measure of ∠JMK =52° and ∠KML = 38°.
Three rays ML, MK, and MJ share an endpoint M. Ray MK forms a bisector as shown in the attached image and the bisector divides angle JML into two parts.
To Prove: is a right angle.
Proof:
Statements Reasons
1. m∠JMK = 52° Given
2. m∠KML = 38° Given
3. m∠JMK + m∠KML = m∠JML
The reason for statement 3 is Angle addition postulate. As angle JML is composed of 2 angles that is angle JMK and angle KML. So by adding the measures of angles JMK and KML, we will get the measure of angle JML which is referred as Angle addition postulate.
4. 52° + 38° =m∠JML Substitution property of equality
5. 90° = m∠JML Simplification
6. ∠JML is a right angle. Definition of right angle
To know more about angle check the below link:
https://brainly.com/question/25716982
#SPJ9

Find the value of x in the equation below. 15.4=x-13.9
Answers
Answer:
29.3
Step-by-step explanation: