Answers
Answer:
A {-3, -2, -1, 0, 1, 2, 3}
Answer:
A
Step-by-step explanation:
Rational numbers are whole numbers
Related Questions
A ball is launched from a 114.24-foot tall platform. The equation for
the ball's height hat time t seconds after launch is h (t)=-16f² +
59.2t+114.24, where his in feet. What is the maximum height the
ball achieves before landing?
Answers
To find the maximum height the ball achieves before landing, we need to find the vertex of the parabola represented by the equation h(t) = -16t^2 + 59.2t + 114.24. The vertex form of a parabola's equation is y = a(x-h)² + k where (h, k) is the coordinates of the vertex.
We can get the vertex form by completing the square of x term.
h(t) = -16t² + 59.2t + 114.24
h(t) = -16(t² - 3.68t) + 114.24 + 3.68t
h(t) = -16(t-0.92)² + 118.92
The maximum height is 118.92 ft which is k.
Uday Tahlan
Find the value of y (2y+5) (5x - 17) (3x-11)

Answers
Answer:
Hope it help, please give me BRAINLEST!
Step-by-step explanation:
2y + 5= |3y| + 3 = 3
x - 3= 2
\(\sqrt{x} + 2 = 2\)
5x - 17= -3x = 2
3^(x )= 4
3 cos^(2)(x) + 2 cos(x)- 1 = 0
3x-11= \(\sqrt{x}\) +3 = 8
2 = |3x|
x - 5= -3
Force f acts between a pair of charges, q1 and q2, separated by a distance d. for each of the statements, use the drop-down menus to express the new force in terms of f. q1 is halved, q2 is doubled, but the distance between the charges remains d. q1 and q2 are unchanged. the distance between the charges is doubled to 2d. q1 is doubled and q2 is tripled. the distance between the charges remains d.
Answers
The initial force between the two charges is given by:
\(F=k\frac{q_{1} q_{2} }{d^2}\)
where k is Coulomb's constant, q1 and q2 are the two charges, and d is their separation. Let's analyze now the other situations:
1. F
In this case, q1 is halved, q2 is doubled, but the distance between the charges remains d.
So, we have:
q'₁=q₁/2
q'₂=2q₂
d'=d
So, the new force is:
\(F'=k\frac{q'_{1}q'_{2} }{d^2} \\\\F'=k\frac{(\frac{q_{1} }{2})(\frac{q_{2} }{2}) }{d^2} \\\\F'=k\frac{q_{1}q_{2} }{d^2} =F\)
So the force has not changed.
2. F/4
In this case, q1 and q2 are unchanged. The distance between the charges is doubled to 2d.
So, we have:
q'₁=q₁
q'₂=q₂
d'=2d
So, the new force is:
\(\\F'=k\frac{q'_{1} q'_{2} }{d^2} \\\\F'=k\frac{q_{1}q_{2} }{2d^2} \\\\F'=\frac{1}{4} k\frac{q_{1}q_{2} }{d^2} \\\\F'=\frac{F}{4}\)
So the force has decreased by a factor of 4.
3. 6F
In this case, q1 is doubled and q2 is tripled. The distance between the charges remains d.
So, we have:
q'₁=2q₁
q'₂=3q₂
d'=d
So, the new force is:
\(F'=k\frac{q'_{1} q'_{2} }{d^2} \\\\F'=k\frac{2q_{1}3q_{2} }{d^2}\\ \\F'=6k\frac{q_{1}q_{2} }{d^2} =6F\)
So the force has increased by a factor of 6.
To know more about the distance between charges:
https://brainly.com/question/12288272
#SPJ4
Answer:
1. F
2. F/4
3. 6F
Solve for x.
(13x + 12)
Answers
Answer:
=13x+12
Step-by-step explanation:
Let U=f(P,V,T) be the internal energy of a gas that obeys the ideal gas law PV=nRT (n and r constant). Finda.dUdPv andb.dUdTv.
Answers
The dU/dT at constant P and V is simply nR/P.
According to the ideal gas law, PV = nRT, so we can write P = nRT/V. Using this relationship, we can express the internal energy U as a function of P, V, and T:
U = f(P,V,T) = f(nRT/V, V, T)
To find dU/dP at constant V and T, we can use the chain rule:
dU/dP = (∂U/∂P)V,T + (∂U/∂V)P,T(dP/dP)V,T + (∂U/∂T)P,V(dT/dP)V,T
Since V and T are being held constant, we can simplify the second and third terms to just 0:
dU/dP = (∂U/∂P)V,T
To find (∂U/∂P)V,T, we can differentiate f(nRT/V, V, T) with respect to P, keeping V and T constant:
(∂U/∂P)V,T = (∂f/∂P)nRT/V(-nRT/V²) = -nRT/V²
So, dU/dP at constant V and T is simply -nRT/V².
To find dU/dT at constant P and V, we can again use the chain rule:
dU/dT = (∂U/∂T)P,V + (∂U/∂V)P,T(dV/dT)P,V + (∂U/∂P)V,T(dP/dT)P,V
Since P and V are being held constant, we can simplify the third term to just 0:
dU/dT = (∂U/∂T)P,V + (∂U/∂V)P,T(dV/dT)P,V
To find (∂U/∂T)P,V, we can differentiate f(nRT/V, V, T) with respect to T, keeping P and V constant:
(∂U/∂T)P,V = (∂f/∂T)nRT/V(1) = nR/V
To find (∂U/∂V)P,T, we can differentiate f(nRT/V, V, T) with respect to V, keeping P and T constant:
(∂U/∂V)P,T = (∂f/∂V)nRT/V(-nRT/V²) + (∂f/∂V)V,T = nRT/V² - nRT/V² = 0
Since the ideal gas law shows that PV = nRT, we can write V = nRT/P. Using this relationship, we can simplify the second term of dU/dT to just:
dU/dT = (∂U/∂T)P,V = nR/P
So, dU/dT at constant P and V is simply nR/P.
For more such answers on ideal gas law
https://brainly.com/question/27870704
#SPJ11
a. To find dU/dPv, we need to differentiate U with respect to both P and V while treating T as a constant. Using the chain rule, we have:
dU/dPv = (∂U/∂P)v + (∂U/∂V)p * (dV/dP)v
Since U is a function of P, V, and T, we can express it as U(P,V,T). Using the ideal gas law, we substitute P = nRT/V into U:
U = f(P,V,T) = f(nRT/V, V, T)
Differentiating U with respect to P while treating V and T as constants, we get (∂U/∂P)v = -nRT/V².
Similarly, differentiating U with respect to V while treating P and T as constants, we get (∂U/∂V)p = nRT/V.
Hence, dU/dPv = -nRT/V² + nRT/V * (dV/dP)v.
b. To find dU/dTv, we differentiate U with respect to both T and V while treating P as a constant. Using the chain rule:
dU/dTv = (∂U/∂T)v + (∂U/∂V)t * (dV/dT)v
Differentiating U with respect to T while treating V and P as constants, we get (∂U/∂T)v = (∂f/∂T)v.
Similarly, differentiating U with respect to V while treating T and P as constants, we get (∂U/∂V)t = (∂f/∂V)t.
Hence, dU/dTv = (∂f/∂T)v + (∂f/∂V)t * (dV/dT)v.
Note: The specific form of the function f(P,V,T) is not provided, so we cannot determine the exact values of (∂f/∂T)v, (∂f/∂V)t, and (dV/dT)v without additional information.
Learn more about differentiate here: brainly.com/question/31962094
#SPJ11
Can you help me find x?
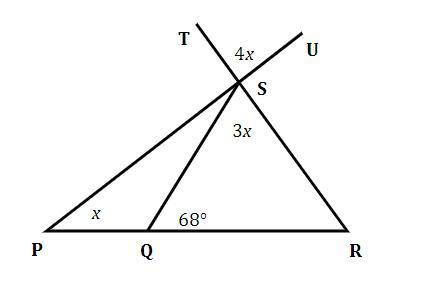
Answers
Answer:
x = 34 degrees
Step-by-step explanation:
angles TWU and PSR are vertical and equal
if TSU = 4x and QSR = x then PSQ = 3x
triangle PSQ has 180 degrees
we know the three interior angles of the triangle are:
x, x, 112
we found the 112 by subtracting 68 from 180 since PQR is a straight line which has 180 degrees
to find 'x' we do the following:
x + x + 112 = 180
2x + 112 = 180
2x = 68
x = 34
If Sam bakes cookies at a rate of 35 per hour, at a constant rate, how many cookies can she bake in 12 hours?
Answers
Answer:
420
Step-by-step explanation:
(Have a great day)!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!1!!!
In an effort to limit my calories, I have decided to only fill my ice cream to the top of the cone. The height of the cone is 458inches and the diameter is 178 inches. What is the volume of my ice cream?

Answers
Answer:
136 is the write ans ok
Step-by-step explanation:
by which your
i need help with this, no idea what i'm doing

Answers
Answer:
\(- 5 {p}^{2} {q}^{2} \)
Step-by-step explanation:
\( \frac{ {p}^{4}q }{ - 3 {p}^{3} } \times 15pq \\ \frac{ {p}^{4} q \times 15pq}{ - 3 {p}^{3} } \\ \frac{15 {p}^{(4 + 1)} {q}^{(1 + 1)} }{ - 3 {p}^{3} } \\ \frac{15 {p}^{5} {q}^{2} }{ - 3 {p}^{3} } \\ 15 {p}^{5} {q}^{2} \div - 3 {p}^{3} \\ (15 \div - 3)( {p}^{5 - 3} ) {q}^{2} \\ - 5 {p}^{2} {q}^{2} \)
Hope this helps you.
Please let me know if you have another questions :-)
A cross section is made by the intersection of a plane and a square pyramid at an angle either parallel or perpendicular to the base. The cross section can be which of these shapes
Answers
The cross section can be of these shapes square and triangle.
A cross-segment is a plane segment that is a segment of a three-dimensional item that is parallel to one among its planes of symmetry or perpendicular to considered one of its traces of symmetry.
An instance of a go-segment would be a circle. A cross-phase is seen whilst a 3-dimensional picture is reduce through a plane. within the case of the cone, a circular go-segment emerges while the cone is cut parallel with its base
The go-sectional region is the location of a 3-dimensional shape that is acquired whilst a three-dimensional object - inclusive of a cylinder - is sliced perpendicular to some designated axis at a point.
Learn more about cross section here https://brainly.com/question/2459472
#SPJ4
Protractor postulate: given any angle, we can express its measure as a unique ______________ number from 0 to 180 degrees.
Answers
Protractor postulate: given any angle, we can express its measure as a unique real number from 0 to 180 degrees.
The protractor postulate is a fundamental concept in geometry that establishes a way to measure angles using a protractor. According to this postulate, every angle can be uniquely represented by a real number between 0 and 180 degrees.
A protractor is a geometric tool with a semicircular shape and marked degrees along its edge. To measure an angle using a protractor, we align the center of the protractor with the vertex of the angle and the baseline of the protractor with one side of the angle. We then read the degree measure where the other side of the angle intersects the protractor.
The protractor is divided into 180 degrees, with 0 degrees being the starting point at the baseline of the protractor, and 180 degrees being at the opposite end of the baseline. By aligning the protractor with an angle, we can determine its measure as a real number within this range.
For example, if we measure an angle using a protractor and find that the other side intersects the protractor at 45 degrees, we can express the measure of the angle as 45 degrees. Similarly, if the intersection point is at 90 degrees, the angle measure would be 90 degrees. The protractor postulate guarantees that these angle measures are unique within the range of 0 to 180 degrees.
It is important to note that the protractor postulate assumes that angles can be measured using a protractor and that the measurement is accurate and reliable. The postulate provides a consistent and standardized way to assign a numerical value to an angle, allowing for precise communication and comparison of angles in geometric contexts.
In summary, the protractor postulate establishes that the measure of any angle can be expressed as a unique real number between 0 and 180 degrees. This concept is fundamental in geometry and allows for the measurement, comparison, and communication of angles using a protractor.
To learn more about angles click here:
brainly.com/question/22891563
#SPJ11
What is the procedure for quickly stopping a car with ABS
Answers
Answer:
Press down the brake firmly and smoothly. ...
Don't brake and swerve the car at the same time. ...
Avoid using your transmission for quick stops. ...
Focus on where you want to go, not what you want to avoid.
6700 dollars is placed in an account with an annual interest rate of 8.25%. How much will be in the account after 28 years, to the nearest cent?
Answers
Answer:
In 28 years, you will have $61,667.47
Step-by-step explanation:
pls write the steps u did

Answers
Answer:
They aren't parallel because the intersections with Via La Playa do not make equal alternate interior, alternate exterior angles, or corresponding angles.
Step-by-step explanation:
Angle a is 140 degrees since it and 40 degrees are supplementary. The angle supplementary to 130 degrees is 70 degrees. All of the vertical angles are congruent.
Guido tiene la cuarta parte de la edad de su padre Andrés y el triple de la edad de su hermano David. ¿Qué edad tiene cada uno, si sus edades suman 48 años?
Answers
Answer:
Guido tiene 9 años, Andrés tiene 36 años y David tiene 3 años.
Step-by-step explanation:
Con la información proporcionada, sabes que la edad de los tres suma 48, lo que se puede expresar como:
x+y+z=48, donde:
x es la edad de Guido
y es la edad de Andrés
z es la edad de David
Además, de acuerdo al enunciado puedes decir que la edad del papá Andrés es cuatro veces la edad de Guido y que la edad de David es la tercera parte de la edad de Guido y puedes escribir las siguientes ecuaciones:
y=4x
z=x/3
Ahora puedes reemplazar estas dos ecuaciones en la primera y despejar x:
x+4x+x/3=48
5x+x/3=48
16x/3=48
16x=48*3
16x=144
x=144/16
x=9
Después, puedes reemplazar el valor de x en y=4x para encontrar el valor de y:
y=4x
y=4*(9)
y=36
Finalmente, debes reemplazar el valor de x en z=x/3 para encontrar el valor de z:
z=x/3
z=9/3
z=3
De acuerdo a esto, Guido tiene 9 años, Andrés tiene 36 años y David tiene 3 años.
11.Use the Pythagorean Theorem to find the
unknown side length of the right triangle.
Need answer ASAP

Answers
Answer:
26 meters
Step-by-step explanation:
a^2 + b^2 = c^2
10^2 + 24^2 = c^2
100 + 576 = c^2
676 = c^2 (square root both sides)
26 = c
Answer:
Kathete +Kathete=Hypothenuse
24^2+10^2
576+100
=676
=26
unknown side lenght=26
Can I maybe be Brainliest.
have a nice day
Best regards
Can anyone help me find w

Answers
A city bus driver says the number of miles to gallons of gas for his bus can be represented by the ratio 12 : 4. How many miles can the bus travel on 1 gallon of gas?
Answers
Answer:
The bus can travel 3 miles with 1 gallon of gas
Step-by-step explanation:
Hope this helps!
A group of students examines a Bohr model of an atom with 8 valence electrons. What conclusion can the students make about the chemical reactivity of the element?
The element is extremely reactive because the outer energy level is full.
The element is nonreactive because the outer energy level is full.
The element is somewhat reactive because the outer energy level is full.
Additional information is necessary to determine reactivity.
Answers
the correct statement is: The element is nonreactive because the outer energy level is full.
Due to the element's entire outer energy level, it is possible to infer that the element with 8 valence electrons has no chemical reactivity.
Atoms tend to be most stable when their outer energy level is full, as this configuration satisfies the octet rule. When the outer energy level is complete, atoms are less likely to gain or lose electrons to form chemical bonds with other atoms. This reduces their reactivity.
Therefore, the correct statement is:
The element is nonreactive because the outer energy level is full.
Learn more about Bohr model here
https://brainly.com/question/3964366
#SPJ4
Evaluate:
\(\bf{\sum^{10}_{n-1}\:8(\cfrac{1}{4})^{n-1}\)
\(\bf{\overline{\underline{\overline{\underline{Please\:show\;work!}}}}\)
Answers
Let S be the given sum, so
\(S = 8 + 8 \left(\dfrac14\right) + 8 \left(\dfrac14\right)^2 + \cdots + 8 \left(\dfrac14\right)^9\)
\(\displaystyle S = 8 \left(1 + \dfrac14 + \frac1{4^2} + \cdots + \frac1{4^9}\right)\)
Multiply both sides by 1/4.
\(\displaystyle \frac S4 = 8 \left(\frac14 + \frac1{4^2} + \frac1{4^3} + \cdots + \frac1{4^{10}}\right)\)
Subtract this from S to eliminate all the but the first term in S and the last term in S/4 :
\(\displaystyle S - \frac S4 = 8 \left(1 - \frac1{4^{10}}\right)\)
Solve for S :
\(\displaystyle \frac{3S}4 = 8 \left(1 - \frac1{4^{10}}\right)\)
\(\displaystyle S = \frac{32}3 \left(1 - \frac1{4^{10}}\right) = \boxed{\frac{349,525}{32,768}}\)
a poll shows that of all voters approve of the mayor's work. on three separate occasions a pollster selects a voter at random. what is the probability that on exactly one of these three occasions the voter approves of the mayor's work?

Answers
The probability that on exactly one of these three occasions the voter approves of the mayor's work is given as follows:
0.189 = 18.9%.
What is the binomial distribution formula?The mass probability formula, giving the probability of x successes, is of:
\(P(X = x) = C_{n,x}.p^{x}.(1-p)^{n-x}\)
\(C_{n,x} = \frac{n!}{x!(n-x)!}\)
The parameters are given by:
n is the number of trials of the experiment.p is the probability of a success on a single trial of the experiment.The values of these parameters in the context of this problem are given as follows:
n = 3, p = 0.7.
Then the probability of exactly one success is calculated as follows:
P(X = 1) = 3!/(1!2!) x 0.7 x (0.3)² = 0.189 = 18.9%.
Missing InformationThe proportion of voters that approve the mayor's work is of 70%.
More can be learned about the binomial distribution at https://brainly.com/question/24756209
#SPJ1
WILL MARK BRAINLIEST!!!! PLZ HELP!!!! URGENT!!! Which of the following is a two-way conditional relative frequency table for gender?



Answers
Answer:
The second one
Step-by-step explanation:
It mentions that the total is 100%, which is the total for all the event sin the probability,
Hope this helps
1, 6, 36, 216, 1296...
Write the recursive form of the sequence above.
Answers
Tn = 6Tn-1 is the recursive form of the sequence
Finding the recursive function of a geometric sequenceA geometric sequence is determined by its common ratio. The standard recursive formula is expressed as:
Tn = rTn-1
Determine the common ratio of the sequence
r = 6/1 = 36/6
r = 6
Substitute the common ratio into the formula to have:
Tn = 6Tn-1
Hence the recursive form of the sequence above is Tn = 6Tn-1
Learn more on recursive form here: https://brainly.com/question/1275192
#SPJ1
10) Consider the contour plot of a function f(x,y) given below. (a) The points labeled A,B,C,D are the only critical points of f. Classify each of the points as a local maximum, local minimum, or saddle. Briefly justify your answer. (b) Give a unit vector in the direction of ∇f(−1,0). Briefly justify your answer.
Answers
to determine the unit vector in the direction of ∇f(-1,0), we need to calculate the gradient vector at (-1,0), normalize it, and obtain the resulting unit vector.
(a)
- Point A: Local minimum. In the vicinity of point A, the contour lines are concentric and decrease in value, indicating a bowl-like shape. This suggests that point A is a local minimum, as the function attains its lowest value in that region.
- Point B: Saddle point. At point B, the contour lines intersect and change direction. This behavior indicates that the function neither reaches a local minimum nor a local maximum, but instead has a saddle-like shape.
- Point C: Local maximum. The contour lines around point C are concentric and increase in value, suggesting a peak-like shape. Thus, point C is a local maximum since the function attains its highest value in the vicinity of C.
- Point D: Saddle point. Similar to point B, point D exhibits contour lines that intersect and change direction, indicating a saddle-like shape.
(b) The unit vector in the direction of ∇f(-1,0) can be obtained by calculating the gradient vector at (-1,0) and normalizing it to have unit length. The gradient vector ∇f represents the direction of steepest increase of the function.
Let's denote the function as f(x,y). To find the gradient vector, we compute the partial derivatives of f with respect to x and y, respectively: ∂f/∂x and ∂f/∂y.
Once we have the gradient vector ∇f(-1,0)=[∂f/∂x(-1,0), ∂f/∂y(-1,0)], we normalize it by dividing it by its magnitude ||∇f(-1,0)||.
to determine the unit vector in the direction of ∇f(-1,0), we need to calculate the gradient vector at (-1,0), normalize it, and obtain the resulting unit vector.
To know more about unit vector follow the link:
https://brainly.com/question/31046870
#SPJ11
please help asap!!!look at the box

Answers
Answer:
y
Step-by-step explanation:
Please teach me how to solve this!!!

Answers
What is the value of x?
Enter your answer in the box. x = °

Answers
Answer:
x=80
Step-by-step explanation:
my god this should be easy and I thought I was a bit slow
is a parallelogram. is the midpoint of . and trisect .
Let ⃗⃗⃗⃗⃗ = ⃗ and ⃗⃗⃗⃗⃗ = . Show your work on the diagram as well.

Answers
Answer:
option 6b):) is correct
the maker of a cell phone screen protector would like to estimate the proportion
Answers
Answer:
The maker of a cell phone screen protector would like to estimate the proportion of customers who file a warranty claim. To do so, they select a random sample of 200 customers and determine that the 96% confidence interval for the true proportion of customers who file a warranty claim to be 0.15 to 0.28.
Step-by-step explanation:
question 3 in the analyze stage of the data life cycle, what might a data analyst do? select all that apply.
Answers
In the analyze stage of the data life cycle, the data analyst will use the spreadsheet to aggregate the data and use the formulas to perform the calculations
The data life cycle is defined as the time period that that data exist in you system. Usually the data management experts finds the six or more stages in the data life cycle
The data analyst is the person who use the interpreted data and analyze the data in order to solve the problems
The analyze stage is the one of the main stages of the data life cycle. In the stage data analyst will use the spreadsheet to aggregate the data in the system and use the formulas to perform the calculations in the system
Therefore, the data analyst will use the spreadsheet to aggregate the data and use the formulas to perform the calculations
Learn more about data life cycle here
brainly.com/question/28039445
#SPJ4
