Write the standard form of the equation of the circle with the given characteristics.
Center: (-3, 4); Radius: 3
4. [-/6 Points]
DETAILS
LARPCALC11 1.3.027.
Find the slope of the line passing through the pair of points. (If an answer does not exist, enter DNE.)
(-8, -12), (1, 24)
Answers
The standard form of the equation of the circle is (x+3)²+(y-4)²=9 .
The slope of the line is
A circle is defined as the locus of a moving point which is always equidistant from a given point.
This given point is called the center of the circle.The distance between the center and the circumference of the circle is the radius if the circle.The standard form of a circle is given by the equation : (x-x₁)²+(y-y₁)²=r² , where (x₁,y₁) is a point on the circle and r is the radius.The given point on the circle is (-3, 4) and the radius is 3 units.
Hence the standard form of the equation of the circle is :
(x-{-3})²+(y-4)²=3²
or, (x+3)²+(y-4)²=9
Let the two given two points as A(-8, -12) and B(1, 24).
Now the slope of the line AB can be calculated by the formula:
\(m=\frac{y_2-y_1}{x_2-x_1}.\)
Now let us put the given values in the equation to calculate slope:
\(m_{AB}=\frac{24-(-12)}{1-(-8)}\\\\or, m_{AB}=\frac{36}{9} \\\\or, m_{AB}=4\)
Hence the slope of the line is 4.
To learn more about circle visit:
https://brainly.com/question/23799314
#SPJ9
Related Questions
ASAP Find the slope of The ordered pairs (0,-17),(2,13)
Answers
Answer:
m=15
Step-by-step explanation:
Answer:
1 5
Step-by-step explanation:
Find the value of x in the figure below

Answers
Answer:
37
Step-by-step explanation:
Both the x and the 53 add up to 90 so you subtract 53 from 90 and you get 37
The rate constant for first-order degradation of a N2O2 in a solution (1.0 mg/ml) at 40°C
is 0.00351 hr-1, activation energy is 2000 cal/mol, and R = 1.987 cal/mol/degree.
Calculate
a. The rate constant for first-order degradation of N2O2 at room temperature (25°C).
Answers
The 0.0035 hr⁻¹ first-order degradation rate constant in the 40 °C, 1.0 mg/ml solution, where R = 1.987 cal/mol/degree and 2,000 cal/mol activation energy indicates;
a. The rate constant for first-order degradation of N₂O₂ at room temperature is approximately 4.12595 × 10⁻² hr⁻¹
What is a first-order reaction?A first order reaction is one that has a rate that varies linearly with the concentration of one reactant
The given parameters are;
Temperature of the solution = 40°C
T₁ = 40 °C + 273.15 = 313.15 K
The rate constant, K₁ = 0.00351 hr⁻¹
Concentration of the solution = 1.0 mg/ml
Activation energy, Eₐ = 2000 Cal/mol
R = 1.987 cal/mol/degree
The formula by which the rate constant can be found is presented as follows;
\(log\dfrac{k_2}{k_1} =\dfrac{E_a}{2.303\times R} \times \dfrac{T_2-T_1}{T_1\cdot T_2}\)
a. Room temperature = 25 °C
T₂ = 25 °C + 273.15 = 298.15 K
Therefore;
\(log\dfrac{k_2}{0.00351} =\dfrac{2000}{2.303\times 1.987} \times \dfrac{313.15-298.15}{313.15 \times 298.15}\)
\(k_2=0.00351 \times 10^{\left(\dfrac{2000}{2.303\times 1.987} \times \dfrac{313.15-298.15}{313.15 \times 298.15}\right)} \approx 4.12595\times 10^{-2}\)
The rate constant for first-order degradation of N₂O₂ at room temperature is k₂ ≈ 4.12595 × 10⁻² hr⁻¹Learn more about the rate constant for first-order degradation here:
https://brainly.com/question/14595397
#SPJ1
Gena wants to estimate the quotient of –21.87 divided by 4.79. Which expression shows the estimate using front-end estimation?
Negative 21 divided by 4
Negative 21 divided by 5
Negative 20 divided by 4
Negative 20 divided by 5
Answers
Answer:
Negative 21 divided by 5
Step-by-step explanation:
since we use rounding off
What annual rate of interest is required to double an investment in 9 years
Answers
Answer:
8%
Step-by-step explanation:
If an investment scheme promises an 8% annual compounded rate of return, it will take approximately (72 / 8) = 9 years to double the invested money
PLEASE HELP circle a has area 500in squared. the diameter of circle b is three times the diameter of circle a. esitmate the area of circle b.
Answers
Answer:
Step-by-step explanation:
r and d are the radius and diameter of a, respectively.
area of a = πr² = 500 in²
r = √500/√π = 10√5/√π
d = 20√5/√π
radius of b = 3r = 30√5/√π
area of b = π(30√5/√π)² = π(4500/π) = 4500 in²
96. Sara took a test that had 24 questions on it. She got 4/6 of them correct. How many questions did she get correct? A 12 B. 15 16 D 25
Answers
Answer:
16
Step-by-step explanation:
the answer is 16
An equation is shown below.
2-3x = 8
Which of the following shows a model of the equation and the value of x that makes the equation true?
How would you solve it
Answers
Answer:
Step-by-step explanation:
Suppose we have a random sample of size n = 5 from a continuous uniform distribution on the interval [0, 1]. Find the probability that the third largest observation in the
sample is less than 0.7.
Answers
The probability that the third largest observation in the sample is less than 0.7 is 0.2401 = 24.01%.
How do we calculate?The sample size n = 5,
Therefore the order statistics will be represented as X₁, X₂, X₃, X₄, and X₅.
Probability that X₃ is less than 0.7:
Since X₃ is the third largest observation = (X₁ and X₂) < 0.7.
The probability that X₃ is less than 0.7 is (0.7)² = 0.49.
The Probability that X₁ and X₂ < or equal to 0.7 is found as:.
The probability that both X₁ and X₂ are less than or equal to 0.7 is (0.7)² = 0.49 because in a continuous uniform distribution, the probability of any single observation being less than 0.7 is 0.7 - 0 = 0.7.
We then get the product of both cases:
Probability = 0.49 * 0.49 = 0.2401
Learn more about Probability at:
https://brainly.com/question/24756209
#SPJ1
la quinta parte de 20
Answers
Answer:
4
Step-by-step explanation:
20/5 = 4
Which error was hardest to find?
Answers
Answer:
May I ask what error are we talking ab?
Step-by-step explanation:
How many pairs of parallel faces does this shape have?

Answers
Answer: one
Step-by-step explanation:
parallel faces are the ones that are opposite to each other.
Answer:
one
Step-by-step explanation:
there is only one pair of parallel faces.
When 58,511 is divided by 21, the quotient is 2,786 R ___.
Numerical Answers Expected!
Answer for Blank
Answers
If you take the answer, 2786 and multiple by 21
You get 58,506
Which if you subtract from 58,511 you would get 5
Which is r
Find two numbers the quotient is between. Then estimate the quotient. Estimate. 34 ÷ 3 The quotient is between and . The estimate for the quotient is .
Answers
Answer:
34 / 3 = 11.33333.....
the quotient is between 11 and 12
The estimate is 11
Using Symmetry and the Empirical Rule A standardized test is approximately normally distributed with a mean score of 78 and a standard deviation of 3. Use the symmetry of the normal curve and apply the Empirical rule to answer the questions. Make a sketch of the curve to help you determine the areas. Note: You must use the Empirical Rule values 68 % , 95 % , and 99.7% in your computations. The computer calculator will not give you the correct answer. What percent of students scored less than 78? 75 % de What percent of students scored between 75 and 78? What percent of students scored between 72 and 81? Se % What percent of students scored between 69 and 75?
Answers
a. About 50% of the students scored less than 78.
b. Approximately 68% of the students scored between 75 and 78.
c. Approximately 95% of the students scored between 72 and 81.
d. Approximately 99.7% of the students scored between 69 and 75.
a) The normal curve is symmetrical, meaning that the area to the left of the mean is equal to the area to the right of the mean. Since the mean score is 78, 50% of the students scored above 78 and 50% scored below 78.
b) To apply the Empirical Rule, we will assume that 68% of the data falls within one standard deviation of the mean, which in this case is 3 points. So, 68% of the students scored between 75 and 81.
c) The percentage of students who scored between 72 and 81 can be found by subtracting the area to the left of 72 from the area to the left of 81. Using the Empirical Rule, we know that 95% of the observations are within two standard deviations of the mean, so the area between 72 and 81 is equal to 95%.
d) The percentage of students who scored between 69 and 75 can be found by subtracting the area to the left of 69 from the area to the left of 75. Using the Empirical Rule, we know that 99.7% of the observations are within three standard deviations of the mean, so the area between 69 and 75 is equal to approximately 99.7%.
Read more about normal distribution:
brainly.com/question/4079902
#SPJ4
need help i dont understand

Answers
Answer:
Step-by-step explanation:
it would be "c" because the question asks for the number that makes the inequality true
4x≤ x+3
the graph in c is saying that every number that is 1 or less than 1 makes the inequality true. so lets take -7 as an example
4 x -7=-28
-28+3=-25
-25 is greater than -28 so the inequality is true. the reason why graph d doesnt work is because if we plug in 2 into the equation, then 4x =8 and x+3=5
5 is not greater than 8 so it doesnt work
Sally buys a shirt of 5.00. New York's sales tax is 8.200. What is the final price?
Show your work and explain or I report and delete answer.
Answers
Answer: $5.40
Sales tax is a price you add to every 100 cents purchased.
Sally purchased a shirt of 5.00, and New York's sales tax is 8.200.
So, we multiply 8 by 5. We get the number 40, right?
You now add 0.40 to 5.00.
The answer totals at 5.40 because 5.00 + 0.40 = 5.40.
Hope this helps you out!
Television sizes are described by length of their diagonal measure. What would be the listen sizes of tv shown (to the nearest whole inch)

Answers
Step 1
Draw the triangle involving the diagonal of the TV
Pyramid A and Pyramid B are similar. Pyramid A has a volume of 648m° and Pyramid B has a volume of 1029m?. What is the ratio of the surface areas of Pyramid A to Pyramid B?
Answers
The ratio of the surface area of Pyramid A to Pyramid B is: 36/49
We have the information from the question is:
Pyramid A and Pyramid B are similar.
The volume of Pyramid A is 648 \(m^3\)
The volume of Pyramid B is 1029 \(m^3\)
To find the ratio of the surface areas of Pyramid A to Pyramid B.
Now, As we know that:
If two solids are similar, then the ratio of their volumes is equal to the cube
of the ratio of their corresponding linear measures.
\(\frac{Vol of A}{Vol of B} =(\frac{a}{b} )^3 =\frac{684}{1029}=\frac{216}{343}\\ \\ \frac{a}{b}=\frac{6}{7}\)
If two solids are similar, then the n ratio of their surface areas is equal to the square of the ratio of their corresponding linear measures.
Surface area of A/ Surface area of B \(=(\frac{a}{b} )^2=\frac{36}{49}\)
So, the ratio of the surface area of Pyramid A to Pyramid B is: 36/49
Learn more about Volume of pyramid at:
https://brainly.com/question/17615619
#SPJ1
Fiad the sample variance and standard deviation.
21, 10, 3, 7, 11
Answers
Answer:
SD = 5.987, Var(X) = 35.85
Step-by-step explanation:
Apply the standard deviation formula, remembering that n represents the sample size. Then, just take the square of the standard deviation to obtain the variance.
Hope this helps!

The population of Boomtown is 475,000 and is increasing at a rate of 3.75% each year. When will the population exceed 1 million people (to the nearest year)
Using the Growth and Decay method.
Answers
Answer:
21 years
Step-by-step explanation:
can someone help me solve for x and show the work please

Answers
Answer:
D. -10
Step-by-step explanation:
The sum of a triangle = 180°, therefore,
64° + 63° + x + 63 = 180°
Add like terms
x + 190° = 180°
Subtract 190 from each side
x + 190 - 190 = 180 - 190
x = -10
Alana is working on creating a scale
drawing of her mom's bakery store. She
has set her scale 1/2 in = 6 ft. If the
bakery store is 33 ft long, then how many
inches should it be on the scale drawing?
length - 33 feet
Answers
Answer:
2 3/4
Step-by-step explanation:
every 6 ft is equivelent to a half of an inch. So if you take 33 ft and divide it by 6 to find how many 6 ft are in the bakery's length you will get 5.5 so you know there are 5 and a half, halves in the bakery's length. so you have 5 halves, which is 2 and a half, plus you have a half of a half, so 1/4. adding this all together you have 2 and 3/4 for your total scaled drawing.
In the figure below, two secants are drawn to a circle from exterior point V.
Suppose that VZ=30, VW=45, and VX=22.5. Find XY.
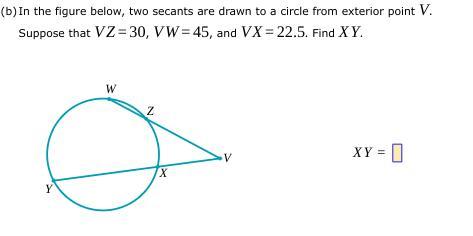
Answers
The value of secant XY is 37.5 units.
How to find the value of secant XY?The Secant-Secant Theorem states that "if two secant segments which share an endpoint outside of the circle, the product of one secant segment and its external segment is equal to the product of the other secant segment and its external segment".
Using the theorem above, we can say:
VZ * VW = VX * VY
30 * 45 = 22.5 * VY
22.5VY = 1350
VY = 1350/22.5
VY = 60 units
Since VY = VX + XY. We have:
60 = 22.5 + XY
XY = 60 - 22.5
XY = 37.5 units
Learn more about Secant-Secant Theorem on:
https://brainly.com/question/30242636
#SPJ1
The statement of cash flows is an optional financial statement.
True
Or False
Answers
Answer:
The statement of cash flows is an optional financial statement. The statement of cash flows shows the effects on cash of a company's operating, investing, and financing activities. The statement of cash flows reports a firm's major sources of cash receipts and major uses of cash payments for a period.
For any nonnegative real number c,(_/c)^2=_____.

Answers
Answer:
A. c
Step-by-step explanation:
We don't really need the restriction. One part of the definition of
√c
is that when it's squared, we'll get
(√c)^2=c
For a non negative real number c, (√c)² is equivalent to c
Exponential expressionsGiven the following exponential expression
(√c)²
The square root of can also be expressed as:
√c = \(c^{1/2}\)
Substitute
(√c)² = \((c^{1/2})^2\)
Multiply the exponents to have;
(√c)² = c
Hence for a non negative real number c, (√c)² is equivalent to c
Learn more on exponent here: https://brainly.com/question/11975096
#SPJ6
Implicit differentiation Please help

Answers

Answer:
\(y''(-1) =8\)
General Formulas and Concepts:
Pre-Algebra
Order of Operations: BPEMDAS
BracketsParenthesisExponentsMultiplicationDivisionAdditionSubtractionLeft to RightEquality Properties
Algebra I
FactoringCalculus
Implicit Differentiation
The derivative of a constant is equal to 0
Basic Power Rule:
f(x) = cxⁿf’(x) = c·nxⁿ⁻¹Product Rule: \(\frac{d}{dx} [f(x)g(x)]=f'(x)g(x) + g'(x)f(x)\)
Chain Rule: \(\frac{d}{dx}[f(g(x))] =f'(g(x)) \cdot g'(x)\)
Quotient Rule: \(\frac{d}{dx} [\frac{f(x)}{g(x)} ]=\frac{g(x)f'(x)-g'(x)f(x)}{g^2(x)}\)
Step-by-step explanation:
Step 1: Define
-xy - 2y = -4
Rate of change of the tangent line at point (-1, 4)
Step 2: Differentiate Pt. 1
Find 1st Derivative
Implicit Differentiation [Product Rule/Basic Power Rule]: \(-y - xy' - 2y' = 0\)[Algebra] Isolate y' terms: \(-xy' - 2y' = y\)[Algebra] Factor y': \(y'(-x - 2) = y\)[Algebra] Isolate y': \(y' = \frac{y}{-x-2}\)[Algebra] Rewrite: \(y' = \frac{-y}{x+2}\)Step 3: Find y
Define equation: \(-xy - 2y = -4\)Factor y: \(y(-x - 2) = -4\)Isolate y: \(y = \frac{-4}{-x-2}\)Simplify: \(y = \frac{4}{x+2}\)Step 4: Rewrite 1st Derivative
[Algebra] Substitute in y: \(y' = \frac{-\frac{4}{x+2} }{x+2}\)[Algebra] Simplify: \(y' = \frac{-4}{(x+2)^2}\)Step 5: Differentiate Pt. 2
Find 2nd Derivative
Differentiate [Quotient Rule/Basic Power Rule]: \(y'' = \frac{0(x+2)^2 - 8 \cdot 2(x + 2) \cdot 1}{[(x + 2)^2]^2}\)[Derivative] Simplify: \(y'' = \frac{8}{(x+2)^3}\)Step 6: Find Slope at Given Point
[Algebra] Substitute in x: \(y''(-1) = \frac{8}{(-1+2)^3}\)[Algebra] Evaluate: \(y''(-1) =8\)I need help on this question!!!!!!!!!!!!

Answers
Answer:
Step-by-step explanation:
m<9= 126
m<4= 36
m<11= 36
m<14= 144
what is the value of -3 to the second power +(-4+7)(2)
Answers
Answer:
15
Step-by-step explanation:
=> (-3)²+(-4+7)(2)
=> 9+(3)(2)
=> 9+6
=> 15
The perimeter of a triangle is 17 inches. One side is 5 inches, and another is 4 inches. What is the length of the third side?
Answers
Answer:
8inches
Step-by-step explanation:
Length of the third side = perimeter - ( side1 + side2 )
= 17 - (5+4)
= 17 - 9
= 8 inches