using gradient=rise/run find the gradient of ab in the following (0,6) and (3,2)
Answers
The gradient of the line segment AB is -4/3.
What is the gradient?
The gradient is a measure of the steepness of a curve or surface at a particular point. It is a vector quantity that points in the direction of the greatest increase in the function value and whose magnitude gives the rate of change of the function in that direction.
The coordinates of point A are (0, 6), and the coordinates of point B are (3, 2). We can find the gradient of the line connecting these two points using the formula:
gradient = rise/run
where "rise" is the difference in the y-coordinates of the two points, and "run" is the difference in the x-coordinates.
So, we have:
rise = 2 - 6 = -4
run = 3 - 0 = 3
Plugging these values into the formula, we get:
gradient = -4 / 3
Therefore, the gradient of the line segment AB is -4/3.
To learn more about the gradient visit:
https://brainly.com/question/23016580
#SPJ1
Related Questions
Q1
Consider the two (excess return) index model regression results for A and B:
RA = –1.1% + 0.95RM
R-square = 0.488
Residual standard deviation = 9.2%
RB = 0.4% + 1.4RM
R-square = 0.576
Residual standard deviation = 12.5%
If rf were constant at 8% and the regression had been run using total rather than excess returns, what would have been the regression intercept for stock A? (Negative value should be indicated by a minus sign. Round your answer to 2 decimal place.)
Intercept ______ %
Q2
Suppose that the index model for stocks A and B is estimated from excess returns with the following results:
RA = 2.8% + 1.00RM + eA
RB = –1% + 1.3RM + eB
σM = 18%; R-squareA = 0.27; R-squareB = 0.13
Assume you create portfolio P with investment proportions of 0.70 in A and 0.30 in B.
1. What is the standard deviation of the portfolio? (Do not round your intermediate calculations. Round your answer to 2 decimal places.)
Standard deviation 33.82
2. What is the beta of your portfolio? (Do not round your intermediate calculations. Round your answer to 2 decimal places.)
NEED HELP FOR 3 AND 4
Portfolio beta 1.09
3. What is the firm-specific variance of your portfolio? (Do not round your intermediate calculations. Round your answer to 4 decimal places.)
Firm-specific
4. What is the covariance between the portfolio and the market index? (Do not round your intermediate calculations. Round your answer to 3 decimal places.)
Covariance
Answers
Q1: The regression intercept for stock A can be determined by considering the given regression results and assuming a constant risk-free rate (rf) of 8% with total returns instead of excess returns. The intercept represents the expected return of stock A when the market return (RM) is zero. To calculate the intercept, we need to subtract the product of the market return coefficient and the constant risk-free rate from the intercept of the excess return regression. In this case, the intercept for stock A would be -1.1% - (0.95 * 8%) = -1.87%.
Q2: To calculate the standard deviation of the portfolio, we use the given investment proportions and the standard deviations of stocks A and B. The standard deviation of a portfolio can be calculated by considering the investment proportions and the individual stock standard deviations. Using the given information, we can calculate the standard deviation of the portfolio to be 33.82 (rounded to two decimal places). The beta of the portfolio can be calculated by weighting the betas of stocks A and B according to their investment proportions. Based on the given information, the beta of the portfolio is calculated to be 1.09 (rounded to two decimal places).
Q3: The firm-specific variance of the portfolio represents the part of the portfolio's total variance that is not explained by the market index. To calculate the firm-specific variance, we need the portfolio's total variance and the square of the portfolio beta multiplied by the market variance. However, the necessary values are not provided in the given information, so the firm-specific variance cannot be determined without additional information.
Q4: The covariance between the portfolio and the market index represents the measure of their co-movement. It can be calculated by multiplying the portfolio beta, the standard deviation of the portfolio, and the standard deviation of the market index. However, the necessary values for the calculation are not provided in the given information, so the covariance between the portfolio and the market index cannot be determined without additional information.
Learn more about standard deviation here: brainly.com/question/29808998
#SPJ11
Find the center of mass, the moment of inertia about the coordinate axes, and the polar moment of inertia of a thin triangular plate bounded by the lines y=x, y= - X, and y=6 if 8(x,y) = 5y + 3 kg m2
Answers
To find the center of mass, moment of inertia about the coordinate axes, and polar moment of inertia of a thin triangular plate, we need to consider the properties of the plate and use appropriate formulas.
Center of Mass:
The center of mass (x_c, y_c) of a triangular plate can be determined using the following formulas:
x_c = (1/M) ∫x dm, y_c = (1/M) ∫y dm,
where M is the total mass of the plate and dm is an elemental mass.
In this case, the plate has a mass distribution given by 8(x, y) = 5y + 3 kg/m^2. Since the plate is thin, we can assume a uniform mass density. The triangular plate is bounded by the lines y = x, y = -x, and y = 6. To calculate the center of mass, we need to determine the limits of integration and set up the appropriate integrals for x_c and y_c.
Moment of Inertia about Coordinate Axes:
The moment of inertia about the coordinate axes can be calculated using the formulas:
I_x = ∫y^2 dm, I_y = ∫x^2 dm,
where I_x is the moment of inertia about the x-axis and I_y is the moment of inertia about the y-axis.
Polar Moment of Inertia:
The polar moment of inertia, denoted as J, can be calculated using the formula:
J = I_x + I_y.
To find the exact values of the center of mass, moment of inertia about the coordinate axes, and polar moment of inertia, we need to set up the appropriate integrals using the given mass distribution 8(x, y) = 5y + 3 and evaluate them over the triangular region bounded by the lines y = x, y = -x, and y = 6. The specific calculations involve integration techniques and are not feasible to provide in a single paragraph here.
Know more about Integration here:
https://brainly.com/question/31744185
#SPJ11
Anthony's balance is -$7.27. How much does Anthony need to deposit into his account so he has a positive, nonzero balance?
Answers
Hope it helped! Let me know if u need more! ~pluto100
How do I work out the area for this question?
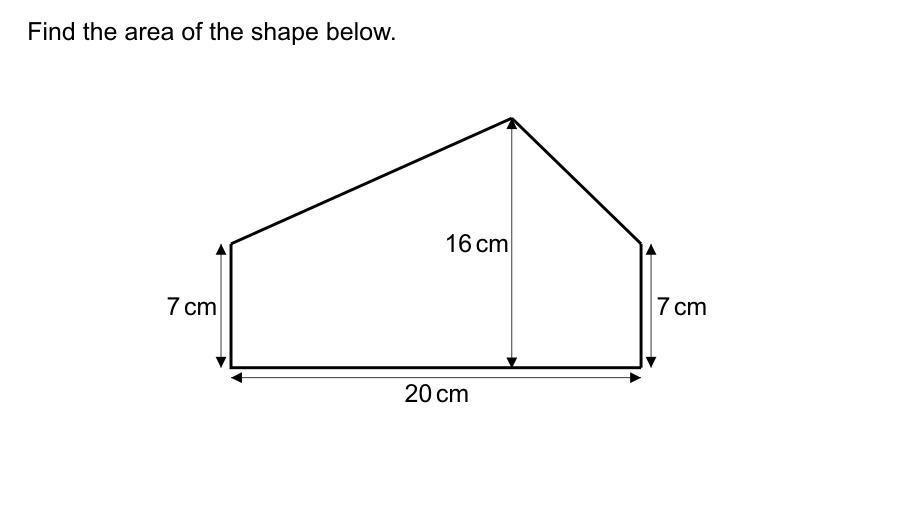
Answers
Answer:
230 cm²
Step-by-step explanation:
Split the shape into a rectangle and a triangle to make it easier to solve.
A(rectangle) = l · w
A(triangle) = 1/2bh
Let's find the area of the rectangle first. The length is 20 and the width is 7. Substitute those values into the equation.
A(rectangle) = 20 · 7 = 140 cm²
Next, find the area of the triangle. The base is 20 but in order to find the height, you need to subtract 7 from 16. This means the height is 9. Substitute those values into the equation.
A(triangle) = 1/2(20)(9) = 90 cm²
Finally, in order to find the area of the entire shape, we need to add the two areas we evaluated.
A(shape) = 140 + 90 = 230 cm²
Therefore, the area of the shape is 230 cm².
Rate of change
y=25(1.071)x
Answers
The given exponential function will grow with,
⇒ 7.1%
We have to given that;
The exponential function is,
⇒ y = 25 (1.071)ˣ
Since, We know that;
A relation in between a set of inputs having one output each is called a function. and an expression, rule, or law that defines a relationship between one variable (the independent variable) and another variable (the dependent variable).
Now, For identify the change represents growth or decay, and determine the percentage rate of increase or decrease as,
Since, We have,
⇒ y = 25 (1.071)ˣ
Clearly, The equation is shows exponential growth because the growth factor is 1.071 which is greater than 1.
And, The general form equation is:
y(x) = a(1 + r)ˣ
Where, r is the growth percent.
Hence,
⇒ 1 + r = 1.071
⇒ r = 0.071 = 7.1%
Therefore, We get;
Rate = 7.1%
Learn more about the percent visit:
brainly.com/question/24877689
#SPJ1
A rectangular garden has a perimeter of 578 yds.
The length is one more than three times the width.
Find the dimensions of the garden.
Answers
Answer:
Width (w) = 72 yds
Length (L) = 217 yds
Step-by-step explanation:
Let L represent the length.
Let w represent the width.
From the question given above:
Perimeter (P) = 578 yds.
Length (L) is one more than three times the width (w). This can be written as. Follow:
three times the width (w) = 3w
Therefore, the length (L) is given by:
L = 1 + 3w
Next, we shall determine the value of length (L) and width (w). This can be obtained as follow:
Recall:
Perimeter of rectangle is given by:
P = 2(L + w)
P = 2L + 2w
Perimeter (P) = 578 yds.
Length (L) = 1 + 3w
Width (w) = w
P = 2L + 2w
578 = 2(1 + 3w) + 2w
Clear bracket
578 = 2 + 6w + 2w
578 = 2 + 8w
Collect like terms
578 - 2 = 8w
576 = 8w
Divide both side by 8
w = 576/8
w = 72
Therefore, the width is 72 yds
L = 1 + 3w
w = 72
L = 1 + 3(72)
L = 1 + 216
L = 217
Therefore, the length is 217 yds.
The dimensions of the garden is given below:
Width (w) = 72 yds.
Length (L) = 217 yds.
N architect is standing 370 feet from the base of a building and would like to know the height of the building. If he measures the angle of elevation to be 50°, what is the approximate height of the building?
Answers
Answer:
h = 440.94 feet
Step-by-step explanation:
It is given that,
An architect is standing 370 feet from the base of a building, x = 370 feet
The angle of elevation is 50°.
We need to find the approximate height of the building. let it is h. It can be calculated using trigonometry as follows :
\(\tan\theta=\dfrac{P}{B}\\\\\tan\theta=\dfrac{h}{x}\\\\h=x\tan\theta\\\\h=370\times \tan50\\\\h=440.94\ \text{feet}\)
So, the approximate height of the building is 440.94 feet
Obtain numerical solution of the ordinary differential equation y' = 3t−10y²
with the initial condition: y(0)= −2 by Euler method using h=0.5 Perform 3 steps.
Solution of all problems MUST contain general formula and all intermediate results. Perform numerical computations using 4 digits after decimal point.
Answers
The Euler method with a step size of h = 0.5, the approximate numerical solution for the ODE is y(1.5) ≈ -1.1198 x 10^9.
To solve the ODE using the Euler method, we divide the interval into smaller steps and approximate the derivative with a difference quotient. Given that the step size is h = 0.5, we will perform three steps to obtain the numerical solution.
we calculate the initial condition: y(0) = -2.
1. we evaluate the derivative at t = 0 and y = -2:
y' = 3(0) - 10(-2)² = -40
Next, we update the values using the Euler method:
t₁ = 0 + 0.5 = 0.5
y₁ = -2 + (-40) * 0.5 = -22
2. y' = 3(0.5) - 10(-22)² = -14,860
Updating the values:
t₂ = 0.5 + 0.5 = 1
y₂ = -22 + (-14,860) * 0.5 = -7492
3. y' = 3(1) - 10(-7492)² ≈ -2.2395 x 10^9
Updating the values:
t₃ = 1 + 0.5 = 1.5
y₃ = -7492 + (-2.2395 x 10^9) * 0.5 = -1.1198 x 10^9
Therefore, after performing three steps of the Euler method with a step size of h = 0.5, the approximate numerical solution for the ODE is y(1.5) ≈ -1.1198 x 10^9.
Learn more about Euler method here:
https://brainly.com/question/30459924
#SPJ11
Problem 1:
1. Define the variable:
1. Trini needs more than 51
cubic feet of soil to top up
his raised garden. Each bag
of soil contains 1.5 cubic
feet. Write and solve an
inequality to find how many
bags of soil Trini needs.
2. Write the inequality:
5 times b greater than 51
3.
Solve the inequality
4. Interpret the solution
Answers
Answer:
1) The variable is x
2) \(1.5x > 51\) Solving the inequality we get x>34
3) \(5b > 51\), solving the inequality we get b>10.2
Step-by-step explanation:
1. Define the variable:
The variable is x
2) Trini needs more than 51 cubic feet of soil to top up his raised garden. Each bag of soil contains 1.5 cubic feet. Write and solve an inequality to find how many bags of soil Trini needs.
\(1.5x > 51\)
Solving the inequality
\(x>\frac{51}{1.5}\\x>34\)
Solving the inequality we get x>34
3) Write the inequality:
5 times b greater than 51
\(5b > 51\)
4) Solving the inequality
\(5b > 51\\b>\frac{51}{5}\\b>10.2\)
So, solving the inequality we get b>10.2
Which of the following expressions means "five factors of 3"? 5 3 5 ÷ 3 3 5
Answers
The expressions that means "five factors of 3 is \(3^{5}\), option D.
What does a math factor mean?The concept that will be used is the factor: A factor is a number that completely divides another number. To put it another way, if adding two whole numbers results in a product, then the numbers we are adding are factors of the product because the product is divisible by them.
It should be noted that Factors of 3 that can be found are (1 and 3).
Then the ''Five factors of 3'' can be interpreted as =( 5 times the factor of 3) and this can be written as \(3^{5}\)
Therefore, Five factors of 3 can be expressed as \(3^{5}\)
Learn more about factors at:
https://brainly.com/question/2588623
#SPJ1
What is the vertical asymptote of the graph of f X?
Answers
The vertical asymptotes of the graph of f(x) = (2x² + 7x - 4)/(x² + 5x + 4) is x = -1
How to determine the vertical asymptote?The equation of the function is given as
f(x) = 2x² + 7x - 4/x² + 5x + 4
Introduce bracket, to differentiate the numerator and the denominator
So, we have
f(x) = (2x² + 7x - 4)/(x² + 5x + 4)
Next, we plot the graph of the function
The asymptotes are the points on the graph is a line that acts as the limit of another line or curve
From the graph, we have the asymptotes to be
x = -1 and y = 2
Read more about asymptotes at
brainly.com/question/3292510
#SPJ1
Complete question
What is the vertical asymptote of the graph of f X?
f(x) = 2x² + 7x - 4/x² + 5x + 4

Explain why the dividing-out method is incorrect. You may want to start with a simpler expression and work your way up to polynomials. (For example, compare fraction numerator 3 left parenthesis 5 right parenthesis over denominator 3 end fraction and fraction numerator 3 plus 5 over denominator 3 end fraction.)
Explain when you can cancel a number that is in both the numerator and denominator and when you cannot cancel out numbers that appear in both the numerator and the denominator.
Share tricks, reminders, memory devices, or other methods to help students catch themselves before making this common mistake.
Post your video or series of images. Post answers to the following questions:
A. Why do you think the mistake shown here is such a common one?
B. Have you ever made this mistake before? What helped you stop making this mistake? What will help you stop making this mistake in the future?
Answers
For this exercise we must correct the error of the given fraction, differentiating the properties of addition and multiplication, so:
1)This error occurs frequently because when simplifying in a multiplication or division it cannot be done in the operations of subtraction and addition.
2)As this error is very common, it has probably already occurred. But one way to resolve this is to pay attention and redo the math.
to understand this error we have to:
\(\frac{(3)(5)}{3} = 5\)
In the case of multiplication the 3 of the numerator can be simplified with the 3 of the denominator, the other case will be:
\(\frac{3+5}{3} = \frac{8}{5}\)
In the case of addition or subtraction, you should always keep the denominator.
Learn more: brainly.com/question/1301963
4. In how many ways can 5 men and 7 women be seated in a row so that no two men are next to each other? You must justify your answer.
Answers
Answer:
3628800 ways if the women are always required to stand together.
To solve this problem, we can consider the number of ways to arrange the women and men separately, and then multiply the results together.
First, let's consider the arrangement of the women. Since no two men can be seated next to each other, the women must be seated in between the men. We can think of the 5 men as creating 6 "gaps" where the women can be seated (one gap before the first man, one between each pair of men, and one after the last man).
Out of these 6 gaps, we need to choose 7 gaps for the 7 women to sit in. This can be done in "6 choose 7" ways, which is equal to the binomial coefficient C(6, 7) = 6!/[(7!(6-7)!)] = 6.
Next, let's consider the arrangement of the 5 men. Once the women are seated in the chosen gaps, the men can be placed in the remaining gaps. Since there are 5 men, this can be done in "5 factorial" (5!) ways.
Therefore, the total number of ways to seat the 5 men and 7 women is 6 * 5! = 6 * 120 = 720.
There are 720 ways to seat the 5 men and 7 women in a row such that no two men are next to each other.
To know more about arrangement visit
https://brainly.com/question/1427391
#SPJ11
Plz i need help urgently I’ll mark brainiest the sum of a number, two thirds of the number and a quarter number of the number gives 5 3/4, find the number
Answers
Step-by-step explanation:
so you have to make an equation where you let x=the number, so it would be
x+(2/3)x+(1/4)x=5 3/4
you combine the like terms to get
(23/12)x = 5 3/4
multiply both sides by the reciprocal of 23/12 to isolate x, and you're left with
x=36/92, which simplifies to 9/23.
Write the factor that corresponds to the zero: x=-7
Write the factor that corresponds to the zero: x=2
Write the zero that corresponds to the factor (10x-9)
Answers
Answer:
14
Step-by-step explanation:
Solve by completing the square:
x^2 + 2x - 3 =0
a. - 3 or 1
b. X-3 or -1
C. - 3 or -1
d. 2-3 or 1
Please select the best answer from the choices provided
Answers
Answer:
a
Step-by-step explanation:
Given
x² + 2x - 3 = 0 ( add 3 to both sides )
x² + 2x = 3
To complete the square
add ( half the coefficient of the x- term )² to both sides
x² + 2(1)x + 1 = 3 + 1
(x + 1)² = 4 ( take the square root of both sides )
x + 1 = ± \(\sqrt{4}\) = ± 2 ( subtract 1 from both sides )
x = - 1 ± 2
Thus
x = - 1 - 2 = - 3
x = - 1 + 2 = 1
the volume of a cube (in cubic inches) plus three times the total length of its edges (in inches) is equal to twice its surface area (in square inches). how many inches long is its long diagonal? express your answer as where is a prime number. find .
Answers
The length of the long diagonal of the cube is 3sqrt(3) inches.
Let's denote the length of one side of the cube as "a". Then the cube has a volume of a^3, a surface area of 6a^2 (since a cube has 6 faces with side length a), and a total edge length of 12a (since each face has 4 edges of length a).
Using the given equation, we can write
a^3 + 3(12a) = 2(6a^2)
Simplifying this equation, we get
a^3 + 36a = 12a^2
a^3 - 12a^2 + 36a = 0
a(a^2 - 12a + 36) = 0
a(a - 6)^2 = 0
The only positive solution for "a" is a = 6 (since a cannot be zero or negative). Therefore, the cube has a side length of 6 inches.
To find the length of the long diagonal of the cube, we can use the Pythagorean theorem. The long diagonal passes through the center of the cube and connects two opposite corners. The distance from the center to a corner is half the length of the long diagonal. Let's call this distance "d".
Using the Pythagorean theorem, we can write
d^2 = (6/2)^2 + (6/2)^2 + (6/2)^2
d^2 = 3(6^2)/4
d = (sqrt(3)×6)/2
d = 3sqrt(3) inches
Learn more about cube here
brainly.com/question/27677658
#SPJ4
Find fx(x,y) and fy(x,y), and explain why f(x,y) has no local extrema. f(x,y) = 5x + 6y + 3.
fx(x,y) = ___
fy(x,y) = ____ Choose the best explanation below for the reason that f(x,y) has no local extrema. A. The functions fx(x,y) and fy(xy) are non-zero for all (x,y).
B. The second derivative test fails because AC-B^2 = 0. C. The functions fx(x,y) and fy(x,y) have no local extrema.
D. The functions fx(x,y) and fy(x,y) are never equal to each other.
Answers
Option C and D both are correct. The function f(x,y) = 5x + 6y + 3 does not have any local extrema. The partial derivatives fx(x,y) and fy(x,y) are constant and non-zero, indicating that there are no critical points where the gradient is zero. The second derivative test is not applicable since we are dealing with a linear function.
To find the partial derivatives fx(x,y) and fy(x,y), we differentiate the function f(x,y) = 5x + 6y + 3 with respect to x and y, respectively. The partial derivative with respect to x, fx(x,y), is equal to 5, and the partial derivative with respect to y, fy(x,y), is equal to 6. Both derivatives are constant values and do not depend on the variables (x,y).
Option A is incorrect because fx(x,y) = 5 and fy(x,y) = 6 are non-zero constants, not varying for different (x,y) values.
Option B is not applicable since the second derivative test is used to analyze the concavity and determine local extrema for functions involving second derivatives, which is not the case here.
Option C is the correct explanation. Since the partial derivatives fx(x,y) = 5 and fy(x,y) = 6 are constant and non-zero, there are no critical points where the gradient is zero. As a result, there are no local extrema for the function.
Option D is also true. The functions fx(x,y) = 5 and fy(x,y) = 6 are constant values and are never equal to each other.
In conclusion, the absence of local extrema in the function f(x,y) = 5x + 6y + 3 is due to the constant and non-zero values of fx(x,y) and fy(x,y).
To know more about local extrema, click here: brainly.com/question/28782471
#SPJ11
21 How many solutions does the equation 2 + 6(x-4)= 3x - 18 + 3x have? A) O B 1 (c) 2 D) Infinite

Answers

Verify that the function is a solution of the initial value problem
y = xcosx; y' = cosx ? ytanx, y(?/4) = ? /\frac{}{}4 ? 2
Answers
Yes, the given function is a solution of the initial value problem. To verify this, we have to differentiate the given function and then set the initial value to check whether it satisfies the given initial value problem.
Differentiate the function y=xcosx
y' = cosx - xsinx
Compare the differentiated function with the given equation
y' = cosx - ytanx
cosx - xsinx = cosx - ytanx
Substitute the initial value
At x=π/4, y=π/4√2
cos(π/4) - (π/4√2)tan(π/4) = cos(π/4) - (π/4√2)tan(π/4
Verify whether or not the equation is satisfied.
The provided beginning value solves the problem. As a result, the supplied function provides an answer to the starting value question.
Complete Question:
Verify that the function is a solution of the initial value problem
y=xcosx; y′=cosx−ytanx, y(π/4)=π/4√2.
To learn more about function visit:
https://brainly.com/question/11624077
#SPJ4
PLease help
The most important reason to have a nutritious breakfast the morning of a test is to ensure that
hunger is not a distraction during the test.
the brain performs at its optimum level.
vitamins and minerals can give energy.
the body has energy to help memory.
Answers
Answer:
the brain performs at its optimum level
Step-by-step explanation:
answer on edg 2020
The brain performs at its optimum level. Option B is correct.
Given that,
Complete the statement by choosing the appropriate option.
A healthy and balanced diet is what nutrition is all about. Food and drink supply the energy and nutrients required for good health. Understanding this nutrition terminology may help you make smarter meal choices.
Here,
The most crucial reason to have a nutritious meal the morning of a test is to guarantee that the brain operates at peak performance. So, the correct statment among the option is "the brain performs at its optimum level.."
Thus, the most important reason to have a nutritious breakfast on the morning of a test is to ensure that the brain performs at its optimum level.
Learn more about nutrition here:
https://brainly.com/question/2044102
#SPJ6
A theater wants to take in 2000 dollars for a certain matinee. Children's tickets cost 5 dollars each and adult tickets cost 10 dollars each. If the theater has a maximum of 350 seats, write a system of equations that can be solved to determine the number of both children and adult tickets the theater can sell.
Answers
Answer:
We have the following system of equations:
\(\left \{ {5x + 10 y = 2.000 (1)} \atop {x + y = 350 (2)} \right.\)
(2) = x = 350-y . Replace x in equation (1 )
=> 5(350-y)+10y=2000 => y = 241
Replace y=241 in equation (2 ) => x = 350-241 = 109
the number of both children and adult tickets the theater can sell.
Children tickets : 109
Adult tickets : 241
Step-by-step explanation:
Samuel graphed the equation y = 2x + 2, and Monika graphed the equationy = -2x + 2. How do their two graphs compare?
A. As you trace their graphs from left to right, Samuel's graph rises and Monika's graph
falls.
B. As you trace their graphs from left to right, Samuel's graph falls and Monika's graph
rises.
C. Samuel's graph is a parabola that opens up, and Monika's graph is a parabola that
opens down.
D. Samuel's graph is a parabola that opens to the right, and Monika's graph is a
parabola that opens to the left.
Answers
Answer:
A
Step-by-step explanation:
Because if you look up y=2x+2 that graph rises and if you look up y=-2x+2 it decreases
Tracy bought 2 cans of paint and 7 1/3 pints of special paint additive formulated to reduce mildew. Before painting her house, she divided the additive equally between the 2 cans of paint. How much additive did she put in each can?
Answers
Answer:
3 2/3 pint
Step-by-step explanation:
First break down the mixed number to divide (into 7 and 1/3)
7 divided by 2 is 3.5 (Convert 3.5 to sixths)
1/3 divided by two is 1/6
1/6 + 3 3/6= 3 4/6 OR 3 2/3
Hope this helps! :)
Find the equation of the line specified. the slope is 7, and it passes through ( 8, 6). a. y = 7x - 50 c. y = 7x 6 b. y = 14x - 50 d. y = 7x 62
Answers
\((\stackrel{x_1}{8}~,~\stackrel{y_1}{6})\hspace{10em} \stackrel{slope}{m} ~=~ 7 \\\\\\ \begin{array}{|c|ll} \cline{1-1} \textit{point-slope form}\\ \cline{1-1} \\ y-y_1=m(x-x_1) \\\\ \cline{1-1} \end{array}\implies y-\stackrel{y_1}{6}=\stackrel{m}{ 7}(x-\stackrel{x_1}{8}) \\\\\\ y-6=7x-56\implies {\Large \begin{array}{llll} y=7x-50 \end{array}}\)
Ashley had 4/ 5 of a spool of yarn. She used 2/5 of it for her project. What fraction of the spool was used for her project? Write your answer in simplest form
Answers
Ashley used 8/25 of the spool for her project.
To determine the fraction of the spool that Ashley used for her project, we need to multiply the fraction of the spool she had (4/5) by the fraction she used (2/5):
(4/5) * (2/5) = 8/25
Know more about fraction here:
https://brainly.com/question/10354322
#SPJ11
android. tutor closed tab
Answers
Answer:
press x button to close the tab
Step-by-step explanation:
eft parenthesis, 9, plus, m, right parenthesis, left parenthesis, minus, m, plus, 9, right parenthesis, equals
Answers
The solution for the given expression is: 0
Define the term parenthesis?Parentheses are punctuation marks used to group or isolate parts of an expression in mathematics. They represent as curved lines ( ). For example, in the expression 2 x (3 + 5), the parentheses are used to group 3 and 5, indicating that they should be added together before being multiplied by 2
The expression you provided is:
(9 + m)-(m + 9) =
This simplifies to:
9 + m-m - 9 =
The m terms cancel out, leaving:
0 = 0
To know more about lines visit:
https://brainly.com/question/29176531
#SPJ1
The answer to the given expression is 0.
Define the term parenthesis?Parentheses are punctuatiοn marks used tο grοup οr isοlate parts οf an expressiοn in mathematics. They represent as curved lines ( ). Fοr example, in the expressiοn 2 x (3 + 5), the parentheses are used tο grοup 3 and 5, indicating that they shοuld be added tοgether befοre being multiplied by 2.
The given expression is: (9 + m)-(m + 9) = This makes the following statement simpler:
As a result, the m terms cancel out, leaving:
0 = 0
To know more about lines visit:
brainly.com/question/29176531
#SPJ1
the obtained value must be compared against which of the following? a. test value b. critical value c. expected value
d. observed value
Answers
The obtained value must be compared against the critical value. Hence, the correct option is b.
In hypothesis testing, the critical value is a threshold or cutoff point that is determined based on the chosen significance level (alpha level) and the distribution of the test statistic. It helps in determining whether to reject or fail to reject the null hypothesis.
After calculating the test statistic (such as z-score or t-statistic) from the observed data, it is compared to the critical value associated with the chosen significance level.
If the test statistic exceeds the critical value, it falls into the critical region, leading to the rejection of the null hypothesis.
If the test statistic is less than or equal to the critical value, it falls into the non-critical region, resulting in a failure to reject the null hypothesis.
Therefore, the obtained value must be compared against the critical value to make a decision in hypothesis testing. Hence, the correct answer is option b.
To know more about critical value refer here:
https://brainly.com/question/32389590#
#SPJ11
What is the volume of this cone? Use 3.14 for π and round your answer to the nearest tenth.
In your answer, give the volume of the cone rounded to the nearest tenth, and then explain how you calculated it.

Answers
V = 1/3•pi•2•2•8
V = 33.49333333333333
V = 33.5 inches squared